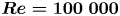 $\boldsymbol{Re=100}\ \boldsymbol{000}$
$\boldsymbol{Re=100}\ \boldsymbol{000}$Published online by Cambridge University Press: 20 December 2022
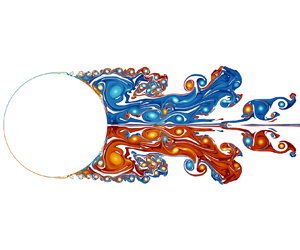
Direct numerical simulations of the flow past an impulsively started cylinder at high Reynolds numbers (25k–100k) reveal an intriguing portrait of unsteady separation. Vorticity generation and vortex shedding entails a cascade of separation events on the cylinder surface that are reminiscent of Kelvin–Helmholtz instabilities. Primary vortices roll up along the cylinder surface as a result of instabilities of the initially attached vortex sheets, followed by vortex eruptions, creation of secondary vorticity and formation of dipole structures that are subsequently ejected from the surface of the cylinder. We analyse the vortical structures and their relationship to the forces experienced by the cylinder. This striking cascade of vortex instabilities may serve as reference for reduced-order models of flow separation and as guide for flow control of separated flows at high Reynolds numbers.