Hostname: page-component-8448b6f56d-m8qmq Total loading time: 0 Render date: 2024-04-16T17:06:20.329Z Has data issue: false hasContentIssue false
  • English
  • Français

Analysis of the forward and backward in time pair-separation probability density functions for inertial particles in isotropic turbulence

Published online by Cambridge University Press:  29 September 2017

Andrew D. Bragg Open the ORCID record for Andrew D. Bragg [Opens in a new window]
Andrew D. Bragg*
Affiliation:
Department of Civil and Environmental Engineering, Duke University, Durham, North Carolina, USA
*
†Email address for correspondence: andrew.bragg@duke.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper we investigate, using theory and direct numerical simulations (DNS), the forward in time (FIT) and backward in time (BIT) probability density functions (PDFs) of the separation of inertial particle pairs in isotropic turbulence. In agreement with our earlier study (Bragg et al., Phys. Fluids, vol. 28, 2016, 013305), where we compared the FIT and BIT mean-square separations, we find that inertial particles separate much faster BIT than FIT, with the strength of the irreversibility depending upon the final/initial separation of the particle pair and their Stokes number $St$. However, we also find that the irreversibility shows up in subtle ways in the behaviour of the full PDF that it does not in the mean-square separation. In the theory, we derive new predictions, including a prediction for the BIT/FIT PDF for 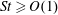$St\geqslant O(1)$, and for final/initial separations in the dissipation regime. The prediction shows how caustics in the particle relative velocities in the dissipation range affect the scaling of the pair-separation PDF, leading to a PDF with an algebraically decaying tail. The predicted functional behaviour of the PDFs is universal, in that it does not depend upon the level of intermittency in the underlying turbulence. We also analyse the pair-separation PDFs for fluid particles at short times, and construct theoretical predictions using the multifractal formalism to describe the fluid relative velocity distributions. The theoretical and numerical results both suggest that the extreme events in the inertial particle-pair dispersion at the small scales are dominated by their non-local interaction with the turbulent velocity field, rather than due to the strong dissipation range intermittency of the turbulence itself. In fact, our theoretical results predict that for final/initial separations in the dissipation range, when 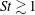$St\gtrsim 1$, the tails of the pair-separation PDFs decay faster as the Taylor Reynolds number $Re_{\unicode[STIX]{x1D706}}$ is increased, the opposite of what would be expected for fluid particles.

JFM classification

Multiphase and Particle-laden Flows: Multiphase and Particle-laden Flows Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 830 , 10 November 2017 , pp. 63 - 92
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Batchelor, G. K. 1952a Diffusion in a field of homogeneous turbulence II. The relative motion of particles. Proc. Camb. Phil. Soc. 48, 345–362.CrossRefGoogle Scholar
Batchelor, G. K. 1952b The effect of homogeneous turbulence on material lines and surfaces. Proc. R. Soc. Lond. A 213, 349–366.Google Scholar
Bec, J., Biferale, L., Cencini, M., Lanotte, A. S. & Toschi, F. 2010a Intermittency in the velocity distribution of heavy particles in turbulence. J. Fluid Mech. 646, 527–536.CrossRefGoogle Scholar
Bec, J., Biferale, L., Lanotte, A. S., Scagliarini, A. & Toschi, F. 2010b Turbulent pair dispersion of inertial particles. J. Fluid Mech. 645, 497–528.Google Scholar
Benzi, R., Biferale, L., Paladin, G., Vulpiani, A. & Vergassola, M. 1991 Multifractality in the statistics of the velocity gradients in turbulence. Phys. Rev. Lett. 67, 2299–2302.Google Scholar
Berg, J., Lüthi, B., Mann, J. & Ott, S. 2006 Backwards and forwards relative dispersion in turbulent flow: an experimental investigation. Phys. Rev. E 74, 016304.Google ScholarPubMed
Biferale, L., Boffetta, G., Celani, A., Devenish, B. J., Lanotte, A. & Toschi, F. 2005 Lagrangian statistics of particle pairs in homogeneous isotropic turbulence. Phys. Fluids 17, 115101.Google Scholar
Biferale, L., Lanotte, A. S., Scatamacchia, R. & Toschi, F. 2014 Intermittency in the relative separations of tracers and of heavy particles in turbulent flows. J. Fluid Mech. 757, 550–572.CrossRefGoogle Scholar
Bitane, R., Homann, H. & Bec, J. 2013 Geometry and violent events in turbulent pair dispersion. J. Turbul. 14 (2), 23–45.CrossRefGoogle Scholar
Boffetta, G., Mazzino, A. & Vulpiani, A. 2008 Twenty-five years of multifractals in fully developed turbulence: a tribute to Giovanni Paladin. J. Phys. A 41 (36), 363001.Google Scholar
Boffetta, G. & Sokolov, I. M. 2002a Relative dispersion in fully developed turbulence: the Richardson law and intermittency corrections. Phys. Rev. Lett. 88, 094501.CrossRefGoogle ScholarPubMed
Boffetta, G. & Sokolov, I. M. 2002b Statistics of two-particle dispersion in two-dimensional turbulence. Phys. Fluids 14, 3224–3232.CrossRefGoogle Scholar
Bragg, A. D. & Collins, L. R. 2014a New insights from comparing statistical theories for inertial particles in turbulence: I. Spatial distribution of particles. New J. Phys. 16, 055013.Google Scholar
Bragg, A. D. & Collins, L. R. 2014b New insights from comparing statistical theories for inertial particles in turbulence: II. Relative velocities of particles. New J. Phys. 16, 055014.Google Scholar
Bragg, A. D., Ireland, P. J. & Collins, L. R. 2016 Forward and backward in time dispersion of fluid and inertial particles in isotropic turbulence. Phys. Fluids 28 (1), 013305.Google Scholar
Bragg, A. D. 2017 Developments and difficulties in predicting the relative velocities of inertial particles at the small-scales of turbulence. Phys. Fluids 29 (4), 043301.Google Scholar
Bragg, A. D., Ireland, P. J. & Collins, L. R. 2015a Mechanisms for the clustering of inertial particles in the inertial range of isotropic turbulence. Phys. Rev. E 92, 023029.Google ScholarPubMed
Bragg, A. D., Ireland, P. J. & Collins, L. R. 2015b On the relationship between the non-local clustering mechanism and preferential concentration. J. Fluid Mech. 780, 327–343.Google Scholar
Buaria, D., Sawford, B. L. & Yeung, P. K. 2015 Characteristics of backward and forward two-particle relative dispersion in turbulence at different Reynolds numbers. Phys. Fluids 27 (10).Google Scholar
Buaria, D., Yeung, P. K. & Sawford, B. L. 2016 A Lagrangian study of turbulent mixing: forward and backward dispersion of molecular trajectories in isotropic turbulence. J. Fluid Mech. 799, 352–382.Google Scholar
Chevillard, L., Castaing, B., Arneodo, A., Lvque, E., Pinton, J.-F. & Roux, S. G. 2012 A phenomenological theory of Eulerian and Lagrangian velocity fluctuations in turbulent flows. C. R. Physique 13 (9), 899–928.Google Scholar
Computational and Information Systems Laboratory2012 Yellowstone: IBM iDataPlex System (University Community Computing) http://n2t.net/ark:/85065/d7wd3xhc.Google Scholar
De Lillo, F., Cencini, M., Durham, W. M., Barry, M., Stocker, R., Climent, E. & Boffetta, G. 2014 Turbulent fluid acceleration generates clusters of gyrotactic microorganisms. Phys. Rev. Lett. 112, 044502.Google Scholar
Devenish, B. J., Bartello, P., Brenguier, J.-L., Collins, L. R., Grabowski, W. W., Ijzermans, R. H. A., Malinowski, S. P., Reeks, M. W., Vassilicos, J. C., Wang, L.-P. et al. 2012 Droplet growth in warm turbulent clouds. Q. J. R. Meteorol. Soc. 138, 1401–1429.CrossRefGoogle Scholar
Falkovich, G. & Frishman, A. 2013 Single flow snapshot reveals the future and the past of pairs of particles in turbulence. Phys. Rev. Lett. 110, 214502.Google Scholar
Falkovich, G., Gawedzki, K. & Vergassola, M. 2001 Particles and fields in fluid turbulence. Rev. Mod. Phys. 73, 913–975.CrossRefGoogle Scholar
Falkovich, G., Xu, H., Pumir, A., Bodenschatz, E., Biferale, L., Boffetta, G., Lanotte, A. S., Toschi, F.& for Turbulence Research, International Collaboration 2012 On Lagrangian single-particle statistics. Phys. Fluids 24 (5), 055102.Google Scholar
Folch, A., Costa, A. & Basart, S. 2012 Validation of the {FALL3D} ash dispersion model using observations of the 2010 Eyjafjallajökull volcanic ash clouds. Atmos. Environ. 48, 165–183; Volcanic ash over Europe during the eruption of Eyjafjallajökull on Iceland, April–May 2010.CrossRefGoogle Scholar
Fouxon, I. & Horvai, P. 2008 Separation of heavy particles in turbulence. Phys. Rev. Lett. 100, 040601.Google Scholar
Fox, R. O. 2012 Large-eddy-simulation tools for multiphase flows. Annu. Rev. Fluid Mech. 44 (1), 47–76.Google Scholar
Frisch, U. 1995 Turbulence: The Legacy of A. N. Kolmogorov. Cambridge University Press.CrossRefGoogle Scholar
Gustavsson, K. & Mehlig, B. 2011 Distribution of relative velocities in turbulent aerosols. Phys. Rev. E 84, 045304.Google ScholarPubMed
Gustavsson, K. & Mehlig, B. 2014 Relative velocities of inertial particles in turbulent aerosols. J. Turbul. 15 (1), 34–69.CrossRefGoogle Scholar
Heydel, F., Cunze, S., Bernhardt-Römermann, M. & Tackenberg, O. 2014 Long-distance seed dispersal by wind: disentangling the effects of species traits, vegetation types, vertical turbulence and wind speed. Ecol. Res. 29 (4), 641–651.CrossRefGoogle Scholar
Ireland, P. J., Bragg, A. D. & Collins, L. R. 2016 The effect of Reynolds number on inertial particle dynamics in isotropic turbulence. Part 1. Simulations without gravitational effects. J. Fluid Mech. 796, 617–658.Google Scholar
Jucha, J., Xu, H., Pumir, A. & Bodenschatz, E. 2014 Time-reversal-symmetry breaking in turbulence. Phys. Rev. Lett. 113, 054501.Google Scholar
Kolmogorov, A. N. 1962 A refinement of previous hypotheses concerning the local structure of turbulence in a viscous incompressible fluid at high Reynolds number. J. Fluid Mech. 13, 82–85.CrossRefGoogle Scholar
Maxey, M. R. 1987 The gravitational settling of aerosol particles in homogeneous turbulence and random flow fields. J. Fluid Mech. 174, 441–465.CrossRefGoogle Scholar
Ni, R. & Xia, K. Q. 2013 Experimental investigation of pair dispersion with small initial separation in convective turbulent flows. Phys. Rev. E 87, 063006.Google ScholarPubMed
Ouellette, N. T., Xu, H., Bourgoin, M. & Bodenschatz, E. 2006 An experimental study of turbulent relative dispersion models. New J. Phys. 8, 109.CrossRefGoogle Scholar
Pan, L. & Padoan, P. 2010 Relative velocity of inertial particles in turbulent flows. J. Fluid Mech. 661, 73–107.CrossRefGoogle Scholar
Perrin, V. E. & Jonker, H. J. J. 2015 Relative velocity distribution of inertial particles in turbulence: a numerical study. Phys. Rev. E 92, 043022.Google ScholarPubMed
Pumir, A., Xu, H., Bodenschatz, E. & Grauer, R. 2016 Single-particle motion and vortex stretching in three-dimensional turbulent flows. Phys. Rev. Lett. 116, 124502.Google Scholar
Richardson, L. F. 1922 Weather Prediction by Numerical Process. Cambridge University Press.Google Scholar
Richardson, L. F. 1926 Atmospheric diffusion shown on a distance-neighbour graph. Proc. R. Soc. Lond. Ser. A 110, 709–737.Google Scholar
Salazar, J. P. L. C. & Collins, L. R. 2009 Two-particle dispersion in isotropic turbulent flows. Annu. Rev. Fluid Mech. 41, 405–432.Google Scholar
Sawford, B. L., Yeung, P.-K. & Borgas, M. S. 2005 Comparison of backwards and forwards relative dispersion in turbulence. Phys. Fluids 17, 095109.Google Scholar
Scatamacchia, R., Biferle, L. & Toschi, F. 2012 Extreme events in the dispersions of two neighboring particles under the influence of fluid turbulence. Phys. Rev. Lett. 109, 144501.CrossRefGoogle ScholarPubMed
Wilkinson, M. & Mehlig, B. 2005 Caustics in turbulent aerosols. Europhys. Lett. 71, 186–192.CrossRefGoogle Scholar
Wilkinson, M., Mehlig, B. & Bezuglyy, V. 2006 Caustic activation of rain showers. Phys. Rev. Lett. 97, 048501.CrossRefGoogle ScholarPubMed
Xu, H., Pumir, A. & Bodenschatz, E. 2015 Lagrangian view of time irreversibility of fluid turbulence. Sci. China Phys. Mech. Astron. 59 (1), 1–9.Google Scholar
Zaichik, L. I. & Alipchenkov, V. M. 2009 Statistical models for predicting pair dispersion and particle clustering in isotropic turbulence and their applications. New J. Phys. 11, 103018.Google Scholar