Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-18T10:26:00.825Z Has data issue: false hasContentIssue false
  • English
  • Français

Variational formulation of marine ice-sheet and subglacial-lake grounding-line dynamics

Published online by Cambridge University Press:  26 May 2021

Aaron G. Stubblefield Open the ORCID record for Aaron G. Stubblefield [Opens in a new window] ,
Marc Spiegelman  and
Timothy T. Creyts
Aaron G. Stubblefield*
Affiliation:
Lamont-Doherty Earth Observatory, Columbia University, Palisades, NY, USA
Marc Spiegelman
Affiliation:
Lamont-Doherty Earth Observatory, Columbia University, Palisades, NY, USA Department of Applied Physics and Applied Math, Columbia University, New York, NY, USA
Timothy T. Creyts
Affiliation:
Lamont-Doherty Earth Observatory, Columbia University, Palisades, NY, USA
*
†Email address for correspondence: aaron@ldeo.columbia.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

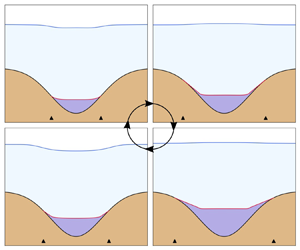
Grounding lines exist where land-based glacial ice flows on to a body of water. Accurately modelling grounding-line migration at the ice–ocean interface is essential for estimating future ice-sheet mass change. On the interior of ice sheets, the shores of subglacial lakes are also grounding lines. Grounding-line positions are sensitive to water volume changes such as sea-level rise or subglacial-lake drainage. Here, we introduce numerical methods for simulating grounding-line dynamics in the marine ice sheet and subglacial-lake settings. Variational inequalities arise from contact conditions that relate normal stress, water pressure and velocity at the base. Existence and uniqueness of solutions to these problems are established using a minimisation argument. A penalty method is used to replace the variational inequalities with variational equations that are solved using a finite-element method. We illustrate the grounding-line response to tidal cycles in the marine ice-sheet problem and filling–draining cycles in the subglacial-lake problem. We introduce two computational benchmarks where the known lake volume change is used to measure the accuracy of the numerical method.

JFM classification

Geophysical and Geological Flows: Ice sheets Mathematical Foundations: Variational methods Mathematical Foundations: Computational methods
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 919 , 25 July 2021 , A23
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Alnæs, M.S., Blechta, J., Hake, J., Johansson, A., Kehlet, B., Logg, A., Richardson, C., Ring, J., Rognes, M.E. & Wells, G.N. 2015 The FEniCS Project Version 1.5. Arch. Numer. Softw. 3 (100), 9–23.Google Scholar
Amrouche, C. & Girault, V. 1994 Decomposition of vector spaces and application to the Stokes problem in arbitrary dimension. Czech. Math. J. 44 (1), 109–140.CrossRefGoogle Scholar
Brunt, K.M., Fricker, H.A. & Padman, L. 2011 Analysis of ice plains of the Filchner–Ronne Ice Shelf, Antarctica, using ICESat laser altimetry. J. Glaciol. 57 (205), 965–975.CrossRefGoogle Scholar
Brunt, K.M., Fricker, H.A., Padman, L., Scambos, T.A. & O'Neel, S. 2010 Mapping the grounding zone of the Ross Ice Shelf, Antarctica, using ICESat laser altimetry. Ann. Glaciol. 51 (55), 71–79.CrossRefGoogle Scholar
Chen, Q., Gunzburger, M. & Perego, M. 2013 Well-posedness results for a nonlinear Stokes problem arising in glaciology. SIAM J. Math. Anal. 45 (5), 2710–2733.CrossRefGoogle Scholar
Cheng, G., Lötstedt, P. & von Sydow, L. 2020 A full stokes subgrid scheme in two dimensions for simulation of grounding line migration in ice sheets using elmer/ice (v8.3). Geosci. Model Dev. 13 (5), 2245–2258.CrossRefGoogle Scholar
Conway, J.B. 2007 A Course in Functional Analysis. Springer.CrossRefGoogle Scholar
Creyts, T.T. & Schoof, C.G. 2009 Drainage through subglacial water sheets. J. Geophys. Res.: Earth 114 (F4), F04008.Google Scholar
Cuffey, K.M. & Paterson, W.S.B. 2010 The Physics of Glaciers. Academic Press.Google Scholar
Durand, G., Gagliardini, O., De Fleurian, B., Zwinger, T. & Le Meur, E. 2009 a Marine ice sheet dynamics: hysteresis and neutral equilibrium. J. Geophys. Res.: Sol. Ea. 114 (3), 1–10.Google Scholar
Durand, G., Gagliardini, O., Zwinger, T., Le Meur, E. & Hindmarsh, R.C.A. 2009 b Full Stokes modeling of marine ice sheets: influence of the grid size. Ann. Glaciol. 50 (52), 109–114.CrossRefGoogle Scholar
Ekeland, I. & Temam, R. 1999 Convex Analysis and Variational Problems. SIAM.CrossRefGoogle Scholar
Favier, L., Durand, G., Cornford, S.L., Gudmundsson, G.H., Gagliardini, O., Gillet- Chaulet, F., Zwinger, T., Payne, A.J. & Le Brocq, A.M. 2014 Retreat of Pine Island Glacier controlled by marine ice-sheet instability. Nat. Clim. Change 4 (2), 117–121.CrossRefGoogle Scholar
Favier, L., Gagliardini, O., Durand, G. & Zwinger, T. 2012 A three-dimensional full Stokes model of the grounding line dynamics: effect of a pinning point beneath the ice shelf. Cryosphere 6 (1), 101–112.CrossRefGoogle Scholar
Fowler, A.C. 1986 A sliding law for glaciers of constant viscosity in the presence of subglacial cavitation. Proc. R. Soc. Lond. A 407 (1832), 147–170.Google Scholar
Fowler, A.C. 1999 Breaking the seal at Grímsvötn, Iceland. J. Glaciol. 45 (151), 506–516.CrossRefGoogle Scholar
Fowler, A.C. 2009 Dynamics of subglacial floods. Proc. R. Soc. Lond. A 465 (2106), 1809–1828.Google Scholar
Fricker, H.A. & Padman, L. 2006 Ice shelf grounding zone structure from ICESat laser altimetry. Geophys. Res. Lett. 33 (15), L15502.CrossRefGoogle Scholar
Fricker, H.A. & Scambos, T. 2009 Connected subglacial lake activity on lower Mercer and Whillans Ice Streams, West Antarctica, 2003–2008. J. Glaciol. 55 (190), 303–315.CrossRefGoogle Scholar
Fürst, J.J., Durand, G., Gillet-Chaulet, F., Tavard, L., Rankl, M., Braun, M. & Gagliardini, O. 2016 The safety band of Antarctic ice shelves. Nat. Clim. Change 6 (5), 479–482.CrossRefGoogle Scholar
Gagliardini, O., Brondex, J., Gillet-Chaulet, F., Tavard, L., Peyaud, V. & DURAND, G. 2016 Impact of mesh resolution for MISMIP and MISMIP3d experiments using Elmer/Ice. Cryosphere 10 (1), 307–312.CrossRefGoogle Scholar
Gagliardini, O., Cohen, D., Råback, P. & Zwinger, T. 2007 Finite-element modeling of subglacial cavities and related friction law. J. Geophys. Res.: Earth 112 (F2), F02027.Google Scholar
Glen, J.W. 1955 The creep of polycrystalline ice. Proc. R. Soc. Lond. A 228 (1175), 519–538.Google Scholar
Gudlaugsson, E., Humbert, A., Kleiner, T., Kohler, J. & Andreassen, K. 2016 The influence of a model subglacial lake on ice dynamics and internal layering. Cryosphere 10 (2), 751–760.CrossRefGoogle Scholar
Gudmundsson, G.H. 2007 Tides and the flow of Rutford ice stream, West Antarctica. J. Geophys. Res.: Earth 112 (F4), F04007.Google Scholar
Gudmundsson, G.H., Krug, J., Durand, G., Favier, L. & Gagliardini, O. 2012 The stability of grounding lines on retrograde slopes. Cryosphere 6 (6), 1497–1505.CrossRefGoogle Scholar
Helanow, C. & Ahlkrona, J. 2018 Stabilized equal low-order finite elements in ice sheet modeling – accuracy and robustness. Comput. Geosci. 22 (4), 951–974.CrossRefGoogle Scholar
Hewitt, I.J., Schoof, C. & Werder, M.A. 2012 Flotation and free surface flow in a model for subglacial drainage. Part 2. Channel flow. J. Fluid Mech. 702, 157–187.CrossRefGoogle Scholar
Howell, J.S. & Walkington, N.J. 2011 Inf-sup conditions for twofold saddle point problems. Numer. Math. 118 (4), 663–693.CrossRefGoogle Scholar
Isaac, T., Stadler, G. & Ghattas, O. 2015 Solution of nonlinear Stokes equations discretized by high-order finite elements on nonconforming and anisotropic meshes, with application to ice sheet dynamics. SIAM J. Sci. Comput. 37 (6), B804–B833.CrossRefGoogle Scholar
Ito, K. & Kunisch, K. 2008 Semi-smooth Newton methods for the Signorini problem. Appl. Maths 53 (5), 455–468.CrossRefGoogle Scholar
Joughin, I., Smith, B.E. & Medley, B. 2014 Marine ice sheet collapse potentially under way for the Thwaites Glacier Basin, West Antarctica. Science 344 (6185), 735–738.CrossRefGoogle ScholarPubMed
Jouvet, G. & Rappaz, J. 2011 Analysis and finite element approximation of a nonlinear stationary Stokes problem arising in glaciology. Adv. Numer. Anal. 2011, 164581.Google Scholar
Kamb, B. 1970 Sliding motion of glaciers: theory and observation. Rev. Geophys. 8 (4), 673–728.CrossRefGoogle Scholar
Kikuchi, N. & Oden, J.T. 1988 Contact Problems in Elasticity: A Study of Variational Inequalities and Finite Element Methods. SIAM.CrossRefGoogle Scholar
Kingslake, J. 2015 Chaotic dynamics of a glaciohydraulic model. J. Glaciol. 61 (227), 493–502.CrossRefGoogle Scholar
Logg, A., Mardal, K.-A. & Wells, G.N. 2012 Automated Solution of Differential Equations by the Finite Element Method. Springer.CrossRefGoogle Scholar
Logg, A. & Wells, G.N. 2010 DOLFIN: automated finite element computing. ACM Trans. Math. Softw. 37 (2), 20.CrossRefGoogle Scholar
MacAyeal, D.R. 1989 Large-scale ice flow over a viscous basal sediment: theory and application to ice stream B, Antarctica. J. Geophys. Res. 94 (B4), 4071–4087.CrossRefGoogle Scholar
Milillo, P., Rignot, E., Rizzoli, P., Scheuchl, B., Mouginot, J., Bueso-Bello, J. & Prats-Iraola, P. 2019 Heterogeneous retreat and ice melt of Thwaites Glacier, West Antarctica. Sci. Adv. 5 (1), eaau3433.CrossRefGoogle ScholarPubMed
Muszynski, I. & Birchfield, G.E. 1987 A coupled marine ice-stream–ice-shelf model. J. Glaciol. 33 (113), 3–15.CrossRefGoogle Scholar
Nye, J.F. 1976 Water flow in glaciers: jökulhlaups, tunnels and veins. J. Glaciol. 17 (76), 181–207.CrossRefGoogle Scholar
Paolo, F.S., Fricker, H.A. & Padman, L. 2015 Volume loss from Antarctic ice shelves is accelerating. Science 348 (6232), 327–331.CrossRefGoogle ScholarPubMed
Pattyn, F. 2008 Investigating the stability of subglacial lakes with a full Stokes ice-sheet model. J. Glaciol. 54 (185), 353–361.CrossRefGoogle Scholar
Pegler, S.S. 2018 Marine ice sheet dynamics: the impacts of ice-shelf buttressing. J. Fluid Mech. 857, 605–647.CrossRefGoogle Scholar
Pegler, S.S., Kowal, K.N., Hasenclever, L.Q. & Worster, M.G. 2013 Lateral controls on grounding-line dynamics. J. Fluid Mech. 722, R1.CrossRefGoogle Scholar
Pegler, S.S. & Worster, M.G. 2013 An experimental and theoretical study of the dynamics of grounding lines. J. Fluid Mech. 728, 5–28.CrossRefGoogle Scholar
Petra, N., Zhu, H., Stadler, G., Hughes, T.J.R. & Ghattas, O. 2012 An inexact Gauss–Newton method for inversion of basal sliding and rheology parameters in a nonlinear Stokes ice sheet model. J. Glaciol. 58 (211), 889–903.CrossRefGoogle Scholar
Ribe, N.M. 2001 Bending and stretching of thin viscous sheets. J. Fluid Mech. 433, 135–160.CrossRefGoogle Scholar
Rignot, E., Mouginot, J., Morlighem, M., Seroussi, H. & Scheuchl, B. 2014 Widespread, rapid grounding line retreat of Pine Island, Thwaites, Smith, and Kohler glaciers, West Antarctica, from 1992 to 2011. Geophys. Res. Lett. 41 (10), 3502–3509.CrossRefGoogle Scholar
Robel, A.A., Tsai, V.C., Minchew, B. & Simons, M. 2017 Tidal modulation of ice shelf buttressing stresses. Ann. Glaciol. 58 (74), 12–20.CrossRefGoogle Scholar
Robison, R.A.V., Huppert, H.E & Worster, M.G. 2010 Dynamics of viscous grounding lines. J. Fluid Mech. 648, 363–380.CrossRefGoogle Scholar
Rosier, S. & Gudmundsson, H. 2020 Exploring mechanisms responsible for tidal modulation in flow of the Filchner–Ronne Ice Shelf. Cryosphere 14 (1), 17–37.CrossRefGoogle Scholar
Rosier, S., Gudmundsson, H. & Green, J.A.M 2014 Insights into ice stream dynamics through modelling their response to tidal forcing. Cryosphere 8 (5), 1763–1775.CrossRefGoogle Scholar
Sayag, R. & Worster, M.G. 2013 Elastic dynamics and tidal migration of grounding lines modify subglacial lubrication and melting. Geophys. Res. Lett. 40 (22), 5877–5881.CrossRefGoogle Scholar
Schoof, C. 2005 The effect of cavitation on glacier sliding. Proc. R. Soc. Lond. A 461 (2055), 609–627.Google Scholar
Schoof, C. 2007 a Ice sheet grounding line dynamics: steady states, stability, and hysteresis. J. Geophys. Res.: Earth 112 (3), F03S28.Google Scholar
Schoof, C. 2007 b Marine ice-sheet dynamics. Part 1. The case of rapid sliding. J. Fluid Mech. 573 (February), 27–55.CrossRefGoogle Scholar
Schoof, C. 2011 Marine ice sheet dynamics. Part 2. A Stokes flow contact problem. J. Fluid Mech. 679, 122–155.CrossRefGoogle Scholar
Schoof, C. 2012 Marine ice sheet stability. J. Fluid Mech. 698, 62–72.CrossRefGoogle Scholar
Schoof, C., Hewitt, I.J. & Werder, M.A. 2012 Flotation and free surface flow in a model for subglacial drainage. Part 1. Distributed drainage. J. Fluid Mech. 702, 126–156.CrossRefGoogle Scholar
Seddik, H., Greve, R., Zwinger, T., Gillet-Chaulet, F. & Gagliardini, O. 2012 Simulations of the Greenland ice sheet 100 years into the future with the full Stokes model Elmer/Ice. J. Glaciol. 58 (209), 427–440.CrossRefGoogle Scholar
Sergienko, O.V., MacAyeal, D.R. & Bindschadler, R.A. 2009 Stick–slip behavior of ice streams: modeling investigations. Ann. Glaciol. 50 (52), 87–94.CrossRefGoogle Scholar
Seroussi, H., Dhia, H.B., Morlighem, M., Larour, E., Rignot, E. & Aubry, D. 2012 Coupling ice flow models of varying orders of complexity with the Tiling method. J. Glaciol. 58 (210), 776–786.CrossRefGoogle Scholar
Seroussi, H., Morlighem, M., Larour, E., Rignot, E. & Khazendar, A. 2014 Hydrostatic grounding line parameterization in ice sheet models. Cryosphere 8 (6), 2075–2087.CrossRefGoogle Scholar
Siegfried, M.R. & Fricker, H.A. 2018 Thirteen years of subglacial lake activity in Antarctica from multi-mission satellite altimetry. Ann. Glaciol. 59, 42–55.CrossRefGoogle Scholar
Smith, B.E., Fricker, H.A., Joughin, I.R. & Tulaczyk, S. 2009 An inventory of active subglacial lakes in Antarctica detected by ICESat (2003–2008). J. Glaciol. 55 (192), 573–595.CrossRefGoogle Scholar
Smith, B.E., Gourmelen, N., Huth, A. & Joughin, I. 2017 Connected subglacial lake drainage beneath Thwaites Glacier, West Antarctica. Cryosphere 11 (1), 451–467.CrossRefGoogle Scholar
Stadler, G. 2007 Path-following and augmented Lagrangian methods for contact problems in linear elasticity. J. Comput. Appl. Maths 203 (2), 533–547.CrossRefGoogle Scholar
Stubblefield, A. 2020 agstub/grounding-line-methods: first release of full-Stokes grounding line dynamics solver. J. Fluid Mech. doi:10.5281/zenodo.4302610.CrossRefGoogle Scholar
Stubblefield, A.G., Creyts, T.T., Kingslake, J. & Spiegelman, M. 2019 Modeling oscillations in connected glacial lakes. J. Glaciol. 65 (253), 745–758.CrossRefGoogle Scholar
Sykes, H.J., Murray, T. & Luckman, A. 2009 The location of the grounding zone of Evans Ice Stream, Antarctica, investigated using SAR interferometry and modelling. Ann. Glaciol. 50 (52), 35–40.CrossRefGoogle Scholar
Walder, J.S. & Fowler, A. 1994 Channelized subglacial drainage over a deformable bed. J. Glaciol. 40 (134), 3–15.CrossRefGoogle Scholar
Warburton, K.L.P, Hewitt, D.R. & Neufeld, J.A. 2020 Tidal grounding-line migration modulated by subglacial hydrology. Geophys. Res. Lett. 47 (17), e2020GL089088.CrossRefGoogle Scholar
Weertman, J. 1957 On the sliding of glaciers. J. Glaciol. 3 (21), 33–38.CrossRefGoogle Scholar
Weertman, J. 1974 Stability of the junction of an ice sheet and an ice shelf. J. Glaciol. 13 (67), 3–11.CrossRefGoogle Scholar
Wright, A. & Siegert, M. 2012 A fourth inventory of Antarctic subglacial lakes. Antarct. Sci. 24 (6), 659–664.CrossRefGoogle Scholar
Zhang, H., Ju, L., Gunzburger, M., Ringler, T. & Price, S. 2011 Coupled models and parallel simulations for three-dimensional full-Stokes ice sheet modeling. Numer. Math.: Theory Me. 4 (3), 396–418.Google Scholar
Zhu, H., Petra, N., Stadler, G., Isaac, T., Hughes, T.J.R. & Ghattas, O. 2016 Inversion of geothermal heat flux in a thermomechanically coupled nonlinear Stokes ice sheet model. Cryosphere 10 (4), 1477–1494.CrossRefGoogle Scholar

Stubblefield et al. supplementary movie 1

See pdf file for movie caption

Download Stubblefield et al. supplementary movie 1(Video)
Video 3.3 MB

Stubblefield et al. supplementary movie 2

See pdf file for movie caption

Download Stubblefield et al. supplementary movie 2(Video)
Video 2.1 MB

Stubblefield et al. supplementary movie 3

See pdf file for movie caption

Download Stubblefield et al. supplementary movie 3(Video)
Video 1.9 MB

Stubblefield et al. supplementary movie 4

See pdf file for movie caption

Download Stubblefield et al. supplementary movie 4(Video)
Video 2.1 MB

Stubblefield et al. supplementary movie 5

See pdf file for movie caption

Download Stubblefield et al. supplementary movie 5(Video)
Video 2.2 MB

Stubblefield et al. supplementary movie 6

See pdf file for movie caption

Download Stubblefield et al. supplementary movie 6(Video)
Video 1.1 MB
Supplementary material: PDF

Stubblefield et al. supplementary material

See pdf file for movie caption

Download Stubblefield et al. supplementary material(PDF)
PDF 18.4 KB