Hostname: page-component-8448b6f56d-t5pn6 Total loading time: 0 Render date: 2024-04-23T16:06:35.548Z Has data issue: false hasContentIssue false
  • English
  • Français

Renewal theory for iterated perturbed random walks on a general branching process tree: Early generations

Part of: Markov processes Stochastic processes Special processes

Published online by Cambridge University Press:  02 September 2022

Alexander Iksanov Open the ORCID record for Alexander Iksanov [Opens in a new window] ,
Bohdan Rashytov  and
Igor Samoilenko
Alexander Iksanov*
Affiliation:
Taras Shevchenko National University of Kyiv
Bohdan Rashytov*
Affiliation:
Taras Shevchenko National University of Kyiv
Igor Samoilenko*
Affiliation:
Taras Shevchenko National University of Kyiv
*
*Postal address: Faculty of Computer Science and Cybernetics, Taras Shevchenko National University of Kyiv, Volodymyrska 64/13, Kyiv, Ukraine, 01601.
*Postal address: Faculty of Computer Science and Cybernetics, Taras Shevchenko National University of Kyiv, Volodymyrska 64/13, Kyiv, Ukraine, 01601.
*Postal address: Faculty of Computer Science and Cybernetics, Taras Shevchenko National University of Kyiv, Volodymyrska 64/13, Kyiv, Ukraine, 01601.
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 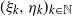$(\xi_k,\eta_k)_{k\in\mathbb{N}}$ be independent identically distributed random vectors with arbitrarily dependent positive components. We call a (globally) perturbed random walk a random sequence 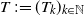$T\,{:\!=}\, (T_k)_{k\in\mathbb{N}}$ defined by 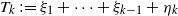$T_k\,{:\!=}\, \xi_1+\cdots+\xi_{k-1}+\eta_k$ for 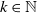$k\in\mathbb{N}$ . Consider a general branching process generated by T and let 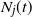$N_j(t)$ denote the number of the jth generation individuals with birth times 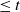$\leq t$ . We treat early generations, that is, fixed generations j which do not depend on t. In this setting we prove counterparts for 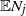$\mathbb{E}N_j$ of the Blackwell theorem and the key renewal theorem, prove a strong law of large numbers for 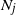$N_j$ , and find the first-order asymptotics for the variance of 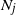$N_j$ . Also, we prove a functional limit theorem for the vector-valued process 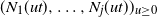$(N_1(ut),\ldots, N_j(ut))_{u\geq0}$ , properly normalized and centered, as 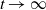$t\to\infty$ . The limit is a vector-valued Gaussian process whose components are integrated Brownian motions.

Keywords

Functional limit theoremgeneral branching processkey renewal theoremperturbed random walkrenewal theorystrong law of large numbers

MSC classification

Primary: 60K05: Renewal theory 60J80: Branching processes (Galton-Watson, birth-and-death, etc.)
Secondary: 60G05: Foundations of stochastic processes
Type
Original Article
Information
Journal of Applied Probability , Volume 60 , Issue 1 , March 2023 , pp. 45 - 67
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Applied Probability Trust

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alsmeyer, G., Iksanov, A. and Marynych, A. (2017). Functional limit theorems for the number of occupied boxes in the Bernoulli sieve. Stoch. Process. Appl. 127, 995–1017.CrossRefGoogle Scholar
Bingham, N. H., Goldie, C. M. and Teugels, J. L. (1989). Regular Variation. Cambridge University Press.Google Scholar
Bohun, V., Iksanov, A., Marynych, A. and Rashytov, B. (2022). Renewal theory for iterated perturbed random walks on a general branching process tree: Intermediate generations. J. Appl. Prob. 59, 421–446.CrossRefGoogle Scholar
Buraczewski, D., Dovgay, B. and Iksanov, A. (2020). On intermediate levels of nested occupancy scheme in random environment generated by stick-breaking I. Electron. J. Prob. 25, 123.10.1214/20-EJP534CrossRefGoogle Scholar
Gnedin, A. and Iksanov, A. (2020). On nested infinite occupancy scheme in random environment. Prob. Theory Related Fields 177, 855–890.CrossRefGoogle Scholar
Gut, A. (2009). Stopped Random Walks: Limit Theorems and Applications, 2nd edn. Springer.CrossRefGoogle Scholar
Iksanov, A. (2016). Renewal Theory for Perturbed Random walks and Similar Processes. Birkhäuser.CrossRefGoogle Scholar
Iksanov, A. and Kabluchko, Z. (2018). A functional limit theorem for the profile of random recursive trees. Electron. Commun. Prob. 23, 87.CrossRefGoogle Scholar
Karlin, S. (1967). Central limit theorems for certain infinite urn schemes. J. Math. Mech. 17, 373–401.Google Scholar
Mitov, K. V. and Omey, E. (2014). Renewal Processes. Springer.CrossRefGoogle Scholar
Resnick, S. I. (2002). Adventures in Stochastic Processes , 3rd printing. Birkhäuser.Google Scholar
Resnick, S. and Rootzén, H. (2000). Self-similar communication models and very heavy tails. Ann. Appl. Prob. 10, 753–778.CrossRefGoogle Scholar
Shi, Z. (2015). Branching Random Walks. Springer.CrossRefGoogle Scholar
Whitt, W. (2002). Stochastic-Process Limits: An Introduction to Stochastic-Process Limits and their Application to Queues. Springer.CrossRefGoogle Scholar