 $\mathrm {GL}(4, \mathbb R) $ (with an appendix by Bingrong Huang)
$\mathrm {GL}(4, \mathbb R) $ (with an appendix by Bingrong Huang)Published online by Cambridge University Press: 07 June 2021
Orthogonality is a fundamental theme in representation theory and Fourier analysis. An orthogonality relation for characters of finite abelian groups (now recognized as an orthogonality relation on 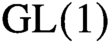 $\mathrm {GL}(1)$) was used by Dirichlet to prove infinitely many primes in arithmetic progressions. Orthogonality relations for
$\mathrm {GL}(1)$) was used by Dirichlet to prove infinitely many primes in arithmetic progressions. Orthogonality relations for  $\mathrm {GL}(2)$ and
$\mathrm {GL}(2)$ and 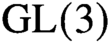 $\mathrm {GL}(3)$ have been worked on by many researchers with a broad range of applications to number theory. We present here, for the first time, very explicit orthogonality relations for the real group
$\mathrm {GL}(3)$ have been worked on by many researchers with a broad range of applications to number theory. We present here, for the first time, very explicit orthogonality relations for the real group  $\mathrm {GL}(4, \mathbb R)$ with a power savings error term. The proof requires novel techniques in the computation of the geometric side of the Kuznetsov trace formula.
$\mathrm {GL}(4, \mathbb R)$ with a power savings error term. The proof requires novel techniques in the computation of the geometric side of the Kuznetsov trace formula.