Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-18T16:35:58.588Z Has data issue: false hasContentIssue false
  • English
  • Français

Graph algebras and orbit equivalence

Published online by Cambridge University Press:  25 August 2015

NATHAN BROWNLOWE ,
TOKE MEIER CARLSEN  and
MICHAEL F. WHITTAKER
NATHAN BROWNLOWE
Affiliation:
School of Mathematics and Applied Statistics, The University of Wollongong, NSW 2522, Australia email nathanb@uow.edu.au, mfwhittaker@gmail.com
TOKE MEIER CARLSEN
Affiliation:
Department of Mathematical Sciences, Norwegian University of Science and Technology (NTNU), 7491 Trondheim, Norway email Toke.Meier.Carlsen@math.ntnu.no
MICHAEL F. WHITTAKER
Affiliation:
School of Mathematics and Applied Statistics, The University of Wollongong, NSW 2522, Australia email nathanb@uow.edu.au, mfwhittaker@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

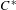We introduce the notion of orbit equivalence of directed graphs, following Matsumoto’s notion of continuous orbit equivalence for topological Markov shifts. We show that two graphs in which every cycle has an exit are orbit equivalent if and only if there is a diagonal-preserving isomorphism between their $C^{\ast }$-algebras. We show that it is necessary to assume that every cycle has an exit for the forward implication, but that the reverse implication holds for arbitrary graphs. As part of our analysis of arbitrary graphs $E$ we construct a groupoid 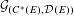${\mathcal{G}}_{(C^{\ast }(E),{\mathcal{D}}(E))}$ from the graph algebra 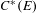$C^{\ast }(E)$ and its diagonal subalgebra 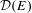${\mathcal{D}}(E)$ which generalises Renault’s Weyl groupoid construction applied to 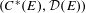$(C^{\ast }(E),{\mathcal{D}}(E))$. We show that ${\mathcal{G}}_{(C^{\ast }(E),{\mathcal{D}}(E))}$ recovers the graph groupoid 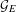${\mathcal{G}}_{E}$ without the assumption that every cycle in 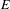$E$ has an exit, which is required to apply Renault’s results to $(C^{\ast }(E),{\mathcal{D}}(E))$. We finish with applications of our results to out-splittings of graphs and to amplified graphs.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 37 , Issue 2 , April 2017 , pp. 389 - 417
Copyright
© Cambridge University Press, 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anantharaman-Delaroche, C. and Renault, J.. Amenable Groupoids (Monographies de L’Enseignement Mathématique, 36) . L’Enseignement Mathématique, Geneva, 2000.Google Scholar
an Huef, A. and Raeburn, I.. The ideal structure of Cuntz–Krieger algebras. Ergod. Th. & Dynam. Sys. 17 (1997), 611–624.CrossRefGoogle Scholar
Bates, T., Hong, J., Raeburn, I. and Szymanski, W.. The ideal structure of the C ∗ -algebras of infinite graphs. Illinois J. Math. 46 (2002), 1159–1176.CrossRefGoogle Scholar
Bates, T. and Pask, D.. Flow equivalence of graph algebras. Ergod. Th. & Dynam. Sys. 24 (2004), 367–382.CrossRefGoogle Scholar
Eilers, S., Ruiz, E. and Sørensen, A.. Amplified graph C ∗ -algebras. Münster J. Math. 5 (2012), 121–150.Google Scholar
Giordano, T., Matui, H., Putnam, I. and Skau, C.. Orbit equivalence for Cantor minimal ℤ d -systems. Invent. Math. 179 (2010), 119–158.CrossRefGoogle Scholar
Giordano, T., Putnam, I. and Skau, C.. Topological orbit equivalence and C ∗ -crossed products. J. Reine Angew. Math. 469 (1995), 51–111.Google Scholar
Kumjian, A.. On C ∗ -diagonals. Canad. J. Math. 4 (1986), 969–1008.CrossRefGoogle Scholar
Kumjian, A., Pask, D., Raeburn, I. and Renault, J.. Graphs, groupoids, and Cuntz–Krieger algebras. J. Func. Anal. 144 (1997), 505–541.CrossRefGoogle Scholar
Matsumoto, K.. Orbit equivalence of topological Markov shifts and Cuntz–Krieger algebras. Pacific J. Math. 246 (2010), 199–225.CrossRefGoogle Scholar
Matsumoto, K.. Full groups of one-sided topological Markov shifts. Israel J. Math. 205 (2015), 1–3.CrossRefGoogle Scholar
Matsumoto, K. and Matui, H.. Continuous orbit equivalence of topological Markov shifts and Cuntz–Krieger algebras. Kyoto J. Math. 54 (2014), 863–877.CrossRefGoogle Scholar
Matui, H.. Homology and topological full groups of étale groupoids on totally disconnected spaces. Proc. Lond. Math. Soc. 104 (2012), 27–56.CrossRefGoogle Scholar
Nagy, G. and Reznikoff, S.. Pseudo-diagonals and uniqueness theorems. Proc. Amer. Math. Soc. 142 (2014), 263–275.CrossRefGoogle Scholar
Paterson, A. L. T.. Graph inverse semigroups, groupoids and their C ∗ -algebras. J. Operator Theory 48 (2002), 645–662.Google Scholar
Raeburn, I.. Graph Algebras. Published for the Conference Board of the Mathematical Sciences, Washington, DC, 2005, vi+113.CrossRefGoogle Scholar
Raeburn, I. and Williams, D.. Morita Equivalence and Continuous-Trace C ∗ -Algebras (Mathematical Surveys and Monographs, 60) . The American Mathematical Society, Providence, RI, 1998, xiv+327.CrossRefGoogle Scholar
Renault, J. N.. A Groupoid Approach to C ∗ -Algebras (Lecture Notes in Mathematics, 793) . Springer, Berlin, 1980.CrossRefGoogle Scholar
Renault, J. N.. Cartan subalgebras in C ∗ -algebras. Irish Math. Soc. Bull. 61 (2008), 29–63.CrossRefGoogle Scholar
Rørdam, M.. Classification of Cuntz–Krieger algebras. K-Theory 9 (1995), 31–58.CrossRefGoogle Scholar
Tomiyama, J.. Topological full groups and structure of normalizers in transformation group C ∗ -algebras. Pacific J. Math. 173 (1996), 571–583.CrossRefGoogle Scholar
Webster, S.. The path space of a directed graph. Proc. Amer. Math. Soc. 142 (2014), 213–225.CrossRefGoogle Scholar
Yeend, T.. Groupoid models for the C ∗ -algebras of topological higher-rank graphs. J. Operator Theory 57 (2007), 95–120.Google Scholar