1 Introduction
Let G be a group and A a finite set. Then
![]() $A^{G}$
, with the product topology, is a compact topological space on which G naturally acts by homeomorphisms. Such a topological dynamical system is called a (topological) shift and any of its (topologically) closed G-invariant subsets are called subshifts. These are the objects of study of symbolic dynamics, whose many results and notions have inspired and have been generalized to more general dynamical systems.
$A^{G}$
, with the product topology, is a compact topological space on which G naturally acts by homeomorphisms. Such a topological dynamical system is called a (topological) shift and any of its (topologically) closed G-invariant subsets are called subshifts. These are the objects of study of symbolic dynamics, whose many results and notions have inspired and have been generalized to more general dynamical systems.
One of those is the Garden of Eden theorem. For
![]() $G={\mathbb {Z}}^{d}$
, Moore in [Reference Moore36], respectively Myhill in [Reference Myhill38], proved that every continuous surjective G-equivariant map
$G={\mathbb {Z}}^{d}$
, Moore in [Reference Moore36], respectively Myhill in [Reference Myhill38], proved that every continuous surjective G-equivariant map
![]() $\tau :A^{G}\to A^{G}$
is pre-injective, respectively conversely every such pre-injective map is surjective (we shall define pre-injectivity later). A dynamical system for which this equivalence holds is said to satisfy the Garden of Eden theorem. This result was generalized to all amenable groups in [Reference Ceccherini-Silberstein, Machì and Scarabotti16] and later also for certain subshifts of amenable groups (see [Reference Ceccherini-Silberstein and Coornaert10, Reference Fiorenzi27]). It did not take long for the Garden of Eden theorem to be considered for many other dynamical systems (see e.g. [Reference Ceccherini-Silberstein and Coornaert7, Reference Ceccherini-Silberstein and Coornaert11, Reference Ceccherini-Silberstein, Coornaert and Li13, Reference Ceccherini-Silberstein, Coornaert and Phung15, Reference Li34]). One of the most general versions of the Myhill property was considered by Li in [Reference Li34] where he proves that every expansive action of an amenable group on a compact metrizable space having the weak specification property satisfies the Myhill property.
$\tau :A^{G}\to A^{G}$
is pre-injective, respectively conversely every such pre-injective map is surjective (we shall define pre-injectivity later). A dynamical system for which this equivalence holds is said to satisfy the Garden of Eden theorem. This result was generalized to all amenable groups in [Reference Ceccherini-Silberstein, Machì and Scarabotti16] and later also for certain subshifts of amenable groups (see [Reference Ceccherini-Silberstein and Coornaert10, Reference Fiorenzi27]). It did not take long for the Garden of Eden theorem to be considered for many other dynamical systems (see e.g. [Reference Ceccherini-Silberstein and Coornaert7, Reference Ceccherini-Silberstein and Coornaert11, Reference Ceccherini-Silberstein, Coornaert and Li13, Reference Ceccherini-Silberstein, Coornaert and Phung15, Reference Li34]). One of the most general versions of the Myhill property was considered by Li in [Reference Li34] where he proves that every expansive action of an amenable group on a compact metrizable space having the weak specification property satisfies the Myhill property.
Our aim is to provide such a general result also for the Moore property. However, the weak specification property alone is not enough for establishing this property. This is known even for subshifts, where a counterexample was provided for strongly irreducible subshifts in [Reference Fiorenzi26] and it was showed in [Reference Li34] that for subshifts, the weak specification property is equivalent to being strongly irreducible. We add one of the topological Markov properties that were introduced, for general expansive dynamical systems, very recently by Barbieri, García-Ramos, and Li in [Reference Barbieri, García-Ramos and Li1] and which generalizes the pseudo-orbit tracing property, also known as shadowing, which for subshifts corresponds to being of finite type (see [Reference Chung and Lee18]).
Therefore, one of our main results is the following. All the notions will be defined in the next section.
Theorem A. Let G be a (countable) amenable group acting continuously and expansively on a compact metrizable space X so that the action has the weak specification and strong topological Markov properties (recall again that it is implied by the pseudo-orbit tracing property). Then the dynamical system
![]() $(X,G)$
has the Moore property. This together with the result of H. Li, which we also re-prove, implies that this dynamical system satisfies the Garden of Eden theorem.
$(X,G)$
has the Moore property. This together with the result of H. Li, which we also re-prove, implies that this dynamical system satisfies the Garden of Eden theorem.
The theorem covers and generalizes many of the previous results, including of [Reference Fiorenzi27], where the Garden of Eden theorem is proved for strongly irreducible subshifts of finite type of amenable groups, and of [Reference Ceccherini-Silberstein and Coornaert11], where the same theorem is proved for certain hyperbolic dynamical systems, namely, Anosov diffeomorphisms on tori; see Corollary 3.6, which describes some of the new examples to which the theorem can be applied.
The theorem also covers many of the algebraic dynamical systems where the result of [Reference Li34] applies, that is, expansive algebraic actions of amenable groups with completely positive entropy. In particular, it also covers principal algebraic actions of amenable groups since they have the weak specification and pseudo-orbit tracing properties (see [Reference Li34, Lemma 2.1] and [Reference Meyerovitch35, Theorem 1.5] respectively).
The restriction to countable groups and metrizable spaces is not at all necessary, although perhaps the most interesting. In §3.2, we hint at how to easily generalize the result also for uncountable groups and non-metrizable spaces. This simplifies and generalizes the result of Ceccherini-Silberstein and Coornaert in [Reference Ceccherini-Silberstein and Coornaert12].
Next we consider periodic, respectively weakly periodic points of dynamical systems. If
![]() $(X,G)$
is a dynamical system, we call a point
$(X,G)$
is a dynamical system, we call a point
![]() $x\in X$
periodic if its orbit is finite, or equivalently, if its stabilizer has a finite index in G. We call a point
$x\in X$
periodic if its orbit is finite, or equivalently, if its stabilizer has a finite index in G. We call a point
![]() $x\in X$
weakly periodic if its stabilizer is infinite. In a recent breakthrough [Reference Cohen20], Cohen showed that if G is a finitely generated group having at least two ends, then every subshift of finite type of G must contain a weakly periodic point. Compare this with the other results for several one-ended groups which admit a subshift of finite type on which the group acts freely (see e.g. the classical result for
$x\in X$
weakly periodic if its stabilizer is infinite. In a recent breakthrough [Reference Cohen20], Cohen showed that if G is a finitely generated group having at least two ends, then every subshift of finite type of G must contain a weakly periodic point. Compare this with the other results for several one-ended groups which admit a subshift of finite type on which the group acts freely (see e.g. the classical result for
![]() ${\mathbb {Z}}^{2}$
in [Reference Berger5] and many other more recent developments in [Reference Cohen and Goodman-Strauss21, Reference Culik and Kari22, Reference Mozes37].
${\mathbb {Z}}^{2}$
in [Reference Berger5] and many other more recent developments in [Reference Cohen and Goodman-Strauss21, Reference Culik and Kari22, Reference Mozes37].
Here we generalize Cohen’s result again to far more general dynamical systems. For this, we introduce a new topological Markov property, called here the cover strong topological Markov property, in the spirit of [Reference Barbieri, García-Ramos and Li1], which is implied by the pseudo-orbit tracing property and implies the uniform strong topological Markov property – we do not know if it is equivalent to the latter. We show the following.
Theorem B. Let G be a finitely generated group having at least two ends. Suppose that G acts continuously and expansively on a compact metrizable space X so that the action has the cover strong topological Markov property. Then
![]() $(X,G)$
has weakly periodic points.
$(X,G)$
has weakly periodic points.
If the action has moreover the weak specification property, then the set of such points is dense in X.
For examples of dynamical systems satisfying various topological Markov properties, the pseudo-orbit tracing property, and of systems satisfying them together with the weak specification property, we refer the reader to e.g. [Reference Barbieri, García-Ramos and Li1, Reference Ceccherini-Silberstein, Coornaert and Li14]. We remark that among the standard examples are, in addition to various subshifts, also various finitely presented algebraic actions.
2 Preliminaries
Throughout the paper, we work with groups acting continuously on compact (most of the time metrizable) spaces. Groups, usually denoted by G, will be always discrete and will be implicitly assumed to be countable, although this assumption is usually either not necessary, or the arguments can be easily modified to work for uncountable groups as well. Topological spaces with a continuous action of a group G are sometimes called G-spaces.
When working with a compact metrizable space, we fix some compatible metric and formulate most of the notions using such a metric. For that reason, many of the statements are formulated for compact metric spaces instead of compact metrizable spaces. However, it should be emphasized that the statements are almost always of a topological, not metric, nature, so the choice of the compatible metric is irrelevant. This can be easily verified e.g. in the following definition, where we specify the topological dynamical systems with which we shall work.
Definition 2.1. Let G be a group acting continuously on a compact metrizable space X. We say that the action is expansive if, having fixed some compatible metric d on X, there exists
![]() $\delta>0$
(called the expansiveness constant) such that for every
$\delta>0$
(called the expansiveness constant) such that for every
![]() $x\neq y\in X$
, there is
$x\neq y\in X$
, there is
![]() $g\in G$
so that
$g\in G$
so that
Let X be a compact G-space and d a continuous pseudometric on X. For every subset
![]() $E\subseteq G$
, we shall denote by
$E\subseteq G$
, we shall denote by
![]() $d_{E}$
the pseudometric
$d_{E}$
the pseudometric
Lemma 2.2. Let G act continuously on a compact metric space expansively, with an expansive constant
![]() $\delta>0$
. Then for every
$\delta>0$
. Then for every
![]() $0<\gamma $
, there exists a finite set
$0<\gamma $
, there exists a finite set
![]() $D_{\gamma }\subseteq G$
such that for every
$D_{\gamma }\subseteq G$
such that for every
![]() $x,y\in X$
, if
$x,y\in X$
, if
![]() $d(x,y)\geq \gamma $
then
$d(x,y)\geq \gamma $
then
![]() $d_{D_{\gamma }}(x,y)>\delta $
.
$d_{D_{\gamma }}(x,y)>\delta $
.
Proof. Suppose that no such finite set exists. Then for every finite
![]() $D\subseteq G$
, there exist elements
$D\subseteq G$
, there exist elements
![]() $x_{D},y_{D}\in X$
such that
$x_{D},y_{D}\in X$
such that
![]() $d(x_{D},y_{D})\geq \gamma $
, yet
$d(x_{D},y_{D})\geq \gamma $
, yet
![]() $d_{D}(x,y)\leq \delta $
. Without loss of generality, we may assume that
$d_{D}(x,y)\leq \delta $
. Without loss of generality, we may assume that
![]() $x_{D}\to x\in X$
and
$x_{D}\to x\in X$
and
![]() $y_{D}\to y\in X$
(where
$y_{D}\to y\in X$
(where
![]() $(x_{D})$
and
$(x_{D})$
and
![]() $(y_{D})$
are nets indexed and ordered by finite subsets of G ordered by inclusion). By continuity,
$(y_{D})$
are nets indexed and ordered by finite subsets of G ordered by inclusion). By continuity,
![]() $d(x,y)\geq \gamma $
and
$d(x,y)\geq \gamma $
and
![]() $d_{D}(x,y)\leq \delta $
for every finite
$d_{D}(x,y)\leq \delta $
for every finite
![]() $D\subseteq G$
. The first inequality implies that
$D\subseteq G$
. The first inequality implies that
![]() $x\neq y$
, while the latter, using expansiveness, that
$x\neq y$
, while the latter, using expansiveness, that
![]() $x=y$
, a contradiction.
$x=y$
, a contradiction.
The following, formally stronger, result follows immediately from Lemma 2.2.
Proposition 2.3. Let G act continuously and expansively on a compact metric space expansively, with an expansive constant
![]() $\delta>0$
. Then for every subset
$\delta>0$
. Then for every subset
![]() $S\subseteq G$
,
$S\subseteq G$
,
![]() $\gamma>0$
, and
$\gamma>0$
, and
![]() $x,y\in X$
, if
$x,y\in X$
, if
![]() $d_{S}(x,y)\geq \gamma $
, then
$d_{S}(x,y)\geq \gamma $
, then
![]() $d_{D_{\gamma }\cdot S}(x,y)>\delta $
.
$d_{D_{\gamma }\cdot S}(x,y)>\delta $
.
Definition 2.4. Let G act continuously on a compact metric space X. For
![]() $x,y\in X$
and
$x,y\in X$
and
![]() $\varepsilon>0$
, we denote by
$\varepsilon>0$
, we denote by
![]() $\Lambda _{\varepsilon }(x,y)$
the set
$\Lambda _{\varepsilon }(x,y)$
the set
![]() $\{g\in G\colon d(gx,gy)>\varepsilon \}$
. If the constant
$\{g\in G\colon d(gx,gy)>\varepsilon \}$
. If the constant
![]() $\varepsilon $
is fixed and clear from the context, we write simply
$\varepsilon $
is fixed and clear from the context, we write simply
![]() $\Lambda (x,y)$
.
$\Lambda (x,y)$
.
Notice that if the action from the previous definition is moreover expansive with the expansiveness constant
![]() $\delta>0$
and
$\delta>0$
and
![]() $\varepsilon \leq \delta $
, then for
$\varepsilon \leq \delta $
, then for
![]() $x,y\in X$
,
$x,y\in X$
,
![]() $\Lambda _{\varepsilon }(x,y)$
is non-empty if and only if
$\Lambda _{\varepsilon }(x,y)$
is non-empty if and only if
![]() $x\neq y$
.
$x\neq y$
.
Next we introduce a crucial notion related to the Garden of Eden theorem.
Definition 2.5. Let G act continuously on a compact metric space X. We say that two elements
![]() $x,y\in X$
are homoclinic,
$x,y\in X$
are homoclinic,
![]() $x\sim y$
in symbols, if
$x\sim y$
in symbols, if
![]() $\lim _{g\to \infty } d(gx,gy)=0$
.
$\lim _{g\to \infty } d(gx,gy)=0$
.
It is easy to see that being homoclinic is an equivalence relation.
Corollary 2.6. Let G act continuously and expansively on a compact metric space X. Then for every
![]() $\delta>0$
and every elements
$\delta>0$
and every elements
![]() $x,y\in X$
, we have
$x,y\in X$
, we have
![]() $x\sim y$
if and only if
$x\sim y$
if and only if
![]() $\Lambda _{\delta }(x,y)$
is finite.
$\Lambda _{\delta }(x,y)$
is finite.
Proof. Fix
![]() $\delta>0$
and
$\delta>0$
and
![]() $x,y\in X$
. If
$x,y\in X$
. If
![]() $x\sim y$
, then by definition,
$x\sim y$
, then by definition,
![]() $\lim _{g\to \infty } d(gx,gy)=0$
, so
$\lim _{g\to \infty } d(gx,gy)=0$
, so
![]() $\Lambda _{\delta }(x,y)$
is finite. Conversely, suppose that
$\Lambda _{\delta }(x,y)$
is finite. Conversely, suppose that
![]() $\Lambda _{\delta }(x,y)$
is finite, however
$\Lambda _{\delta }(x,y)$
is finite, however
![]() $d(gx,gy)$
does not converge to
$d(gx,gy)$
does not converge to
![]() $0$
. Then there is
$0$
. Then there is
![]() $\gamma>0$
and an infinite set
$\gamma>0$
and an infinite set
![]() $S\subseteq G$
such that for all
$S\subseteq G$
such that for all
![]() $s\in S$
,
$s\in S$
,
![]() $d(sx,sy)\geq \gamma $
. By Lemma 2.2, for each
$d(sx,sy)\geq \gamma $
. By Lemma 2.2, for each
![]() $s\in S$
,
$s\in S$
,
![]() $D_{\gamma } s\cap \Lambda _{\gamma }(x,y)\neq \emptyset $
. Since S is infinite and
$D_{\gamma } s\cap \Lambda _{\gamma }(x,y)\neq \emptyset $
. Since S is infinite and
![]() $D_{\gamma }$
is finite, this implies that
$D_{\gamma }$
is finite, this implies that
![]() $\Lambda _{\delta }(x,y)$
is infinite, a contradiction.
$\Lambda _{\delta }(x,y)$
is infinite, a contradiction.
We also recall the topological entropy of amenable group actions that will play an important role in the proof of Theorem A. Here we use Bowen–Dinaburg’s characterization of topological entropy adapted to amenable group actions; we refer the reader to [Reference Kerr and Li33, §9.9] for more details.
In general, having a compact metric space X with a metric d and given some
![]() $\varepsilon>0$
, we write
$\varepsilon>0$
, we write
![]() ${\mathrm {sep}}(d,\varepsilon )$
for the maximal cardinality of a subset
${\mathrm {sep}}(d,\varepsilon )$
for the maximal cardinality of a subset
![]() $A\subseteq X$
that is
$A\subseteq X$
that is
![]() $\varepsilon $
-separated with respect to d, that is, for
$\varepsilon $
-separated with respect to d, that is, for
![]() $x\neq y\in A$
, we have
$x\neq y\in A$
, we have
![]() $d(x,y)\geq \varepsilon $
. Now given a continuous action of an amenable group G on a compact metric space X, we define the topological entropy of the action to be the value
$d(x,y)\geq \varepsilon $
. Now given a continuous action of an amenable group G on a compact metric space X, we define the topological entropy of the action to be the value
where
and
![]() $(F_{n})_{n}$
is an arbitrary Følner sequence (or net if G is uncountable). We again refer to [Reference Kerr and Li33, §9.9] for further explanations.
$(F_{n})_{n}$
is an arbitrary Følner sequence (or net if G is uncountable). We again refer to [Reference Kerr and Li33, §9.9] for further explanations.
The following result, likely well known, shows that the computation of topological entropy is easier for expansive dynamical systems.
Proposition 2.7. Let G be an amenable group and let X be a compact expansive G-metric space with an expansive constant
![]() $\delta>0$
. Then
$\delta>0$
. Then
![]() $h_{\mathrm {top}}(X,G)=h_{\mathrm {sep}}(\delta ,d)$
.
$h_{\mathrm {top}}(X,G)=h_{\mathrm {sep}}(\delta ,d)$
.
Proof. It suffices to show that for every
![]() $\varepsilon>0$
, we have
$\varepsilon>0$
, we have
![]() $h_{\mathrm {sep}}(\varepsilon ,d)=h_{\mathrm {sep}}(\delta ,d)$
. Since for
$h_{\mathrm {sep}}(\varepsilon ,d)=h_{\mathrm {sep}}(\delta ,d)$
. Since for
![]() $0<\varepsilon ^{\prime }<\varepsilon $
, we have
$0<\varepsilon ^{\prime }<\varepsilon $
, we have
![]() $h_{\mathrm {sep}}(\varepsilon ^{\prime },d)\geq h_{\mathrm {sep}}(\varepsilon ,d)$
, it suffices to show that for every
$h_{\mathrm {sep}}(\varepsilon ^{\prime },d)\geq h_{\mathrm {sep}}(\varepsilon ,d)$
, it suffices to show that for every
![]() $0<\gamma <\delta $
, we have
$0<\gamma <\delta $
, we have
![]() $h_{\mathrm {sep}}(\gamma ,d)\leq h_{\mathrm {sep}}(\delta ,d)$
.
$h_{\mathrm {sep}}(\gamma ,d)\leq h_{\mathrm {sep}}(\delta ,d)$
.
Fix
![]() $0<\gamma <\delta $
. By Proposition 2.3, there is a finite set
$0<\gamma <\delta $
. By Proposition 2.3, there is a finite set
![]() $D_{\gamma }\subseteq G$
such that for every subset
$D_{\gamma }\subseteq G$
such that for every subset
![]() $S\subseteq G$
and
$S\subseteq G$
and
![]() $x,y\in X$
, we have
$x,y\in X$
, we have
![]() $d_{D_{\gamma }\cdot S}(x,y)\geq \delta $
if
$d_{D_{\gamma }\cdot S}(x,y)\geq \delta $
if
![]() $d_{S}(x,y)\geq \gamma $
. It follows that for every subset
$d_{S}(x,y)\geq \gamma $
. It follows that for every subset
![]() $S\subseteq G$
,
$S\subseteq G$
,
![]() ${\mathrm {sep}}(d_{D_{\gamma }\cdot S},\delta )\geq {\mathrm {sep}}(d_{S},\gamma )$
. Fixing a Følner sequence (respectively net)
${\mathrm {sep}}(d_{D_{\gamma }\cdot S},\delta )\geq {\mathrm {sep}}(d_{S},\gamma )$
. Fixing a Følner sequence (respectively net)
![]() $(F_{n})_{n}$
, we get
$(F_{n})_{n}$
, we get
Since
![]() $(F_{n})_{n}$
is Følner, so for every finite subset
$(F_{n})_{n}$
is Følner, so for every finite subset
![]() $D\subseteq G$
(in particular for
$D\subseteq G$
(in particular for
![]() $D-D_{\gamma }$
) we have
$D-D_{\gamma }$
) we have
![]() $\lim _{n\to \infty } ({|D\cdot F_{n}\Delta F_{n}|}/{|F_{n}|}) =0$
, we get the following:
$\lim _{n\to \infty } ({|D\cdot F_{n}\Delta F_{n}|}/{|F_{n}|}) =0$
, we get the following:
-
(1)
 $(D_{\gamma }\cdot F_{n})_{n}$
is Følner as well;
$(D_{\gamma }\cdot F_{n})_{n}$
is Følner as well; -
(2)
 $ \limsup _{n\to \infty }({1}/{|F_{n}|})\log {\mathrm {sep}}(d_{D_{\gamma }\cdot F_{n}},\delta ) =\limsup _{n\to \infty } ({1}/{|D_{\gamma }\cdot F_{n}|})\log {\mathrm {sep}}(d_{D_{\gamma }\cdot F_{n}},\delta ). $
$ \limsup _{n\to \infty }({1}/{|F_{n}|})\log {\mathrm {sep}}(d_{D_{\gamma }\cdot F_{n}},\delta ) =\limsup _{n\to \infty } ({1}/{|D_{\gamma }\cdot F_{n}|})\log {\mathrm {sep}}(d_{D_{\gamma }\cdot F_{n}},\delta ). $
This implies that
![]() $h_{\mathrm {sep}}(\gamma ,d)\leq h_{\mathrm {sep}}(\delta ,d)$
and we are done.
$h_{\mathrm {sep}}(\gamma ,d)\leq h_{\mathrm {sep}}(\delta ,d)$
and we are done.
Definition 2.8. Let X and Y be compact metric spaces on which a group G acts continuously and expansively with expansiveness constants
![]() $\delta _{X}>0$
and
$\delta _{X}>0$
and
![]() $\delta _{Y}>0$
respectively. Let
$\delta _{Y}>0$
respectively. Let
![]() $\tau :X\rightarrow Y$
be a continuous G-equivariant map. A memory set for
$\tau :X\rightarrow Y$
be a continuous G-equivariant map. A memory set for
![]() $\tau $
is a set
$\tau $
is a set
![]() $M\subseteq G$
such that for every
$M\subseteq G$
such that for every
![]() $x,y\in X$
and
$x,y\in X$
and
![]() $g\in G$
, if
$g\in G$
, if
![]() $d_{M}(gx,gy)\leq \delta _{X}$
, then
$d_{M}(gx,gy)\leq \delta _{X}$
, then
![]() $d(g\tau (x), g\tau (y))\leq \delta _{Y}$
.
$d(g\tau (x), g\tau (y))\leq \delta _{Y}$
.
Lemma 2.9. Let X, Y, and
![]() $\tau :X\rightarrow Y$
be as above. There exists a finite memory set for
$\tau :X\rightarrow Y$
be as above. There exists a finite memory set for
![]() $\tau $
.
$\tau $
.
Proof. Suppose the contrary. Then for every finite set
![]() $E\subseteq G$
, there are
$E\subseteq G$
, there are
![]() $x_{E},y_{E}\in X$
and
$x_{E},y_{E}\in X$
and
![]() $g_{E}\in G$
such that
$g_{E}\in G$
such that
![]() $d_{E}(g_{E} x_{E},g_{E} y_{E})\leq \delta _{X}$
, yet
$d_{E}(g_{E} x_{E},g_{E} y_{E})\leq \delta _{X}$
, yet
![]() $d(g_{E}\tau (x_{E}),g_{E}\tau (y_{E}))>\delta _{Y}$
. By replacing
$d(g_{E}\tau (x_{E}),g_{E}\tau (y_{E}))>\delta _{Y}$
. By replacing
![]() $x_{E}$
, respectively
$x_{E}$
, respectively
![]() $y_{E}$
, by
$y_{E}$
, by
![]() $g_{E}^{-1}x_{E}$
, respectively
$g_{E}^{-1}x_{E}$
, respectively
![]() $g_{E}^{-1} y_{E}$
, we may and will assume that
$g_{E}^{-1} y_{E}$
, we may and will assume that
![]() $g_{E}\,{=}\,1_{G}$
. By compactness, there is a net
$g_{E}\,{=}\,1_{G}$
. By compactness, there is a net
![]() $(E_{i})_{i\in I}$
such that
$(E_{i})_{i\in I}$
such that
![]() $\bigcup _{i\in I} E_{i}=G$
and
$\bigcup _{i\in I} E_{i}=G$
and
![]() $\lim _{i} x_{E_{i}}=x$
and
$\lim _{i} x_{E_{i}}=x$
and
![]() $\lim _{i} y_{E_{i}}=y$
. By continuity and since
$\lim _{i} y_{E_{i}}=y$
. By continuity and since
![]() $(E_{i})_{i}$
cover the group, we have
$(E_{i})_{i}$
cover the group, we have
![]() $d(gx,gy)\leq \delta _{X}$
for every
$d(gx,gy)\leq \delta _{X}$
for every
![]() $g\in G$
, thus
$g\in G$
, thus
![]() $x=y$
by expansiveness. By continuity, for large enough i, we must have
$x=y$
by expansiveness. By continuity, for large enough i, we must have
![]() $d(\tau (x_{E_{i}}),\tau (y_{E_{i}}))\leq \delta _{Y}$
, a contradiction.
$d(\tau (x_{E_{i}}),\tau (y_{E_{i}}))\leq \delta _{Y}$
, a contradiction.
What follows next is a list of definitions of several specification properties that will be used in this paper.
Definition 2.10. Let X be a G-space. We say that the following hold.
-
• X has the weak specification property if for every
 $\varepsilon>0$
, there exists a finite symmetric
$\varepsilon>0$
, there exists a finite symmetric
 $S\subseteq G$
such that for every subsets
$S\subseteq G$
such that for every subsets
 $A_{1},A_{2}\subseteq G$
and
$A_{1},A_{2}\subseteq G$
and
 $x_{1},x_{2}\in X$
such that
$x_{1},x_{2}\in X$
such that
 $S\cdot A_{1}\cap A_{2}=\emptyset $
, there is
$S\cdot A_{1}\cap A_{2}=\emptyset $
, there is
 $y\in X$
satisfying
$y\in X$
satisfying
 $d(gx_{i},gz)<\varepsilon $
,
$d(gx_{i},gz)<\varepsilon $
,
 $i\in \{1,2\}$
and
$i\in \{1,2\}$
and
 $g\in A_{i}$
.
$g\in A_{i}$
. -
• X has the pseudo-orbit tracing property (POTP), or shadowing, if for every
 $\varepsilon>0$
, there exist
$\varepsilon>0$
, there exist
 $\gamma>0$
and finite
$\gamma>0$
and finite
 $S\subseteq G$
such that for every G-indexed set
$S\subseteq G$
such that for every G-indexed set
 $(x_{g})_{g\in G}\subseteq X$
satisfying
$(x_{g})_{g\in G}\subseteq X$
satisfying
 $d(s x_{g}, x_{sg})<\gamma $
, for all
$d(s x_{g}, x_{sg})<\gamma $
, for all
 $g\in G$
and
$g\in G$
and
 $s\in F$
, there exists
$s\in F$
, there exists
 $z\in X$
such that
$z\in X$
such that
 $d(gz,x_{g})<\varepsilon $
for every
$d(gz,x_{g})<\varepsilon $
for every
 $g\in G$
.
$g\in G$
. -
• X has the strong topological Markov property if for every
 $\varepsilon>0$
, there exist
$\varepsilon>0$
, there exist
 $\gamma>0$
and finite
$\gamma>0$
and finite
 $S\subseteq G$
such that for every
$S\subseteq G$
such that for every
 $x,y\in X$
and every finite
$x,y\in X$
and every finite
 $A\subseteq G$
satisfying
$A\subseteq G$
satisfying
 $d_{SA\setminus A}(x,y)<\gamma $
, there exists
$d_{SA\setminus A}(x,y)<\gamma $
, there exists
 $z\in X$
such that
$z\in X$
such that
 $d_{A}(z,x)<\varepsilon $
and
$d_{A}(z,x)<\varepsilon $
and
 $d_{G\setminus A}(z,y)<\varepsilon $
.
$d_{G\setminus A}(z,y)<\varepsilon $
. -
• X has the uniform strong topological Markov property if for every
 $\varepsilon>0$
, there exist
$\varepsilon>0$
, there exist
 $\gamma>0$
and finite
$\gamma>0$
and finite
 $S\subseteq G$
such that for every subset
$S\subseteq G$
such that for every subset
 $A\subseteq G$
and every
$A\subseteq G$
and every
 $V\subseteq G$
with
$V\subseteq G$
with
 $S\cdot A\cdot v_{1}\cap S\cdot A\cdot v_{2}=\emptyset $
, for
$S\cdot A\cdot v_{1}\cap S\cdot A\cdot v_{2}=\emptyset $
, for
 $v_{1}\neq v_{2}\in V$
and every V-indexed set
$v_{1}\neq v_{2}\in V$
and every V-indexed set
 $(x_{v})_{v\in V}\subseteq X$
and an element
$(x_{v})_{v\in V}\subseteq X$
and an element
 $y\in X$
satisfying
$y\in X$
satisfying
 $d_{(SA\setminus A)v} (x_{v},y)<\gamma $
, for all
$d_{(SA\setminus A)v} (x_{v},y)<\gamma $
, for all
 $v\in V$
, there exists
$v\in V$
, there exists
 $z\in X$
such that
$z\in X$
such that
 $d_{Av} (x_{v},z)<\varepsilon $
, for all
$d_{Av} (x_{v},z)<\varepsilon $
, for all
 $v\in V$
, and
$v\in V$
, and
 $d_{G\setminus AV}(z,y)<\varepsilon $
.
$d_{G\setminus AV}(z,y)<\varepsilon $
.
Remark 2.11. The weak specification in this generality for general group actions was defined in [Reference Chung and Li19], the pseudo-orbit tracing property (or shadowing) in this generality in [Reference Osipov and Tikhomirov40]. The topological Markov properties in this generality were introduced in the recent [Reference Barbieri, García-Ramos and Li1], building on previous definitions from [Reference Gromov29, §8.C] (under the name ‘spliceability’), [Reference Barbieri, Gómez, Marcus and Taati2, Reference Chandgotia, Han, Marcus, Meyerovitch and Pavlov17], where it was, in particular, shown that for expansive actions, the uniform strong topological Markov property and the strong topological Markov property are the same.
Lemma 2.12. Let G act expansively on a compact metric space X with an expansiveness constant
![]() $\delta>0$
. Then X has the pseudo-orbit tracing property if and only if there is a finite set
$\delta>0$
. Then X has the pseudo-orbit tracing property if and only if there is a finite set
![]() $F\subseteq G$
such that for every G-indexed set
$F\subseteq G$
such that for every G-indexed set
![]() $(x_{g})_{g\in G}$
satisfying
$(x_{g})_{g\in G}$
satisfying
![]() $d(s x_{g},x_{sg})\leq \delta /2$
for every
$d(s x_{g},x_{sg})\leq \delta /2$
for every
![]() $g\in G$
and
$g\in G$
and
![]() $s\in F$
, there is
$s\in F$
, there is
![]() $z\in X$
such that
$z\in X$
such that
![]() $d(gx,x_{g})\leq \delta /2$
for every
$d(gx,x_{g})\leq \delta /2$
for every
![]() $g\in G$
.
$g\in G$
.
Proof. Suppose that X has the POTP. Apply the definition for
![]() $\delta /2$
as
$\delta /2$
as
![]() $\varepsilon $
to get some
$\varepsilon $
to get some
![]() $\gamma $
and a finite set
$\gamma $
and a finite set
![]() $S\subseteq G$
. Let
$S\subseteq G$
. Let
![]() $D_{\gamma }$
be a finite symmetric set from Lemma 2.2 and set
$D_{\gamma }$
be a finite symmetric set from Lemma 2.2 and set
![]() $F:=D_{\gamma }\cdot S$
, which we claim to be the desired finite set. Indeed, let
$F:=D_{\gamma }\cdot S$
, which we claim to be the desired finite set. Indeed, let
![]() $(x_{g})_{g\in G}\subseteq X$
be a G-indexed set satisfying
$(x_{g})_{g\in G}\subseteq X$
be a G-indexed set satisfying
![]() $d(t x_{g},x_{tg})\leq \delta /2$
for every
$d(t x_{g},x_{tg})\leq \delta /2$
for every
![]() $g\in G$
and
$g\in G$
and
![]() $t\in F$
. We claim that for every
$t\in F$
. We claim that for every
![]() $g\in G$
and
$g\in G$
and
![]() $s\in S$
, we have
$s\in S$
, we have
![]() $d(s x_{g},x_{sg})\leq \gamma $
which will be enough. Fix
$d(s x_{g},x_{sg})\leq \gamma $
which will be enough. Fix
![]() $g\in G$
and
$g\in G$
and
![]() $s\in S$
. Then for every
$s\in S$
. Then for every
![]() $t\in D_{\gamma }$
, we have
$t\in D_{\gamma }$
, we have
which by definition of
![]() $D_{\gamma }$
implies that
$D_{\gamma }$
implies that
![]() $d(s x_{g},x_{sg})\leq \gamma $
as needed.
$d(s x_{g},x_{sg})\leq \gamma $
as needed.
Now conversely suppose X satisfies the condition from the statement and let us show it has the POTP. Fix
![]() $\varepsilon>0$
. We claim that
$\varepsilon>0$
. We claim that
![]() $\gamma =\delta /2$
and
$\gamma =\delta /2$
and
![]() $S=D_{\varepsilon }\cdot F$
are as desired. Let
$S=D_{\varepsilon }\cdot F$
are as desired. Let
![]() $(x_{g})_{g\in G}\subseteq X$
be such that
$(x_{g})_{g\in G}\subseteq X$
be such that
![]() $d(s x_{g},x_{sg})\leq \delta /2$
for every
$d(s x_{g},x_{sg})\leq \delta /2$
for every
![]() $g\in G$
and
$g\in G$
and
![]() $s\in S$
. Since
$s\in S$
. Since
![]() $F\subseteq S$
, we get that there is
$F\subseteq S$
, we get that there is
![]() $z\in X$
satisfying
$z\in X$
satisfying
![]() $d(gz,x_{g})\leq \delta /2$
for every
$d(gz,x_{g})\leq \delta /2$
for every
![]() $g\in G$
. We claim that in fact
$g\in G$
. We claim that in fact
![]() $d(gz,x_{g})\leq \varepsilon $
is true. Indeed, fix
$d(gz,x_{g})\leq \varepsilon $
is true. Indeed, fix
![]() $g\in G$
. We check that for every
$g\in G$
. We check that for every
![]() $t\in D_{\varepsilon }$
, we have
$t\in D_{\varepsilon }$
, we have
![]() $d((tg)z,t x_{g})\leq \delta $
which will prove the claim. So take
$d((tg)z,t x_{g})\leq \delta $
which will prove the claim. So take
![]() $t\in D_{\varepsilon }$
, we have
$t\in D_{\varepsilon }$
, we have
as desired.
The following two lemmas are proved analogously as Lemma 2.12 and the proofs are left to the reader.
Lemma 2.13. Let G act expansively on a compact metric space X with an expansiveness constant
![]() $\delta>0$
. Then X has the weak specification property if and only if there is a finite symmetric set
$\delta>0$
. Then X has the weak specification property if and only if there is a finite symmetric set
![]() $F\subseteq G$
such that for every subsets
$F\subseteq G$
such that for every subsets
![]() $A_{1},A_{2}\subseteq G$
and
$A_{1},A_{2}\subseteq G$
and
![]() $x_{1},x_{2}\in X$
such that
$x_{1},x_{2}\in X$
such that
![]() $A_{1}\cdot F\cap A_{2}=\emptyset $
, there is
$A_{1}\cdot F\cap A_{2}=\emptyset $
, there is
![]() $y\in X$
satisfying
$y\in X$
satisfying
![]() $d(gx_{i},gz)<\delta /2$
,
$d(gx_{i},gz)<\delta /2$
,
![]() $i\in \{1,2\}$
and
$i\in \{1,2\}$
and
![]() $g\in A_{i}$
.
$g\in A_{i}$
.
Lemma 2.14. Let G act expansively on a compact metric space X with an expansiveness constant
![]() $\delta>0$
. Then X has the strong topological Markov property if and only if there is a finite symmetric set
$\delta>0$
. Then X has the strong topological Markov property if and only if there is a finite symmetric set
![]() $F\subseteq G$
containing the unit such that for every finite set
$F\subseteq G$
containing the unit such that for every finite set
![]() $A\subseteq G$
, every
$A\subseteq G$
, every
![]() $V\subseteq G$
with
$V\subseteq G$
with
![]() $v_{1}\notin FAv_{2}$
, for
$v_{1}\notin FAv_{2}$
, for
![]() $v_{1}\neq v_{2}\in V$
and every V-indexed set
$v_{1}\neq v_{2}\in V$
and every V-indexed set
![]() $(x_{v})_{v\in V}\subseteq X$
and an element
$(x_{v})_{v\in V}\subseteq X$
and an element
![]() $y\in X$
satisfying
$y\in X$
satisfying
![]() $d_{(FA\setminus A)v} (x_{v},y)<\delta /2$
, for all
$d_{(FA\setminus A)v} (x_{v},y)<\delta /2$
, for all
![]() $v\in V$
, there exists
$v\in V$
, there exists
![]() $z\in X$
such that
$z\in X$
such that
![]() $d_{Av} (x_{v},z)<\delta /2$
, for all
$d_{Av} (x_{v},z)<\delta /2$
, for all
![]() $v\in V$
, and
$v\in V$
, and
![]() $d_{G\setminus AV}(z,y)<\delta /2$
.
$d_{G\setminus AV}(z,y)<\delta /2$
.
Remark 2.15. If the expansiveness constant
![]() $\delta>0$
of a G-space X is fixed and clear from the context, we can then obtain the finite set
$\delta>0$
of a G-space X is fixed and clear from the context, we can then obtain the finite set
![]() $F\subseteq G$
from the statement of Lemma 2.12 called a pseudo-orbit tracing set. It also follows from Lemma 2.12 that if X has the POTP and
$F\subseteq G$
from the statement of Lemma 2.12 called a pseudo-orbit tracing set. It also follows from Lemma 2.12 that if X has the POTP and
![]() $F\subseteq G$
is a pseudo-orbit tracing set for X, then if
$F\subseteq G$
is a pseudo-orbit tracing set for X, then if
![]() $(x_{G})_{g\in G}\subseteq X$
is a pseudoorbit for
$(x_{G})_{g\in G}\subseteq X$
is a pseudoorbit for
![]() $D_{\varepsilon }\cdot F$
, meaning that
$D_{\varepsilon }\cdot F$
, meaning that
![]() $d(tx_{g},x_{tg})\leq \delta /2$
for every
$d(tx_{g},x_{tg})\leq \delta /2$
for every
![]() $g\in G$
and
$g\in G$
and
![]() $t\in D_{\varepsilon }\cdot F$
, then there is
$t\in D_{\varepsilon }\cdot F$
, then there is
![]() $z\in X$
satisfying
$z\in X$
satisfying
![]() $d(gz,x_{g})\leq \varepsilon $
for every
$d(gz,x_{g})\leq \varepsilon $
for every
![]() $g\in G$
.
$g\in G$
.
Analogously, we can call the finite set
![]() $F\subseteq G$
from Lemma 2.13 a weak specification set. If we need the weak specification with particular
$F\subseteq G$
from Lemma 2.13 a weak specification set. If we need the weak specification with particular
![]() $\varepsilon>0$
, the weak specification set F can be replaced by
$\varepsilon>0$
, the weak specification set F can be replaced by
![]() $D_{\varepsilon }\cdot F$
.
$D_{\varepsilon }\cdot F$
.
Moreover, we can call the finite set
![]() $F\subseteq G$
from Lemma 2.14 a strong topological Markov set. If we need this property with a specific
$F\subseteq G$
from Lemma 2.14 a strong topological Markov set. If we need this property with a specific
![]() $\varepsilon $
, the strong topological Markov set F can be replaced by
$\varepsilon $
, the strong topological Markov set F can be replaced by
![]() $D_{\varepsilon }\cdot F$
.
$D_{\varepsilon }\cdot F$
.
3 Garden of Eden theorem
In this section, we prove one of the two main results of the paper and we establish the Garden of Eden theorem for continuous and expansive actions of amenable groups on compact metrizable spaces satisfying the strong topological Markov and weak specification properties.
We shall need the definition of a tiling of a group.
Definition 3.1. Let G be a group and
![]() $A\subseteq B\subseteq G$
be two finite subsets. We say that a subset
$A\subseteq B\subseteq G$
be two finite subsets. We say that a subset
![]() $T\subseteq G$
is an
$T\subseteq G$
is an
![]() $(A,B)$
-tiling of G if the following hold:
$(A,B)$
-tiling of G if the following hold:
-
• for
 $s\neq t\in T$
, the sets
$s\neq t\in T$
, the sets
 $A\cdot t$
and
$A\cdot t$
and
 $A\cdot s$
are disjoint; and
$A\cdot s$
are disjoint; and -
•
 $\bigcup _{t\in T} B\cdot t=G$
.
$\bigcup _{t\in T} B\cdot t=G$
.
3.1 The metrizable case
Theorem 3.2. Let G be a countable amenable group and X an expansive and compact G-metric space satisfying the weak specification property and the strong topological Markov property. Then X satisfies the Moore–Myhill property.
Proof. Fix an expansiveness constant
![]() $\delta>0$
. Let us show the Moore property. Let
$\delta>0$
. Let us show the Moore property. Let
![]() ${\tau :X\rightarrow X}$
be a surjective continuous G-equivariant map. We show it is pre-injective. Suppose the contrary and choose
${\tau :X\rightarrow X}$
be a surjective continuous G-equivariant map. We show it is pre-injective. Suppose the contrary and choose
![]() $x\sim y\in X$
satisfying
$x\sim y\in X$
satisfying
![]() $\tau (x)=\tau (y)$
. Set
$\tau (x)=\tau (y)$
. Set
![]() $\Lambda :=\Lambda _{\delta }(x,y)$
, which is by Corollary 2.6 finite and we may assume it is non-empty and contains
$\Lambda :=\Lambda _{\delta }(x,y)$
, which is by Corollary 2.6 finite and we may assume it is non-empty and contains
![]() $1_{G}$
. Also, let
$1_{G}$
. Also, let
![]() $S^{\prime }\subseteq G$
be a finite symmetric memory set for
$S^{\prime }\subseteq G$
be a finite symmetric memory set for
![]() $\tau $
. Finally, let
$\tau $
. Finally, let
![]() $S\subseteq G$
be a finite symmetric subset containing
$S\subseteq G$
be a finite symmetric subset containing
![]() $1_{G}$
such that the following hold:
$1_{G}$
such that the following hold:
-
(1) S is a weak specification and strong topological Markov set for X;
-
(2)
 $(S^{\prime })^{2}\subseteq S$
;
$(S^{\prime })^{2}\subseteq S$
; -
(3)
 $\text {for all } u\in X\text { for all } g\in S^{\prime }\cdot D_{\delta /8}\cdot \Lambda \; (d_{S}(gu,gy)\leq \delta \Rightarrow d(\tau (gu),\tau (gx))\leq \delta /2).$
$\text {for all } u\in X\text { for all } g\in S^{\prime }\cdot D_{\delta /8}\cdot \Lambda \; (d_{S}(gu,gy)\leq \delta \Rightarrow d(\tau (gu),\tau (gx))\leq \delta /2).$
Now set
![]() $E:=D_{\delta /8}\cdot S\cdot D_{\delta /8}\cdot \Lambda $
. Since all of the finite sets involved in the product may be assumed to be symmetric and contain
$E:=D_{\delta /8}\cdot S\cdot D_{\delta /8}\cdot \Lambda $
. Since all of the finite sets involved in the product may be assumed to be symmetric and contain
![]() $1_{G}$
, we expect E to satisfy the same. By [Reference Ceccherini-Silberstein and Coornaert8, Proposition 5.6.3], there exists an
$1_{G}$
, we expect E to satisfy the same. By [Reference Ceccherini-Silberstein and Coornaert8, Proposition 5.6.3], there exists an
![]() $(E^{2},E^{4})$
-tiling of G, denoted by T. Let Z be the closed (not necessarily G-invariant) subset
$(E^{2},E^{4})$
-tiling of G, denoted by T. Let Z be the closed (not necessarily G-invariant) subset
Claim 3.3. We have
![]() $\tau [Z]=\tau [X]$
.
$\tau [Z]=\tau [X]$
.
That is, for every
![]() $w\in X$
, there exists
$w\in X$
, there exists
![]() $z\in Z$
satisfying
$z\in Z$
satisfying
![]() $\tau (w)=\tau (z)$
. Fix
$\tau (w)=\tau (z)$
. Fix
![]() $w\in X$
. What follows is the proof of the existence of such z that will be divided into three steps.
$w\in X$
. What follows is the proof of the existence of such z that will be divided into three steps.
Step 1. Finding z using the strong topological Markov property.
We define a T-indexed set
![]() $(z_{t})_{t\in T}$
in the following way. For
$(z_{t})_{t\in T}$
in the following way. For
![]() $t\in T$
, we set
$t\in T$
, we set
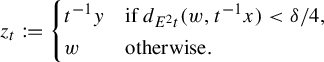 $$ \begin{align*}z_{t}:=\begin{cases}t^{-1}y & \text{if }d_{E^{2} t}(w,t^{-1}x)<\delta/4,\\ w & \text{otherwise.} \end{cases}\end{align*} $$
$$ \begin{align*}z_{t}:=\begin{cases}t^{-1}y & \text{if }d_{E^{2} t}(w,t^{-1}x)<\delta/4,\\ w & \text{otherwise.} \end{cases}\end{align*} $$
We want to use the strong topological Markov property with the constant
![]() $\delta /8$
, thus with the set
$\delta /8$
, thus with the set
![]() $P:=D_{\delta /8}\cdot S$
, for
$P:=D_{\delta /8}\cdot S$
, for
![]() $A=E$
, for
$A=E$
, for
![]() $V=T$
, where the T-indexed set is
$V=T$
, where the T-indexed set is
![]() $(z_{t})_{t\in T}$
, and for
$(z_{t})_{t\in T}$
, and for
![]() $y=w$
. Since T is a
$y=w$
. Since T is a
![]() $(E^{2},E^{4})$
-tiling and
$(E^{2},E^{4})$
-tiling and
![]() $P\subseteq E$
, we have that
$P\subseteq E$
, we have that
![]() $P\cdot E\cdot t_{1}\cap P\cdot E\cdot t_{2}=\emptyset $
, for every
$P\cdot E\cdot t_{1}\cap P\cdot E\cdot t_{2}=\emptyset $
, for every
![]() $t_{1}\neq t_{2}\in T$
. So we need to check that for every
$t_{1}\neq t_{2}\in T$
. So we need to check that for every
![]() $t\in T$
, we have
$t\in T$
, we have
![]() ${d_{(PE\setminus E)t}(z_{t},w)<\delta /2}$
. Fix
${d_{(PE\setminus E)t}(z_{t},w)<\delta /2}$
. Fix
![]() $t\in T$
. If
$t\in T$
. If
![]() $d_{E^{2} t}(w,t^{-1}x)\geq \delta /4$
, then
$d_{E^{2} t}(w,t^{-1}x)\geq \delta /4$
, then
![]() $z_{t}=w$
and the inequality
$z_{t}=w$
and the inequality
![]() $d_{(PE\setminus E)t}(z_{t},w)<\delta /2$
is obvious. So suppose that
$d_{(PE\setminus E)t}(z_{t},w)<\delta /2$
is obvious. So suppose that
![]() $d_{E^{2} t}(w,t^{-1}x)<\delta /4$
and therefore
$d_{E^{2} t}(w,t^{-1}x)<\delta /4$
and therefore
![]() $z_{t}=t^{-1}y$
. Since
$z_{t}=t^{-1}y$
. Since
![]() $d_{G\setminus D_{\delta /8}\cdot \Lambda }(x,y)<\delta /8$
and
$d_{G\setminus D_{\delta /8}\cdot \Lambda }(x,y)<\delta /8$
and
![]() $D_{\delta /8}\cdot \Lambda \subseteq E$
, we have
$D_{\delta /8}\cdot \Lambda \subseteq E$
, we have
![]() $d_{(PE\setminus E)t}(t^{-1}x,t^{-1}y)<\delta /8$
. Since
$d_{(PE\setminus E)t}(t^{-1}x,t^{-1}y)<\delta /8$
. Since
![]() $P\subseteq E$
, we also have by the assumption
$P\subseteq E$
, we also have by the assumption
![]() $d_{(PE\setminus E)t} (w,t^{-1}x)<\delta /4$
, so by the triangle inequality, we get
$d_{(PE\setminus E)t} (w,t^{-1}x)<\delta /4$
, so by the triangle inequality, we get
![]() $d_{(PE\setminus E)t}(z_{t},w)<\delta /2$
as desired.
$d_{(PE\setminus E)t}(z_{t},w)<\delta /2$
as desired.
By the strong topological Markov property, it follows that there exists
![]() $z\in X$
such that
$z\in X$
such that
![]() $d_{Et}(z,z_{t})<\delta /2$
, for all
$d_{Et}(z,z_{t})<\delta /2$
, for all
![]() $t\in T$
, and
$t\in T$
, and
![]() $d_{G\setminus FT}(z,w)<\delta /2$
.
$d_{G\setminus FT}(z,w)<\delta /2$
.
Step 2. We claim that
![]() $z\in Z$
.
$z\in Z$
.
Suppose the contrary. Then there is
![]() $t\in T$
such that
$t\in T$
such that
![]() $d_{E^{2} t}(z,t^{-1}x)<\delta /8$
. Either we have
$d_{E^{2} t}(z,t^{-1}x)<\delta /8$
. Either we have
![]() $z_{t}=w$
, if
$z_{t}=w$
, if
![]() $d_{E^{2}t}(w,t^{-1}x)\geq \delta /4$
, in which case
$d_{E^{2}t}(w,t^{-1}x)\geq \delta /4$
, in which case
![]() $d_{E^{2}t}(z,w)\leq \delta /8$
, so by the triangle inequality,
$d_{E^{2}t}(z,w)\leq \delta /8$
, so by the triangle inequality,
![]() $d_{E^{2}t}(z,t^{-1}x)\geq \delta /8$
, a contradiction.
$d_{E^{2}t}(z,t^{-1}x)\geq \delta /8$
, a contradiction.
Or we have
![]() $z_{t}=t^{-1}y$
if
$z_{t}=t^{-1}y$
if
![]() $d_{E^{2}t}(w,t^{-1}x)<\delta /4$
, in which case
$d_{E^{2}t}(w,t^{-1}x)<\delta /4$
, in which case
![]() $d_{Et}(z,t^{-1}y)\leq \delta /8$
. Since
$d_{Et}(z,t^{-1}y)\leq \delta /8$
. Since
![]() $d_{Et}(t^{-1}x,t^{-1}y)>\delta $
, by the triangle inequality, we have
$d_{Et}(t^{-1}x,t^{-1}y)>\delta $
, by the triangle inequality, we have
another contradiction.
Step 3. We claim that
![]() $\tau (z)=\tau (w)$
.
$\tau (z)=\tau (w)$
.
Suppose again the contrary. Then there exists
![]() $g\in G$
such that
$g\in G$
such that
Define
![]() $V\subseteq T$
to be the set
$V\subseteq T$
to be the set
![]() $\{t\in T\colon d_{E^{2} t}(w,t^{-1}x)<\delta /4\}$
. We have two cases. Either there exists
$\{t\in T\colon d_{E^{2} t}(w,t^{-1}x)<\delta /4\}$
. We have two cases. Either there exists
![]() $t\in V$
such that
$t\in V$
such that
![]() $S^{\prime }g\cap Et\neq \emptyset $
, or not. In the latter case, we have that for every
$S^{\prime }g\cap Et\neq \emptyset $
, or not. In the latter case, we have that for every
![]() $s\in S^{\prime }$
,
$s\in S^{\prime }$
,
thus since
![]() $S^{\prime }$
is a memory set for
$S^{\prime }$
is a memory set for
![]() $\tau $
, we get
$\tau $
, we get
![]() $d(\tau (gz),\tau (gw))\leq \delta $
, a contradiction.
$d(\tau (gz),\tau (gw))\leq \delta $
, a contradiction.
So suppose that there is
![]() $t\in V$
such that
$t\in V$
such that
![]() $S^{\prime }g\cap Et\neq \emptyset $
. Then we distinguish three more subscases:
$S^{\prime }g\cap Et\neq \emptyset $
. Then we distinguish three more subscases:
-
• either
 $S^{\prime }g\subseteq Et$
, however,
$S^{\prime }g\subseteq Et$
, however,
 $S^{\prime }g\cap D_{\delta /8}\cdot \Lambda t=\emptyset $
;
$S^{\prime }g\cap D_{\delta /8}\cdot \Lambda t=\emptyset $
; -
• or
 $S^{\prime }g\cap D_{\delta /8}\cdot \Lambda t\neq \emptyset $
;
$S^{\prime }g\cap D_{\delta /8}\cdot \Lambda t\neq \emptyset $
; -
• or
 $S^{\prime }g\nsubseteq Et$
.
$S^{\prime }g\nsubseteq Et$
.
In the first case, we have for every
![]() $s\in S^{\prime }$
,
$s\in S^{\prime }$
,
and since
![]() $S^{\prime }g\cap D_{\delta /8}\cdot \Lambda t=\emptyset $
, we also have for every
$S^{\prime }g\cap D_{\delta /8}\cdot \Lambda t=\emptyset $
, we also have for every
![]() $s\in S^{\prime }$
,
$s\in S^{\prime }$
,
so for every
![]() $s\in S^{\prime }$
, we have
$s\in S^{\prime }$
, we have
Combining this with the fact that, since
![]() $t\in V$
, for every
$t\in V$
, for every
![]() $s\in S^{\prime }$
,
$s\in S^{\prime }$
,
we finally obtain that for all
![]() $s\in S^{\prime }$
,
$s\in S^{\prime }$
,
thus, since
![]() $S^{\prime }$
is a memory set for
$S^{\prime }$
is a memory set for
![]() $\tau $
,
$\tau $
,
![]() $d(\tau (gz),\tau (gw))\leq \delta $
, again a contradiction.
$d(\tau (gz),\tau (gw))\leq \delta $
, again a contradiction.
In the second case, we have
![]() $g\in S^{\prime }\cdot D_{\delta /8}\cdot \Lambda t$
. Then, since
$g\in S^{\prime }\cdot D_{\delta /8}\cdot \Lambda t$
. Then, since
![]() $S\cdot g\subseteq Et$
, we have
$S\cdot g\subseteq Et$
, we have
since for every
![]() $s\in S$
, we have
$s\in S$
, we have
![]() $d((sg)z,(sgt^{-1})y)\leq \delta /8$
, so by item
$d((sg)z,(sgt^{-1})y)\leq \delta /8$
, so by item
![]() $(3)$
, we get
$(3)$
, we get
However, since
![]() $d_{D_{\delta /8}\cdot S^{\prime }}(gw,(gt^{-1})x)<\delta /4$
and
$d_{D_{\delta /8}\cdot S^{\prime }}(gw,(gt^{-1})x)<\delta /4$
and
![]() $S^{\prime }$
is a memory set for
$S^{\prime }$
is a memory set for
![]() $\tau $
, we get
$\tau $
, we get
So finally, by triangle inequality, we get
which is again a contradiction.
For the last third case, pick
![]() $s\in S^{\prime }$
. If
$s\in S^{\prime }$
. If
![]() $sg\notin Et$
, then
$sg\notin Et$
, then
![]() $d((sg)z,(sg)w)\leq \delta /8$
. If
$d((sg)z,(sg)w)\leq \delta /8$
. If
![]() $sg\in Et$
, then we have
$sg\in Et$
, then we have
![]() $d((sg)z,(sgt^{-1})y)\leq \delta /8$
. However, since there is by assumption
$d((sg)z,(sgt^{-1})y)\leq \delta /8$
. However, since there is by assumption
![]() $s^{\prime }\in S^{\prime }$
such that
$s^{\prime }\in S^{\prime }$
such that
![]() $s^{\prime }g\notin Et$
, we have
$s^{\prime }g\notin Et$
, we have
![]() $sg\notin D_{\delta /8}\cdot \Lambda t$
since otherwise
$sg\notin D_{\delta /8}\cdot \Lambda t$
since otherwise
![]() $s^{\prime }s^{-1}sg\in Et$
as
$s^{\prime }s^{-1}sg\in Et$
as
![]() $s^{\prime }s^{-1}\in S$
and
$s^{\prime }s^{-1}\in S$
and
![]() $S\cdot D_{\delta 8}\cdot \Lambda \subseteq E$
. It follows that
$S\cdot D_{\delta 8}\cdot \Lambda \subseteq E$
. It follows that
![]() $d((sgt^{-1})y,(sgt^{-1})x)<\delta /8$
and so by the triangle inequality,
$d((sgt^{-1})y,(sgt^{-1})x)<\delta /8$
and so by the triangle inequality,
Combining this with the fact that
![]() $d((sg)w,(sgt^{-1})x)<\delta /4$
, we finally obtain by another application of the triangle inequality that
$d((sg)w,(sgt^{-1})x)<\delta /4$
, we finally obtain by another application of the triangle inequality that
So again, since S is a memory set for
![]() $\tau $
, we get
$\tau $
, we get
![]() $d(\tau (gz),\tau (gw))\leq \delta $
, a contradiction. This finishes the proof of Claim 3.3.
$d(\tau (gz),\tau (gw))\leq \delta $
, a contradiction. This finishes the proof of Claim 3.3.
Claim 3.4. We have
![]() $h_{\mathrm {top}}(Z)<h_{\mathrm {top}}(X)$
.
$h_{\mathrm {top}}(Z)<h_{\mathrm {top}}(X)$
.
First notice that for every closed subset
![]() $Y\subseteq X$
,
$Y\subseteq X$
,
![]() $\gamma>0$
, and every
$\gamma>0$
, and every
![]() $E\subseteq F\subseteq G$
, where
$E\subseteq F\subseteq G$
, where
![]() $E,F$
are finite, we have
$E,F$
are finite, we have
Indeed, suppose the contrary and let
![]() $N\subseteq Y$
be a
$N\subseteq Y$
be a
![]() $\gamma $
-separated subset of
$\gamma $
-separated subset of
![]() $(Y,d_{F})$
of size strictly bigger than
$(Y,d_{F})$
of size strictly bigger than
![]() ${\mathrm {sep}}(Y,\gamma ,F\setminus E)\cdot {\mathrm {sep}}(Y,\gamma /2,E)$
. Let
${\mathrm {sep}}(Y,\gamma ,F\setminus E)\cdot {\mathrm {sep}}(Y,\gamma /2,E)$
. Let
![]() $M\subseteq N$
be a maximal
$M\subseteq N$
be a maximal
![]() $\gamma /2$
-separated subset of N with respect to
$\gamma /2$
-separated subset of N with respect to
![]() $d_{E}$
. Since
$d_{E}$
. Since
![]() $|N|>{\mathrm {sep}}(Y,\delta ,F\setminus E)\cdot {\mathrm {sep}}(Y,\delta /2,E)$
, while
$|N|>{\mathrm {sep}}(Y,\delta ,F\setminus E)\cdot {\mathrm {sep}}(Y,\delta /2,E)$
, while
![]() $|M|\leq {\mathrm {sep}}(Y,\gamma /2,E)$
, there must exist
$|M|\leq {\mathrm {sep}}(Y,\gamma /2,E)$
, there must exist
![]() $m\in M$
and set
$m\in M$
and set
![]() $A\subseteq N$
of size strictly bigger than
$A\subseteq N$
of size strictly bigger than
![]() ${\mathrm {sep}}(Y,\gamma , F\setminus E)$
such that for every
${\mathrm {sep}}(Y,\gamma , F\setminus E)$
such that for every
![]() $a\in A$
, we have
$a\in A$
, we have
![]() $d_{E}(a,m)\leq \gamma /2$
. It follows, by triangle inequality, that for every
$d_{E}(a,m)\leq \gamma /2$
. It follows, by triangle inequality, that for every
![]() $a,b\in A$
, we have
$a,b\in A$
, we have
![]() $d_{E}(a,b)\leq \gamma $
. However, for every
$d_{E}(a,b)\leq \gamma $
. However, for every
![]() $a\neq b\in A$
, we have
$a\neq b\in A$
, we have
![]() $d_{F}(a,b)>\gamma $
, so there must be
$d_{F}(a,b)>\gamma $
, so there must be
![]() $e\in F\setminus E$
such that
$e\in F\setminus E$
such that
![]() $d(ea,eb)>\gamma $
. It follows that A is
$d(ea,eb)>\gamma $
. It follows that A is
![]() $\gamma $
-separated for
$\gamma $
-separated for
![]() $d_{F\setminus E}$
which contradicts that
$d_{F\setminus E}$
which contradicts that
![]() $|A|>{\mathrm {sep}}(Y,\gamma , F\setminus E)$
.
$|A|>{\mathrm {sep}}(Y,\gamma , F\setminus E)$
.
Now fix
![]() $j\in {\mathbb {N}}$
. Enumerate
$j\in {\mathbb {N}}$
. Enumerate
![]() $T_{j}$
as
$T_{j}$
as
![]() $\{t_{1},\ldots ,t_{n}\}$
. For every
$\{t_{1},\ldots ,t_{n}\}$
. For every
![]() $1\leq i\leq n$
, define
$1\leq i\leq n$
, define
Moreover, set
![]() $Z_{0}:=X$
. Next we claim that for every
$Z_{0}:=X$
. Next we claim that for every
![]() $1\leq i\leq n$
, we have
$1\leq i\leq n$
, we have
where
![]() $\Sigma _{i-1}:=1/{\mathrm {sep}}(Z_{i-1},E t_{i},\delta /2)$
. To show that, pick an arbitrary
$\Sigma _{i-1}:=1/{\mathrm {sep}}(Z_{i-1},E t_{i},\delta /2)$
. To show that, pick an arbitrary
![]() $\delta /2$
-separated set
$\delta /2$
-separated set
![]() $N\subseteq Z_{i-1}$
with respect to
$N\subseteq Z_{i-1}$
with respect to
![]() $d_{F_{j}\setminus E t_{i}}$
. By the weak specification property (recall that S is a weak specification set) and since E contains
$d_{F_{j}\setminus E t_{i}}$
. By the weak specification property (recall that S is a weak specification set) and since E contains
![]() $D_{\delta /8}\cdot S\cdot \Lambda $
, for each
$D_{\delta /8}\cdot S\cdot \Lambda $
, for each
![]() $w\in N$
, we can find
$w\in N$
, we can find
![]() $w_{x}\in X$
such that the following hold:
$w_{x}\in X$
such that the following hold:
-
• for every
 $e\in F_{j}\setminus E t_{i}$
, we have
$e\in F_{j}\setminus E t_{i}$
, we have
 $d(e w, e w_{x})<\delta /8$
;
$d(e w, e w_{x})<\delta /8$
; -
• for every
 $g\in \Lambda t_{i}$
, we have
$g\in \Lambda t_{i}$
, we have
 $d((g t_{i}^{-1}) x, g w_{x})<\delta /8$
.
$d((g t_{i}^{-1}) x, g w_{x})<\delta /8$
.
In particular, it follows that
![]() $N_{x}:=\{w_{x}\colon w\in N\}\subseteq ((X\setminus Z_{i})\cap Z_{i-1})$
and that
$N_{x}:=\{w_{x}\colon w\in N\}\subseteq ((X\setminus Z_{i})\cap Z_{i-1})$
and that
![]() $N_{x}$
is
$N_{x}$
is
![]() $\delta /4$
-separated with respect to
$\delta /4$
-separated with respect to
![]() $d_{F_{j}\setminus E t_{i}}$
. Since by (1) we have
$d_{F_{j}\setminus E t_{i}}$
. Since by (1) we have
![]() ${\mathrm {sep}}(Z_{i-1}, F_{j}\setminus E t_{i},\delta /2)\geq {\mathrm {sep}}(Z_{i-1},F_{j},\delta /4)\cdot \Sigma _{i}$
, we obtain
${\mathrm {sep}}(Z_{i-1}, F_{j}\setminus E t_{i},\delta /2)\geq {\mathrm {sep}}(Z_{i-1},F_{j},\delta /4)\cdot \Sigma _{i}$
, we obtain
 $$ \begin{align*}\begin{split}{\mathrm{sep}}(Z_{i},F_{j},\delta/4) & \leq {\mathrm{sep}}(Z_{i-1},F_{j},\delta/4)-{\mathrm{sep}}(Z_{i-1},F_{j}\setminus E t_{i},\delta/2)\\ & \leq {\mathrm{sep}}(Z_{i-1},F_{j},\delta/4)-{\mathrm{sep}}(Z_{i-1},F_{j},\delta/4)\cdot\Sigma_{i}),\end{split} \end{align*} $$
$$ \begin{align*}\begin{split}{\mathrm{sep}}(Z_{i},F_{j},\delta/4) & \leq {\mathrm{sep}}(Z_{i-1},F_{j},\delta/4)-{\mathrm{sep}}(Z_{i-1},F_{j}\setminus E t_{i},\delta/2)\\ & \leq {\mathrm{sep}}(Z_{i-1},F_{j},\delta/4)-{\mathrm{sep}}(Z_{i-1},F_{j},\delta/4)\cdot\Sigma_{i}),\end{split} \end{align*} $$
which verifies (2).
If we show that for every
![]() $i< n$
, we have
$i< n$
, we have
![]() $\Sigma _{i}\leq \Sigma :={\mathrm {sep}}(X,E,\delta /2)$
, then we get from (2) that
$\Sigma _{i}\leq \Sigma :={\mathrm {sep}}(X,E,\delta /2)$
, then we get from (2) that
 $$ \begin{align*}\begin{split}{\mathrm{sep}}(Z,F_{j},\delta/4) & \leq {\mathrm{sep}}(Z_{n}, F_{j},\delta/4) \leq \prod_{i=0}^{n-1}(1-\Sigma_{i}){\mathrm{sep}}(Z_{0},F_{j},\delta/4)\\ & \leq (1-\Sigma)^{|T_{j}|}{\mathrm{sep}}(X,F_{j},\delta/4).\end{split}\end{align*} $$
$$ \begin{align*}\begin{split}{\mathrm{sep}}(Z,F_{j},\delta/4) & \leq {\mathrm{sep}}(Z_{n}, F_{j},\delta/4) \leq \prod_{i=0}^{n-1}(1-\Sigma_{i}){\mathrm{sep}}(Z_{0},F_{j},\delta/4)\\ & \leq (1-\Sigma)^{|T_{j}|}{\mathrm{sep}}(X,F_{j},\delta/4).\end{split}\end{align*} $$
However, it is clear since for every
![]() $i< n$
, if
$i< n$
, if
![]() $M\subseteq Z_{i}$
is a
$M\subseteq Z_{i}$
is a
![]() $\delta /2$
-separated set with respect to
$\delta /2$
-separated set with respect to
![]() $d_{E t_{i+1}}$
, then
$d_{E t_{i+1}}$
, then
![]() $t_{i+1}^{-1} M$
is a
$t_{i+1}^{-1} M$
is a
![]() $\delta /2$
-separated set in X with respect to
$\delta /2$
-separated set in X with respect to
![]() $d_{E}$
.
$d_{E}$
.
Finally, using that for all sufficiently large
![]() $n\in {\mathbb {N}}$
we have by [Reference Ceccherini-Silberstein and Coornaert8, Proposition 5.6.4]
$n\in {\mathbb {N}}$
we have by [Reference Ceccherini-Silberstein and Coornaert8, Proposition 5.6.4]
![]() $|T_{n}|\geq K|F_{n}|$
, for some constant
$|T_{n}|\geq K|F_{n}|$
, for some constant
![]() $K>0$
, we get
$K>0$
, we get
 $$ \begin{align*}\begin{split}\log {\mathrm{sep}}(Z,d_{F_{n}},\delta/4) & \leq \log (1-\Sigma)^{|T_{n}|}{\mathrm{sep}}(X,F_{n},\delta/4)\\ & =|T_{n}|\log (1-\Sigma)+\log {\mathrm{sep}}(X,F_{n},\delta/4)\\ & \leq K|F_{n}|\log(1-\Sigma)+ \log {\mathrm{sep}}(X,F_{n},\delta/4);\end{split} \end{align*} $$
$$ \begin{align*}\begin{split}\log {\mathrm{sep}}(Z,d_{F_{n}},\delta/4) & \leq \log (1-\Sigma)^{|T_{n}|}{\mathrm{sep}}(X,F_{n},\delta/4)\\ & =|T_{n}|\log (1-\Sigma)+\log {\mathrm{sep}}(X,F_{n},\delta/4)\\ & \leq K|F_{n}|\log(1-\Sigma)+ \log {\mathrm{sep}}(X,F_{n},\delta/4);\end{split} \end{align*} $$
therefore, we get
 $$ \begin{align*}\begin{split}h_{\mathrm{top}}(Z,G) & =h_{\mathrm{sep}}(Z, \delta/4,d)=\limsup_{n\to\infty} \frac{1}{|F_{n}|}\log {\mathrm{sep}}(Z,d_{F_{n}},\delta/4)\\ & \leq \limsup_{n\to\infty} \frac{1}{|F_{n}|}(K|F_{n}|\log(1-\Sigma)+ \log {\mathrm{sep}}(X,F_{n},\delta/4))\\ & = \limsup_{n\to\infty} \bigg(K\log(1-\Sigma)+ \frac{1}{|F_{n}|}(\log {\mathrm{sep}}(X,F_{n},\delta/4))\bigg)\\ & = K\log(1-\Sigma)+h_{\mathrm{sep}}(X,\delta/4,d)\\ & < h_{\mathrm{top}}(X,G),\end{split}\end{align*} $$
$$ \begin{align*}\begin{split}h_{\mathrm{top}}(Z,G) & =h_{\mathrm{sep}}(Z, \delta/4,d)=\limsup_{n\to\infty} \frac{1}{|F_{n}|}\log {\mathrm{sep}}(Z,d_{F_{n}},\delta/4)\\ & \leq \limsup_{n\to\infty} \frac{1}{|F_{n}|}(K|F_{n}|\log(1-\Sigma)+ \log {\mathrm{sep}}(X,F_{n},\delta/4))\\ & = \limsup_{n\to\infty} \bigg(K\log(1-\Sigma)+ \frac{1}{|F_{n}|}(\log {\mathrm{sep}}(X,F_{n},\delta/4))\bigg)\\ & = K\log(1-\Sigma)+h_{\mathrm{sep}}(X,\delta/4,d)\\ & < h_{\mathrm{top}}(X,G),\end{split}\end{align*} $$
which finishes the proof of Claim 3.4.
Claim 3.5. We have
![]() $h_{\mathrm {top}}(\tau [X])<h_{\mathrm {top}}(X)$
.
$h_{\mathrm {top}}(\tau [X])<h_{\mathrm {top}}(X)$
.
Since we already proved that
![]() $h_{\mathrm {top}}(Z)<h_{\mathrm {top}}(X)$
and that
$h_{\mathrm {top}}(Z)<h_{\mathrm {top}}(X)$
and that
![]() $\tau [Z]=\tau [X]$
, it is enough to show that
$\tau [Z]=\tau [X]$
, it is enough to show that
![]() $h_{\mathrm {top}}(\tau [Z])\leq h_{\mathrm {top}}(Z)$
. By Proposition 2.7, it is enough to show that
$h_{\mathrm {top}}(\tau [Z])\leq h_{\mathrm {top}}(Z)$
. By Proposition 2.7, it is enough to show that
![]() $h_{\mathrm {sep}}(\tau [Z],\delta ,d)\leq h_{\mathrm {sep}}(Z,\delta ,d)$
. Let
$h_{\mathrm {sep}}(\tau [Z],\delta ,d)\leq h_{\mathrm {sep}}(Z,\delta ,d)$
. Let
![]() $N\subseteq \tau [Z]$
be a
$N\subseteq \tau [Z]$
be a
![]() $\delta $
-separated set with respect to
$\delta $
-separated set with respect to
![]() $d_{F}$
for some
$d_{F}$
for some
![]() $F\subseteq G$
. For each
$F\subseteq G$
. For each
![]() $x\in N$
, let
$x\in N$
, let
![]() $x^{\prime }\in Z$
be any element from
$x^{\prime }\in Z$
be any element from
![]() $\tau ^{-1}(x)$
and let
$\tau ^{-1}(x)$
and let
![]() $N^{\prime }:=\{x^{\prime }\colon x\in N\}\subseteq Z$
. We claim that
$N^{\prime }:=\{x^{\prime }\colon x\in N\}\subseteq Z$
. We claim that
![]() $N^{\prime }$
is
$N^{\prime }$
is
![]() $\delta $
-separated for
$\delta $
-separated for
![]() $d_{S\cdot F}$
. Indeed, this follows since S is a memory set for
$d_{S\cdot F}$
. Indeed, this follows since S is a memory set for
![]() $\tau $
as follows. If
$\tau $
as follows. If
![]() $x\neq y\in N$
, then there is
$x\neq y\in N$
, then there is
![]() $e\in F$
such that
$e\in F$
such that
![]() $d(ex,ey)>\delta $
. If there were not
$d(ex,ey)>\delta $
. If there were not
![]() $s\in S$
such that
$s\in S$
such that
![]() $d((se)x^{\prime },(se)y^{\prime })>\delta $
, then by the definition of a memory set and the fact that S is symmetric, we would have that
$d((se)x^{\prime },(se)y^{\prime })>\delta $
, then by the definition of a memory set and the fact that S is symmetric, we would have that
![]() $d(ex,ey)\leq \delta $
, a contradiction. It follows that
$d(ex,ey)\leq \delta $
, a contradiction. It follows that
 $$ \begin{align*}\begin{split}h_{\mathrm{sep}}(Z,\delta,d) & = \limsup_{n\to\infty} \frac{1}{|S\cdot F_{n}|} \log {\mathrm{sep}}(Z,d_{S\cdot F_{n}},\delta)\\ & \leq \limsup_{n\to\infty} \frac{1}{|F_{n}|} \log {\mathrm{sep}}(Z,d_{S\cdot F_{n}},\delta)\\ & \leq \limsup_{n\to\infty} \frac{1}{|F_{n}|} \log {\mathrm{sep}}(\tau[Z],d_{F_{n}},\delta)= h_{\mathrm{sep}}(\tau[Z],\delta,d),\end{split}\end{align*} $$
$$ \begin{align*}\begin{split}h_{\mathrm{sep}}(Z,\delta,d) & = \limsup_{n\to\infty} \frac{1}{|S\cdot F_{n}|} \log {\mathrm{sep}}(Z,d_{S\cdot F_{n}},\delta)\\ & \leq \limsup_{n\to\infty} \frac{1}{|F_{n}|} \log {\mathrm{sep}}(Z,d_{S\cdot F_{n}},\delta)\\ & \leq \limsup_{n\to\infty} \frac{1}{|F_{n}|} \log {\mathrm{sep}}(\tau[Z],d_{F_{n}},\delta)= h_{\mathrm{sep}}(\tau[Z],\delta,d),\end{split}\end{align*} $$
and we are done.
For the sake of completeness, although this has been already shown by Li in [Reference Li34], let us prove the Myhill property under the weaker assumption that the G-space X satisfies the weak specification property. We again assume that the expansiveness constant is
![]() $\delta>0$
.
$\delta>0$
.
Let
![]() $\tau :X\rightarrow X$
be a continuous G-equivariant map and suppose that it is not surjective. We shall show that it is not pre-injective. Let
$\tau :X\rightarrow X$
be a continuous G-equivariant map and suppose that it is not surjective. We shall show that it is not pre-injective. Let
![]() $S\subseteq G$
be a finite symmetric set containing
$S\subseteq G$
be a finite symmetric set containing
![]() $1_{G}$
such that the following hold:
$1_{G}$
such that the following hold:
-
(1) there is a memory set
 $S^{\prime }\subseteq G$
such that
$S^{\prime }\subseteq G$
such that
 $D_{\delta /4}\cdot S^{\prime }\subseteq S$
;
$D_{\delta /4}\cdot S^{\prime }\subseteq S$
; -
(2) there is a weak specification set
 $S^{\prime \prime }\subseteq G$
for X such that
$S^{\prime \prime }\subseteq G$
for X such that
 $D_{\delta /4}\cdot S^{\prime \prime }\subseteq S$
.
$D_{\delta /4}\cdot S^{\prime \prime }\subseteq S$
.
Set
![]() $Y:=\tau [X]\subseteq X$
. Since
$Y:=\tau [X]\subseteq X$
. Since
![]() $Y\neq X$
, arguing exactly as in the proof of Claim 3.4 and using Proposition 2.7, we get that
$Y\neq X$
, arguing exactly as in the proof of Claim 3.4 and using Proposition 2.7, we get that
![]() $h_{\mathrm {top}}(Y,G)<h_{\mathrm {top}}(X,G)$
and that moreover, for a given Følner sequence
$h_{\mathrm {top}}(Y,G)<h_{\mathrm {top}}(X,G)$
and that moreover, for a given Følner sequence
![]() $(F_{n})_{n}$
(respectively net in case G is uncountable), there exists
$(F_{n})_{n}$
(respectively net in case G is uncountable), there exists
![]() $j\in {\mathbb {N}}$
such that
$j\in {\mathbb {N}}$
such that
Fix
![]() $x\in X$
. Let
$x\in X$
. Let
![]() $X_{0}$
be the set
$X_{0}$
be the set
![]() $\{z\in X\colon d_{G\setminus S\cdot F_{j}}(x,z)\leq \delta \}$
. By Corollary 2.6,
$\{z\in X\colon d_{G\setminus S\cdot F_{j}}(x,z)\leq \delta \}$
. By Corollary 2.6,
![]() $X_{0}\subseteq [x]_{\sim }$
. Let
$X_{0}\subseteq [x]_{\sim }$
. Let
![]() $N\subseteq X$
be a
$N\subseteq X$
be a
![]() $\delta $
-separated set with respect to
$\delta $
-separated set with respect to
![]() $d_{F_{j}}$
of size
$d_{F_{j}}$
of size
![]() ${\mathrm {sep}}(X,F_{j},\delta )$
. Using the weak specification property with respect to
${\mathrm {sep}}(X,F_{j},\delta )$
. Using the weak specification property with respect to
![]() $D_{\delta /4}\cdot S^{\prime \prime }\subseteq S$
, for each
$D_{\delta /4}\cdot S^{\prime \prime }\subseteq S$
, for each
![]() $y\in N$
, we can find
$y\in N$
, we can find
![]() $z_{y}\in X_{0}$
such that
$z_{y}\in X_{0}$
such that
![]() $d_{F_{j}}(y,z_{j})\leq \delta /4$
. In particular, we get
$d_{F_{j}}(y,z_{j})\leq \delta /4$
. In particular, we get
Since S is also a memory set for
![]() $\tau $
, we get that for every
$\tau $
, we get that for every
![]() $z\in X_{0}$
,
$z\in X_{0}$
,
Therefore, as
![]() ${\mathrm {sep}}(X_{0},F_{j},\delta /2)>{\mathrm {sep}}(Y,S^{2}\cdot F_{j},\delta )$
, there must exist
${\mathrm {sep}}(X_{0},F_{j},\delta /2)>{\mathrm {sep}}(Y,S^{2}\cdot F_{j},\delta )$
, there must exist
![]() $y,y^{\prime }\in X_{0}$
such that
$y,y^{\prime }\in X_{0}$
such that
![]() $d_{F_{j}}(y,y^{\prime })>\delta /2$
, in particular,
$d_{F_{j}}(y,y^{\prime })>\delta /2$
, in particular,
![]() $y\neq y^{\prime }$
, yet
$y\neq y^{\prime }$
, yet
![]() $d_{S^{2}\cdot F_{j}}(\tau (y),\tau (y^{\prime }))\leq \delta $
. Combining with (3), we have that for every
$d_{S^{2}\cdot F_{j}}(\tau (y),\tau (y^{\prime }))\leq \delta $
. Combining with (3), we have that for every
![]() $g\in G$
,
$g\in G$
,
thus
![]() $\tau (y)=\tau (y^{\prime })$
by expansiveness. Since
$\tau (y)=\tau (y^{\prime })$
by expansiveness. Since
![]() $y,y^{\prime }\in X_{0}\subseteq [x]_{\sim }$
, we have
$y,y^{\prime }\in X_{0}\subseteq [x]_{\sim }$
, we have
![]() $y\sim y^{\prime }$
and we are done.
$y\sim y^{\prime }$
and we are done.
In the following corollary, we list several classes of dynamical systems to which Theorem 3.2 applies and for which the result is new.
Corollary 3.6. The dynamical systems below satisfy the Moore–Myhill property.
-
(1) Let
 $(X,f)$
be a mixing uniformly hyperbolic dynamical system.
$(X,f)$
be a mixing uniformly hyperbolic dynamical system. -
(2) Let G be a countable amenable group, A a finite set, and
 $X\subseteq A^{G}$
a strongly irreducible subshift with the strong topological Markov property.
$X\subseteq A^{G}$
a strongly irreducible subshift with the strong topological Markov property.
Proof. For (1), notice that such a dynamical system is an expansive action of
![]() ${\mathbb {Z}}$
that has the weak specification property and the pseudo-orbit tracing property, and thus also the strong topological Markov property. For expansiveness, see [Reference Katok and Hasselblatt32, Corollary 6.4.10], for the pseudo-orbit tracing property, see [Reference Katok and Hasselblatt32, Theorem 18.1.2], and for the weak specification property, see [Reference Katok and Hasselblatt32, Theorem 18.3.9]. It follows that Theorem 3.2 applies.
${\mathbb {Z}}$
that has the weak specification property and the pseudo-orbit tracing property, and thus also the strong topological Markov property. For expansiveness, see [Reference Katok and Hasselblatt32, Corollary 6.4.10], for the pseudo-orbit tracing property, see [Reference Katok and Hasselblatt32, Theorem 18.1.2], and for the weak specification property, see [Reference Katok and Hasselblatt32, Theorem 18.3.9]. It follows that Theorem 3.2 applies.
For (2), we can apply Theorem 3.2 since for subshifts, strong irreducibility is equivalent with the weak specification property (see the appendix of [Reference Li34]).
A source of examples of subshifts with the strong topological Markov property is the supports of Markovian measures on subshifts. We refer to [Reference Barbieri, García-Ramos and Li1, §3.3] for the definitions and proof.
Both Moore and Myhill properties make sense to be investigated only for expansive actions of amenable groups. Indeed, it was shown by Bartholdi in [Reference Bartholdi3], respectively in [Reference Bartholdi4], that the Moore, respectively Myhill, property fails already for a full topological shift of any non-amenable group. However, there is a well-known weakening of the Myhill property, called surjunctivity, which asks whether an injective continuous G-equivariant map of some dynamical system is surjective. It is a famous conjecture of Gottschalk from [Reference Gottschalk28] whether for every group G and finite alphabet A, the full shift
![]() $A^{G}$
is surjunctive. This conjecture has received considerable attention especially since Gromov’s introduction of sofic groups and his proof state that such groups do satisfy Gottschalk’s conjecture (see [Reference Gromov29]). A very recent result of Ceccherini-Silberstein, Coornaert, and Li establishes surjunctivity of expansive actions of sofic groups on compact metrizable spaces having the weak specification and strong topological Markov properties, and having non-negative sofic entropy (see [Reference Ceccherini-Silberstein, Coornaert and Li14]).
$A^{G}$
is surjunctive. This conjecture has received considerable attention especially since Gromov’s introduction of sofic groups and his proof state that such groups do satisfy Gottschalk’s conjecture (see [Reference Gromov29]). A very recent result of Ceccherini-Silberstein, Coornaert, and Li establishes surjunctivity of expansive actions of sofic groups on compact metrizable spaces having the weak specification and strong topological Markov properties, and having non-negative sofic entropy (see [Reference Ceccherini-Silberstein, Coornaert and Li14]).
It is only very recent that an analogous weakening has been introduced also for the Moore property. This is the dual surjunctivity of Capobianco, Kari, and Taati from [Reference Capobianco, Kari and Taati6] (see also the recent [Reference Doucha and Gismatullin23] for additional results, including those concerning more general expansive dynamical systems), which has been also proved for full shifts of sofic groups. Applying Theorem 3.2, we can extend the results about dual surjunctive dynamical systems from [Reference Doucha and Gismatullin23]; we also refer there for unexplained notions from the next corollary.
Corollary 3.7. Let G be a countable amenable group and X an expansive and compact G-metric space satisfying the weak specification and the strong topological Markov properties. Then X is dual surjunctive.
Proof. Let
![]() $T:X\rightarrow X$
be a post-surjective continuous G-equivariant map. It is well known that the weak specification property implies that each equivalence class
$T:X\rightarrow X$
be a post-surjective continuous G-equivariant map. It is well known that the weak specification property implies that each equivalence class
![]() $[x]_{\sim }$
, for
$[x]_{\sim }$
, for
![]() $x\in X$
, is dense, so we can apply [Reference Doucha and Gismatullin23, Lemma 5.7] to get that T is surjective. Applying Theorem 3.2 gives us then that T is pre-injective. The proof is finished by the application of [Reference Doucha and Gismatullin23, Theorem 5.6] showing that T is injective.
$x\in X$
, is dense, so we can apply [Reference Doucha and Gismatullin23, Lemma 5.7] to get that T is surjective. Applying Theorem 3.2 gives us then that T is pre-injective. The proof is finished by the application of [Reference Doucha and Gismatullin23, Theorem 5.6] showing that T is injective.
With respect to the recent result [Reference Ceccherini-Silberstein, Coornaert and Li14], it is natural to ask whether actually all expansive actions of sofic groups on compact metrizable spaces satisfying certain additional properties, as in [Reference Ceccherini-Silberstein, Coornaert and Li14], are dual surjunctive.
We finish this subsection with a discussion on endomorphisms of general expansive dynamical systems. Although such maps have appeared in the literature extensively (in addition to the papers on the Moore and Myhill properties and surjunctivity of expansive dynamical systems cited above, see e.g. [Reference Nasu39] and references therein), we are not aware of any detailed research on them comparable to what has been investigated for endomorphisms of shifts, where a thorough investigation was presented already in the influential [Reference Hedlund30].
In the symbolic case, the Curtis–Hedlund–Lyndom theorem characterizes all the endomorphisms of a given shift dynamical system, which both provides some restrictions, e.g. makes the set of all endomorphisms at most countable, and – at least in the full shift case – allows one to generate endomorphisms.
Using the notion of a memory set of an endomorphism of a general expansive dynamical system
![]() $(X,G)$
and the fact that such a set is finite (see Definition 2.8 and Lemma 2.9 respectively), one can show that given a finite set
$(X,G)$
and the fact that such a set is finite (see Definition 2.8 and Lemma 2.9 respectively), one can show that given a finite set
![]() $F\subseteq G$
, there are only finitely many endomorphisms of X whose memory set is F. We leave the verification to the reader. Thus the set of all endomorphisms of X is at most countable – as in the symbolic case.
$F\subseteq G$
, there are only finitely many endomorphisms of X whose memory set is F. We leave the verification to the reader. Thus the set of all endomorphisms of X is at most countable – as in the symbolic case.
Finding how to generate endomorphisms seems to be a much more complicated task and we propose here a way to turn a continuous approximately G-equivariant map
![]() $\tau _{0}:X\rightarrow X$
into a continuous G-equivariant map – in the case when X has the POTP. Here we call a map
$\tau _{0}:X\rightarrow X$
into a continuous G-equivariant map – in the case when X has the POTP. Here we call a map
![]() $\tau _{0}:X\rightarrow X$
approximately G-equivariant or, to be coherent with the term ‘pseudo-orbit’, pseudoequivariant with respect to
$\tau _{0}:X\rightarrow X$
approximately G-equivariant or, to be coherent with the term ‘pseudo-orbit’, pseudoequivariant with respect to
![]() $(S,\varepsilon )$
, where
$(S,\varepsilon )$
, where
![]() $S\subseteq G$
and
$S\subseteq G$
and
![]() $\varepsilon>0$
, if for every
$\varepsilon>0$
, if for every
![]() $x\in X$
and
$x\in X$
and
![]() $s\in S$
, we have
$s\in S$
, we have
![]() $d(\tau _{0}(sx),s\tau _{0}(x))<\varepsilon $
. Now, assuming that the expansiveness constant of X is
$d(\tau _{0}(sx),s\tau _{0}(x))<\varepsilon $
. Now, assuming that the expansiveness constant of X is
![]() $\delta>0$
and
$\delta>0$
and
![]() $S\subseteq G$
is the pseudo-orbit tracing set, any pseudoequivariant map
$S\subseteq G$
is the pseudo-orbit tracing set, any pseudoequivariant map
![]() $\tau _{0}:X\rightarrow X$
, with respect to
$\tau _{0}:X\rightarrow X$
, with respect to
![]() $(S,\delta /3)$
, gives rise to a continuous G-equivariant map
$(S,\delta /3)$
, gives rise to a continuous G-equivariant map
![]() $\tau :X\rightarrow X$
as follows. For any
$\tau :X\rightarrow X$
as follows. For any
![]() $x\in X$
, set
$x\in X$
, set
![]() $\tau (x)$
to be the unique element of X whose orbit traces the pseudo-orbit
$\tau (x)$
to be the unique element of X whose orbit traces the pseudo-orbit
![]() $(\tau _{0}(gx))_{g\in G}$
. We leave the straightforward verifications that the latter is indeed a pseudo-orbit and that
$(\tau _{0}(gx))_{g\in G}$
. We leave the straightforward verifications that the latter is indeed a pseudo-orbit and that
![]() $\tau $
is continuous and G-equivariant again to the reader.
$\tau $
is continuous and G-equivariant again to the reader.
3.2 Beyond countable groups and metrizable spaces
Although Theorem 3.2 was stated for countable groups, one can check that the proof can be easily adapted to handle the uncountable case as well, replacing the limits of sequences by limits of nets in appropriate places. Here we hint how to remove the assumption of metrizability of the compact space X from Theorem 3.2. First, we state the following general definition of expansiveness of an action, not using any metric. It is an exercise that this coincides with Definition 2.1 when X is equipped with a compatible metric.
Definition 3.8. Let G be an arbitrary group acting continuously on a compact topological space X. The action is expansive if there exists a finite open cover
![]() $(U_{i})_{i=1}^{n}$
of X such that the sets
$(U_{i})_{i=1}^{n}$
of X such that the sets
![]() $\{gU_{i}\colon g\in G, i\leq n\}$
form a subbase of the topology of X.
$\{gU_{i}\colon g\in G, i\leq n\}$
form a subbase of the topology of X.
A general definition of expansive actions of groups on uniform spaces was given in [Reference Ceccherini-Silberstein and Coornaert9]. It is again an exercise that it coincides with the definition above.
The following are well-known facts from general topology (we shall refer to the book [Reference Engelking25]).
-
• The topology of X is induced by a unique uniform structure
 ${\mathcal {U}}$
(see e.g. [Reference Engelking25, Theorem 8.3.13]).
${\mathcal {U}}$
(see e.g. [Reference Engelking25, Theorem 8.3.13]). -
• For every
 $U\in {\mathcal {U}}$
, there is a uniformly continuous pseudometric
$U\in {\mathcal {U}}$
, there is a uniformly continuous pseudometric
 $\rho $
on X such that
$\rho $
on X such that
 $\rho (x,y)<1$
for every
$\rho (x,y)<1$
for every
 $(x,y)\in U$
(see e.g. [Reference Engelking25, Corollary 8.1.11]).
$(x,y)\in U$
(see e.g. [Reference Engelking25, Corollary 8.1.11]). -
• For every two uniformly continuous pseudometrics
 $\rho _{1}$
and
$\rho _{1}$
and
 $\rho _{2}$
on X, also
$\rho _{2}$
on X, also
 $\max \{\rho _{1},\rho _{2}\}$
is a uniformly continuous pseudometric (this is obvious).
$\max \{\rho _{1},\rho _{2}\}$
is a uniformly continuous pseudometric (this is obvious).
Since the finite open cover from Definition 3.8 can be replaced by any finite open subcover, it follows from the general facts above that if a group G acts expansively on a compact space X, then there exists a single uniformly continuous pseudometric
![]() $\rho $
on X and a constant
$\rho $
on X and a constant
![]() $\delta>0$
such that for every
$\delta>0$
such that for every
![]() $x\neq y$
, there is
$x\neq y$
, there is
![]() $g\in G$
so that
$g\in G$
so that
![]() $\rho (gx,gy)>\delta $
.
$\rho (gx,gy)>\delta $
.
If
![]() $\tau :X\rightarrow X$
is continuous and G-equivariant, Definition 2.8 and Lemma 2.9 are adapted in a straightforward way to this pseudometric
$\tau :X\rightarrow X$
is continuous and G-equivariant, Definition 2.8 and Lemma 2.9 are adapted in a straightforward way to this pseudometric
![]() $\rho $
. The topological entropy of the action on X can be also computed using this pseudometric
$\rho $
. The topological entropy of the action on X can be also computed using this pseudometric
![]() $\rho $
. This follows from the fact that
$\rho $
. This follows from the fact that
![]() $\rho $
is dynamically generating in the sense of [Reference Kerr and Li33, Definition 9.35] and then applying [Reference Kerr and Li33, Theorem 9.38]. Note that the argument there is for a metrizable compact space; however, it can be also used for non-metrizable spaces by replacing the genuine metric from [Reference Kerr and Li33, Lemma 9.37] by the pseudometric
$\rho $
is dynamically generating in the sense of [Reference Kerr and Li33, Definition 9.35] and then applying [Reference Kerr and Li33, Theorem 9.38]. Note that the argument there is for a metrizable compact space; however, it can be also used for non-metrizable spaces by replacing the genuine metric from [Reference Kerr and Li33, Lemma 9.37] by the pseudometric
![]() $\max \{\rho ,\rho ^{\prime }\}$
, where
$\max \{\rho ,\rho ^{\prime }\}$
, where
![]() $\rho ^{\prime }$
is a uniformly continuous pseudometric generating the open sets of the finite open cover (which varies) from [Reference Kerr and Li33, Lemma 9.37].
$\rho ^{\prime }$
is a uniformly continuous pseudometric generating the open sets of the finite open cover (which varies) from [Reference Kerr and Li33, Lemma 9.37].
The other notions such as the strong topological Markov property, the weak specification property, and the homoclinic relation are defined as in the metrizable case with the exception that their definition starts with the quantifier ‘for every uniformly continuous pseudometric
![]() $\unicode{x3bb} $
’ and the rest of the definition is with respect to
$\unicode{x3bb} $
’ and the rest of the definition is with respect to
![]() $\unicode{x3bb} $
. The set
$\unicode{x3bb} $
. The set
![]() $\Lambda _{\delta }(x,y)$
is defined with respect to the distinguished pseudometric
$\Lambda _{\delta }(x,y)$
is defined with respect to the distinguished pseudometric
![]() $\rho $
and Corollary 2.6 is still valid.
$\rho $
and Corollary 2.6 is still valid.
We can then repeat verbatim the whole proof of Theorem 3.2, using the pseudometric
![]() $\rho $
instead of the metric d.
$\rho $
instead of the metric d.
4 Weakly periodic points
The goal of this section is to prove the second main result, that certain expansive actions of finitely generated groups with at least two ends have weakly periodic points, generalizing the result from [Reference Cohen20].
We shall need another variant of the topological Markov property, different from those introduced in [Reference Barbieri, García-Ramos and Li1], although it may turn out that it is equivalent to one of them. We formulate the following definition only for expansive actions, since our work is limited to them; however, a straightforward modification allows us to formulate it generally.
Definition 4.1. Let a group G act continuously and expansively on a compact metric space X with an expansiveness constant
![]() $\delta>0$
. We say that the action has the cover strong topological Markov property if there is a finite symmetric set
$\delta>0$
. We say that the action has the cover strong topological Markov property if there is a finite symmetric set
![]() $S\subseteq G$
containing the unit such that for every cover
$S\subseteq G$
containing the unit such that for every cover
![]() $(F_{n})_{n\in {\mathbb {N}}}$
of G by disjoint, not necessarily finite, sets and for every sequence of elements
$(F_{n})_{n\in {\mathbb {N}}}$
of G by disjoint, not necessarily finite, sets and for every sequence of elements
![]() $(x_{n})_{n\in {\mathbb {N}}}\subseteq X$
satisfying that for every
$(x_{n})_{n\in {\mathbb {N}}}\subseteq X$
satisfying that for every
![]() $n\neq m\in {\mathbb {N}}$
and every
$n\neq m\in {\mathbb {N}}$
and every
![]() $g\in S\cdot F_{n}\cap F_{m}$
,
$g\in S\cdot F_{n}\cap F_{m}$
,
then there exists
![]() $z\in X$
with the property that for every n and
$z\in X$
with the property that for every n and
![]() $g\in F_{n}$
, we have
$g\in F_{n}$
, we have
The next result says that for expansive actions, this topological Markov property lies, in principle, somewhere in between the pseudo-orbit tracing property and the uniform strong topological Markov property, but we conjecture that it might be actually equivalent to the latter.
Proposition 4.2. Let a group G act continuously and expansively on a compact metric space X with an expansiveness constant
![]() $\delta>0$
. If the action has the pseudo-orbit tracing property, then it has the cover strong topological Markov property, and if it has the latter, then it has the uniform strong topological Markov property.
$\delta>0$
. If the action has the pseudo-orbit tracing property, then it has the cover strong topological Markov property, and if it has the latter, then it has the uniform strong topological Markov property.
Proof. Let us fix the group G, the space X, and the action of G on X. Suppose first it has the pseudo-orbit tracing property and let us show it has the cover strong topological Markov property. Let S be a finite pseudo-orbit tracing set for the action, assuming as usual that it is symmetric and containing the unit. Let
![]() $(F_{n})_{n\in {\mathbb {N}}}$
be a cover of G by disjoint sets and let
$(F_{n})_{n\in {\mathbb {N}}}$
be a cover of G by disjoint sets and let
![]() $(x_{n})_{n\in {\mathbb {N}}}\subseteq X$
be a sequence of elements satisfying that for every
$(x_{n})_{n\in {\mathbb {N}}}\subseteq X$
be a sequence of elements satisfying that for every
![]() $n\neq m\in {\mathbb {N}}$
and every
$n\neq m\in {\mathbb {N}}$
and every
![]() $g\in S\cdot F_{n}\cap F_{m}$
,
$g\in S\cdot F_{n}\cap F_{m}$
,
We produce a G-indexed set
![]() $(y_{g})_{g\in G}$
as follows. For any
$(y_{g})_{g\in G}$
as follows. For any
![]() $g\in G$
, we set
$g\in G$
, we set
Let us verify that
![]() $(y_{g})_{g}$
is a pseudo-orbit for the set S. Pick any
$(y_{g})_{g}$
is a pseudo-orbit for the set S. Pick any
![]() $g\in G$
and
$g\in G$
and
![]() $s\in S$
. We need to check that
$s\in S$
. We need to check that
![]() $d(s y_{g},y_{sg})<\delta /2$
. Let
$d(s y_{g},y_{sg})<\delta /2$
. Let
![]() $n\in {\mathbb {N}}$
be such that
$n\in {\mathbb {N}}$
be such that
![]() $g\in F_{n}$
. If
$g\in F_{n}$
. If
![]() $sg\in F_{n}$
, then
$sg\in F_{n}$
, then
![]() $s y_{g}$
is, by definition, equal to
$s y_{g}$
is, by definition, equal to
![]() $y_{sg}$
, and there is nothing to prove. So we assume that
$y_{sg}$
, and there is nothing to prove. So we assume that
![]() $sg\in F_{m}$
, where
$sg\in F_{m}$
, where
![]() $m\neq n$
, and we have
$m\neq n$
, and we have
![]() $sg\in S\cdot F_{n}\cap F_{m}$
. It follows we have
$sg\in S\cdot F_{n}\cap F_{m}$
. It follows we have
and the verification is finished. Therefore, there exists
![]() $z\in X$
shadowing the pseudo-orbit, that is, for every
$z\in X$
shadowing the pseudo-orbit, that is, for every
![]() $g\in G$
, we have
$g\in G$
, we have
![]() $d(gz, y_{g})<\delta /2$
, consequently for for every n and
$d(gz, y_{g})<\delta /2$
, consequently for for every n and
![]() $g\in F_{n}$
, we have
$g\in F_{n}$
, we have
and we are done.
Now assume that the action has the cover strong topological Markov property and we show that it has the uniform strong topological Markov property. Let S be the finite symmetric set from the definition and we show it is also a strong topological Markov set. Let
![]() $A\subseteq G$
be a finite set and let
$A\subseteq G$
be a finite set and let
![]() $V\subseteq G$
be such that for every
$V\subseteq G$
be such that for every
![]() $v\neq w\in V$
, we have
$v\neq w\in V$
, we have
![]() $S\cdot A\cdot v\cap S\cdot A\cdot w-\emptyset $
. Let
$S\cdot A\cdot v\cap S\cdot A\cdot w-\emptyset $
. Let
![]() $(x_{v})_{v\in V}\subseteq X$
and
$(x_{v})_{v\in V}\subseteq X$
and
![]() $y\in X$
be such that
$y\in X$
be such that
![]() $d_{(SA\setminus A)v} (x_{v},y)<\delta /2$
, for every
$d_{(SA\setminus A)v} (x_{v},y)<\delta /2$
, for every
![]() $v\in V$
. Then
$v\in V$
. Then
![]() ${\mathcal {U}}:=(A\cdot v)_{v\in V}\cup \{G\setminus A\cdot V\}$
is a cover of G by disjoint sets. Pick
${\mathcal {U}}:=(A\cdot v)_{v\in V}\cup \{G\setminus A\cdot V\}$
is a cover of G by disjoint sets. Pick
![]() $B\neq C\in {\mathcal {U}}$
and assume that there is
$B\neq C\in {\mathcal {U}}$
and assume that there is
![]() $g\in B$
and
$g\in B$
and
![]() $s\in S$
so that
$s\in S$
so that
![]() $sg\in S\cdot B\cap C$
. By the assumption, necessarily
$sg\in S\cdot B\cap C$
. By the assumption, necessarily
![]() $C=G\setminus A\cdot V$
, and again by the assumption, we get that
$C=G\setminus A\cdot V$
, and again by the assumption, we get that
![]() $d(sg x_{v}, sg y)<\delta /2$
, where
$d(sg x_{v}, sg y)<\delta /2$
, where
![]() $v\in G$
is such that
$v\in G$
is such that
![]() $B=A\cdot v$
. By the cover strong topological Markov property, there is
$B=A\cdot v$
. By the cover strong topological Markov property, there is
![]() $z\in X$
so that the following hold:
$z\in X$
so that the following hold:
-
• for every
 $v\in V$
and every
$v\in V$
and every
 $g\in A\cdot v$
, we have
$g\in A\cdot v$
, we have
 $d(gz, g x_{v})<\delta /2$
;
$d(gz, g x_{v})<\delta /2$
; -
• for every
 $g\in G\setminus A\cdot V$
, we have
$g\in G\setminus A\cdot V$
, we have
 $d(gz,gy)<\delta /2$
,
$d(gz,gy)<\delta /2$
,
which is exactly what we were supposed to show.
We are ready to state the main result of this section. We shall not define here the ends of groups since we will not directly need them. The reader can find it either in Cohen’s article [Reference Cohen20], or we refer to [Reference Druţu and Kapovich24, §9.1.3] for a general treatment and more information.
Theorem 4.3. Let G be a finitely generated group with at least two ends and let G act expansively on a compact metrizable space X so that the action satisfies the cover strong topological Markov property. Then X has a weakly periodic point.
Moreover, if the action satisfies the weak specification property, then the set of such points is dense in X.
The following lemma extracts the main geometric group theoretic ingredient from [Reference Cohen20] that is sufficient for our generalization.
Lemma 4.4. (Extracted from the proof of [Reference Cohen20, Theorem 2.1])
Let
![]() $S\subseteq G$
be a finite symmetric set containing the unit. There exists an infinite order
$S\subseteq G$
be a finite symmetric set containing the unit. There exists an infinite order
![]() $g\in G$
such that for any
$g\in G$
such that for any
![]() $m\in {\mathbb {N}}$
, there is a subset
$m\in {\mathbb {N}}$
, there is a subset
![]() $A_{m}\subseteq G$
satisfying the following properties.
$A_{m}\subseteq G$
satisfying the following properties.
-
• The sets
 $\{A_{m}\cdot g^{nm}\}_{n\in {\mathbb {Z}}}$
form a disjoint partition of G.
$\{A_{m}\cdot g^{nm}\}_{n\in {\mathbb {Z}}}$
form a disjoint partition of G. -
• We have
 $S\cdot A_{m}\subseteq A_{m}\cup S^{2}\cup S^{2}\cdot g^{m}$
.
$S\cdot A_{m}\subseteq A_{m}\cup S^{2}\cup S^{2}\cdot g^{m}$
. -
• If for some
 $k\neq l\in {\mathbb {Z}}$
we have
$k\neq l\in {\mathbb {Z}}$
we have
 $S\cdot A_{m}\cdot g^{km}\cap A_{m}\cdot g^{lm}\neq \emptyset $
, then
$S\cdot A_{m}\cdot g^{km}\cap A_{m}\cdot g^{lm}\neq \emptyset $
, then
 $|k-l|=1$
and for any
$|k-l|=1$
and for any
 $h\in S\cdot A_{m}\cdot g^{km}\cap A_{m}\cdot g^{lm}$
, we have either or
$h\in S\cdot A_{m}\cdot g^{km}\cap A_{m}\cdot g^{lm}$
, we have either or $$ \begin{align*}h\in S^{2}\cdot g^{km}\quad\text{and}\quad hg^{m}\in A_{m}\cdot g^{km}\quad (\text{when }k=l+1),\end{align*} $$
$$ \begin{align*}h\in S^{2}\cdot g^{km}\quad\text{and}\quad hg^{m}\in A_{m}\cdot g^{km}\quad (\text{when }k=l+1),\end{align*} $$
 $$ \begin{align*}h\in S^{2}\cdot g^{(k+1)m}\quad\text{and}\quad hg^{-m}\in A_{m}\cdot g^{km}\quad (\text{when }k=l-1).\end{align*} $$
$$ \begin{align*}h\in S^{2}\cdot g^{(k+1)m}\quad\text{and}\quad hg^{-m}\in A_{m}\cdot g^{km}\quad (\text{when }k=l-1).\end{align*} $$
Proof of Lemma 4.4
Let
![]() $S\subseteq G$
be the given finite set as in the statement of the lemma. Fix a word metric
$S\subseteq G$
be the given finite set as in the statement of the lemma. Fix a word metric
![]() $\rho $
on G with respect to some finite symmetric generating set of G and let
$\rho $
on G with respect to some finite symmetric generating set of G and let
![]() $n\in {\mathbb {N}}$
be big enough so that
$n\in {\mathbb {N}}$
be big enough so that
![]() $n\geq N_{G}$
, where
$n\geq N_{G}$
, where
![]() $N_{G}$
is a constant depending on G from [Reference Cohen20, Lemma 2.5], and
$N_{G}$
is a constant depending on G from [Reference Cohen20, Lemma 2.5], and
![]() $S\subseteq B_{G}(n)$
, where
$S\subseteq B_{G}(n)$
, where
![]() $B_{G}(n)$
is a ball around the unit of G with respect to
$B_{G}(n)$
is a ball around the unit of G with respect to
![]() $\rho $
. In fact, we may without loss of generality assume that
$\rho $
. In fact, we may without loss of generality assume that
![]() $S=B_{G}(n)$
.
$S=B_{G}(n)$
.
Let
![]() $g^{\prime }\in G$
be the element obtained by [Reference Cohen20, Lemma 2.5] as n-axial (we refer for the terminology to [Reference Cohen20], but here we do not need to know its precise meaning). We have that
$g^{\prime }\in G$
be the element obtained by [Reference Cohen20, Lemma 2.5] as n-axial (we refer for the terminology to [Reference Cohen20], but here we do not need to know its precise meaning). We have that
![]() $g^{\prime }$
has infinite order (see the paragraph after the proof of [Reference Cohen20, Lemma 2.5]) and we set g to be some power of
$g^{\prime }$
has infinite order (see the paragraph after the proof of [Reference Cohen20, Lemma 2.5]) and we set g to be some power of
![]() $g^{\prime }$
so that
$g^{\prime }$
so that
![]() $S^{2}\cdot g^{k}\cap S^{2}=\emptyset $
, exactly as in [Reference Cohen20, p. 609].
$S^{2}\cdot g^{k}\cap S^{2}=\emptyset $
, exactly as in [Reference Cohen20, p. 609].
In the rest of the proof, we show that
![]() $g\in G$
is as desired (we already know it has infinite order) and we fix additionally some
$g\in G$
is as desired (we already know it has infinite order) and we fix additionally some
![]() $m\in {\mathbb {N}}$
. Now we let
$m\in {\mathbb {N}}$
. Now we let
![]() $A_{m}$
be equal to the set
$A_{m}$
be equal to the set
![]() $\mathcal {S}$
from [Reference Cohen20, Definition 2.6] using the same integer m. The first two items of Lemma 4.4 then follow from [Reference Cohen20, Lemma 2.7] (notice that the order of multiplication in [Reference Cohen20] is reversed).
$\mathcal {S}$
from [Reference Cohen20, Definition 2.6] using the same integer m. The first two items of Lemma 4.4 then follow from [Reference Cohen20, Lemma 2.7] (notice that the order of multiplication in [Reference Cohen20] is reversed).
Finally, we show the last item. So pick some
![]() $k\neq l\in {\mathbb {N}}$
such that
$k\neq l\in {\mathbb {N}}$
such that
![]() $S\cdot A_{m}\cdot g^{km}\cap A_{m}\cdot g^{lm}\neq \emptyset $
. Translating these sets from the right by
$S\cdot A_{m}\cdot g^{km}\cap A_{m}\cdot g^{lm}\neq \emptyset $
. Translating these sets from the right by
![]() $g^{-km}$
, we can assume that
$g^{-km}$
, we can assume that
![]() $k=0$
. So suppose that there is
$k=0$
. So suppose that there is
![]() $h\in S\cdot A_{m}\cap A_{m}\cdot g^{lm}$
. We have already shown that we also have that
$h\in S\cdot A_{m}\cap A_{m}\cdot g^{lm}$
. We have already shown that we also have that
![]() $h\in A_{m}\cup S^{2}\cup S^{2}\cdot g^{m}$
. Since
$h\in A_{m}\cup S^{2}\cup S^{2}\cdot g^{m}$
. Since
![]() $A_{m}$
and
$A_{m}$
and
![]() $A_{m}\cdot g^{lm}$
are disjoint, we must have that
$A_{m}\cdot g^{lm}$
are disjoint, we must have that
![]() $h\in S^{2}\cup S^{2}\cdot g^{m}$
.
$h\in S^{2}\cup S^{2}\cdot g^{m}$
.
-
(1) If we have that
 $h\in S^{2}$
, then we are in the situation as in the last item on [Reference Cohen20, pp. 610] (notice the typo there: our case here corresponds to the case
$h\in S^{2}$
, then we are in the situation as in the last item on [Reference Cohen20, pp. 610] (notice the typo there: our case here corresponds to the case
 $x\in B^{2}\setminus \mathcal {S}$
, although there is mistakenly stated
$x\in B^{2}\setminus \mathcal {S}$
, although there is mistakenly stated
 $x\in g^{m} B^{2}\setminus \mathcal {S}$
) and by the argument there, we have
$x\in g^{m} B^{2}\setminus \mathcal {S}$
) and by the argument there, we have
 $hg^{m}\in A_{m}$
, and so
$hg^{m}\in A_{m}$
, and so
 $h\in A_{m}\cdot g^{-m}$
and
$h\in A_{m}\cdot g^{-m}$
and
 $l=-1$
.
$l=-1$
. -
(2) If we have that
 $h\in S^{2}\cdot g^{m}$
, then like in the first item on [Reference Cohen20, p. 611] (the case there when
$h\in S^{2}\cdot g^{m}$
, then like in the first item on [Reference Cohen20, p. 611] (the case there when
 $x\in g^{m} B^{2}\setminus \mathcal {S}$
), we get that
$x\in g^{m} B^{2}\setminus \mathcal {S}$
), we get that
 $hg^{-m}\in A_{m}$
, so
$hg^{-m}\in A_{m}$
, so
 $h\in A_{m}\cdot g^{m}$
and
$h\in A_{m}\cdot g^{m}$
and
 $l=1$
.
$l=1$
.
Proof of Theorem 4.3
Let
![]() $S\subseteq G$
be a finite symmetric set containing the unit that is a cover strong topological Markov set for the action.
$S\subseteq G$
be a finite symmetric set containing the unit that is a cover strong topological Markov set for the action.
Fix now some compatible metric d on X and suppose the expansiveness constant, with respect to d, is some
![]() $\delta>0$
. Fix also a finite
$\delta>0$
. Fix also a finite
![]() $\delta /4$
-dense net
$\delta /4$
-dense net
![]() $N\subseteq X$
. That is, for every
$N\subseteq X$
. That is, for every
![]() $x\in X$
, there is
$x\in X$
, there is
![]() $y\in N$
such that
$y\in N$
such that
![]() $d(x,y)<\delta /4$
. Let
$d(x,y)<\delta /4$
. Let
![]() ${\mathcal {N}}$
denote the set of non-empty subsets of N. Let
${\mathcal {N}}$
denote the set of non-empty subsets of N. Let
![]() $\eta :X\rightarrow {\mathcal {N}}$
be the function associating to every
$\eta :X\rightarrow {\mathcal {N}}$
be the function associating to every
![]() $x\in X$
the set of those elements of N that realize the distance of x from N; that is, for every
$x\in X$
the set of those elements of N that realize the distance of x from N; that is, for every
![]() $x\in X$
and
$x\in X$
and
![]() $y\in \eta (x)$
,
$y\in \eta (x)$
,
Finally, let
![]() $\xi :x\rightarrow {\mathcal {N}}^{S^{2}}$
be the function defined by, for
$\xi :x\rightarrow {\mathcal {N}}^{S^{2}}$
be the function defined by, for
![]() $x\in X$
and
$x\in X$
and
![]() $s\in S^{2}$
,
$s\in S^{2}$
,
Pick now some arbitrary
![]() $x\in X$
. Since
$x\in X$
. Since
![]() ${\mathcal {N}}^{S^{2}}$
is a finite set, there must exist
${\mathcal {N}}^{S^{2}}$
is a finite set, there must exist
![]() $m,m^{\prime }\in {\mathbb {N}}$
so that
$m,m^{\prime }\in {\mathbb {N}}$
so that
![]() $\xi (g^{m} x)=\xi (g^{m^{\prime }}x)$
. By replacing x by
$\xi (g^{m} x)=\xi (g^{m^{\prime }}x)$
. By replacing x by
![]() $g^{-m}x$
if necessary, we may without loss of generality assume that in fact there is
$g^{-m}x$
if necessary, we may without loss of generality assume that in fact there is
![]() $m\in {\mathbb {N}}$
so that
$m\in {\mathbb {N}}$
so that
By the definition of the function
![]() $\xi $
and by the triangle inequality, we obtain
$\xi $
and by the triangle inequality, we obtain
For every
![]() $n\in {\mathbb {Z}}$
, set
$n\in {\mathbb {Z}}$
, set
![]() $x_{n}:=g^{-nm}x$
. We wish to apply the cover strong topological Markov property with respect to the partition
$x_{n}:=g^{-nm}x$
. We wish to apply the cover strong topological Markov property with respect to the partition
![]() $\{A_{m}\cdot g^{nm}\colon n\in {\mathbb {N}}\}$
and the set of elements
$\{A_{m}\cdot g^{nm}\colon n\in {\mathbb {N}}\}$
and the set of elements
![]() $(x_{n})_{n\in {\mathbb {Z}}}$
to obtain an element
$(x_{n})_{n\in {\mathbb {Z}}}$
to obtain an element
![]() $z\in X$
satisfying
$z\in X$
satisfying
To show that, we need to check that for every
![]() $k\neq l\in {\mathbb {Z}}$
and
$k\neq l\in {\mathbb {Z}}$
and
![]() $s\in S$
, if
$s\in S$
, if
![]() $s\cdot A_{m}\cdot g^{km}\cap A_{m}\cdot g^{lm}\neq \emptyset $
, that is, there are
$s\cdot A_{m}\cdot g^{km}\cap A_{m}\cdot g^{lm}\neq \emptyset $
, that is, there are
![]() $a_{1},a_{2}\in A_{m}$
such that
$a_{1},a_{2}\in A_{m}$
such that
![]() $sa:{1}g^{km}=a_{2}g^{lm}$
, then
$sa:{1}g^{km}=a_{2}g^{lm}$
, then
By Lemma 4.4, we have that
![]() $|k-l|=1$
and one of the following:
$|k-l|=1$
and one of the following:
-
(1) either
 $k=l+1$
and
$k=l+1$
and
 $sa:{1}g^{km}\in S^{2}\cdot g^{km}$
and
$sa:{1}g^{km}\in S^{2}\cdot g^{km}$
and
 $sa:{1}g^{(k+1)m}\in A_{m}\cdot g^{km}$
;
$sa:{1}g^{(k+1)m}\in A_{m}\cdot g^{km}$
; -
(2) or
 $k=l-1$
and
$k=l-1$
and
 $sa:{1}g^{km}\in S^{2}\cdot g^{(k+1)m}$
and
$sa:{1}g^{km}\in S^{2}\cdot g^{(k+1)m}$
and
 $sa:{1}g^{(k-1)m}\in A_{m}\cdot g^{km}$
.
$sa:{1}g^{(k-1)m}\in A_{m}\cdot g^{km}$
.
In the former case, since
![]() $d_{S^{2}}(x,g^{m} x)<\delta /2$
and
$d_{S^{2}}(x,g^{m} x)<\delta /2$
and
![]() $sa:{1}\in S^{2}$
, we have
$sa:{1}\in S^{2}$
, we have
 $$ \begin{align*}\begin{split}d((sa:{1}g^{km})x_{k},(a_{2}g^{lm})x_{l}) &=d((sa:{1}g^{km}g^{-km})x,(sa:{1}g^{km}g^{-lm})x)\\ &=d((sa:{1})x,(sa:{1}g^{m})x)<\delta/2.\end{split}\end{align*} $$
$$ \begin{align*}\begin{split}d((sa:{1}g^{km})x_{k},(a_{2}g^{lm})x_{l}) &=d((sa:{1}g^{km}g^{-km})x,(sa:{1}g^{km}g^{-lm})x)\\ &=d((sa:{1})x,(sa:{1}g^{m})x)<\delta/2.\end{split}\end{align*} $$
In the latter case, we have analogously, since
![]() $d_{S^{2}}(x,g^{m} x)<\delta /2$
and
$d_{S^{2}}(x,g^{m} x)<\delta /2$
and
![]() $sa:{1}g^{-m}\in S^{2}$
,
$sa:{1}g^{-m}\in S^{2}$
,
 $$ \begin{align*}\begin{split}d((sa:{1}g^{km})x_{k},(a_{2}g^{lm})x_{l})&=d((sa:{1}g^{km}g^{-km})x,(sa:{1}g^{km}g^{-lm})x)\\ &=d((sa:{1})x,(sa:{1}g^{-m})x)\\ &=d((sa:{1}g^{-m}g^{m})x,(sa:{1}g^{-m})x)<\delta/2.\end{split}\end{align*} $$
$$ \begin{align*}\begin{split}d((sa:{1}g^{km})x_{k},(a_{2}g^{lm})x_{l})&=d((sa:{1}g^{km}g^{-km})x,(sa:{1}g^{km}g^{-lm})x)\\ &=d((sa:{1})x,(sa:{1}g^{-m})x)\\ &=d((sa:{1}g^{-m}g^{m})x,(sa:{1}g^{-m})x)<\delta/2.\end{split}\end{align*} $$
It follows that there indeed is
![]() $z\in X$
satisfying, as desired,
$z\in X$
satisfying, as desired,
We show that
![]() $g^{m} z=z$
. If not, there is, by expansiveness, some
$g^{m} z=z$
. If not, there is, by expansiveness, some
![]() $h\in G$
such that
$h\in G$
such that
![]() $d(hz,(hg^{m})z)>\delta $
. Since the sets
$d(hz,(hg^{m})z)>\delta $
. Since the sets
![]() $(A_{m}\cdot g^{km})_{k\in {\mathbb {Z}}}$
form a cover of G, there is
$(A_{m}\cdot g^{km})_{k\in {\mathbb {Z}}}$
form a cover of G, there is
![]() $k\in {\mathbb {Z}}$
and
$k\in {\mathbb {Z}}$
and
![]() $a\in A_{m}$
such that
$a\in A_{m}$
such that
![]() $h=ag^{km}$
. It follows that
$h=ag^{km}$
. It follows that
 $$ \begin{align*}\begin{split}d(hz,(hg^{m})z)&\leq d(hz,h x_{k})+d(h x_{k},(hg^{m})x_{k+1})+d((hg^{m})x_{k+1},(hg^{m})z)\\ &= d(hz,h x_{k})+d(a x,a x)+d((hg^{m})x_{k+1},(hg^{m})z)\\ & \leq \delta/2+\delta/2=\delta,\end{split}\end{align*} $$
$$ \begin{align*}\begin{split}d(hz,(hg^{m})z)&\leq d(hz,h x_{k})+d(h x_{k},(hg^{m})x_{k+1})+d((hg^{m})x_{k+1},(hg^{m})z)\\ &= d(hz,h x_{k})+d(a x,a x)+d((hg^{m})x_{k+1},(hg^{m})z)\\ & \leq \delta/2+\delta/2=\delta,\end{split}\end{align*} $$
a contradiction.
We now show the ‘moreover’ part of the statement of the theorem. We retain the notation established during the proof.
Let
![]() $W\subseteq G$
be a finite symmetric set containing the unit that is a weak specification set for the action. To prove the density, pick some
$W\subseteq G$
be a finite symmetric set containing the unit that is a weak specification set for the action. To prove the density, pick some
![]() $w\in X$
and
$w\in X$
and
![]() $\delta /2>\varepsilon >0$
and we shall find a weakly periodic point
$\delta /2>\varepsilon >0$
and we shall find a weakly periodic point
![]() $z_{w}\in X$
such that
$z_{w}\in X$
such that
![]() $d(z_{w},w)<\varepsilon $
. By Lemma 2.2, there is a finite set
$d(z_{w},w)<\varepsilon $
. By Lemma 2.2, there is a finite set
![]() $D_{\varepsilon }\subseteq G$
such that for every
$D_{\varepsilon }\subseteq G$
such that for every
![]() $z^{\prime }\in X$
, if
$z^{\prime }\in X$
, if
![]() $d_{D_{\varepsilon }}(z^{\prime },w)\leq \delta $
, then
$d_{D_{\varepsilon }}(z^{\prime },w)\leq \delta $
, then
![]() $d(z^{\prime },w)<\varepsilon $
. Using the weak specification property, we can find
$d(z^{\prime },w)<\varepsilon $
. Using the weak specification property, we can find
![]() $z^{\prime }_{w}\in X$
such that the following hold:
$z^{\prime }_{w}\in X$
such that the following hold:
-
•
 $d_{D^{2}_{\varepsilon }}(z^{\prime }_{w},w)\leq \delta /2$
(thus
$d_{D^{2}_{\varepsilon }}(z^{\prime }_{w},w)\leq \delta /2$
(thus
 $d_{D_{\varepsilon }}(z^{\prime }_{w},w)<\varepsilon $
);
$d_{D_{\varepsilon }}(z^{\prime }_{w},w)<\varepsilon $
); -
• and
 $d_{G\setminus (D_{\delta /4}\cdot W\cdot D^{2}_{\varepsilon })}(z^{\prime }_{w},z)\leq \delta /4$
.
$d_{G\setminus (D_{\delta /4}\cdot W\cdot D^{2}_{\varepsilon })}(z^{\prime }_{w},z)\leq \delta /4$
.
Let
![]() $l\in {\mathbb {N}}$
be such that
$l\in {\mathbb {N}}$
be such that
![]() $D_{\delta /4}\cdot W\cdot D^{2}_{\varepsilon }\subseteq \bigcup _{i\in [-l,l]} A_{m}\cdot g^{im}$
. We now set
$D_{\delta /4}\cdot W\cdot D^{2}_{\varepsilon }\subseteq \bigcup _{i\in [-l,l]} A_{m}\cdot g^{im}$
. We now set
![]() $\bar A:=\bigcup _{i\in [-l,l]} A_{m}\cdot g^{im}$
. We consider the cover of G by the disjoint sets
$\bar A:=\bigcup _{i\in [-l,l]} A_{m}\cdot g^{im}$
. We consider the cover of G by the disjoint sets
![]() $\{\bar A\cdot g^{lmn}\colon n\in {\mathbb {Z}}\}$
and we define the elements
$\{\bar A\cdot g^{lmn}\colon n\in {\mathbb {Z}}\}$
and we define the elements
![]() $(w_{n})_{n\in {\mathbb {Z}}}$
by setting
$(w_{n})_{n\in {\mathbb {Z}}}$
by setting
![]() $w_{n}:=g^{-lmn}z^{\prime }_{w}$
. We wish to apply the cover strong topological Markov property with this cover of G and this set of elements of X. We need to check that for any
$w_{n}:=g^{-lmn}z^{\prime }_{w}$
. We wish to apply the cover strong topological Markov property with this cover of G and this set of elements of X. We need to check that for any
![]() $n,n^{\prime }\in {\mathbb {Z}}$
and any
$n,n^{\prime }\in {\mathbb {Z}}$
and any
![]() $h\in S\cdot \bar A\cdot g^{lmn}\cap \bar A\cdot g^{lmn^{\prime }}$
, we have
$h\in S\cdot \bar A\cdot g^{lmn}\cap \bar A\cdot g^{lmn^{\prime }}$
, we have
![]() $d(hw_{n},hw_{n^{\prime }})\leq \delta /2$
. We may assume that
$d(hw_{n},hw_{n^{\prime }})\leq \delta /2$
. We may assume that
![]() $h\notin \bar A\cdot g^{lmn}$
, in particular,
$h\notin \bar A\cdot g^{lmn}$
, in particular,
![]() $hg^{-lmn}\notin D_{\delta /4}\cdot W\cdot D^{2}_{\varepsilon }$
, and that
$hg^{-lmn}\notin D_{\delta /4}\cdot W\cdot D^{2}_{\varepsilon }$
, and that
![]() $h=sag^{lmn}$
for some
$h=sag^{lmn}$
for some
![]() $s\in S$
and
$s\in S$
and
![]() $a\in \bar A$
. Then we have
$a\in \bar A$
. Then we have
 $$ \begin{align*}\begin{split}d(hw_{n},hw_{n^{\prime}}) & \leq d((sa) z^{\prime}_{w},(sa)z)\\ &+d((sa)z,(sag^{(n-n^{\prime})lm})z)+d((sag^{(n-n^{\prime})lm})z, (sag^{(n-n^{\prime})lm})z^{\prime}_{w})\\ & \leq \delta/4+0+\delta/4=\delta/2,\end{split}\end{align*} $$
$$ \begin{align*}\begin{split}d(hw_{n},hw_{n^{\prime}}) & \leq d((sa) z^{\prime}_{w},(sa)z)\\ &+d((sa)z,(sag^{(n-n^{\prime})lm})z)+d((sag^{(n-n^{\prime})lm})z, (sag^{(n-n^{\prime})lm})z^{\prime}_{w})\\ & \leq \delta/4+0+\delta/4=\delta/2,\end{split}\end{align*} $$
which verifies the claim. So there exists
![]() $z_{w}\in X$
such that for every n and
$z_{w}\in X$
such that for every n and
![]() $h\in \bar A\cdot g^{lmn}$
, we have
$h\in \bar A\cdot g^{lmn}$
, we have
![]() $d(h z_{w}, (hg^{-lmn})z^{\prime }_{w})\leq \delta /2$
.
$d(h z_{w}, (hg^{-lmn})z^{\prime }_{w})\leq \delta /2$
.
Then we have
thus
![]() $d(z_{w},w)<\varepsilon $
.
$d(z_{w},w)<\varepsilon $
.
Finally, we claim that
![]() $g^{lm}z_{w}=z_{w}$
. However, the proof is completely analogous to the proof that
$g^{lm}z_{w}=z_{w}$
. However, the proof is completely analogous to the proof that
![]() $g^{m} z=z$
and is left to the reader.
$g^{m} z=z$
and is left to the reader.
Cohen mentions in [Reference Cohen20] that he originally conjectured that given a finitely generated group G, there exists a subshift of finite type of G that is strongly aperiodic if and only if G is one-ended. This was refuted by Jeandel in [Reference Jeandel31] who showed that groups with an undecidable word problem do not admit such subshifts of finite type.
While Cohen’s geometric argument can be generalized to more general dynamical systems, as demonstrated above, this is less clear with Jeandel’s computability argument, which seems to be more closely tight to subshifts. It is therefore plausible, although it would be surprising, that the original Cohen’s conjecture is correct within the larger class of expansive dynamical systems satisfying certain topological Markov properties.
Acknowledgements
We would like to thank to Tullio Ceccherini-Silberstein for his comments and for introducing us to topological Markov properties, and to the referee for their comments and suggestions that helped to improve the text. The author was supported by the GAČR project 19-05271Y and RVO: 67985840.





