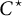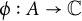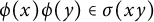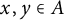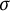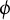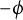1 Introduction
Over the past two decades, there has been considerable interest in the area of nonlinear preserver problems (see a detailed introduction in [Reference Bourhim and Mabrouk1] and the references therein). Loosely speaking, these problems deal with characterizing maps between algebras that preserve a certain (given) algebraic aspect of the spectrum, but without assuming any algebraic conditions, like linearity or multiplicativity, of the map itself. One of the earliest results in this direction is a theorem of Kowalski and Słodkowski [Reference Kowalski and Słodkowski7]; they discovered a nonlinear version of the renowned Gleason–Kahane–Żelazko theorem [Reference Gleason3, Reference Kahane and Żelazko6, Reference Żelazko17], which states that every unital, invertibility-preserving, linear functional on a Banach algebra is necessarily multiplicative, and hence a character of A. The Gleason–Kahane–Żelazko theorem continues to attract attention, and some recent papers of Mashreghi, Ransford, and Ransford include [Reference Mashreghi, Ransford and Ransford9, Reference Mashreghi and Ransford10]. The main result of the current paper simultaneously improves the main result of [Reference Touré, Schulz and Brits16] and the main result of [Reference Brits, Mabrouk and Touré2], thereby settling the problem for general
![]() $C^\star $
-algebras. We start with the statement of the Kowalski–Słodkowski theorem.
$C^\star $
-algebras. We start with the statement of the Kowalski–Słodkowski theorem.
Theorem 1.1 (Kowalski–Słodkowski [Reference Kowalski and Słodkowski7])
Let B be a complex Banach algebra. Then a function
![]() $\phi :B\to \mathbb C$
is linear and multiplicative if and only if
$\phi :B\to \mathbb C$
is linear and multiplicative if and only if
![]() $\phi $
satisfies:
$\phi $
satisfies:
-
(i)
 $\phi (\mathbf 0)=0$
,
$\phi (\mathbf 0)=0$
, -
(ii)
 $\phi (x)-\phi (y)\in \sigma (x-y)$
for every
$\phi (x)-\phi (y)\in \sigma (x-y)$
for every
 $x,y\in B$
.
$x,y\in B$
.
One may observe that the hypothesis of Theorem 1.1 can be stated more economically by requiring only the condition
![]() $\phi (x)+\phi (y)\in \sigma (x+y)$
for every pair
$\phi (x)+\phi (y)\in \sigma (x+y)$
for every pair
![]() $x,y\in B$
. Furthermore, as a consequence of the Kowalski–Słodkowski theorem, one can prove, in a few lines, the following generalization due to Hatori et al.
$x,y\in B$
. Furthermore, as a consequence of the Kowalski–Słodkowski theorem, one can prove, in a few lines, the following generalization due to Hatori et al.
Theorem 1.2 [Reference Hatori, Miura and Takagi5, Theorem 3.1]
Let B be a unital Banach algebra, and let
![]() $B'$
be a unital, semisimple, commutative Banach algebra. Suppose that T is a map from B into
$B'$
be a unital, semisimple, commutative Banach algebra. Suppose that T is a map from B into
![]() $B'$
such that
$B'$
such that
![]() $\sigma (T(a)+T(b))\subseteq \sigma (a+b)$
holds for every pair a and b in B. Then T is linear and multiplicative.
$\sigma (T(a)+T(b))\subseteq \sigma (a+b)$
holds for every pair a and b in B. Then T is linear and multiplicative.
Very close to the current paper are the results that appear in [Reference Hatori, Miura and Takagi4, Reference Hatori, Miura and Takagi5, Reference Molnár11–Reference Rao and Roy13]: In [Reference Molnár11], Molnár studied multiplicatively spectrum-preserving maps
![]() $T:B(H)\to B(H)$
where H is an infinite-dimensional Hilbert space, as well as for maps
$T:B(H)\to B(H)$
where H is an infinite-dimensional Hilbert space, as well as for maps
![]() $T:C(X)\to C(X)$
where X is a first countable compact Hausdorff space. Specifically, he proved that surjective maps T on the aforementioned Banach algebras B satisfying
$T:C(X)\to C(X)$
where X is a first countable compact Hausdorff space. Specifically, he proved that surjective maps T on the aforementioned Banach algebras B satisfying
are “almost” automorphisms. In particular, if T preserves the unit, then T is an automorphism. His results were extended, in several directions, for uniform algebras and semisimple commutative Banach algebras by, among others, Hatori et al. in [Reference Hatori, Miura and Takagi4], and by Rao and Roy in [Reference Rao and Roy12, Reference Rao and Roy13]. Hatori et al., in [Reference Hatori, Miura and Takagi5], proposed that if the Kowalski–Słodkowski theorem could be modified (multiplicatively) so that a map
![]() $\phi $
from a Banach algebra B (with unit
$\phi $
from a Banach algebra B (with unit
![]() $\mathbf {1}$
) into
$\mathbf {1}$
) into
![]() $\mathbb {C}$
satisfying
$\mathbb {C}$
satisfying
was always linear and multiplicative, then some theorems of Molnár and their generalizations could be proved in a way similar to the proof of Theorem 1.2. However, as one sees in [Reference Maouche8, pp. 44–45] and [Reference Touré, Schulz and Brits15, p. 56], a raw multiplicative version of the Kowalski–Słodkowski theorem does not even hold for commutative
![]() $C^\star $
-algebras. However, one should observe that in these examples, the map
$C^\star $
-algebras. However, one should observe that in these examples, the map
![]() $\phi $
is not continuous, and hence any hope of proving the multiplicative result would require continuity to be added as an assumption. What we will show here, at least for the case where A is a
$\phi $
is not continuous, and hence any hope of proving the multiplicative result would require continuity to be added as an assumption. What we will show here, at least for the case where A is a
![]() $C^\star $
-algebra, is that continuity is the only problem which needs to be addressed. To formalize our main result:
$C^\star $
-algebra, is that continuity is the only problem which needs to be addressed. To formalize our main result:
Main Result If A is a unital
![]() $C^\star $
-algebra, and if
$C^\star $
-algebra, and if
![]() $\phi :A\to \mathbb C$
is a continuous function that satisfies
$\phi :A\to \mathbb C$
is a continuous function that satisfies
then
![]() $\phi $
must be a character of A.
$\phi $
must be a character of A.
As an easy corollary of our main result, we can then obtain a multiplicative version of Hatori et al.’ s Theorem 1.2 (stated above).
Corollary Let A be a unital
![]() $C^\star $
-algebra, and let
$C^\star $
-algebra, and let
![]() $B'$
be a unital, semisimple, commutative Banach algebra. Suppose that T is a continuous map from A into
$B'$
be a unital, semisimple, commutative Banach algebra. Suppose that T is a continuous map from A into
![]() $B'$
such that
$B'$
such that
![]() $\sigma (T(a)T(b))\subseteq \sigma (a+b)$
holds for every pair a and b in A. Then T is linear and multiplicative.
$\sigma (T(a)T(b))\subseteq \sigma (a+b)$
holds for every pair a and b in A. Then T is linear and multiplicative.
2 Preliminaries
Throughout the remainder of this paper, A denotes a complex and unital
![]() $C^\star $
-algebra with unit
$C^\star $
-algebra with unit
![]() $\mathbf {1}$
and additive identity
$\mathbf {1}$
and additive identity
![]() $\mathbf {0}.$
Since our proofs are elementary (but far from trivial), it suffices to consult a standard reference, such as [Reference Rudin14], for the basics of
$\mathbf {0}.$
Since our proofs are elementary (but far from trivial), it suffices to consult a standard reference, such as [Reference Rudin14], for the basics of
![]() $C^\star $
-algebras. We denote by
$C^\star $
-algebras. We denote by
![]() ${\mathcal S}_A $
the set of self-adjoint elements of A, and by
${\mathcal S}_A $
the set of self-adjoint elements of A, and by
![]() $G(A)$
the set of invertible elements of A. Furthermore, we write
$G(A)$
the set of invertible elements of A. Furthermore, we write
![]() $G_{\mathbf 1}(A)$
for the connected component of the group
$G_{\mathbf 1}(A)$
for the connected component of the group
![]() $G(A)$
containing the unit. It is well known that
$G(A)$
containing the unit. It is well known that
For any x in
![]() $A $
, we shall use
$A $
, we shall use
![]() $\sigma $
and
$\sigma $
and
![]() $\rho $
to denote, respectively, the spectrum
$\rho $
to denote, respectively, the spectrum
![]() $ \sigma (x) := \{ \lambda \in \mathbb {C} : \lambda \mathbf {1} -x \notin G(A) \},$
and the spectral radius
$ \sigma (x) := \{ \lambda \in \mathbb {C} : \lambda \mathbf {1} -x \notin G(A) \},$
and the spectral radius
![]() $ \rho (x) := \sup \{ \vert \lambda \vert : \lambda \in \sigma (x) \}$
of x. For every
$ \rho (x) := \sup \{ \vert \lambda \vert : \lambda \in \sigma (x) \}$
of x. For every
![]() $x\in A$
,
$x\in A$
,
![]() $|x|$
denotes the positive square root of
$|x|$
denotes the positive square root of
![]() $x^\star x$
. By a functional on A, we shall mean any function that maps A to
$x^\star x$
. By a functional on A, we shall mean any function that maps A to
![]() $\mathbb {C}$
; specifically, for the purpose of this paper, a functional is not necessarily linear. A character of A is a linear functional
$\mathbb {C}$
; specifically, for the purpose of this paper, a functional is not necessarily linear. A character of A is a linear functional
![]() $\chi :A\to \mathbb {C}$
which is simultaneously multiplicative, i.e.,
$\chi :A\to \mathbb {C}$
which is simultaneously multiplicative, i.e.,
![]() $\chi (ab)=\chi (a)\chi (b)$
for every
$\chi (ab)=\chi (a)\chi (b)$
for every
![]() $a,b\in A$
. To shorten the statements of some results, we also introduce the following general terminology.
$a,b\in A$
. To shorten the statements of some results, we also introduce the following general terminology.
Definition 2.1 (Multiplicatively spectral functional)
Let B be a unital complex Banach algebra. Then a functional
![]() $\phi :B\to \mathbb C$
is said to be a multiplicatively spectral functional on B whenever
$\phi :B\to \mathbb C$
is said to be a multiplicatively spectral functional on B whenever
![]() $\phi $
satisfies the following two conditions:
$\phi $
satisfies the following two conditions:
-
(P1)
 $\phi (x)\phi (y) \in \sigma (xy)$
, for all
$\phi (x)\phi (y) \in \sigma (xy)$
, for all
 $x,y\in B$
.
$x,y\in B$
. -
(P2)
 $\phi (\mathbf 1)=1$
.
$\phi (\mathbf 1)=1$
.
Definition 2.2 (Spectrally multiplicative functional)
Let B be a unital complex Banach algebra. Then a functional
![]() $\phi :B\to \mathbb C$
is said to be a spectrally multiplicative functional on B whenever
$\phi :B\to \mathbb C$
is said to be a spectrally multiplicative functional on B whenever
![]() $\phi $
satisfies the following condition:
$\phi $
satisfies the following condition:
-
(P1)
 $\phi (x)\phi (y) \in \sigma (xy)$
, for all
$\phi (x)\phi (y) \in \sigma (xy)$
, for all
 $x,y\in B$
.
$x,y\in B$
.
Our motivation for this terminology follows from the observation that if
![]() $\phi $
satisfies (P1) and (P2), then
$\phi $
satisfies (P1) and (P2), then
![]() $\phi $
takes values in the spectrum; if
$\phi $
takes values in the spectrum; if
![]() $\phi $
satisfies only (P1), then either
$\phi $
satisfies only (P1), then either
![]() $\phi $
or
$\phi $
or
![]() $-\phi $
takes values in the spectrum. So, if
$-\phi $
takes values in the spectrum. So, if
![]() $\phi $
is a spectrally multiplicative functional, then either
$\phi $
is a spectrally multiplicative functional, then either
![]() $\phi $
or
$\phi $
or
![]() $-\phi $
is a multiplicatively spectral functional. For
$-\phi $
is a multiplicatively spectral functional. For
![]() $x\in A$
, we will denote the real and imaginary parts of x by, respectively,
$x\in A$
, we will denote the real and imaginary parts of x by, respectively,
![]() $R_x$
and
$R_x$
and
![]() $I_x$
, that is,
$I_x$
, that is,
If there is no danger of confusion, we will simply write
In [Reference Touré, Schulz and Brits16], the authors showed that if
![]() $\phi :A\to \mathbb {C}$
is continuous and satisfies (P1) and (P2), then
$\phi :A\to \mathbb {C}$
is continuous and satisfies (P1) and (P2), then
![]() $\phi $
generates a character
$\phi $
generates a character
![]() $\psi _\phi $
of A, and, moreover,
$\psi _\phi $
of A, and, moreover,
![]() $\psi _\phi $
and
$\psi _\phi $
and
![]() $\phi $
agree on certain subsets of A; the precise statement, which will be the starting point of the proof of our main result, is as follows.
$\phi $
agree on certain subsets of A; the precise statement, which will be the starting point of the proof of our main result, is as follows.
Theorem 2.1 [Reference Touré, Schulz and Brits16, Theorems 3.7 and 3.9]
Let
![]() $\phi $
be a continuous multiplicatively spectral functional on a
$\phi $
be a continuous multiplicatively spectral functional on a
![]() $C^\star $
-algebra A. Then the formula
$C^\star $
-algebra A. Then the formula
defines a character on A. Furthermore,
![]() $ \phi (x) = \psi _\phi (x) $
whenever x belongs to
$ \phi (x) = \psi _\phi (x) $
whenever x belongs to
![]() $G_{\mathbf 1}(A)\cup {\mathcal S}_A $
.
$G_{\mathbf 1}(A)\cup {\mathcal S}_A $
.
3 Proof of the main result
Proceeding through a series of lemmas, we show that any continuous multiplicatively spectral functional on a
![]() $C^\star $
-algebra is a character. Our first three results are rather easy, and we give the short proofs for the sake of completeness. Furthermore, throughout this section, the function
$C^\star $
-algebra is a character. Our first three results are rather easy, and we give the short proofs for the sake of completeness. Furthermore, throughout this section, the function
![]() $\psi _\phi $
refers to the character in Theorem 2.1.
$\psi _\phi $
refers to the character in Theorem 2.1.
Lemma 3.1 Let
![]() $a, b $
be positive elements of a
$a, b $
be positive elements of a
![]() $C^\star $
-algebra A such that
$C^\star $
-algebra A such that
![]() $a^2\leq b^2$
, and let
$a^2\leq b^2$
, and let
![]() $(v_n)$
be a sequence in A such that
$(v_n)$
be a sequence in A such that
![]() $ \lim _n b v_n= \mathbf {0}$
. Then
$ \lim _n b v_n= \mathbf {0}$
. Then
![]() $\lim _n a v_n= \mathbf {0} $
.
$\lim _n a v_n= \mathbf {0} $
.
Proof It suffices to show that
![]() $\Vert av_n\Vert ^2 \leq \Vert b v_n \Vert ^2$
for every n. Observe first that
$\Vert av_n\Vert ^2 \leq \Vert b v_n \Vert ^2$
for every n. Observe first that
Since
![]() $ a^2\leq b^2$
, we have
$ a^2\leq b^2$
, we have
![]() $v_n^\star a^2 v_n\leq v_n^\star b^2 v_n$
and hence
$v_n^\star a^2 v_n\leq v_n^\star b^2 v_n$
and hence
![]() $ \Vert v_n^\star a^2 v_n \Vert \leq \Vert v_n^\star b^2 v_n \Vert =\Vert b v_n \Vert ^2 $
. Consequently, we get
$ \Vert v_n^\star a^2 v_n \Vert \leq \Vert v_n^\star b^2 v_n \Vert =\Vert b v_n \Vert ^2 $
. Consequently, we get
![]() $ \Vert a v_n \Vert ^2 \leq \Vert b v_n \Vert ^2$
.
$ \Vert a v_n \Vert ^2 \leq \Vert b v_n \Vert ^2$
.
Corollary 3.2 Let A be a
![]() $C^\star $
-algebra,
$C^\star $
-algebra,
![]() $a\in {\mathcal S}_A$
, and let
$a\in {\mathcal S}_A$
, and let
![]() $(v_n)$
be a sequence in A such that
$(v_n)$
be a sequence in A such that
![]() $\lim _n \vert a \vert v_n =\mathbf {0}$
. Then
$\lim _n \vert a \vert v_n =\mathbf {0}$
. Then
![]() $\lim _n a v_n =\mathbf {0} .$
$\lim _n a v_n =\mathbf {0} .$
Proof Clearly,
![]() $\mathbf {0} \leq (\vert a \vert -a)^2 \leq (2\vert a \vert )^2$
. Invoking Lemma 3.1, we infer that
$\mathbf {0} \leq (\vert a \vert -a)^2 \leq (2\vert a \vert )^2$
. Invoking Lemma 3.1, we infer that
![]() $\lim _n (\vert a \vert -a)v_n= \mathbf {0} \implies \lim _n a v_n= \mathbf {0} $
.
$\lim _n (\vert a \vert -a)v_n= \mathbf {0} \implies \lim _n a v_n= \mathbf {0} $
.
Lemma 3.3 Let x be an element of
![]() ${\mathcal S}_A$
. Then
${\mathcal S}_A$
. Then
Proof We shall prove the result where a is any positive element of A: by the Gelfand–Naimark theorem, we can assume, without loss of generality, that A is commutative so that
![]() $A=C(X)$
for some compact set X. For each
$A=C(X)$
for some compact set X. For each
![]() $n\in \mathbb N$
, define
$n\in \mathbb N$
, define
![]() $b_n=a e^{-na} $
and
$b_n=a e^{-na} $
and
![]() $c_n=a \left (\mathbf {1}+in a\right )^{-1}$
. Then
$c_n=a \left (\mathbf {1}+in a\right )^{-1}$
. Then
and hence
![]() $\lim _n b_n=\mathbf {0}.$
Similarly,
$\lim _n b_n=\mathbf {0}.$
Similarly,
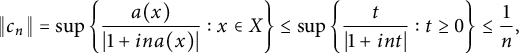 $$ \begin{align*} \Vert c_n \Vert=\sup_{}\left\{ \frac{a(x)}{ \vert 1 +i na(x) \vert } : x \in X\right\} \leq \sup_{ }\left\{ \frac{t}{ \vert 1 +i n t \vert }: t \geq 0 \right\} \leq \frac{1}{n}, \end{align*} $$
$$ \begin{align*} \Vert c_n \Vert=\sup_{}\left\{ \frac{a(x)}{ \vert 1 +i na(x) \vert } : x \in X\right\} \leq \sup_{ }\left\{ \frac{t}{ \vert 1 +i n t \vert }: t \geq 0 \right\} \leq \frac{1}{n}, \end{align*} $$
so that
![]() $\lim _n c_n=\mathbf {0}.$
Since
$\lim _n c_n=\mathbf {0}.$
Since
![]() $\vert x \vert $
is positive, we have the result.
$\vert x \vert $
is positive, we have the result.
The next three lemmas progressively narrow down the connection between
![]() $\phi $
and
$\phi $
and
![]() $\psi _\phi $
: the idea is to construct two sequences (from the sequences
$\psi _\phi $
: the idea is to construct two sequences (from the sequences
![]() $b_n$
and
$b_n$
and
![]() $c_n$
which were defined in the proof of Lemma 3.3) and then to filter them through the spectrum while simultaneously keeping track of
$c_n$
which were defined in the proof of Lemma 3.3) and then to filter them through the spectrum while simultaneously keeping track of
![]() $\phi $
and
$\phi $
and
![]() $\psi _\phi $
via the condition (P1) and Theorem 2.1. The main result then follows from a compactness argument using the results obtained in the preceding lemmas.
$\psi _\phi $
via the condition (P1) and Theorem 2.1. The main result then follows from a compactness argument using the results obtained in the preceding lemmas.
Lemma 3.4 Let
![]() $\phi $
be a continuous multiplicatively spectral functional on a
$\phi $
be a continuous multiplicatively spectral functional on a
![]() $C^\star $
-algebra A, and suppose that
$C^\star $
-algebra A, and suppose that
![]() $x\in A$
satisfies
$x\in A$
satisfies
![]() $\psi _\phi (x) = 0$
. Then
$\psi _\phi (x) = 0$
. Then
![]() $\phi (x)=0$
.
$\phi (x)=0$
.
Proof We know that
![]() $\psi _\phi (x) = 0=\psi _\phi \left ( R+i I\right)\hspace{-1.2pt}{,}$
which implies that
$\psi _\phi (x) = 0=\psi _\phi \left ( R+i I\right)\hspace{-1.2pt}{,}$
which implies that
![]() $\psi _\phi \left ( R\right )=\psi _\phi \left ( I\right )=0.$
For each
$\psi _\phi \left ( R\right )=\psi _\phi \left ( I\right )=0.$
For each
![]() $n\in \mathbb N$
, let
$n\in \mathbb N$
, let
![]() $W_n:=e^{-n \sqrt {\vert R \vert ^2+\vert I \vert ^2} }$
and observe, using Theorem 2.1, that
$W_n:=e^{-n \sqrt {\vert R \vert ^2+\vert I \vert ^2} }$
and observe, using Theorem 2.1, that
![]() $\phi \left ( W_n\right )=\psi _\phi \left ( W_n\right )=1$
. From (P1), it follows that
$\phi \left ( W_n\right )=\psi _\phi \left ( W_n\right )=1$
. From (P1), it follows that
Using Lemmas 3.1 and 3.3 and Corollary 3.2, we deduce that
and similarly,
![]() $\lim _n I W_n= \mathbf {0}.$
Thus,
$\lim _n I W_n= \mathbf {0}.$
Thus,
Using (3.1), we have that
![]() $\vert \phi (x)\vert \leq \|xW_n\|$
and so, by taking the limit as
$\vert \phi (x)\vert \leq \|xW_n\|$
and so, by taking the limit as
![]() $n\to \infty $
, we get
$n\to \infty $
, we get
![]() $\phi (x)=0$
.
$\phi (x)=0$
.
Lemma 3.5 Let
![]() $\phi $
be a continuous multiplicatively spectral functional on a
$\phi $
be a continuous multiplicatively spectral functional on a
![]() $C^\star $
-algebra A,
$C^\star $
-algebra A,
![]() $\alpha \in \mathbb {C} $
, and suppose that
$\alpha \in \mathbb {C} $
, and suppose that
![]() $x\in A$
satisfies
$x\in A$
satisfies
![]() $\psi _\phi (x)=0 $
. Then
$\psi _\phi (x)=0 $
. Then
![]() $\phi (\alpha \mathbf {1}+ x) =c_\alpha \alpha $
, for some
$\phi (\alpha \mathbf {1}+ x) =c_\alpha \alpha $
, for some
![]() $c_\alpha \in [0,1]$
.
$c_\alpha \in [0,1]$
.
Proof Let
![]() $\alpha $
be an arbitrary nonzero complex number. With
$\alpha $
be an arbitrary nonzero complex number. With
![]() $W_n$
defined as in the proof of Lemma 3.4, let
$W_n$
defined as in the proof of Lemma 3.4, let
![]() $Y_n:=\frac {1}{ \alpha }xW_n$
, and set
$Y_n:=\frac {1}{ \alpha }xW_n$
, and set
![]() $c_\alpha :=\frac {1}{ \alpha }\phi (\alpha \mathbf {1}+x)$
. Then, using (P1) together with
$c_\alpha :=\frac {1}{ \alpha }\phi (\alpha \mathbf {1}+x)$
. Then, using (P1) together with
![]() $\phi \left (W_n \right )=1$
, for each
$\phi \left (W_n \right )=1$
, for each
![]() $n\in \mathbb N$
,
$n\in \mathbb N$
,
Observe, from the proof of Lemma 3.4, that
![]() $\lim _n Y_n = \mathbf {0}$
. To show that
$\lim _n Y_n = \mathbf {0}$
. To show that
![]() $c_\alpha \in [0,1],$
assume, to the contrary, that
$c_\alpha \in [0,1],$
assume, to the contrary, that
![]() $c_\alpha \notin [0, 1].$
For each n, we have that
$c_\alpha \notin [0, 1].$
For each n, we have that
![]() $W_n\in {\mathcal S}_A$
and
$W_n\in {\mathcal S}_A$
and
![]() $\sigma (W_n ) \subseteq [0,1] $
. From (3.2), we see that
$\sigma (W_n ) \subseteq [0,1] $
. From (3.2), we see that
Since
![]() $( c_\alpha \mathbf {1} -W_n)^{-1}$
is normal for each
$( c_\alpha \mathbf {1} -W_n)^{-1}$
is normal for each
![]() $n,$
we have the estimation
$n,$
we have the estimation
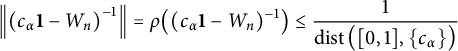 $$ \begin{align*}\left\Vert\left( c_\alpha\mathbf{1} -W_n\right)^{-1} \right\Vert=\rho\big( ( c_\alpha\mathbf{1} -W_n)^{-1} \big) \leq \frac{1}{\operatorname{\mathrm{dist}}\big([0,1] , \{c_\alpha\}\big) }\end{align*} $$
$$ \begin{align*}\left\Vert\left( c_\alpha\mathbf{1} -W_n\right)^{-1} \right\Vert=\rho\big( ( c_\alpha\mathbf{1} -W_n)^{-1} \big) \leq \frac{1}{\operatorname{\mathrm{dist}}\big([0,1] , \{c_\alpha\}\big) }\end{align*} $$
from which it follows that
![]() $\lim _n Y_n( c_\alpha \mathbf {1} -W_n)^{-1} =\mathbf {0}$
, hence contradicting the fact that
$\lim _n Y_n( c_\alpha \mathbf {1} -W_n)^{-1} =\mathbf {0}$
, hence contradicting the fact that
![]() $ G(A) $
is open. Therefore,
$ G(A) $
is open. Therefore,
![]() $c_\alpha \in [0,1]$
, and thus
$c_\alpha \in [0,1]$
, and thus
![]() $\phi (\alpha \mathbf {1}+x)=c_\alpha \alpha .$
$\phi (\alpha \mathbf {1}+x)=c_\alpha \alpha .$
The final lemma shows that the value of
![]() $c_\alpha $
in Lemma 3.5 can be narrowed down to one of two possibilities.
$c_\alpha $
in Lemma 3.5 can be narrowed down to one of two possibilities.
Lemma 3.6 Let
![]() $\phi $
be a continuous multiplicatively spectral functional on a
$\phi $
be a continuous multiplicatively spectral functional on a
![]() $C^\star $
-algebra A. If
$C^\star $
-algebra A. If
![]() $\alpha \in \mathbb {C} $
and
$\alpha \in \mathbb {C} $
and
![]() $x\in A$
satisfies
$x\in A$
satisfies
![]() $\psi _\phi (x)=0 $
, then
$\psi _\phi (x)=0 $
, then
![]() $\phi (\alpha \mathbf {1}+ x)\in \{ 0,\alpha \} .$
$\phi (\alpha \mathbf {1}+ x)\in \{ 0,\alpha \} .$
Proof For each
![]() $n\in \mathbb N$
, let
$n\in \mathbb N$
, let
![]() $V_n:=\left (\mathbf {1}+in\sqrt {\vert R \vert ^2+\vert I \vert ^2} \right )^{-1}\hspace{-2pt}.$
Again, using Lemmas 3.1 and 3.3 and Corollary 3.2, we have that
$V_n:=\left (\mathbf {1}+in\sqrt {\vert R \vert ^2+\vert I \vert ^2} \right )^{-1}\hspace{-2pt}.$
Again, using Lemmas 3.1 and 3.3 and Corollary 3.2, we have that
Similarly,
![]() $\lim _n I V_n=\mathbf {0} $
. Observe that each
$\lim _n I V_n=\mathbf {0} $
. Observe that each
![]() $V_n$
belongs to
$V_n$
belongs to
![]() $ G_{\mathbf 1}(A)$
, whence it follows that
$ G_{\mathbf 1}(A)$
, whence it follows that
![]() $\phi (V_n) = \psi _\phi (V_n)=1 $
. Let
$\phi (V_n) = \psi _\phi (V_n)=1 $
. Let
![]() $\alpha \neq 0 $
. From Lemma 3.5, we have that
$\alpha \neq 0 $
. From Lemma 3.5, we have that
![]() $\phi (\alpha \mathbf {1}+x)=c_\alpha \alpha $
, with
$\phi (\alpha \mathbf {1}+x)=c_\alpha \alpha $
, with
![]() $c_\alpha \in [0,1]$
. To obtain the result, we have to show that
$c_\alpha \in [0,1]$
. To obtain the result, we have to show that
![]() $c_\alpha \in \{0,1\}$
: For the sake of a contradiction, assume that
$c_\alpha \in \{0,1\}$
: For the sake of a contradiction, assume that
![]() $ 0< c_\alpha <1$
. If we set
$ 0< c_\alpha <1$
. If we set
![]() $Z_n:=\frac {1}{\alpha }xV_n=\frac {1}{\alpha }(R+iI) V_n$
, then
$Z_n:=\frac {1}{\alpha }xV_n=\frac {1}{\alpha }(R+iI) V_n$
, then
The first paragraph of the proof shows that
![]() $\lim _n Z_n=\mathbf {0}$
, and (3.3) shows that
$\lim _n Z_n=\mathbf {0}$
, and (3.3) shows that
![]() $c_\alpha \mathbf {1}-V_n-Z_n \notin G(A) $
. From the definition of
$c_\alpha \mathbf {1}-V_n-Z_n \notin G(A) $
. From the definition of
![]() $V_n$
, we have that
$V_n$
, we have that
![]() $\sigma \left (V_n\right ) \subseteq C_r $
, where
$\sigma \left (V_n\right ) \subseteq C_r $
, where
![]() $C_r$
is the circle in
$C_r$
is the circle in
![]() $\mathbb C$
with center
$\mathbb C$
with center
![]() $\frac {1}{2}$
and radius
$\frac {1}{2}$
and radius
![]() $\frac {1}{2}$
: indeed, if
$\frac {1}{2}$
: indeed, if
![]() $\theta \in \sigma \left (V_n\right )$
, then
$\theta \in \sigma \left (V_n\right )$
, then
![]() $\theta =\frac {1}{1+v^2} +i\frac {-v}{1+v^2}$
, for some
$\theta =\frac {1}{1+v^2} +i\frac {-v}{1+v^2}$
, for some
![]() $v \geq 0$
, and so
$v \geq 0$
, and so
![]() $\left ( \operatorname {\mathrm {Re}}(\theta )-\frac {1}{2}\right )^2+\left ( \operatorname {\mathrm {Im}}(\theta )\right )^2=\frac {1}{4} $
. Owing to the fact that
$\left ( \operatorname {\mathrm {Re}}(\theta )-\frac {1}{2}\right )^2+\left ( \operatorname {\mathrm {Im}}(\theta )\right )^2=\frac {1}{4} $
. Owing to the fact that
![]() $C_r \cap \mathbb {R} =\{ 0,1\} $
, we infer that
$C_r \cap \mathbb {R} =\{ 0,1\} $
, we infer that
![]() $c_\alpha \notin \sigma \left (V_n\right )$
. Thus,
$c_\alpha \notin \sigma \left (V_n\right )$
. Thus,
which together implies that
![]() $ \mathbf {1}-Z_n\left ( c_\alpha \mathbf {1} -V_n\right )^{-1} \notin G(A) .$
Since
$ \mathbf {1}-Z_n\left ( c_\alpha \mathbf {1} -V_n\right )^{-1} \notin G(A) .$
Since
![]() $V_n$
is normal, we obtain the estimate
$V_n$
is normal, we obtain the estimate
from which it follows that
![]() $\lim _n Z_n\left ( c_\alpha \mathbf {1} -V_n\right )^{-1}= \mathbf {0}$
, contradicting the fact that
$\lim _n Z_n\left ( c_\alpha \mathbf {1} -V_n\right )^{-1}= \mathbf {0}$
, contradicting the fact that
![]() $G(A)$
is open. Subsequently,
$G(A)$
is open. Subsequently,
![]() $c_\alpha \in \{0,1\}$
, and
$c_\alpha \in \{0,1\}$
, and
![]() $\phi (\alpha \mathbf {1}+x)\in \{0, \alpha \}$
follows as advertised.
$\phi (\alpha \mathbf {1}+x)\in \{0, \alpha \}$
follows as advertised.
We are now in a position to prove the main result.
Theorem 3.7 Let
![]() $\phi $
be a continuous multiplicatively spectral functional on a
$\phi $
be a continuous multiplicatively spectral functional on a
![]() $C^\star $
-algebra A. Then
$C^\star $
-algebra A. Then
![]() $\phi (x) = \psi _\phi (x)$
for all x in A, and hence
$\phi (x) = \psi _\phi (x)$
for all x in A, and hence
![]() $\phi $
is a character of A.
$\phi $
is a character of A.
Proof For
![]() $x\in A$
, define
$x\in A$
, define
![]() $K_x:=\{\alpha \in \mathbb {C} : \phi (\alpha \mathbf {1}+x)=0\}$
, and assume first that
$K_x:=\{\alpha \in \mathbb {C} : \phi (\alpha \mathbf {1}+x)=0\}$
, and assume first that
![]() $\psi _\phi (x)=0$
. Our aim is to prove that
$\psi _\phi (x)=0$
. Our aim is to prove that
![]() $K_x=\{0\}$
. Observe that
$K_x=\{0\}$
. Observe that
![]() $0 \in K_x$
(by Lemma 3.4),
$0 \in K_x$
(by Lemma 3.4),
![]() $K_x \subseteq \sigma (-x)$
(by P(1) and P(2)), and
$K_x \subseteq \sigma (-x)$
(by P(1) and P(2)), and
![]() $K_x$
is closed (since
$K_x$
is closed (since
![]() $\phi $
is continuous). Thus,
$\phi $
is continuous). Thus,
![]() $K_x$
is nonempty and compact. Let m be a maximum modulus element of
$K_x$
is nonempty and compact. Let m be a maximum modulus element of
![]() $K_x$
. From the definition of m, there is a sequence
$K_x$
. From the definition of m, there is a sequence
![]() $(k_n) \subset \mathbb C\setminus K_x$
which converges to m. Therefore, by Lemma 3.6,
$(k_n) \subset \mathbb C\setminus K_x$
which converges to m. Therefore, by Lemma 3.6,
![]() $\lim _n\phi \big (k_n\mathbf {1}+x \big )=\lim _n k_n =m $
, and by continuity of
$\lim _n\phi \big (k_n\mathbf {1}+x \big )=\lim _n k_n =m $
, and by continuity of
![]() $\phi $
,
$\phi $
,
![]() $\lim _n\phi \big (k_n\mathbf {1}+x \big )=\phi \big (m\mathbf {1}+x \big )=0 $
. Thus,
$\lim _n\phi \big (k_n\mathbf {1}+x \big )=\phi \big (m\mathbf {1}+x \big )=0 $
. Thus,
![]() $m=0$
from which it follows that
$m=0$
from which it follows that
![]() $K_x=\{0\}$
. Invoking Lemma 3.6 again, we then obtain
$K_x=\{0\}$
. Invoking Lemma 3.6 again, we then obtain
![]() $\phi (\alpha \mathbf {1}+x)=\alpha $
for each
$\phi (\alpha \mathbf {1}+x)=\alpha $
for each
![]() $\alpha \in \mathbb C$
. For any value of
$\alpha \in \mathbb C$
. For any value of
![]() $ \psi _\phi (x) $
, we use the first part of the proof to deduce that
$ \psi _\phi (x) $
, we use the first part of the proof to deduce that
As a direct consequence of Theorem 3.7, one also has the following.
Corollary 3.8 Let
![]() $\phi $
be a continuous spectrally multiplicative functional on a
$\phi $
be a continuous spectrally multiplicative functional on a
![]() $C^\star $
-algebra A. Then either
$C^\star $
-algebra A. Then either
![]() $\phi $
is a character of A or
$\phi $
is a character of A or
![]() $-\phi $
is a character of A.
$-\phi $
is a character of A.
Proof If
![]() $\phi $
is spectrally multiplicative, then either
$\phi $
is spectrally multiplicative, then either
![]() $\phi $
or
$\phi $
or
![]() $-\phi $
is multiplicatively spectral. The result then follows from Theorem 3.7.
$-\phi $
is multiplicatively spectral. The result then follows from Theorem 3.7.
As a further consequence of Theorem 3.7, we can now address a problem raised by Hatori et al. [Reference Hatori, Miura and Takagi5, p. 286] in the case where A is a
![]() $C^\star $
-algebra.
$C^\star $
-algebra.
Corollary 3.9 Let A be a
![]() $C^\star $
-algebra, let B be a semisimple commutative Banach algebra, and suppose
$C^\star $
-algebra, let B be a semisimple commutative Banach algebra, and suppose
![]() $\phi :A\to B$
is a function that satisfies:
$\phi :A\to B$
is a function that satisfies:
-
(1)
 $\sigma \left (\phi (x)\phi (y)\right ) \subseteq \sigma (xy)$
, for all
$\sigma \left (\phi (x)\phi (y)\right ) \subseteq \sigma (xy)$
, for all
 $x,y\in A$
,
$x,y\in A$
, -
(2)
 $\phi (\mathbf 1)=\mathbf 1$
,
$\phi (\mathbf 1)=\mathbf 1$
, -
(3)
 $\phi $
is continuous on A.
$\phi $
is continuous on A.
Then
![]() $\phi $
is a homomorphism.
$\phi $
is a homomorphism.
Proof Let
![]() $\chi $
be an arbitrary character of B. Then
$\chi $
be an arbitrary character of B. Then
![]() $\chi \circ \phi $
is a continuous multiplicatively spectral functional on A. Hence, by Theorem 3.7,
$\chi \circ \phi $
is a continuous multiplicatively spectral functional on A. Hence, by Theorem 3.7,
![]() $\chi \circ \phi $
is a character. The result then follows from the semisimplicity of B.
$\chi \circ \phi $
is a character. The result then follows from the semisimplicity of B.