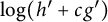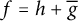1 Introduction
A central problem pertaining to the growth of univalent harmonic mappings is to determine the exact range of
![]() $p>0$
so that these functions belong to the harmonic Hardy space
$p>0$
so that these functions belong to the harmonic Hardy space
![]() $h^p$
. The early developments in this direction were due to Abu-Muhanna and Lyzzaik [Reference Abu-Muhanna and Lyzzaik1], which were later improved by Nowak [Reference Nowak17]. She proved sharp results for the classes of convex and close-to-convex harmonic functions, and conjectured an analogous range of p for the whole class
$h^p$
. The early developments in this direction were due to Abu-Muhanna and Lyzzaik [Reference Abu-Muhanna and Lyzzaik1], which were later improved by Nowak [Reference Nowak17]. She proved sharp results for the classes of convex and close-to-convex harmonic functions, and conjectured an analogous range of p for the whole class
![]() $S_H$
of normalized univalent harmonic functions. The aim of this paper is to verify this conjecture for functions
$S_H$
of normalized univalent harmonic functions. The aim of this paper is to verify this conjecture for functions
![]() $f \in S_H$
with an additional property. The main theorems and their implications are presented in Section 2, while the proofs are given in Section 4. Section 3 contains results from the literature that are useful for our purpose.
$f \in S_H$
with an additional property. The main theorems and their implications are presented in Section 2, while the proofs are given in Section 4. Section 3 contains results from the literature that are useful for our purpose.
A function f analytic in the open unit disk
![]() ${\mathbb D} = \{z: \; \vert z \vert <1\}$
is of class
${\mathbb D} = \{z: \; \vert z \vert <1\}$
is of class
![]() $H^p$
$H^p$
![]() $(0 < p \le \infty )$
if the integral means
$(0 < p \le \infty )$
if the integral means
 $$ \begin{align*}M_p(r, f) = \left\{ \begin{array}{ll} \displaystyle \left( \frac{1}{2\pi}\int_{0}^{2\pi}\vert f(r e^{i\theta}) \vert ^p d\theta \right)^{\frac{1}{p}}, \quad & 0 < p < \infty, \\ \displaystyle \sup_{ \vert z \vert=r} \vert f(z) \vert, \quad & p = \infty \end{array} \right.\end{align*} $$
$$ \begin{align*}M_p(r, f) = \left\{ \begin{array}{ll} \displaystyle \left( \frac{1}{2\pi}\int_{0}^{2\pi}\vert f(r e^{i\theta}) \vert ^p d\theta \right)^{\frac{1}{p}}, \quad & 0 < p < \infty, \\ \displaystyle \sup_{ \vert z \vert=r} \vert f(z) \vert, \quad & p = \infty \end{array} \right.\end{align*} $$
remain bounded as
![]() $r \to 1^-$
. The norm of a function
$r \to 1^-$
. The norm of a function
![]() $f \in H^p$
is defined as
$f \in H^p$
is defined as
![]() ${\|f\|_p = \lim _{r \to 1^-} M_p(r,f).}$
Integral means and
${\|f\|_p = \lim _{r \to 1^-} M_p(r,f).}$
Integral means and
![]() $H^p$
spaces play a fundamental role in studies concerning the growth of functions; we refer to the books [Reference Duren9, Reference Koosis14, Reference Pavlović18] for a detailed survey.
$H^p$
spaces play a fundamental role in studies concerning the growth of functions; we refer to the books [Reference Duren9, Reference Koosis14, Reference Pavlović18] for a detailed survey.
A harmonic function f in the unit disk has a unique representation
![]() $f=h+\overline {g}$
, where h, g are analytic functions in
$f=h+\overline {g}$
, where h, g are analytic functions in
![]() $\mathbb {D}$
with
$\mathbb {D}$
with
![]() $g(0)=0$
. The function f is locally univalent and sense-preserving if, and only if, the Jacobian
$g(0)=0$
. The function f is locally univalent and sense-preserving if, and only if, the Jacobian
![]() $J_f(z)=\vert h'(z)\vert ^2-\vert g'(z)\vert ^2$
is positive for all
$J_f(z)=\vert h'(z)\vert ^2-\vert g'(z)\vert ^2$
is positive for all
![]() $z \in {\mathbb D}$
. Let
$z \in {\mathbb D}$
. Let
![]() $S_H$
be the class of all sense-preserving univalent harmonic functions f in
$S_H$
be the class of all sense-preserving univalent harmonic functions f in
![]() ${\mathbb D}$
, normalized by the conditions
${\mathbb D}$
, normalized by the conditions
![]() $h(0)=g(0)=h'(0)-1=0$
. Denote by
$h(0)=g(0)=h'(0)-1=0$
. Denote by
![]() $K_H$
and
$K_H$
and
![]() $C_H$
the subclasses of
$C_H$
the subclasses of
![]() $S_H$
consisting of harmonic mappings onto convex and close-to-convex regions, respectively. Let
$S_H$
consisting of harmonic mappings onto convex and close-to-convex regions, respectively. Let
![]() $S_H^0=\{f=h+\overline {g} \in S_H : g'(0)=0\}$
,
$S_H^0=\{f=h+\overline {g} \in S_H : g'(0)=0\}$
,
![]() $K_H^0=K_H \cap S_H^0$
, and
$K_H^0=K_H \cap S_H^0$
, and
![]() $C_H^0=C_H \cap S_H^0$
. Two leading examples of univalent harmonic functions are the harmonic Koebe function
$C_H^0=C_H \cap S_H^0$
. Two leading examples of univalent harmonic functions are the harmonic Koebe function
 $$ \begin{align*}K(z) = H(z) + \overline{G(z)} = \left(\frac{z-\frac{1}{2}z^2+\frac{1}{6}z^3}{(1-z)^3}\right) + \overline{\left(\frac{\frac{1}{2}z^2+\frac{1}{6}z^3}{(1-z)^3}\right)}\end{align*} $$
$$ \begin{align*}K(z) = H(z) + \overline{G(z)} = \left(\frac{z-\frac{1}{2}z^2+\frac{1}{6}z^3}{(1-z)^3}\right) + \overline{\left(\frac{\frac{1}{2}z^2+\frac{1}{6}z^3}{(1-z)^3}\right)}\end{align*} $$
which maps
![]() ${\mathbb D}$
onto
${\mathbb D}$
onto
![]() ${\mathbb C} \backslash (-\infty , -1/6]$
, and the function
${\mathbb C} \backslash (-\infty , -1/6]$
, and the function
 $$ \begin{align*}L(z) = H_1(z) + \overline{G_1(z)} = \left(\frac{z-\frac{1}{2}z^2}{(1-z)^2}\right) + \overline{\left(\frac{-\frac{1}{2}z^2}{(1-z)^2}\right)}\end{align*} $$
$$ \begin{align*}L(z) = H_1(z) + \overline{G_1(z)} = \left(\frac{z-\frac{1}{2}z^2}{(1-z)^2}\right) + \overline{\left(\frac{-\frac{1}{2}z^2}{(1-z)^2}\right)}\end{align*} $$
which maps
![]() ${\mathbb D}$
onto the half-plane
${\mathbb D}$
onto the half-plane
![]() ${\operatorname {Re}\,}\{w\}>-1/2$
. It is obvious that
${\operatorname {Re}\,}\{w\}>-1/2$
. It is obvious that
![]() $K \in C_H^0$
and
$K \in C_H^0$
and
![]() ${L \in K_H^0}$
. More details on univalent harmonic functions can be found in [Reference Clunie and Sheil-Small6, Reference Duren10].
${L \in K_H^0}$
. More details on univalent harmonic functions can be found in [Reference Clunie and Sheil-Small6, Reference Duren10].
A harmonic function f is said to be of class
![]() $h^p$
$h^p$
![]() $(0<p\le \infty )$
if
$(0<p\le \infty )$
if
![]() $\|f\|_p < \infty $
. Let us give an account of the problem considered in this paper. Every function
$\|f\|_p < \infty $
. Let us give an account of the problem considered in this paper. Every function
![]() $f=h+\overline {g} \in S_H$
admits the representation
$f=h+\overline {g} \in S_H$
admits the representation
 $$ \begin{align} h(z) = z+\sum_{n=2}^{\infty} a_n z^n \quad \text{and} \quad g(z)=\sum_{n=1}^{\infty} b_n z^n.\end{align} $$
$$ \begin{align} h(z) = z+\sum_{n=2}^{\infty} a_n z^n \quad \text{and} \quad g(z)=\sum_{n=1}^{\infty} b_n z^n.\end{align} $$
Let us define
![]() $\alpha =\sup _{f \in S_H} \vert a_2\vert $
. Then
$\alpha =\sup _{f \in S_H} \vert a_2\vert $
. Then
![]() $\alpha $
has crucial influence in the growth of functions in
$\alpha $
has crucial influence in the growth of functions in
![]() $S_H$
(see [Reference Sheil-Small21] for an exposition). Interest in the boundary behavior of functions
$S_H$
(see [Reference Sheil-Small21] for an exposition). Interest in the boundary behavior of functions
![]() $f \in S_H$
was initiated by Abu-Muhanna and Lyzzaik [Reference Abu-Muhanna and Lyzzaik1], who proved that
$f \in S_H$
was initiated by Abu-Muhanna and Lyzzaik [Reference Abu-Muhanna and Lyzzaik1], who proved that
![]() $f \in h^p$
for
$f \in h^p$
for
![]() $p<1/(2\alpha +2)^2$
. Bshouty and Hengartner [Reference Bshouty and Hengartner5] proposed to find the exact range of
$p<1/(2\alpha +2)^2$
. Bshouty and Hengartner [Reference Bshouty and Hengartner5] proposed to find the exact range of
![]() $p>0$
for which
$p>0$
for which
![]() $f \in h^p$
. In [Reference Nowak17], Nowak improved the range to
$f \in h^p$
. In [Reference Nowak17], Nowak improved the range to
![]() $p<1/\alpha ^2$
, and obtained the sharp results that
$p<1/\alpha ^2$
, and obtained the sharp results that
![]() $f \in h^p$
for
$f \in h^p$
for
![]() $p<1/2$
(resp.
$p<1/2$
(resp.
![]() $p<1/3$
) whenever f is a convex (resp. close-to-convex) harmonic function. These observations led her to conjecture that if
$p<1/3$
) whenever f is a convex (resp. close-to-convex) harmonic function. These observations led her to conjecture that if
![]() $f \in S_H$
, then
$f \in S_H$
, then
![]() $f \in h^p$
for
$f \in h^p$
for
![]() $p<1/\alpha $
. The conjecture seems challenging, and in the relatively recent development [Reference Ponnusamy, Qiao and Wang20], the authors verified it by confining interest to harmonic quasiconformal mappings.
$p<1/\alpha $
. The conjecture seems challenging, and in the relatively recent development [Reference Ponnusamy, Qiao and Wang20], the authors verified it by confining interest to harmonic quasiconformal mappings.
In this paper, we first give a relation between
![]() $M_p(r,f)$
and
$M_p(r,f)$
and
![]() $M_p(r,h')$
, which naturally allows us to check the boundedness of
$M_p(r,h')$
, which naturally allows us to check the boundedness of
![]() $\|f\|_p$
whenever
$\|f\|_p$
whenever
![]() $h'$
behaves “nicely.” As it turns out, this can be achieved by placing the simple restriction that
$h'$
behaves “nicely.” As it turns out, this can be achieved by placing the simple restriction that
![]() $h'$
takes no value infinitely often. An analytic function
$h'$
takes no value infinitely often. An analytic function
![]() $\varphi $
in
$\varphi $
in
![]() ${\mathbb D}$
has valency m if
${\mathbb D}$
has valency m if
![]() $\varphi $
takes no value more than m times. More generally, let for a function
$\varphi $
takes no value more than m times. More generally, let for a function
![]() $\varphi $
analytic in
$\varphi $
analytic in
![]() $\mathbb {D}$
,
$\mathbb {D}$
,
![]() $W(R)$
denote the area (regions covered multiply being counted multiply) of the image of
$W(R)$
denote the area (regions covered multiply being counted multiply) of the image of
![]() $\mathbb {D}$
under
$\mathbb {D}$
under
![]() $\varphi $
that lies in the disk
$\varphi $
that lies in the disk
![]() $|w|\le R$
. If
$|w|\le R$
. If
![]() $W(R)\le m\pi R^2$
for all
$W(R)\le m\pi R^2$
for all
![]() $R>0$
, where m is a positive number, then we say that
$R>0$
, where m is a positive number, then we say that
![]() $\varphi $
has mean valency m. This notion is due to Spencer, who proved the following inequality on the integral means of such functions.
$\varphi $
has mean valency m. This notion is due to Spencer, who proved the following inequality on the integral means of such functions.
Theorem A [Reference Spencer22]
If f has mean valency
![]() $m\ge 1$
,
$m\ge 1$
,
![]() $f(0)=0$
, and
$f(0)=0$
, and
![]() $p>0$
, then
$p>0$
, then
where
![]() $K=K(m,p)$
is independent of f.
$K=K(m,p)$
is independent of f.
This result was initially proved by Prawitz (see [Reference Pommerenke19, Theorem 5.1]) for univalent functions.
Over the years, extremal problems on the growth of univalent functions have been widely studied. Let
![]() $\mathcal {S}$
denote the class of univalent analytic functions f in
$\mathcal {S}$
denote the class of univalent analytic functions f in
![]() ${\mathbb D}$
with
${\mathbb D}$
with
![]() $f(0)=f'(0)-1=0$
. A seminal result in this direction is the following inequality of Baernstein.
$f(0)=f'(0)-1=0$
. A seminal result in this direction is the following inequality of Baernstein.
Theorem B [Reference Baernstein2]
If
![]() $f \in \mathcal {S}$
and
$f \in \mathcal {S}$
and
![]() $0<p<\infty $
, then
$0<p<\infty $
, then
where
![]() $k(z)=z/(1-z)^2$
is the Koebe function.
$k(z)=z/(1-z)^2$
is the Koebe function.
Baernstein’s theorem was extended to derivatives by Leung [Reference Leung15] and Brown [Reference Brown4] for certain subclasses of
![]() $\mathcal {S}$
. We refer to [Reference Baernstein3, Reference Girela12, Reference Girela13, Reference Nowak16] for more problems of this type. In [Reference Girela12], Girela obtained similar results for the functions
$\mathcal {S}$
. We refer to [Reference Baernstein3, Reference Girela12, Reference Girela13, Reference Nowak16] for more problems of this type. In [Reference Girela12], Girela obtained similar results for the functions
![]() $\log (f(z)/z)$
. These functions appear in the definition of logarithmic coefficients
$\log (f(z)/z)$
. These functions appear in the definition of logarithmic coefficients
![]() $\gamma _n$
of a function
$\gamma _n$
of a function
![]() $f \in \mathcal {S}$
:
$f \in \mathcal {S}$
:
 $$ \begin{align*}\log \frac{f(z)}{z} = 2 \sum_{n=1}^\infty \gamma_n z^n.\end{align*} $$
$$ \begin{align*}\log \frac{f(z)}{z} = 2 \sum_{n=1}^\infty \gamma_n z^n.\end{align*} $$
The logarithmic coefficients were instrumental in de Branges’ proof of the Bieberbach conjecture (see [Reference de Branges8]). Girela’s work readily led to the sharp inequality
 $$ \begin{align*}\sum_{n=1}^\infty \vert\gamma_n\vert^2 \le \frac{\pi^2}{6},\end{align*} $$
$$ \begin{align*}\sum_{n=1}^\infty \vert\gamma_n\vert^2 \le \frac{\pi^2}{6},\end{align*} $$
an important estimate earlier obtained by Duren and Leung [Reference Duren and Leung11]. Interestingly, Girela proved the following extremal result for close-to-convex functions.
Theorem C [Reference Girela12]
Let
![]() $f \in \mathcal {S}$
be close-to-convex, and let
$f \in \mathcal {S}$
be close-to-convex, and let
![]() $0<p\le 2$
. Then
$0<p\le 2$
. Then
where k is the Koebe function.
On the other hand, similar problems for harmonic functions remained unexplored until very recently, when the present authors initiated the study of Baernstein-type inequalities for harmonic functions.
Theorem D [Reference Das and Sairam Kaliraj7]
Let
![]() $0<p<\infty $
. If
$0<p<\infty $
. If
![]() $f=h+\overline {g} \in C_H^0$
, then
$f=h+\overline {g} \in C_H^0$
, then
For
![]() $f=h+\overline {g} \in K_H^0$
, we have
$f=h+\overline {g} \in K_H^0$
, we have
The functions
![]() $H, G$
and
$H, G$
and
![]() $H_1, G_1$
come from the harmonic functions K and L, respectively.
$H_1, G_1$
come from the harmonic functions K and L, respectively.
To explore the logarithmic coefficients in the setting of a harmonic mapping
![]() ${f=h+\overline {g}}$
, it is not feasible to consider
${f=h+\overline {g}}$
, it is not feasible to consider
![]() $f(z)/z$
, as this function need not be harmonic, neither is the logarithm of a harmonic function defined in the literature. One cannot consider the functions
$f(z)/z$
, as this function need not be harmonic, neither is the logarithm of a harmonic function defined in the literature. One cannot consider the functions
![]() $(h(z)+cg(z))/z$
(c constant) either, since
$(h(z)+cg(z))/z$
(c constant) either, since
![]() $h(z)+cg(z)$
may have zeros at points other than the origin. Therefore, proceeding along the line of Theorem C, the functions
$h(z)+cg(z)$
may have zeros at points other than the origin. Therefore, proceeding along the line of Theorem C, the functions
![]() $\log (h'+cg')$
seem to be the most natural choice.
$\log (h'+cg')$
seem to be the most natural choice.
Thus, we conclude the paper with a harmonic analogue of Girela’s result: we prove that Theorem C remains true for the functions
![]() $\log (h'+cg')$
, whenever
$\log (h'+cg')$
, whenever
![]() $f=h+\overline {g}$
is a close-to-convex harmonic function and c is a constant. This takes forward the authors’ earlier line of work in [Reference Das and Sairam Kaliraj7].
$f=h+\overline {g}$
is a close-to-convex harmonic function and c is a constant. This takes forward the authors’ earlier line of work in [Reference Das and Sairam Kaliraj7].
2 Main theorems and auxiliary results
As discussed, we start with the following simple but useful lemma, the proof of which is just a slight modification of standard techniques.
Lemma 1 Let
![]() $0 < p \le 1$
. Suppose
$0 < p \le 1$
. Suppose
![]() $f=h+\overline {g}$
is a locally univalent, sense-preserving harmonic function in
$f=h+\overline {g}$
is a locally univalent, sense-preserving harmonic function in
![]() ${\mathbb D}$
with
${\mathbb D}$
with
![]() $f(0)=0$
. Then
$f(0)=0$
. Then
where C is a constant independent of f.
This, together with Theorem D, immediately give integral mean estimates for convex and close-to-convex harmonic functions.
Corollary 1 Let
![]() $0 < p \le 1$
, then we have the inequalities
$0 < p \le 1$
, then we have the inequalities
 $$ \begin{align*} M_p(r,f) & \le C \int_{0}^{r} (r-s)^{p-1} M_p^p(s,H') ds, \, \mbox{ whenever } f \in C_H^0,\\ M_p(r,f) & \le C \int_{0}^{r} (r-s)^{p-1} M_p^p(s,H_1') ds, \, \mbox{ whenever } f \in K_H^0. \end{align*} $$
$$ \begin{align*} M_p(r,f) & \le C \int_{0}^{r} (r-s)^{p-1} M_p^p(s,H') ds, \, \mbox{ whenever } f \in C_H^0,\\ M_p(r,f) & \le C \int_{0}^{r} (r-s)^{p-1} M_p^p(s,H_1') ds, \, \mbox{ whenever } f \in K_H^0. \end{align*} $$
Remark 1 The estimates for
![]() $p> 1$
for these classes are already obtained in [Reference Das and Sairam Kaliraj7]. It is pertinent to mention that an elementary upper bound can be easily given in the case of convex harmonic functions. If
$p> 1$
for these classes are already obtained in [Reference Das and Sairam Kaliraj7]. It is pertinent to mention that an elementary upper bound can be easily given in the case of convex harmonic functions. If
![]() $f=h+\overline {g}$
is convex, it is well known [Reference Clunie and Sheil-Small6, Theorem 5.7] that h is close-to-convex, and
$f=h+\overline {g}$
is convex, it is well known [Reference Clunie and Sheil-Small6, Theorem 5.7] that h is close-to-convex, and
![]() $|g(z)|\le |h(z)|$
,
$|g(z)|\le |h(z)|$
,
![]() $z \in {\mathbb D}$
. Therefore, from [Reference Pommerenke19, Theorem 5.1], we have
$z \in {\mathbb D}$
. Therefore, from [Reference Pommerenke19, Theorem 5.1], we have
The last integral is the incomplete beta function
![]() $B(r;p,1-2p)$
.
$B(r;p,1-2p)$
.
As a consequence of Lemma 1, we verify Nowak’s conjecture for certain functions in
![]() $S_H$
. Indeed, the result is true for a more general class of functions. Let us recall that a family
$S_H$
. Indeed, the result is true for a more general class of functions. Let us recall that a family
![]() $\mathcal {L}$
of harmonic functions in
$\mathcal {L}$
of harmonic functions in
![]() ${\mathbb D}$
is said to be linear invariant (see [Reference Sheil-Small21]) if for every
${\mathbb D}$
is said to be linear invariant (see [Reference Sheil-Small21]) if for every
![]() $f=h+\overline {g}\in \mathcal {L}$
, the functions
$f=h+\overline {g}\in \mathcal {L}$
, the functions
 $$ \begin{align*} T_\varphi(f(z)) =\frac{f(\varphi(z))-f(\varphi(0))}{\varphi'(0)h'(\varphi(0))}, \quad \varphi \in \operatorname{Aut}({\mathbb D}), \end{align*} $$
$$ \begin{align*} T_\varphi(f(z)) =\frac{f(\varphi(z))-f(\varphi(0))}{\varphi'(0)h'(\varphi(0))}, \quad \varphi \in \operatorname{Aut}({\mathbb D}), \end{align*} $$
belong to
![]() $\mathcal {L}$
, where
$\mathcal {L}$
, where
![]() $\operatorname {Aut}({\mathbb D})$
denotes the set of analytic automorphisms of
$\operatorname {Aut}({\mathbb D})$
denotes the set of analytic automorphisms of
![]() ${\mathbb D}$
. Our result does not require univalence, and holds for any linear invariant class
${\mathbb D}$
. Our result does not require univalence, and holds for any linear invariant class
![]() $\mathcal {H}$
of locally univalent and sense-preserving harmonic functions (with usual normalizations), for which
$\mathcal {H}$
of locally univalent and sense-preserving harmonic functions (with usual normalizations), for which
![]() $\alpha (\mathcal {H})=\sup _{f \in \mathcal {H}} \vert a_2\vert $
is finite. For the remainder of this paper, we preserve the notation
$\alpha (\mathcal {H})=\sup _{f \in \mathcal {H}} \vert a_2\vert $
is finite. For the remainder of this paper, we preserve the notation
![]() $\mathcal {H}$
to mean any such class of locally univalent harmonic functions.
$\mathcal {H}$
to mean any such class of locally univalent harmonic functions.
Theorem 1 Let
![]() $f=h+\overline {g} \in S_H$
be such that
$f=h+\overline {g} \in S_H$
be such that
![]() $h'$
has finite mean valency. Then
$h'$
has finite mean valency. Then
![]() $f \in h^p$
for
$f \in h^p$
for
![]() $p<1/\alpha $
. If
$p<1/\alpha $
. If
![]() $f \in \mathcal {H}$
and
$f \in \mathcal {H}$
and
![]() $h'$
has finite mean valency, then
$h'$
has finite mean valency, then
![]() $f \in h^p$
for
$f \in h^p$
for
![]() $p<1/\alpha (\mathcal {H})$
.
$p<1/\alpha (\mathcal {H})$
.
Lemma 1 also leads us to the following coefficient bound for these functions.
Theorem 2 Suppose
![]() $f=h+\overline {g} \in S_H$
has series representation (1.1), and
$f=h+\overline {g} \in S_H$
has series representation (1.1), and
![]() $h'$
has finite mean valency. Then
$h'$
has finite mean valency. Then
![]() $\vert a_n \vert $
and
$\vert a_n \vert $
and
![]() $\vert b_n \vert $
are
$\vert b_n \vert $
are
![]() $O(n^{\alpha -1})$
,
$O(n^{\alpha -1})$
,
![]() $n=2,3,4,\ldots $
. For
$n=2,3,4,\ldots $
. For
![]() $f \in \mathcal {H}$
with
$f \in \mathcal {H}$
with
![]() $h'$
having finite mean valency,
$h'$
having finite mean valency,
![]() $\vert a_n \vert $
and
$\vert a_n \vert $
and
![]() $\vert b_n \vert $
are
$\vert b_n \vert $
are
![]() $O(n^{\alpha (\mathcal {H})-1})$
.
$O(n^{\alpha (\mathcal {H})-1})$
.
Remark 2 This coefficient estimate for
![]() $f \in S_H$
is in a sense best possible. The conjectured value of
$f \in S_H$
is in a sense best possible. The conjectured value of
![]() $\alpha $
is
$\alpha $
is
![]() $3$
. Given this, Theorem 2 asserts that
$3$
. Given this, Theorem 2 asserts that
![]() $\vert a_n \vert $
and
$\vert a_n \vert $
and
![]() $\vert b_n \vert $
are
$\vert b_n \vert $
are
![]() $O(n^2)$
, which is the same order as in the harmonic analogue of the Bieberbach conjecture [Reference Clunie and Sheil-Small6].
$O(n^2)$
, which is the same order as in the harmonic analogue of the Bieberbach conjecture [Reference Clunie and Sheil-Small6].
The problem, even without the assumption of finite mean valency, can be explored in another direction to produce a very interesting result. Since
![]() $S_H$
is known to be linear invariant, for
$S_H$
is known to be linear invariant, for
![]() $f=h+\overline {g} \in S_H$
and any
$f=h+\overline {g} \in S_H$
and any
![]() $\zeta \in {\mathbb D}$
, the function
$\zeta \in {\mathbb D}$
, the function
 $$ \begin{align*}T(z)=\frac{f\left(\frac{z+\zeta}{1+\overline{\zeta}z}\right)-f(\zeta)}{(1-|\zeta|^2)h'(\zeta)}=A(z)+\overline{B(z)} \quad (z \in {\mathbb D})\end{align*} $$
$$ \begin{align*}T(z)=\frac{f\left(\frac{z+\zeta}{1+\overline{\zeta}z}\right)-f(\zeta)}{(1-|\zeta|^2)h'(\zeta)}=A(z)+\overline{B(z)} \quad (z \in {\mathbb D})\end{align*} $$
again lies in
![]() $S_H$
. Let us write
$S_H$
. Let us write
A customary computation gives
 $$ \begin{align*}a_2(\zeta)=\frac{1}{2}\left\{(1-|\zeta|^2)\frac{h"(\zeta)}{h'(\zeta)}-2\overline{\zeta}\right\}.\end{align*} $$
$$ \begin{align*}a_2(\zeta)=\frac{1}{2}\left\{(1-|\zeta|^2)\frac{h"(\zeta)}{h'(\zeta)}-2\overline{\zeta}\right\}.\end{align*} $$
Since
![]() $|a_2(\zeta )| \le \alpha $
, we find that
$|a_2(\zeta )| \le \alpha $
, we find that
 $$ \begin{align*}\left|\frac{h"(\zeta)}{h'(\zeta)}\right| \le \frac{C}{1-|\zeta|} \quad (\zeta \in {\mathbb D}),\end{align*} $$
$$ \begin{align*}\left|\frac{h"(\zeta)}{h'(\zeta)}\right| \le \frac{C}{1-|\zeta|} \quad (\zeta \in {\mathbb D}),\end{align*} $$
for some positive constant C. However, this is the extreme bound on
![]() $h"/h'$
that a function
$h"/h'$
that a function
![]() $f=h+\overline {g} \in S_H$
can possess. In general, it is reasonable to expect a large subclass of
$f=h+\overline {g} \in S_H$
can possess. In general, it is reasonable to expect a large subclass of
![]() $S_H$
to have slightly restricted growth, or more precisely, to exhibit the bound
$S_H$
to have slightly restricted growth, or more precisely, to exhibit the bound
 $$ \begin{align*}\left|\frac{h"(\zeta)}{h'(\zeta)}\right| \le \frac{C}{(1-|\zeta|)^\beta} \quad (0 \le \beta <1).\end{align*} $$
$$ \begin{align*}\left|\frac{h"(\zeta)}{h'(\zeta)}\right| \le \frac{C}{(1-|\zeta|)^\beta} \quad (0 \le \beta <1).\end{align*} $$
The expression
![]() $h"/h'$
is of special interest in the theory of univalent functions. For example, it appears in the definition of the Schwarzian derivative, as well as in characterization results for certain geometric subclasses (e.g., convex and close-to-convex). The growth condition on
$h"/h'$
is of special interest in the theory of univalent functions. For example, it appears in the definition of the Schwarzian derivative, as well as in characterization results for certain geometric subclasses (e.g., convex and close-to-convex). The growth condition on
![]() $h"/h'$
leads us to the following result on the membership of univalent and locally univalent harmonic functions in the Hardy space.
$h"/h'$
leads us to the following result on the membership of univalent and locally univalent harmonic functions in the Hardy space.
Theorem 3 Let
![]() $f=h+\overline {g} \in S_H$
be such that
$f=h+\overline {g} \in S_H$
be such that
 $$ \begin{align} \left|\frac{h"(z)}{h'(z)}\right| \le \frac{C}{(1-|z|)^\beta},\end{align} $$
$$ \begin{align} \left|\frac{h"(z)}{h'(z)}\right| \le \frac{C}{(1-|z|)^\beta},\end{align} $$
for some
![]() $\beta $
with
$\beta $
with
![]() $0 \le \beta <1$
. Then
$0 \le \beta <1$
. Then
![]() $f \in h^p$
for
$f \in h^p$
for
![]() $p<2(1-\beta )/\alpha $
. Analogously, if
$p<2(1-\beta )/\alpha $
. Analogously, if
![]() $f=h+\overline {g} \in \mathcal {H}$
satisfies the growth estimate (2.1), then
$f=h+\overline {g} \in \mathcal {H}$
satisfies the growth estimate (2.1), then
![]() $f \in h^p$
for
$f \in h^p$
for
![]() $p<2(1-\beta )/\alpha (\mathcal {H})$
.
$p<2(1-\beta )/\alpha (\mathcal {H})$
.
Finally, we prove Girela’s result (Theorem C) in the setting of harmonic functions.
Theorem 4 Suppose
![]() $0 < p \le 2$
and
$0 < p \le 2$
and
![]() $f =h+\overline {g}\in C_H^0$
. Then, for any constant
$f =h+\overline {g}\in C_H^0$
. Then, for any constant
![]() $c \in {\mathbb D}$
, we have
$c \in {\mathbb D}$
, we have
The bound is sharp.
Like logarithmic coefficients in the case of analytic functions, it is interesting to study the power series coefficients of
![]() $\log (h'(z)+cg'(z))$
. Suppose
$\log (h'(z)+cg'(z))$
. Suppose
![]() $\log (h'(z)+cg'(z)) = \sum _{n=1}^\infty \lambda _n z^n$
. Then Theorem 4 has the following implication.
$\log (h'(z)+cg'(z)) = \sum _{n=1}^\infty \lambda _n z^n$
. Then Theorem 4 has the following implication.
Corollary 2 For
![]() $f=h+\overline {g} \in C_H^0$
, we have the sharp inequality
$f=h+\overline {g} \in C_H^0$
, we have the sharp inequality
 $$ \begin{align*}\sum_{n=1}^\infty \vert\lambda_n\vert^2 \le \frac{14 \pi^2}{3}.\end{align*} $$
$$ \begin{align*}\sum_{n=1}^\infty \vert\lambda_n\vert^2 \le \frac{14 \pi^2}{3}.\end{align*} $$
3 Preliminaries
Definition 1 [Reference Baernstein2]
For a real-valued function
![]() $g(x)$
integrable over
$g(x)$
integrable over
![]() $[-\pi ,\pi ]$
, the Baernstein star-function is defined as
$[-\pi ,\pi ]$
, the Baernstein star-function is defined as
where
![]() $\vert E\vert $
is the Lebesgue measure of the set
$\vert E\vert $
is the Lebesgue measure of the set
![]() $E \subseteq [-\pi , \pi ]$
.
$E \subseteq [-\pi , \pi ]$
.
The following properties of the star-function are due to Leung [Reference Leung15].
Lemma A For
![]() $g,h \in L^1[-\pi , \pi ]$
,
$g,h \in L^1[-\pi , \pi ]$
,
Equality holds if g, h are both symmetric in
![]() $[-\pi , \pi ]$
and nonincreasing in
$[-\pi , \pi ]$
and nonincreasing in
![]() $[0, \pi ]$
.
$[0, \pi ]$
.
Lemma B If g, h are subharmonic functions in
![]() ${\mathbb D}$
and g is subordinate to h, then for each r in
${\mathbb D}$
and g is subordinate to h, then for each r in
![]() $(0,1)$
,
$(0,1)$
,
Lemma C If
![]() $p(z)=e^{i\beta }+p_1z+\cdots $
is analytic and of positive real part in
$p(z)=e^{i\beta }+p_1z+\cdots $
is analytic and of positive real part in
![]() ${\mathbb D}$
, then
${\mathbb D}$
, then
 $$ \begin{align*}\left(\log\vert p(r{e^{i\theta}})\vert\right)^* \le \left(\log\left\vert\frac{1+r{e^{i\theta}}}{1-r{e^{i\theta}}}\right\vert\right)^*, \quad 0 \le \theta \le \pi.\end{align*} $$
$$ \begin{align*}\left(\log\vert p(r{e^{i\theta}})\vert\right)^* \le \left(\log\left\vert\frac{1+r{e^{i\theta}}}{1-r{e^{i\theta}}}\right\vert\right)^*, \quad 0 \le \theta \le \pi.\end{align*} $$
An important feature in the proof of Lemma C is that a rotation factor does not affect the star-function. This observation will be suitably deployed in our work.
Definition 2 [Reference Girela12]
A domain D in
![]() ${\mathbb C}$
is said to be Steiner symmetric if its intersection with each vertical line is either empty or a segment placed symmetrically with respect to the real axis.
${\mathbb C}$
is said to be Steiner symmetric if its intersection with each vertical line is either empty or a segment placed symmetrically with respect to the real axis.
The next result by Girela is crucial in the proof of Theorem 4.
Lemma D [Reference Girela12]
Let F and
![]() $\mathcal {F}$
be analytic in
$\mathcal {F}$
be analytic in
![]() $\overline {{\mathbb D}}$
and satisfy:
$\overline {{\mathbb D}}$
and satisfy:
-
(i)
 $F(0)=\mathcal {F}(0)=0$
,
$F(0)=\mathcal {F}(0)=0$
, -
(ii)
 $({\operatorname {Re}\,} F)^* \le ({\operatorname {Re}\,} \mathcal {F})^*$
in
$({\operatorname {Re}\,} F)^* \le ({\operatorname {Re}\,} \mathcal {F})^*$
in
 ${\mathbb D}^+=\{z \in {\mathbb D} : \; \operatorname {Im} z>0\}$
,
${\mathbb D}^+=\{z \in {\mathbb D} : \; \operatorname {Im} z>0\}$
, -
(iii)
 $\displaystyle \min _{z\in {\mathbb D}} {\operatorname {Re}\,} \mathcal {F}(z) \le \min _{z\in {\mathbb D}} {\operatorname {Re}\,} F(z) \le \max _{z\in {\mathbb D}} {\operatorname {Re}\,} F(z) \le \max _{z\in {\mathbb D}} {\operatorname {Re}\,} \mathcal {F}(z)$
,
$\displaystyle \min _{z\in {\mathbb D}} {\operatorname {Re}\,} \mathcal {F}(z) \le \min _{z\in {\mathbb D}} {\operatorname {Re}\,} F(z) \le \max _{z\in {\mathbb D}} {\operatorname {Re}\,} F(z) \le \max _{z\in {\mathbb D}} {\operatorname {Re}\,} \mathcal {F}(z)$
, -
(iv)
 $\mathcal {F}$
is univalent and
$\mathcal {F}$
is univalent and
 $\mathcal {F}({\mathbb D})$
is a Steiner symmetric domain.
$\mathcal {F}({\mathbb D})$
is a Steiner symmetric domain.
Then, for
![]() $0<p\le 2$
,
$0<p\le 2$
,
4 Proofs
4.1 Proof of Lemma 1
In what follows, C will denote a positive constant that is not necessarily the same at each occurrence. Let
![]() $\vert \nabla f\vert =(\vert h'\vert ^2+\vert g'\vert ^2)^{1/2}$
. For
$\vert \nabla f\vert =(\vert h'\vert ^2+\vert g'\vert ^2)^{1/2}$
. For
![]() $0 \le r_1<r_2<1$
, we have
$0 \le r_1<r_2<1$
, we have
 $$ \begin{align*} \vert f(r_2 {e^{i\theta}})-f(r_1 {e^{i\theta}})\vert & =\left\vert\int_{r_1}^{r_2} \frac{d}{d t} f(t {e^{i\theta}}) dt\right\vert\\ & \le \int_{r_1}^{r_2}\left\vert{e^{i\theta}} h'(t{e^{i\theta}}) + \overline{{e^{i\theta}} g'(t{e^{i\theta}})}\right\vert dt\\ & \le \sqrt{2} \int_{r_1}^{r_2} \left(\vert h'(t {e^{i\theta}})\vert^2 + \vert g'(t{e^{i\theta}})\vert^2\right)^{1/2} dt \\ & = \sqrt{2} \int_{r_1}^{r_2} \vert\nabla f(t {e^{i\theta}})\vert dt\\ & \le \sqrt{2} \left(r_2-r_1\right) \sup _{r_1 \le t \le r_2}\vert\nabla f(t {e^{i\theta}})\vert. \end{align*} $$
$$ \begin{align*} \vert f(r_2 {e^{i\theta}})-f(r_1 {e^{i\theta}})\vert & =\left\vert\int_{r_1}^{r_2} \frac{d}{d t} f(t {e^{i\theta}}) dt\right\vert\\ & \le \int_{r_1}^{r_2}\left\vert{e^{i\theta}} h'(t{e^{i\theta}}) + \overline{{e^{i\theta}} g'(t{e^{i\theta}})}\right\vert dt\\ & \le \sqrt{2} \int_{r_1}^{r_2} \left(\vert h'(t {e^{i\theta}})\vert^2 + \vert g'(t{e^{i\theta}})\vert^2\right)^{1/2} dt \\ & = \sqrt{2} \int_{r_1}^{r_2} \vert\nabla f(t {e^{i\theta}})\vert dt\\ & \le \sqrt{2} \left(r_2-r_1\right) \sup _{r_1 \le t \le r_2}\vert\nabla f(t {e^{i\theta}})\vert. \end{align*} $$
Since f is sense-preserving, i.e.,
![]() $|g'(z)| < |h'(z)|$
for every
$|g'(z)| < |h'(z)|$
for every
![]() $z \in {\mathbb D}$
, we find that
$z \in {\mathbb D}$
, we find that
Therefore,
 $$ \begin{align*} M_p^p(r_2,f)-M_p^p(r_1,f) & \le \frac{1}{2\pi} \int_{0}^{2\pi} \vert f(r_2 {e^{i\theta}})-f(r_1 {e^{i\theta}})\vert^p d\theta\\ & \le 2^{3p / 2}\left(r_2-r_1\right)^p \frac{1}{2\pi} \int_{0}^{2\pi} \left(\sup _{r_1 \le t \le r_2}\vert h'(t {e^{i\theta}})\vert\right)^p d\theta. \end{align*} $$
$$ \begin{align*} M_p^p(r_2,f)-M_p^p(r_1,f) & \le \frac{1}{2\pi} \int_{0}^{2\pi} \vert f(r_2 {e^{i\theta}})-f(r_1 {e^{i\theta}})\vert^p d\theta\\ & \le 2^{3p / 2}\left(r_2-r_1\right)^p \frac{1}{2\pi} \int_{0}^{2\pi} \left(\sup _{r_1 \le t \le r_2}\vert h'(t {e^{i\theta}})\vert\right)^p d\theta. \end{align*} $$
An appeal to the Hardy–Littlewood maximal theorem gives
 $$ \begin{align*}\frac{1}{2\pi} \int_{0}^{2\pi} \left(\sup _{r_1 \le t \le r_2}\vert h'(t {e^{i\theta}})\vert\right)^p d\theta \le C M_p^p(r_2,h'),\end{align*} $$
$$ \begin{align*}\frac{1}{2\pi} \int_{0}^{2\pi} \left(\sup _{r_1 \le t \le r_2}\vert h'(t {e^{i\theta}})\vert\right)^p d\theta \le C M_p^p(r_2,h'),\end{align*} $$
so that
Let
![]() $0<r<1$
be arbitrary, and let
$0<r<1$
be arbitrary, and let
![]() $r_n=r(1-2^{-n})$
,
$r_n=r(1-2^{-n})$
,
![]() $n=0,1,2,\ldots $
. Clearly,
$n=0,1,2,\ldots $
. Clearly,
![]() $M_p(0,f)=0$
as
$M_p(0,f)=0$
as
![]() $f(0)=0$
. Using (4.1), we find that
$f(0)=0$
. Using (4.1), we find that
 $$ \begin{align*} M_p^p(r_{n+1},f) & =\sum_{k=1}^{n+1}\left[M_p^p(r_k,f)-M_p^p(r_{k-1},f)\right] \\ & \le C \sum_{k=1}^{n+1} (r_{k}-r_{k-1})^p M_p^p(r_k,h')\\ & = C \sum_{k=1}^{n+1} (r_{k}-r_{k-1}) (r-r_k)^{p-1} M_p^p(r_k,h'), \end{align*} $$
$$ \begin{align*} M_p^p(r_{n+1},f) & =\sum_{k=1}^{n+1}\left[M_p^p(r_k,f)-M_p^p(r_{k-1},f)\right] \\ & \le C \sum_{k=1}^{n+1} (r_{k}-r_{k-1})^p M_p^p(r_k,h')\\ & = C \sum_{k=1}^{n+1} (r_{k}-r_{k-1}) (r-r_k)^{p-1} M_p^p(r_k,h'), \end{align*} $$
since
![]() $r_k-r_{k-1}=2^{-k}r=r-r_k$
. We now let
$r_k-r_{k-1}=2^{-k}r=r-r_k$
. We now let
![]() $n \to \infty $
and obtain
$n \to \infty $
and obtain
by means of Riemann integration.
4.2 Proof of Theorem 1
Let
![]() $1/(\alpha +1)<p \le 1$
and
$1/(\alpha +1)<p \le 1$
and
![]() $f \in S_H$
. We may choose
$f \in S_H$
. We may choose
![]() $r_n=1-2^{-n}$
in the proof of Lemma 1 to obtain
$r_n=1-2^{-n}$
in the proof of Lemma 1 to obtain
whenever the integral is finite. We break the integral in two parts, to separately deal with possible complications around
![]() $0$
and
$0$
and
![]() $1$
. For example, let us write
$1$
. For example, let us write
 $$ \begin{align} \| f\|_p^p \le C \left[\int_{0}^{1/4} (1-s)^{p-1} M_p^p(s,h') ds + \int_{1/4}^{1} (1-s)^{p-1} M_p^p(s,h') ds\right]. \end{align} $$
$$ \begin{align} \| f\|_p^p \le C \left[\int_{0}^{1/4} (1-s)^{p-1} M_p^p(s,h') ds + \int_{1/4}^{1} (1-s)^{p-1} M_p^p(s,h') ds\right]. \end{align} $$
Throughout our computations, the constants will be denoted by C, K, etc., and they need not be the same at each occurrence. We do this for convenience, as constants do not affect our purpose.
We appeal to Theorem A for an estimate of
![]() $M_p^p(s,h')$
. Since
$M_p^p(s,h')$
. Since
![]() $h'$
is finitely mean valent, so is
$h'$
is finitely mean valent, so is
![]() $zh'$
. Therefore, we have
$zh'$
. Therefore, we have
It is known (see [Reference Duren10, p. 98]) that
 $$ \begin{align} M_\infty(r,h') \le \frac{(1+r)^{\alpha-1}}{(1-r)^{\alpha+1}}.\end{align} $$
$$ \begin{align} M_\infty(r,h') \le \frac{(1+r)^{\alpha-1}}{(1-r)^{\alpha+1}}.\end{align} $$
This, together with (4.4), implies
 $$ \begin{align} M_p^p(s,h') \le \frac{K}{s^p} \int_{0}^s \frac{r^{p-1}}{(1-r)^{(\alpha+1)p}} dr.\end{align} $$
$$ \begin{align} M_p^p(s,h') \le \frac{K}{s^p} \int_{0}^s \frac{r^{p-1}}{(1-r)^{(\alpha+1)p}} dr.\end{align} $$
For
![]() $s \le 1/4$
,
$s \le 1/4$
,
 $$ \begin{align*}M_p^p(s,h') \le \frac{K}{s^p} \int_{0}^{1/4} \frac{r^{p-1}}{(1-r)^{(\alpha+1)p}} dr \le \frac{K_1}{s^p} \int_{0}^{1/4} r^{p-1} dr \le \frac{K_2}{s^p} \quad (\text{as } p \le 1).\end{align*} $$
$$ \begin{align*}M_p^p(s,h') \le \frac{K}{s^p} \int_{0}^{1/4} \frac{r^{p-1}}{(1-r)^{(\alpha+1)p}} dr \le \frac{K_1}{s^p} \int_{0}^{1/4} r^{p-1} dr \le \frac{K_2}{s^p} \quad (\text{as } p \le 1).\end{align*} $$
For
![]() $s> 1/4$
,
$s> 1/4$
,
 $$ \begin{align*} M_p^p(s,h') & \le \frac{K}{s^p} \int_{0}^{1/4} \frac{r^{p-1}}{(1-r)^{(\alpha+1)p}} dr + \frac{K}{s^p} \int_{1/4}^{s} \frac{r^{p-1}}{(1-r)^{(\alpha+1)p}} dr\\ & \le \frac{K_2}{s^p} + K_3 \int_{1/4}^{s} \frac{dr}{(1-r)^{(\alpha+1)p}} \\& = \frac{K_2}{s^p} + \frac{K_3}{(\alpha+1)p-1} \left[\frac{1}{(1-s)^{(\alpha+1)p-1}} - K_4\right] \quad (\text{as } p>1/(\alpha+1))\\ & \le \frac{K_2}{s^p} + \frac{K_5}{(1-s)^{(\alpha+1)p-1}}. \end{align*} $$
$$ \begin{align*} M_p^p(s,h') & \le \frac{K}{s^p} \int_{0}^{1/4} \frac{r^{p-1}}{(1-r)^{(\alpha+1)p}} dr + \frac{K}{s^p} \int_{1/4}^{s} \frac{r^{p-1}}{(1-r)^{(\alpha+1)p}} dr\\ & \le \frac{K_2}{s^p} + K_3 \int_{1/4}^{s} \frac{dr}{(1-r)^{(\alpha+1)p}} \\& = \frac{K_2}{s^p} + \frac{K_3}{(\alpha+1)p-1} \left[\frac{1}{(1-s)^{(\alpha+1)p-1}} - K_4\right] \quad (\text{as } p>1/(\alpha+1))\\ & \le \frac{K_2}{s^p} + \frac{K_5}{(1-s)^{(\alpha+1)p-1}}. \end{align*} $$
Substituting these bounds in (4.3), we see that
 $$ \begin{align*} \| f\|_p^p & \le C_1 \int_{0}^{1/4} s^{-p} (1-s)^{p-1} ds + C_1 \int_{1/4}^{1} s^{-p} (1-s)^{p-1} ds + C_2 \int_{1/4}^{1} \frac{ds}{(1-s)^{p\alpha}}\\ & = C_1 \int_{0}^{1} s^{-p} (1-s)^{p-1} ds + C_2 \int_{1/4}^{1} \frac{ds}{(1-s)^{p\alpha}}. \end{align*} $$
$$ \begin{align*} \| f\|_p^p & \le C_1 \int_{0}^{1/4} s^{-p} (1-s)^{p-1} ds + C_1 \int_{1/4}^{1} s^{-p} (1-s)^{p-1} ds + C_2 \int_{1/4}^{1} \frac{ds}{(1-s)^{p\alpha}}\\ & = C_1 \int_{0}^{1} s^{-p} (1-s)^{p-1} ds + C_2 \int_{1/4}^{1} \frac{ds}{(1-s)^{p\alpha}}. \end{align*} $$
The first integral is the beta function
![]() $B(1-p,p)$
and converges for every
$B(1-p,p)$
and converges for every
![]() $p\in (0,1)$
. The second integral is finite for
$p\in (0,1)$
. The second integral is finite for
![]() $p<1/\alpha $
. Therefore,
$p<1/\alpha $
. Therefore,
![]() $f \in h^p$
for
$f \in h^p$
for
![]() $p < 1/\alpha $
.
$p < 1/\alpha $
.
To prove the result for
![]() $f \in \mathcal {H}$
, we just need to establish the bound
$f \in \mathcal {H}$
, we just need to establish the bound
 $$ \begin{align*}M_\infty(r,h') \le \frac{(1+r)^{\alpha(\mathcal{H})-1}}{(1-r)^{\alpha(\mathcal{H})+1}}.\end{align*} $$
$$ \begin{align*}M_\infty(r,h') \le \frac{(1+r)^{\alpha(\mathcal{H})-1}}{(1-r)^{\alpha(\mathcal{H})+1}}.\end{align*} $$
The argument presented here is well known (see, for example, [Reference Duren10, p. 98]), and will be useful in the later results. Since
![]() $\mathcal {H}$
is linear invariant, for any
$\mathcal {H}$
is linear invariant, for any
![]() $\zeta \in {\mathbb D}$
, the function
$\zeta \in {\mathbb D}$
, the function
 $$ \begin{align*}T(z)=\frac{f\left(\frac{z+\zeta}{1+\overline{\zeta}z}\right)-f(\zeta)}{(1-|\zeta|^2)h'(\zeta)}=A(z)+\overline{B(z)} \quad (z \in {\mathbb D})\end{align*} $$
$$ \begin{align*}T(z)=\frac{f\left(\frac{z+\zeta}{1+\overline{\zeta}z}\right)-f(\zeta)}{(1-|\zeta|^2)h'(\zeta)}=A(z)+\overline{B(z)} \quad (z \in {\mathbb D})\end{align*} $$
is in
![]() $\mathcal {H}$
. We write
$\mathcal {H}$
. We write
so that
 $$ \begin{align*}a_2(\zeta)=\frac{1}{2}\left\{(1-|\zeta|^2)\frac{h"(\zeta)}{h'(\zeta)}-2\overline{\zeta}\right\}.\end{align*} $$
$$ \begin{align*}a_2(\zeta)=\frac{1}{2}\left\{(1-|\zeta|^2)\frac{h"(\zeta)}{h'(\zeta)}-2\overline{\zeta}\right\}.\end{align*} $$
Since
![]() $|a_2(\zeta )| \le \alpha (\mathcal {H})$
, we find that
$|a_2(\zeta )| \le \alpha (\mathcal {H})$
, we find that
 $$ \begin{align*}\frac{2r^2-2r\alpha(\mathcal{H})}{1-r^2} \le {\operatorname{Re}\,}\left\{\frac{zh"(z)}{h'(z)}\right\} \le \frac{2r^2+2r\alpha(\mathcal{H})}{1-r^2} \quad (|z|=r),\end{align*} $$
$$ \begin{align*}\frac{2r^2-2r\alpha(\mathcal{H})}{1-r^2} \le {\operatorname{Re}\,}\left\{\frac{zh"(z)}{h'(z)}\right\} \le \frac{2r^2+2r\alpha(\mathcal{H})}{1-r^2} \quad (|z|=r),\end{align*} $$
which is equivalent to
Now we integrate from
![]() $0$
to r to reach the estimate
$0$
to r to reach the estimate
 $$ \begin{align} \frac{(1-r)^{\alpha(\mathcal{H})-1}}{(1+r)^{\alpha(\mathcal{H})+1}} \le |h'(z)| \le \frac{(1+r)^{\alpha(\mathcal{H})-1}}{(1-r)^{\alpha(\mathcal{H})+1}}.\end{align} $$
$$ \begin{align} \frac{(1-r)^{\alpha(\mathcal{H})-1}}{(1+r)^{\alpha(\mathcal{H})+1}} \le |h'(z)| \le \frac{(1+r)^{\alpha(\mathcal{H})-1}}{(1-r)^{\alpha(\mathcal{H})+1}}.\end{align} $$
The rest of the proof follows through an identical argument, and the details are omitted.
4.3 Proof of Theorem 2
We see that
 $$ \begin{align*}(n+1) \vert a_{n+1}\vert= \left\vert\frac{1}{2\pi i} \int_{\vert z\vert=r}\frac{h'(z)}{z^{n+1}} dz \right\vert \le r^{-n} M_1(r,h').\end{align*} $$
$$ \begin{align*}(n+1) \vert a_{n+1}\vert= \left\vert\frac{1}{2\pi i} \int_{\vert z\vert=r}\frac{h'(z)}{z^{n+1}} dz \right\vert \le r^{-n} M_1(r,h').\end{align*} $$
We see from (4.6), for
![]() $p=1$
, that
$p=1$
, that
for some absolute constant K which varies through occurrences. Therefore,
The function on the right-hand side attains a minimum at
![]() $r=(n+1)/(n+1+\alpha )$
. With this choice of r, we obtain
$r=(n+1)/(n+1+\alpha )$
. With this choice of r, we obtain
 $$ \begin{align*}(n+1) \vert a_{n+1}\vert \le K \left(1+\frac{\alpha}{n+1}\right)^{n+1} \left(n+1+\alpha\right)^{\alpha} \le K (n+1)^{\alpha}.\end{align*} $$
$$ \begin{align*}(n+1) \vert a_{n+1}\vert \le K \left(1+\frac{\alpha}{n+1}\right)^{n+1} \left(n+1+\alpha\right)^{\alpha} \le K (n+1)^{\alpha}.\end{align*} $$
Therefore, replacing
![]() $n+1$
by n,
$n+1$
by n,
Using a similar argument, one can show that
![]() $\vert b_n \vert \le K n^{\alpha -1}$
. The proof for the second part of the theorem is identical, except for
$\vert b_n \vert \le K n^{\alpha -1}$
. The proof for the second part of the theorem is identical, except for
![]() $\alpha $
suitably replaced by
$\alpha $
suitably replaced by
![]() $\alpha (\mathcal {H})$
.
$\alpha (\mathcal {H})$
.
4.4 Proof of Theorem 3
The Hardy–Stein identity (see [Reference Pommerenke19, p. 126]) for the function
![]() $h'$
implies that
$h'$
implies that
Since
![]() $M_p^p(r,h')$
is a (strictly) increasing function of r, we have
$M_p^p(r,h')$
is a (strictly) increasing function of r, we have
Therefore,
 $$ \begin{align*} \frac{d^2}{dr^2}M_p^p(r,h') & \le \frac{p^2}{2\pi} \int_{0}^{2\pi} |h'(r{e^{i\theta}})|^{p-2}|h"(r{e^{i\theta}})|^2 d\theta\\ & = \frac{p^2}{2\pi} \int_{0}^{2\pi} |h'(r{e^{i\theta}})|^{p}\left|\frac{h"(r{e^{i\theta}})}{h'(r{e^{i\theta}})}\right|{}^2 d\theta\\ & \le \frac{p^2}{2\pi} \int_{0}^{2\pi} \frac{(1+r)^{(\alpha-1)p}}{(1-r)^{(\alpha+1)p}} \cdot \frac{C^2}{(1-r)^{2\beta}} \, d\theta \quad \left(\text{by } (4.5)\right)\\ & \le \frac{K}{(1-r)^{(\alpha+1)p+2\beta}}, \end{align*} $$
$$ \begin{align*} \frac{d^2}{dr^2}M_p^p(r,h') & \le \frac{p^2}{2\pi} \int_{0}^{2\pi} |h'(r{e^{i\theta}})|^{p-2}|h"(r{e^{i\theta}})|^2 d\theta\\ & = \frac{p^2}{2\pi} \int_{0}^{2\pi} |h'(r{e^{i\theta}})|^{p}\left|\frac{h"(r{e^{i\theta}})}{h'(r{e^{i\theta}})}\right|{}^2 d\theta\\ & \le \frac{p^2}{2\pi} \int_{0}^{2\pi} \frac{(1+r)^{(\alpha-1)p}}{(1-r)^{(\alpha+1)p}} \cdot \frac{C^2}{(1-r)^{2\beta}} \, d\theta \quad \left(\text{by } (4.5)\right)\\ & \le \frac{K}{(1-r)^{(\alpha+1)p+2\beta}}, \end{align*} $$
for some positive constant K, which is not the same in subsequent occurrences. Integrating twice from
![]() $0$
to s
$0$
to s
![]() $(s<1)$
, we arrive at the estimate
$(s<1)$
, we arrive at the estimate
Thus, an appeal to (4.2) gives
 $$ \begin{align*}\| f\|_p^p \le C \int_{0}^{1} (1-s)^{p-1} M_p^p(s,h') ds \le C \int_{0}^{1} \frac{ds}{(1-s)^{\alpha p +2\beta-1}}.\end{align*} $$
$$ \begin{align*}\| f\|_p^p \le C \int_{0}^{1} (1-s)^{p-1} M_p^p(s,h') ds \le C \int_{0}^{1} \frac{ds}{(1-s)^{\alpha p +2\beta-1}}.\end{align*} $$
The last integral converges for
![]() $\alpha p +2\beta -1<1$
, or equivalently,
$\alpha p +2\beta -1<1$
, or equivalently,
![]() $p<2(1-\beta )/\alpha $
. Therefore,
$p<2(1-\beta )/\alpha $
. Therefore,
![]() $f \in h^p$
for
$f \in h^p$
for
![]() $p<2(1-\beta )/\alpha $
.
$p<2(1-\beta )/\alpha $
.
The proof for
![]() $f \in \mathcal {H}$
is similar, one only needs to replace
$f \in \mathcal {H}$
is similar, one only needs to replace
![]() $\alpha $
by
$\alpha $
by
![]() $\alpha (\mathcal {H})$
, wherever applicable.
$\alpha (\mathcal {H})$
, wherever applicable.
4.5 Proof of Theorem 4
Let
![]() $0<r<1$
and write
$0<r<1$
and write
Clearly,
![]() $F(0)=\mathcal {F}(0)=0$
. Lemma 4 of [Reference Wang, Liang and Zhang23] implies that there exist real numbers
$F(0)=\mathcal {F}(0)=0$
. Lemma 4 of [Reference Wang, Liang and Zhang23] implies that there exist real numbers
![]() $\mu $
,
$\mu $
,
![]() $\theta _0$
and an analytic function
$\theta _0$
and an analytic function
![]() $Q(z)$
with positive real part, such that
$Q(z)$
with positive real part, such that
Let
Without any loss of generality, we may assume
![]() $\vert Q(0)\vert =1$
, so that
$\vert Q(0)\vert =1$
, so that
![]() $\vert P(0)\vert =1$
. Since
$\vert P(0)\vert =1$
. Since
![]() $Q(z)$
has positive real part, so does
$Q(z)$
has positive real part, so does
![]() $1/Q(z)$
. The dilatation
$1/Q(z)$
. The dilatation
![]() $w(z) = g'(z)/h'(z)$
satisfies
$w(z) = g'(z)/h'(z)$
satisfies
![]() $w(0)=0$
and
$w(0)=0$
and
![]() $\vert w(z)\vert <1$
for all
$\vert w(z)\vert <1$
for all
![]() $z \in {\mathbb D}$
. We see that
$z \in {\mathbb D}$
. We see that
 $$ \begin{align*} \log\vert h'(e^{i\theta_0}z)+c g'(e^{i\theta_0}z)\vert & =\log \vert P(z)\vert +\log\left\vert\frac{1}{Q(z)}\right\vert +\log\left\vert\frac{1}{1-z^2}\right\vert\\ & \qquad +\log\left\vert\frac{1}{1+e^{2i\mu}w(e^{i\theta_0}z)}\right\vert+ \log\vert1+cw(e^{i\theta_0}z)\vert. \end{align*} $$
$$ \begin{align*} \log\vert h'(e^{i\theta_0}z)+c g'(e^{i\theta_0}z)\vert & =\log \vert P(z)\vert +\log\left\vert\frac{1}{Q(z)}\right\vert +\log\left\vert\frac{1}{1-z^2}\right\vert\\ & \qquad +\log\left\vert\frac{1}{1+e^{2i\mu}w(e^{i\theta_0}z)}\right\vert+ \log\vert1+cw(e^{i\theta_0}z)\vert. \end{align*} $$
In view of Lemmas A–C, we have for
![]() $z \in {\mathbb D}^+$
,
$z \in {\mathbb D}^+$
,
 $$ \begin{align*} (\log\vert h'(z)+cg'(z)\vert)^* & \le \left(\log\left\vert\frac{1+z}{1-z}\right\vert\right)^* + \left(\log\left\vert\frac{1+z}{1-z}\right\vert\right)^*\\ & \quad +\left(\log\left\vert\frac{1}{1-z^2}\right\vert\right)^*+\left(\log\left\vert\frac{1}{1-z}\right\vert\right)^* +\left(\log\vert 1+z\vert\right)^*,\end{align*} $$
$$ \begin{align*} (\log\vert h'(z)+cg'(z)\vert)^* & \le \left(\log\left\vert\frac{1+z}{1-z}\right\vert\right)^* + \left(\log\left\vert\frac{1+z}{1-z}\right\vert\right)^*\\ & \quad +\left(\log\left\vert\frac{1}{1-z^2}\right\vert\right)^*+\left(\log\left\vert\frac{1}{1-z}\right\vert\right)^* +\left(\log\vert 1+z\vert\right)^*,\end{align*} $$
which implies
 $$ \begin{align*}(\log\vert h'(z)+cg'(z)\vert)^* \le \left(\log\left\vert \frac{(1+z)^2}{(1-z)^4}\right\vert\right)^* = \left(\log\vert H'(z)+G'(z) \vert\right)^*,\end{align*} $$
$$ \begin{align*}(\log\vert h'(z)+cg'(z)\vert)^* \le \left(\log\left\vert \frac{(1+z)^2}{(1-z)^4}\right\vert\right)^* = \left(\log\vert H'(z)+G'(z) \vert\right)^*,\end{align*} $$
i.e.,
![]() $({\operatorname {Re}\,} F)^* \le ({\operatorname {Re}\,} \mathcal {F})^*$
. For
$({\operatorname {Re}\,} F)^* \le ({\operatorname {Re}\,} \mathcal {F})^*$
. For
![]() $f=h+\overline {g} \in C_H^0$
, the function
$f=h+\overline {g} \in C_H^0$
, the function
![]() $f+c \overline {f} \in C_H$
for every constant
$f+c \overline {f} \in C_H$
for every constant
![]() $c \in {\mathbb D}$
. Also, it is known that
$c \in {\mathbb D}$
. Also, it is known that
![]() $C_H$
is linear invariant and
$C_H$
is linear invariant and
![]() $\alpha (C_H)=3$
. Therefore, (4.7) leads to the inequalities
$\alpha (C_H)=3$
. Therefore, (4.7) leads to the inequalities
 $$ \begin{align*}\frac{(1-r)^2}{(1+r)^4} \le |h'(r{e^{i\theta}})+cg'(r{e^{i\theta}})| \le \frac{(1+r)^2}{(1-r)^4},\end{align*} $$
$$ \begin{align*}\frac{(1-r)^2}{(1+r)^4} \le |h'(r{e^{i\theta}})+cg'(r{e^{i\theta}})| \le \frac{(1+r)^2}{(1-r)^4},\end{align*} $$
so that
That
![]() $\mathcal {F}$
is univalent and
$\mathcal {F}$
is univalent and
![]() $\mathcal {F}({\mathbb D})$
is a Steiner symmetric domain can be proved using an argument similar to the one presented in [Reference Girela12, Lemma 1], we include the details below for the convenience of the reader. Therefore, the proof of the theorem is completed through an appeal to Lemma D. Since the harmonic Koebe function
$\mathcal {F}({\mathbb D})$
is a Steiner symmetric domain can be proved using an argument similar to the one presented in [Reference Girela12, Lemma 1], we include the details below for the convenience of the reader. Therefore, the proof of the theorem is completed through an appeal to Lemma D. Since the harmonic Koebe function
![]() $K=H+\overline {G}\in C_H^0$
, the sharpness can be seen by letting
$K=H+\overline {G}\in C_H^0$
, the sharpness can be seen by letting
![]() $c \to 1^-$
.
$c \to 1^-$
.
Proposition 1 Let
![]() $\mathcal {G}(z) = \log (H'(z)+G'(z))$
. Then
$\mathcal {G}(z) = \log (H'(z)+G'(z))$
. Then
![]() $\mathcal {G}$
is univalent and
$\mathcal {G}$
is univalent and
![]() $\mathcal {G}({\mathbb D})$
is a Steiner symmetric domain.
$\mathcal {G}({\mathbb D})$
is a Steiner symmetric domain.
Proof For
![]() $0<r<1$
, we see that
$0<r<1$
, we see that
 $$ \begin{align*}{\operatorname{Re}\,} \mathcal{G}(r e^{i \theta})=\log \frac{1}{\left|1-r e^{i \theta}\right|{}^2}+2 \log \left|\frac{1+r e^{i \theta}}{1-r e^{i \theta}}\right|. \end{align*} $$
$$ \begin{align*}{\operatorname{Re}\,} \mathcal{G}(r e^{i \theta})=\log \frac{1}{\left|1-r e^{i \theta}\right|{}^2}+2 \log \left|\frac{1+r e^{i \theta}}{1-r e^{i \theta}}\right|. \end{align*} $$
Thus,
![]() $\operatorname {Re} \mathcal {G}(r e^{i \theta })$
is symmetric with respect to
$\operatorname {Re} \mathcal {G}(r e^{i \theta })$
is symmetric with respect to
![]() $\theta $
on
$\theta $
on
![]() $[-\pi , \pi ]$
, and strictly decreases on
$[-\pi , \pi ]$
, and strictly decreases on
![]() $[0, \pi ]$
. It is easy to see that
$[0, \pi ]$
. It is easy to see that
![]() $\operatorname {Im} \mathcal {G}(r e^{i \theta })>0$
in
$\operatorname {Im} \mathcal {G}(r e^{i \theta })>0$
in
![]() $0<\theta <\pi $
, because the same holds for
$0<\theta <\pi $
, because the same holds for
![]() $\log \left (1 /(1-z)^2\right )$
and
$\log \left (1 /(1-z)^2\right )$
and
![]() $\log ((1+z) /(1-z))$
. Also,
$\log ((1+z) /(1-z))$
. Also,
![]() $\mathcal {G}(r e^{-i \theta })=\overline {\mathcal {G}(r e^{i \theta })}$
. Therefore,
$\mathcal {G}(r e^{-i \theta })=\overline {\mathcal {G}(r e^{i \theta })}$
. Therefore,
![]() $\mathcal {G}$
is injective on
$\mathcal {G}$
is injective on
![]() $|z|=r$
and hence the argument principle implies that
$|z|=r$
and hence the argument principle implies that
![]() $\mathcal {G}$
is univalent in
$\mathcal {G}$
is univalent in
![]() $|z| \le r$
. Finally,
$|z| \le r$
. Finally,
![]() $\mathcal {G}$
maps
$\mathcal {G}$
maps
![]() $\{|z|=r\}$
onto a simple closed curve, which is symmetric with respect to the real axis, with decreasing real part for
$\{|z|=r\}$
onto a simple closed curve, which is symmetric with respect to the real axis, with decreasing real part for
![]() $\theta $
increasing from 0 to
$\theta $
increasing from 0 to
![]() $\pi $
. This shows that
$\pi $
. This shows that
![]() $\mathcal {G}(|z|<r)$
is a Steiner symmetric domain. As this is true for every
$\mathcal {G}(|z|<r)$
is a Steiner symmetric domain. As this is true for every
![]() $r \in (0,1)$
, the desired conclusion follows.
$r \in (0,1)$
, the desired conclusion follows.
4.6 Proof of Corollary 2
Let
![]() $\log (H'(z)+G'(z)) =\sum _{n=1}^\infty c_n z^n$
. Through a routine computation, we see that
$\log (H'(z)+G'(z)) =\sum _{n=1}^\infty c_n z^n$
. Through a routine computation, we see that
For
![]() $p=2$
, Theorem 4 gives
$p=2$
, Theorem 4 gives
 $$ \begin{align*}\sum_{n=1}^\infty \vert\lambda_n\vert^2 r^{2n} \le \sum_{n=1}^\infty \vert c_n\vert^2 r^{2n}.\end{align*} $$
$$ \begin{align*}\sum_{n=1}^\infty \vert\lambda_n\vert^2 r^{2n} \le \sum_{n=1}^\infty \vert c_n\vert^2 r^{2n}.\end{align*} $$
Letting
![]() $r \to 1$
, we obtain
$r \to 1$
, we obtain
 $$ \begin{align*}\sum_{n=1}^\infty \vert\lambda_n\vert^2 \le \sum_{n=1}^\infty \vert c_n\vert^2 = \frac{14\pi^2}{3}.\end{align*} $$
$$ \begin{align*}\sum_{n=1}^\infty \vert\lambda_n\vert^2 \le \sum_{n=1}^\infty \vert c_n\vert^2 = \frac{14\pi^2}{3}.\end{align*} $$
The sharpness follows from Theorem 4.
Acknowledgments
The authors thank Prof. S. Ponnusamy and Prof. D. Bshouty for useful suggestions on an earlier version of this paper. The first author thanks Mr. Kapil Jaglan for helpful discussions during the course of this work.
Competing interests
The authors have no competing interests to declare that are relevant to the content of this paper.