No CrossRef data available.
Article contents
THE 
$L_{r}$ CONVERGENCE AND WEAK LAWS OF LARGE NUMBERS FOR 
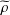$\widetilde{\unicode[STIX]{x1D70C}}$-MIXING RANDOM VARIABLES
Part of:
Limit theorems
Published online by Cambridge University Press: 19 April 2017
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
The 
$L_{r}$ convergence and a class of weak laws of large numbers are obtained for sequences of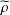 
$\widetilde{\unicode[STIX]{x1D70C}}$-mixing random variables under the uniform Cesàro-type condition. This is weaker than the 
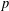$p$th-order Cesàro uniform integrability.
MSC classification
Primary:
60F15: Strong theorems
- Type
- Research Article
- Information
- Copyright
- © 2017 Australian Mathematical Society
References
Bradley, R. C., “On the spectral density and asymptotic normality of weakly dependent random fields”, J. Theoret. Probab.
5 (1992) 355–373; doi:10.1007/BF01046741.Google Scholar
Bradley, R. C., “Equivalent mixing conditions for random fields”, Ann. Probab.
21 (1993) 1921–1926; doi:10.1214/aop/1176989004.Google Scholar
Bryc, W. and Smolenski, W., “Moment conditions for almost sure convergence of weakly correlated random variables”, Proc. Amer. Math. Soc.
119 (1993) 629–635; doi:10.1090/S0002-9939-1993-1149969-7.Google Scholar
Chandra, T. K. and Goswami, A., “Cesàro uniform integrability and the strong law of large numbers”, Sankhyā Ser. A
54 (1992) 215–231; www.jstor.org/stable/25050875.Google Scholar
Kuczmaszewska, A., “On Chung–Teicher type strong law of large numbers for
$\unicode[STIX]{x1D70C}^{\ast }$
-mixing random variables”, Discrete Dyn. Nat. Soc.
3 (2008) 77–86; doi:10.1155/2008/140548.Google Scholar
$\unicode[STIX]{x1D70C}^{\ast }$
-mixing random variables”, Discrete Dyn. Nat. Soc.
3 (2008) 77–86; doi:10.1155/2008/140548.Google ScholarLin, Z. Y., Lu, C. R. and Su, Z. G, Foundations of probability limit theory (Higher Education Press, Beijing, 1999).Google Scholar
Meng, Y. J. and Lin, Z. Y., “On the weak laws of large numbers for arrays of random variables”, Statist. Probab. Lett.
79 (2009) 2405–2414; doi:10.1016/j.spl.2009.08.014.Google Scholar
Miller, C., “Three mixing conditions on
$\unicode[STIX]{x1D70C}^{\ast }$
-mixing random fields”, J. Theoret. Probab.
7 (1994) 867–882.CrossRefGoogle Scholar
$\unicode[STIX]{x1D70C}^{\ast }$
-mixing random fields”, J. Theoret. Probab.
7 (1994) 867–882.CrossRefGoogle ScholarPeligrad, M., “On the asymptotic normality of sequences of weak dependent random variables”, J. Theoret. Probab.
9 (1996) 703–715; doi:10.1007/BF02214083.Google Scholar
Peligrad, M., “Maximum of partial sums and an invariance principle for a class of weak dependent random variables”, Proc. Amer. Math. Soc.
126 (1998) 1181–1189; http://www.jstor.org/stable/118631?seq=1#page_scan_tab_contents.Google Scholar
Peligrad, M. and Gut, A., “Almost-sure results for a class of dependent random variables”, J. Theoret. Probab.
12 (1999) 87–104; doi:10.1023/A:1021744626773.Google Scholar
Utev, S. and Peligrad, M., “Maximal inequalities and an invariance principle for a class of weakly dependent random variables”, J. Theoret. Probab.
16 (2003) 101–115; doi:10.1023/A:1022278404634.CrossRefGoogle Scholar
Wu, Q. Y., Probability limit theory for sequences of mixing random variables (Science Press, Beijing, 2006).Google Scholar
Wu, Q. Y., “Complete convergence and weak law of large numbers for
$\unicode[STIX]{x1D70C}$
-mixing sequences of random variables”, Open J. Stat.
2 (2012) 484–490; doi:10.4236/ojs.2012.25062.Google Scholar
$\unicode[STIX]{x1D70C}$
-mixing sequences of random variables”, Open J. Stat.
2 (2012) 484–490; doi:10.4236/ojs.2012.25062.Google ScholarYang, S. C., “Some moment inequalities for partial sums of random variables and their applications”, Chin. Sci. Bull.
43 (1998) 1823–1827.Google Scholar
You have
Access