Hostname: page-component-8448b6f56d-mp689 Total loading time: 0 Render date: 2024-04-25T06:03:12.268Z Has data issue: false hasContentIssue false
  • English
  • Français

The Oseen Type Finite Element Iterative Method for the Stationary Incompressible Magnetohydrodynamics

Part of: Equations of mathematical physics and other areas of application Incompressible viscous fluids Partial differential equations, boundary value problems Partial differential equations, initial value and time-dependent initial-boundary value problems

Published online by Cambridge University Press:  18 January 2017

Xiaojing Dong  and
Yinnian He
Xiaojing Dong*
Affiliation:
School of Mathematics and Statistics, Xi'an Jiaotong University, Xi'an 710049, China School of Mathematics and Statistics, Henan University of Science and Technology, Luoyang 471023, China
Yinnian He*
Affiliation:
School of Mathematics and Statistics, Xi'an Jiaotong University, Xi'an 710049, China
*
*Corresponding author. Email:dongxiaojing99@126.com (X. J. Dong), heyn@mail.xjtu.edu.cn (Y. N. He)
*Corresponding author. Email:dongxiaojing99@126.com (X. J. Dong), heyn@mail.xjtu.edu.cn (Y. N. He)
Get access

Abstract

In this article, by applying the Stokes projection and an orthogonal projection with respect to curl and div operators, some new error estimates of finite element method (FEM) for the stationary incompressible magnetohydrodynamics (MHD) are obtained. To our knowledge, it is the first time to establish the error bounds which are explicitly dependent on the Reynolds numbers, coupling number and mesh size. On the other hand, The uniform stability and convergence of an Oseen type finite element iterative method for MHD with respect to high hydrodynamic Reynolds number Re and magnetic Reynolds number Rm, or small δ=1–σ with

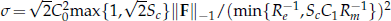
(C0, C1 are constants depending only on Ω and F is related to the source terms of equations) are analyzed under the condition that 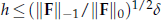. Finally, some numerical tests are presented to demonstrate the effectiveness of this algorithm.

Keywords

Uniform stabilityconvergenceOseen type iterative methodfinite element methodstationary incompressible magnetohydrodynamics

MSC classification

Secondary: 35Q30: Navier-Stokes equations 65M60: Finite elements, Rayleigh-Ritz and Galerkin methods, finite methods 65N30: Finite elements, Rayleigh-Ritz and Galerkin methods, finite methods 76D05: Navier-Stokes equations
Type
Research Article
Information
Advances in Applied Mathematics and Mechanics , Volume 9 , Issue 4 , August 2017 , pp. 775 - 794
Copyright
Copyright © Global-Science Press 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1] Moreau, R., Magneto-Hydrodynamics, Kluwer Academic Publishers, 1990.Google Scholar
[2] Gerbeau, J.-F., Le Bris, C. and Lelièvre, T., Mathematical Methods for the Magnetohydrodynamics of Liquid Metals, Numerical Mathematics and Scientific Computation, Oxford University Press, New York, 2006.Google Scholar
[3] Gunzburger, M. D., Meir, A. J. and Peterson, J. S., On the existence, uniqueness and finite element approximation of solutions of the equations of stationary, incompressible magnetohydrodynamics, Math. Comput., 56 (1991), pp. 523–563.Google Scholar
[4] Schötzau, D., Mixed finite element methods for stationary incompressible magnetohydrodynamics, Numer. Math., 96 (2004), pp. 771–800.Google Scholar
[5] Hasler, U., Schneebeli, A. and Schötzau, D., Mixed finite element approximation of incompressible MHD problems based on weighted regularization, Appl. Numer. Math., 51 (2004), pp. 19–45.CrossRefGoogle Scholar
[6] Greif, C., Li, D., Schötzau, D. and Wei, X. X., A mixed finite element method with exactly divergence-free velocities for incompressible magnetohydrodynamics, Comput. Methods Appl. Mech. Eng., 199 (2010), pp. 2840–2855.Google Scholar
[7] Salah, N. B., Soulaimani, A., Habashi, W. G. and Fortin, M., A conservative stabilizedfinite element method for the magneto-hydrodynamic equations, Int. J. Numer. Meth. Fluids, 29 (1999), pp. 535–554.3.0.CO;2-D>CrossRefGoogle Scholar
[8] Codina, R. and Silva, N. H., Stabilized finite element approximation of the stationary magneto-hydrodynamics equations, Comput. Mech., 38 (2006), pp. 344–355.Google Scholar
[9] Gerbeau, J.-F., A stabilized finite element method for the incompressible magnetohydrodynamic equations, Numer. Math., 87 (2000), pp. 83–111.CrossRefGoogle Scholar
[10] Layton, W. J., Meir, A. J. and Schmidt, P. G., A two-level discretization method for the stationary MHD equations, Elec. Tran. Numer. Anal., 6 (1997), pp. 198–210.Google Scholar
[11] Aydin, S. H., Nesliturk, A. I. and Tezer-Sezgin, M., Two-level finite element method with a stabilizing subgrid for the incompressible MHD equations, Int. J. Numer. Meth. Fluids, 62 (2010), pp. 188–210.CrossRefGoogle Scholar
[12] Dong, X. J. and He, Y. N., Two-level Newton iterative method for the 2D/3D stationary incompressible magnetohydrodynamics, J. Sci. Comput., 63 (2015), pp. 426–451.Google Scholar
[13] He, Y. N., Unconditional convergence of the Euler semi-implicit scheme for the 3D incompressible MHD equations, IMA J. Numer. Anal., 35 (2015), pp. 767–801.Google Scholar
[14] Yuksel, G. and Ingram, R., Numerical analysis of a finite element, Crank-Nicolson discretization for MHD flows at small magnetic Reynolds numbers, Int. J. Numer. Anal. Model., 10 (2013), pp. 74–98.Google Scholar
[15] He, Y. N. and Li, J., Convergence of three iterative methods based on the finite element discretization for the stationary Navier-Stokes equations, Comput. Methods Appl. Mech. Eng., 198 (2009), pp. 1351–1359.CrossRefGoogle Scholar
[16] Zhang, Y., Xu, H. and He, Y. N., On two-level Oseen iterative methods for the 2D/3D steady Navier-Stokes equations, Comput. Fluids, 107 (2015), pp. 89–99.Google Scholar
[17] He, Y. N., Stability and convergence of iterative methods related to viscosities for the 2D/3D steady Navier-Stokes equations, J. Math. Anal. Appl., 423 (2015), pp. 1129–1149.Google Scholar
[18] Dong, X. J., He, Y. N. and Zhang, Y., Convergence analysis of three finite element iterative methods for the 2D/3D stationary incompressible magnetohydrodynamics, Comput. Methods Appl. Mech. Eng., 276 (2014), pp. 287–311.CrossRefGoogle Scholar
[19] Adams, R. A., Sobolev Space, Academic Press, New York, 1975.Google Scholar
[20] Girault, V. and Raviart, P. A., Finite Element Approximation of Navier-Stokes Equations, Springer-Verlag, Berlin, 1986.Google Scholar
[21] Heywood, J. and Rannacher, R., Finite element approximation of the nonstationary Navier-Stokes problem I: regularity of solutions and second-order error estimates for spatial discretization, SIAM J. Numer. Anal., 19 (1982), pp. 275–311.CrossRefGoogle Scholar
[22] Brenner, S. C., Cui, J., Li, F. and Sung, L. Y., A nonconforming finite element method for a two-dimensional curl-curl and grad-div problem, Numer. Math., 109 (2008), pp. 509–533.CrossRefGoogle Scholar
[23] He, Y. N., Euler implicit/ explicit iterative scheme for the stationary Navier-Stokes equations, Numer. Math., 123 (2013), pp. 67–96.CrossRefGoogle Scholar
[24] Sermane, M. and Temam, R., Some mathematics questions related to theMHD equations, Commun. Pure Appl. Math., XXXIV (1984), pp. 635–664.Google Scholar
[25] He, Y. N., Two-level method based on finite element and Crank-Nicolson extrapolation for the time-dependent Navier-Stokes equations, SIAM J. Numer. Anal., 41 (2003), pp. 1263–1285.CrossRefGoogle Scholar
[26] Heywood, J. G. and Rannacher, R., Finite element approximation of the nonstationary Navier-Stokes problem III: Smoothing property and high order error estimates for spatial discretization, SIAM J. Numer. Anal., 25 (1988), pp. 489–512.CrossRefGoogle Scholar
[27] Bernardi, C. and Raugel, G., A conforming finite element method for the time-dependent Navier-Stokes equations, SIAM J. Numer. Anal., 22 (1985), pp. 211–224 Google Scholar