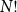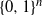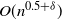76 results in 94Axx
Convergence rate of entropy-regularized multi-marginal optimal transport costs
- Part of
-
- Journal:
- Canadian Journal of Mathematics , First View
- Published online by Cambridge University Press:
- 15 March 2024, pp. 1-21
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
On the Ziv–Merhav theorem beyond Markovianity I
- Part of
-
- Journal:
- Canadian Journal of Mathematics , First View
- Published online by Cambridge University Press:
- 07 March 2024, pp. 1-25
-
- Article
- Export citation
Unified bounds for the independence number of graphs
- Part of
-
- Journal:
- Canadian Journal of Mathematics , First View
- Published online by Cambridge University Press:
- 11 December 2023, pp. 1-21
-
- Article
- Export citation
t-Design Curves and Mobile Sampling on the Sphere
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 11 / 2023
- Published online by Cambridge University Press:
- 23 November 2023, e105
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Approximate discrete entropy monotonicity for log-concave sums
- Part of
-
- Journal:
- Combinatorics, Probability and Computing / Volume 33 / Issue 2 / March 2024
- Published online by Cambridge University Press:
- 13 November 2023, pp. 196-209
-
- Article
- Export citation
Markov capacity for factor codes with an unambiguous symbol
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems , First View
- Published online by Cambridge University Press:
- 07 November 2023, pp. 1-30
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
The unified extropy and its versions in classical and Dempster–Shafer theories
- Part of
-
- Journal:
- Journal of Applied Probability , First View
- Published online by Cambridge University Press:
- 23 October 2023, pp. 1-12
-
- Article
- Export citation
Encoding subshifts through sliding block codes
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems , First View
- Published online by Cambridge University Press:
- 03 August 2023, pp. 1-20
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Information-theoretic convergence of extreme values to the Gumbel distribution
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 61 / Issue 1 / March 2024
- Published online by Cambridge University Press:
- 21 June 2023, pp. 244-254
- Print publication:
- March 2024
-
- Article
- Export citation
On asymptotic fairness in voting with greedy sampling
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 55 / Issue 3 / September 2023
- Published online by Cambridge University Press:
- 10 May 2023, pp. 999-1032
- Print publication:
- September 2023
-
- Article
- Export citation
Costa’s concavity inequality for dependent variables based on the multivariate Gaussian copula
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 60 / Issue 4 / December 2023
- Published online by Cambridge University Press:
- 12 April 2023, pp. 1136-1156
- Print publication:
- December 2023
-
- Article
- Export citation
A new lifetime distribution by maximizing entropy: properties and applications
- Part of
-
- Journal:
- Probability in the Engineering and Informational Sciences / Volume 38 / Issue 1 / January 2024
- Published online by Cambridge University Press:
- 28 February 2023, pp. 189-206
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Random feedback shift registers and the limit distribution for largest cycle lengths
- Part of
-
- Journal:
- Combinatorics, Probability and Computing / Volume 32 / Issue 4 / July 2023
- Published online by Cambridge University Press:
- 14 February 2023, pp. 559-593
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Constant rank factorisations of smooth maps, with applications to sonar
- Part of
-
- Journal:
- European Journal of Applied Mathematics / Volume 35 / Issue 1 / February 2024
- Published online by Cambridge University Press:
- 01 December 2022, pp. 1-39
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
A negative binomial approximation in group testing
- Part of
-
- Journal:
- Probability in the Engineering and Informational Sciences / Volume 37 / Issue 4 / October 2023
- Published online by Cambridge University Press:
- 28 October 2022, pp. 973-996
-
- Article
- Export citation
Fast mixing of a randomized shift-register Markov chain
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 60 / Issue 1 / March 2023
- Published online by Cambridge University Press:
- 02 September 2022, pp. 253-266
- Print publication:
- March 2023
-
- Article
- Export citation
Varentropy of doubly truncated random variable
- Part of
-
- Journal:
- Probability in the Engineering and Informational Sciences / Volume 37 / Issue 3 / July 2023
- Published online by Cambridge University Press:
- 08 July 2022, pp. 852-871
-
- Article
- Export citation
UNIVERSAL CODING AND PREDICTION ON ERGODIC RANDOM POINTS
- Part of
-
- Journal:
- Bulletin of Symbolic Logic / Volume 28 / Issue 3 / September 2022
- Published online by Cambridge University Press:
- 02 May 2022, pp. 387-412
- Print publication:
- September 2022
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Variational model with nonstandard growth conditions for restoration of satellite optical images using synthetic aperture radar
- Part of
-
- Journal:
- European Journal of Applied Mathematics / Volume 34 / Issue 1 / February 2023
- Published online by Cambridge University Press:
- 11 March 2022, pp. 77-105
-
- Article
-
- You have access
- HTML
- Export citation
On reconsidering entropies and divergences and their cumulative counterparts: Csiszár's, DPD's and Fisher's type cumulative and survival measures
- Part of
-
- Journal:
- Probability in the Engineering and Informational Sciences / Volume 37 / Issue 1 / January 2023
- Published online by Cambridge University Press:
- 21 February 2022, pp. 294-321
-
- Article
-
- You have access
- Open access
- HTML
- Export citation