Hostname: page-component-8448b6f56d-xtgtn Total loading time: 0 Render date: 2024-04-19T08:13:54.265Z Has data issue: false hasContentIssue false
  • English
  • Français

II.—Asymptotic Renewal Theorems

Published online by Cambridge University Press:  14 February 2012

Walter L. Smith
Walter L. Smith
Affiliation:
Statistical Laboratory, University of Cambridge.
Get access Rights & Permissions [Opens in a new window]

Synopsis

A sequence of non-negative random variables {Xi} is called a renewal process, and if the Xi may only take values on some sequence 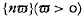it is termed a discrete renewal process. The greatest k such that X1 + X2 + … + Xk ≤ x(> o) is a random variable N(x) and theorems concerning N(x) are renewal theorems. This paper is concerned with the proofs of a number of renewal theorems, the main emphasis being on processes which are not discrete. It is assumed throughout that the {Xi} are independent and identically distributed.

If H(x) = Ɛ{N(x)} and K(x) is the distribution function of any non-negative random variable with mean K > o, then it is shown that for the non-discrete process

where Ɛ{Xi} need not be finite; a similar result is proved for the discrete process. This general renewal theorem leads to a number of new results concerning the non-discrete process, including a discussion of the stationary “age-distribution” of “renewals” and a discussion of the variance of N(x). Lastly, conditions are established under which

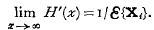
These new conditions are much weaker than those of previous theorems by Feller, Täcklind, and Cox and Smith.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 64 , Issue 1 , 1953 , pp. 9 - 48
Copyright
Copyright © Royal Society of Edinburgh 1953

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES TO LITERATURE

(1)Blackwell, D., 1948. “A renewal theorem”, Duke Math. J., 15, 145–150.CrossRefGoogle Scholar
(2)Bochner, S., and Chandrasekharan, K., 1949. “Fourier transforms”, Ann. Math. Stud., No. 19, Princeton.Google Scholar
(3)Chung, K. L., and Pollard, H., 1952. “An extension of renewal theory”, Proc. Amer. Math. Soc, 3, 303–309.CrossRefGoogle Scholar
(4)Chung, K. L., and Wolfowitz, J., 1952. “On a limit theorem in renewal theory”, Ann. Math., 55, 1–6.CrossRefGoogle Scholar
(5)Cox, D. R., and Smith, W. L., 1953. “A direct proof of a fundamental theorem of renewal theory”, Skand. Aktuar Tidskr. [In the press.]CrossRefGoogle Scholar
(6)Doob, J. L., 1948. “Renewal theory from the point of view of the theory of probability”, Trans. Amer. Math. Soc, 63, 422–438.CrossRefGoogle Scholar
(7)Erdös, P., Feller, W., and Pollard, H., 1949. “A theorem on power series”, Bull. Amer. Math. Soc, 55, 201–204.CrossRefGoogle Scholar
(8)Feller, W., 1941. “On the integral equation of renewal theory”, Ann. Math. Statist., 12, 243–267.CrossRefGoogle Scholar
(9)Feller, W., 1949. “Fluctuation theory of recurrent events”, Trans. Amer. Math. Soc, 67, 98–119.CrossRefGoogle Scholar
(10)Harris, T. E., 1951. “Some mathematical models for branching processes”, Proc Second Berkeley Symposium, 305–328. University of California Press.Google Scholar
(11)Paley, R. E. A. C., and Wiener, N., 1934. “Fourier transforms in the complex domain”, Amer. Math. Soc. Colloquium Publications, XIX, New York.Google Scholar
(12)Pitt, H. R., 1940. “General Tauberian theorems (II)”, J. Lond. Math. Soc, 15, 97–112.CrossRefGoogle Scholar
(13)Smith, W. L., “Transient queue and storage phenomena”. [To be published.]Google Scholar
(14)Stein, C., 1946. “A note on cumulative sums”, Ann. Math. Statist., 17, 498–499.CrossRefGoogle Scholar
(15)Täcklind, S., 1945. “Fourieranalytische behandlung vom erneuerungs-problem”, Skand. Aktuar Tidskr., 68–105.Google Scholar
(16)Titchmarsh, E. C., 1939. Theory of Functions. 2nd Ed.Oxford University Press.Google Scholar
(17)Titchmarsh, E. C., 1948. Theory of Fourier Integrals. 2nd Ed.Oxford University Press.Google Scholar
(18)Widder, D. V., 1941. The Laplace Transform. Princeton University Press.Google Scholar
(19)Wiener, N., 1933. The Fourier Integral and Certain of its Applications. Cambridge.Google Scholar
(20)Wiener, N., and Pitt, H. R., 1938. “On absolutely convergent Fourier-Stieltjes transforms”, Duke Math. J., 4, 420–436.CrossRefGoogle Scholar
(21)Wintner, A., 1947. The Fourier Transforms of Probability Distributions. Baltimore.Google Scholar