Article contents
Fullness of crossed products of factors by discrete groups
Published online by Cambridge University Press: 23 April 2019
Abstract
Let M be an arbitrary factor and 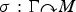 $\sigma : \Gamma \curvearrowright M$ an action of a discrete group. In this paper, we study the fullness of the crossed product
$\sigma : \Gamma \curvearrowright M$ an action of a discrete group. In this paper, we study the fullness of the crossed product  $M \rtimes _\sigma \Gamma $. When Γ is amenable, we obtain a complete characterization: the crossed product factor
$M \rtimes _\sigma \Gamma $. When Γ is amenable, we obtain a complete characterization: the crossed product factor 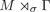 $M \rtimes _\sigma \Gamma $ is full if and only if M is full and the quotient map
$M \rtimes _\sigma \Gamma $ is full if and only if M is full and the quotient map 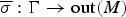 $\overline {\sigma } : \Gamma \rightarrow {\rm out}(M)$ has finite kernel and discrete image. This answers the question of Jones from [11]. When M is full and Γ is arbitrary, we give a sufficient condition for
$\overline {\sigma } : \Gamma \rightarrow {\rm out}(M)$ has finite kernel and discrete image. This answers the question of Jones from [11]. When M is full and Γ is arbitrary, we give a sufficient condition for 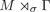 $M \rtimes _\sigma \Gamma $ to be full which generalizes both Jones' criterion and Choda's criterion. In particular, we show that if M is any full factor (possibly of type III) and Γ is a non-inner amenable group, then the crossed product
$M \rtimes _\sigma \Gamma $ to be full which generalizes both Jones' criterion and Choda's criterion. In particular, we show that if M is any full factor (possibly of type III) and Γ is a non-inner amenable group, then the crossed product 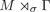 $M \rtimes _\sigma \Gamma $ is full.
$M \rtimes _\sigma \Gamma $ is full.
MSC classification
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 150 , Issue 5 , October 2020 , pp. 2368 - 2378
- Copyright
- Copyright © Royal Society of Edinburgh 2019
References
- 1
- Cited by



