Article contents
Embeddings for the space 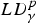 $LD_\gamma ^{p}$ on sets of finite perimeter
$LD_\gamma ^{p}$ on sets of finite perimeter
Published online by Cambridge University Press: 07 May 2019
Abstract
Given an open set with finite perimeter  $\Omega \subset {\open R}^n$, we consider the space
$\Omega \subset {\open R}^n$, we consider the space  $LD_\gamma ^{p}(\Omega )$,
$LD_\gamma ^{p}(\Omega )$, 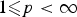 $1\les p<\infty $, of functions with pth-integrable deformation tensor on Ω and with pth-integrable trace value on the essential boundary of Ω. We establish the continuous embedding
$1\les p<\infty $, of functions with pth-integrable deformation tensor on Ω and with pth-integrable trace value on the essential boundary of Ω. We establish the continuous embedding  $LD_\gamma ^{p}(\Omega )\subset L^{pN/(N-1)}(\Omega )$. The space
$LD_\gamma ^{p}(\Omega )\subset L^{pN/(N-1)}(\Omega )$. The space  $LD_\gamma ^{p}(\Omega )$ and this embedding arise naturally in studying the motion of rigid bodies in a viscous, incompressible fluid.
$LD_\gamma ^{p}(\Omega )$ and this embedding arise naturally in studying the motion of rigid bodies in a viscous, incompressible fluid.
Keywords
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 150 , Issue 5 , October 2020 , pp. 2442 - 2461
- Copyright
- Copyright © Royal Society of Edinburgh 2019
References
- 1
- Cited by



