No CrossRef data available.
Article contents
THE MIXED SCHMIDT CONJECTURE IN THE THEORY OF DIOPHANTINE APPROXIMATION
Published online by Cambridge University Press: 10 June 2011
Abstract
Let 𝒟=(dn)∞n=1 be a sequence of integers with dn≥2, and let (i,j) be a pair of strictly positive numbers with i+j=1. We prove that the set of x∈ℝ for which there exists some constant c(x)≧0 such that 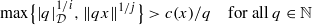 is one-quarter winning (in the sense of Schmidt games). Thus the intersection of any countable number of such sets is of full dimension. This, in turn, establishes the natural analogue of Schmidt’s conjecture within the framework of the de Mathan–Teulié conjecture, also known as the “mixed Littlewood conjecture”.
is one-quarter winning (in the sense of Schmidt games). Thus the intersection of any countable number of such sets is of full dimension. This, in turn, establishes the natural analogue of Schmidt’s conjecture within the framework of the de Mathan–Teulié conjecture, also known as the “mixed Littlewood conjecture”.
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © University College London 2011


