Hostname: page-component-8448b6f56d-c4f8m Total loading time: 0 Render date: 2024-04-23T17:12:08.143Z Has data issue: false hasContentIssue false
  • English
  • Français

DISCREPANCY OF SECOND ORDER DIGITAL SEQUENCES IN FUNCTION SPACES WITH DOMINATING MIXED SMOOTHNESS

Part of: Probabilistic theory: distribution modulo $1$; metric theory of algorithms Nontrigonometric harmonic analysis Holomorphic functions of several complex variables Probabilistic methods, simulation and stochastic differential equations Linear function spaces and their duals

Published online by Cambridge University Press:  29 November 2017

Josef Dick ,
Aicke Hinrichs ,
Lev Markhasin  and
Friedrich Pillichshammer
Josef Dick
Affiliation:
School of Mathematics and Statistics, The University of New South Wales, Sydney NSW 2052, Australia email josef.dick@unsw.edu.au
Aicke Hinrichs
Affiliation:
Institut für Funktionalanalysis, Johannes Kepler Universität Linz, Altenbergerstraße 69, 4040 Linz, Austria email aicke.hinrichs@jku.at
Lev Markhasin
Affiliation:
Institut für Stochastik uand Anwendungen, Universität Stuttgart, Pfaffenwaldring 57, 70569 Stuttgart, Germany email lev.markhasin@mathematik.uni-stuttgart.de
Friedrich Pillichshammer
Affiliation:
Institut für Finanzmathematik und angewandte Zahlentheorie, Johannes Kepler Universität Linz, Altenbergerstraße 69, 4040 Linz, Austria email friedrich.pillichshammer@jku.at
Get access

Abstract

The discrepancy function measures the deviation of the empirical distribution of a point set in 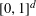$[0,1]^{d}$ from the uniform distribution. In this paper, we study the classical discrepancy function with respect to the bounded mean oscillation and exponential Orlicz norms, as well as Sobolev, Besov and Triebel–Lizorkin norms with dominating mixed smoothness. We give sharp bounds for the discrepancy function under such norms with respect to infinite sequences.

MSC classification

Primary: 11K06: General theory of distribution modulo $1$ 11K38: Irregularities of distribution, discrepancy
Secondary: 32A37: Other spaces of holomorphic functions (e.g. bounded mean oscillation (BMOA), vanishing mean oscillation (VMOA)) 42C10: Fourier series in special orthogonal functions (Legendre polynomials, Walsh functions, etc.) 46E30: Spaces of measurable functions ($L^p$-spaces, Orlicz spaces, Köthe function spaces, Lorentz spaces, rearrangement invariant spaces, ideal spaces, etc.) 46E35: Sobolev spaces and other spaces of “smooth” functions, embedding theorems, trace theorems 65C05: Monte Carlo methods
Type
Research Article
Information
Mathematika , Volume 63 , Issue 3 , 2017 , pp. 863 - 894
Copyright
Copyright © University College London 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Beck, J. and Chen, W. W. L., Irregularities of Distribution, Cambridge University Press (Cambridge, 1987).Google Scholar
Bilyk, D., Lacey, M. T., Parissis, I. and Vagharshakyan, A., Exponential squared integrability of the discrepancy function in two dimensions. Mathematika 55 2009, 2470–2502.Google Scholar
Bilyk, D., Lacey, M. T. and Vagharshakyan, A., On the small ball inequality in all dimensions. J. Funct. Anal. 254 2008, 2470–2502.Google Scholar
Bilyk, D. and Markhasin, L., BMO and exponential Orlicz space estimates of the discrepancy function in arbitrary dimension. J. Anal. Math. (to appear).Google Scholar
Chang, S.-Y. A. and Fefferman, R., A continuous version of duality of H 1 with BMO on the bidisc. Ann. of Math. (2) 112 1980, 179–201.Google Scholar
Chang, S.-Y. A., Wilson, J. M. and Wolff, T. H., Some weighted norm inequalities concerning the Schrödinger operators. Comment. Math. Helv. 60 1985, 217–246.CrossRefGoogle Scholar
Chen, W. W. L., On irregularities of distribution. Mathematika 27 1981, 153–170.Google Scholar
Chen, W. W. L. and Skriganov, M. M., Explicit constructions in the classical mean squares problem in irregularities of point distribution. J. Reine Angew. Math. 545 2002, 67–95.Google Scholar
Dick, J., Explicit constructions of quasi-Monte Carlo rules for the numerical integration of high-dimensional periodic functions. SIAM J. Numer. Anal. 45 2007, 2141–2176.Google Scholar
Dick, J., Walsh spaces containing smooth functions and quasi-Monte Carlo rules of arbitrary high order. SIAM J. Numer. Anal. 46 2008, 1519–1553.CrossRefGoogle Scholar
Dick, J., Discrepancy bounds for infinite-dimensional order two digital sequences over F2 . J. Number Theory 136 2014, 204–232.Google Scholar
Dick, J. and Baldeaux, J., Equidistribution properties of generalized nets and sequences. In Monte Carlo and Quasi-Monte Carlo Methods 2008 (eds L’Ecuyer, P. and Owen, A.), Springer (Berlin–Heidelberg, 2009), 305–323.CrossRefGoogle Scholar
Dick, J., Hinrichs, A., Markhasin, L. and Pillichshammer, F., Optimal L p -discrepancy bounds for second order digital sequences. Israel J. Math. 221 2017, 489–510.Google Scholar
Dick, J. and Pillichshammer, F., Digital Nets and Sequences: Discrepancy Theory and Quasi-Monte Carlo Integration, Cambridge University Press (Cambridge, 2010).CrossRefGoogle Scholar
Dick, J. and Pillichshammer, F., Optimal L2 discrepancy bounds for higher order digital sequences over the finite field F2 . Acta Arith. 162 2014, 65–99.CrossRefGoogle Scholar
Dick, J. and Pillichshammer, F., Explicit constructions of point sets and sequences with low discrepancy. In Uniform Distribution and Quasi-Monte Carlo Methods – Discrepancy, Integration and Applications (Radon Series of Computational and Applied Mathematics) (eds Kritzer, P., Niederreiter, H., Pillichshammer, F. and Winterhof, A.), De Gruyter (Berlin/Boston, 2014), 63–86.CrossRefGoogle Scholar
Drmota, M. and Tichy, R. F., Sequences, Discrepancies and Applications (Lecture Notes in Mathematics 1651 ), Springer (Berlin, 1997).CrossRefGoogle Scholar
Hinrichs, A., Discrepancy of Hammersley points in Besov spaces of dominating mixed smoothness. Math. Nachr. 283 2010, 478–488.CrossRefGoogle Scholar
Kritzinger, R., L p - and S p, q r B-discrepancy of the symmetrized van der Corput sequence and modified Hammersley point sets in arbitrary bases. J. Complexity 33 2016, 145–168.CrossRefGoogle Scholar
Kuipers, L. and Niederreiter, H., Uniform Distribution of Sequences, John Wiley (New York, NY, 1974) , reprinted by Dover (Mineola, NY, 2006).Google Scholar
Leobacher, G. and Pillichshammer, F., Introduction to Quasi-Monte Carlo Integration and Applications (Compact Textbooks in Mathematics), Birkhäuser/Springer (Cham, 2014).Google Scholar
Lindenstrauss, J. and Tzafriri, L., Classical Banach Spaces, I, Springer (Berlin, 1977).Google Scholar
Markhasin, L., Discrepancy of generalized Hammersley type point sets in Besov spaces with dominating mixed smoothness. Unif. Distrib. Theory 8 2013, 135–164.Google Scholar
Markhasin, L., Discrepancy and integration in function spaces with dominating mixed smoothness. Dissertationes Math. (Rozprawy Mat.) 494 2013, 1–81.CrossRefGoogle Scholar
Markhasin, L., Quasi-Monte Carlo methods for integration of functions with dominating mixed smoothness in arbitrary dimension. J. Complexity 29 2013, 370–388.Google Scholar
Markhasin, L., L p - and S p, q r B-discrepancy of (order 2) digital nets. Acta Arith. 168 2015, 139–159.Google Scholar
Matoušek, J., Geometric Discrepancy. An Illustrated Guide (Algorithms and Combinatorics 18 ), Springer (Berlin, 1999).CrossRefGoogle Scholar
Niederreiter, H., Point sets and sequences with small discrepancy. Monatsh. Math. 104 1987, 273–337.CrossRefGoogle Scholar
Niederreiter, H., Random Number Generation and Quasi-Monte Carlo Methods (CBMS-NSF Regional Conference Series in Applied Mathematics 63 ), SIAM (Philadelphia, 1992).CrossRefGoogle Scholar
Niederreiter, H. and Xing, C. P., Low-discrepancy sequences and global function fields with many rational places. Finite Fields Appl. 2 1996, 241–273.Google Scholar
Proinov, P. D., On irregularities of distribution. C. R. Acad. Bulgare Sci. 39 1986, 31–34.Google Scholar
Roth, K. F., On irregularities of distribution. Mathematika 1 1954, 73–79.CrossRefGoogle Scholar
Roth, K. F., On irregularities of distribution. IV. Acta Arith. 37 1980, 67–75.Google Scholar
Schmidt, W. M., Irregularities of distribution. VII. Acta Arith. 21 1972, 45–50.CrossRefGoogle Scholar
Schmidt, W. M., Irregularities of distribution X. In Number Theory and Algebra, Academic Press (New York, NY, 1977), 311–329.Google Scholar
Skriganov, M. M., Harmonic analysis on totally disconnected groups and irregularities of point distributions. J. Reine Angew. Math. 600 2006, 25–49.Google Scholar
Sobol’, I. M., The distribution of points in a cube and the approximate evaluation of integrals. Zh. Vychisl. Mat. Mat. Fiz. 7 1967, 784–802.Google Scholar
Tao, T., A type diagram for function spaces. What’s new – a blog by T. Tao, 11 March 2010, https://terrytao.wordpress.com/tag/besov-spaces.Google Scholar
Tezuka, S., Polynomial arithmetic analogue of Halton sequences. ACM Trans. Model. Comput. Simul. 3 1993, 99–107.Google Scholar
Triebel, H., Bases in Function Spaces, Sampling, Discrepancy, Numerical Integration, European Mathematical Society (Zürich, 2010).CrossRefGoogle Scholar
Triebel, H., Numerical integration and discrepancy. A new approach. Math. Nachr. 283 2010, 139–159.CrossRefGoogle Scholar