Hostname: page-component-77c89778f8-cnmwb Total loading time: 0 Render date: 2024-07-17T08:22:43.689Z Has data issue: false hasContentIssue false
  • English
  • Français

On the minimal dimension of a homology sphere on which a finite group acts

Published online by Cambridge University Press:  01 March 2008

BRUNO P. ZIMMERMANN
BRUNO P. ZIMMERMANN*
Affiliation:
Università degli Studi di Trieste, Dipartimento di Matematica e Informatica, 34100 Trieste, Italy. e-mail: zimmer@units.it
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that the minimal dimension of a faithful action of a metacyclic group 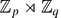, for primes p and q, on a homology sphere coincides with the minimal dimension of a faithful linear action on a sphere; as a consequence, we obtain the analogous result for various finite simple groups.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 144 , Issue 2 , March 2008 , pp. 397 - 401
Copyright
Copyright © Cambridge Philosophical Society 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[Bo]Borel, A.. Seminar on transformation groups. Ann. Math. Stud. 46 (Princeton University Press, 1960).CrossRefGoogle Scholar
[Br]Bredon, G.. Introduction to Compact Transformation Groups (Academic Press, 1972).Google Scholar
[Bw]Brown, K. S.. Cohomology of groups. Graduate Texts in Mathematics 87 (Springer, 1982).Google Scholar
[C]Conway, J. H., Curtis, R. T., Norton, S. P., Parker, R. A. and Wilson, R. A.. Atlas of Finite Groups (Oxford University Press, 1985).Google Scholar
[CL]Cooper, D. and Long, D. D.. Free actions of finite groups on rational homology 3-spheres. Topol. Appl. 101 (2000), 143–148.CrossRefGoogle Scholar
[D]Dotzel, R. M.. Orientation preserving actions of finite abelian groups on spheres. Proc. Amer. Math. Soc. 100 (1987), 159–163.Google Scholar
[DH]Dotzel, R. M. and Hamrick, G. C.. p-group actions on homology spheres. Invent. Math. 62 (1981), 437–442.CrossRefGoogle Scholar
[E1]Edmonds, A. L.. Aspects of group actions on four-manifolds. Topol. Appl. 31 (1989), 109–124.CrossRefGoogle Scholar
[E2]Edmonds, A. L.. Homologically trivial group actions on 4-manifolds. Electronic version available in arXiv:math.GT/9809055.Google Scholar
[Mc]McCooey, M. P.. Symmetry groups of 4-manifolds. Topology 41 (2002), 835–851.CrossRefGoogle Scholar
[MeZ1]Mecchia, M. and Zimmermann, B.. On finite groups acting on ℤ2-homology 3-spheres. Math. Z. 248 (2004), 675–693.CrossRefGoogle Scholar
[MeZ2]Mecchia, M. and Zimmermann, B.. On finite simple groups acting on integer and mod 2 homology 3-spheres. J. Algebra 298 (2006), 460–467.CrossRefGoogle Scholar
[MeZ3]Mecchia, M. and Zimmermann, B.. On finite simple and nonsolvable groups acting on homology 4-spheres. Topology Appl. 153 (2006), 2933–2942.CrossRefGoogle Scholar
[Mg]Milgram, R. J.. Evaluating the Swan finiteness obstruction for finite groups. Algebraic and Geometric Topology. Lecture Notes in Math. 1126 (Springer, 1985), 127–158.Google Scholar
[Mn]Milnor, J.. Groups which act on S n without fixed points. Amer. J. Math. 79 (1957), 623–630.Google Scholar
[Z1]Zimmermann, B.. Some results and conjectures on finite groups acting on homology spheres. Sib. Elektron. Mat. Izv. 2 (2005), 233–288 (http://semr.math.nsc.ru).Google Scholar
[Z2]Zimmermann, B.. Cyclic branched coverings and homology 3-spheres with large group actions. Fund. Math. 184 (2004), 343–353.CrossRefGoogle Scholar
[Z3]Zimmermann, B.. On the classification of finite groups acting on homology 3-spheres. Pacific J. Math. 217 (2004), 387–395.CrossRefGoogle Scholar