Article contents
Linear bounds on characteristic polynomials of matroids
Published online by Cambridge University Press: 10 January 2019
Abstract
Let χ(t) = a0tn – a1tn−1 + ⋯ + (−1)rartn−r be the chromatic polynomial of a graph, the characteristic polynomial of a matroid, or the characteristic polynomial of an arrangement of hyperplanes. For any integer k = 0, 1, …, r and real number x ⩾ k − r − 1, we obtain a linear bound of the coefficient sequence, that is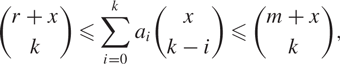 \begin{align*} {r+x\choose k}\leqslant \sum_{i=0}^{k}a_{i}{x\choose k-i}\leqslant {m+x\choose k}, \end{align*}
\begin{align*} {r+x\choose k}\leqslant \sum_{i=0}^{k}a_{i}{x\choose k-i}\leqslant {m+x\choose k}, \end{align*}
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 168 , Issue 3 , May 2020 , pp. 505 - 518
- Copyright
- Copyright © Cambridge Philosophical Society 2019
Footnotes
This work was supported by the National Natural Science Foundation of China (11871204).
References
REFERENCES
- 1
- Cited by




