Article contents
REDUCTIONS OF POINTS ON ALGEBRAIC GROUPS
Published online by Cambridge University Press: 14 November 2019
Abstract
Let  $A$ be the product of an abelian variety and a torus defined over a number field
$A$ be the product of an abelian variety and a torus defined over a number field 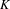 $K$. Fix some prime number
$K$. Fix some prime number 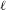 $\ell$. If
$\ell$. If 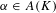 $\unicode[STIX]{x1D6FC}\in A(K)$ is a point of infinite order, we consider the set of primes
$\unicode[STIX]{x1D6FC}\in A(K)$ is a point of infinite order, we consider the set of primes  $\mathfrak{p}$ of
$\mathfrak{p}$ of 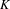 $K$ such that the reduction
$K$ such that the reduction 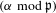 $(\unicode[STIX]{x1D6FC}\hspace{0.2em}{\rm mod}\hspace{0.2em}\mathfrak{p})$ is well-defined and has order coprime to
$(\unicode[STIX]{x1D6FC}\hspace{0.2em}{\rm mod}\hspace{0.2em}\mathfrak{p})$ is well-defined and has order coprime to 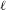 $\ell$. This set admits a natural density. By refining the method of Jones and Rouse [Galois theory of iterated endomorphisms, Proc. Lond. Math. Soc. (3)100(3) (2010), 763–794. Appendix A by Jeffrey D. Achter], we can express the density as an
$\ell$. This set admits a natural density. By refining the method of Jones and Rouse [Galois theory of iterated endomorphisms, Proc. Lond. Math. Soc. (3)100(3) (2010), 763–794. Appendix A by Jeffrey D. Achter], we can express the density as an  $\ell$-adic integral without requiring any assumption. We also prove that the density is always a rational number whose denominator (up to powers of
$\ell$-adic integral without requiring any assumption. We also prove that the density is always a rational number whose denominator (up to powers of  $\ell$) is uniformly bounded in a very strong sense. For elliptic curves, we describe a strategy for computing the density which covers every possible case.
$\ell$) is uniformly bounded in a very strong sense. For elliptic curves, we describe a strategy for computing the density which covers every possible case.
Keywords
MSC classification
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 20 , Issue 5 , September 2021 , pp. 1637 - 1669
- Copyright
- © Cambridge University Press 2019
References
- 4
- Cited by



