Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-17T19:27:22.320Z Has data issue: false hasContentIssue false
  • English
  • Français

A VARIANT OF HARISH-CHANDRA FUNCTORS

Part of: Representation theory of groups Lie groups Linear algebraic groups and related topics

Published online by Cambridge University Press:  14 August 2017

Tyrone Crisp ,
Ehud Meir  and
Uri Onn
Tyrone Crisp
Affiliation:
Department of Mathematics, Radboud University Nijmegen, PO Box 9010, 6500 GL Nijmegen, The Netherlands (t.crisp@math.ru.nl)
Ehud Meir
Affiliation:
Department of Mathematics, University of Hamburg, Bundesstr. 55, 20146 Hamburg, Germany (meirehud@gmail.com)
Uri Onn
Affiliation:
Department of Mathematics, Ben Gurion University of the Negev, Beer-Sheva 84105, Israel (urionn@math.bgu.ac.il)
Get access Rights & Permissions [Opens in a new window]

Abstract

Harish-Chandra induction and restriction functors play a key role in the representation theory of reductive groups over finite fields. In this paper, extending earlier work of Dat, we introduce and study generalisations of these functors which apply to a wide range of finite and profinite groups, typical examples being compact open subgroups of reductive groups over non-archimedean local fields. We prove that these generalisations are compatible with two of the tools commonly used to study the (smooth, complex) representations of such groups, namely Clifford theory and the orbit method. As a test case, we examine in detail the induction and restriction of representations from and to the Siegel Levi subgroup of the symplectic group 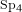$\text{Sp}_{4}$ over a finite local principal ideal ring of length two. We obtain in this case a Mackey-type formula for the composition of these induction and restriction functors which is a perfect analogue of the well-known formula for the composition of Harish-Chandra functors. In a different direction, we study representations of the Iwahori subgroup $I_{n}$ of 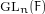$\text{GL}_{n}(F)$, where 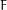$F$ is a non-archimedean local field. We establish a bijection between the set of irreducible representations of 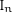$I_{n}$ and tuples of primitive irreducible representations of smaller Iwahori subgroups, where primitivity is defined by the vanishing of suitable restriction functors.

Keywords

Harish-Chandra inductionparabolic inductioncompact p-adic groupsrepresentations of profinite groups

MSC classification

Primary: 22E50: Representations of Lie and linear algebraic groups over local fields
Secondary: 20G25: Linear algebraic groups over local fields and their integers 20C33: Representations of finite groups of Lie type 20C15: Ordinary representations and characters 20C07: Group rings of infinite groups and their modules
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 18 , Issue 5 , September 2019 , pp. 993 - 1049
Copyright
© Cambridge University Press 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Baumgartner, U. and Willis, G. A., Contraction groups and scales of automorphisms of totally disconnected locally compact groups, Israel J. Math. 142 (2004), 221–248.Google Scholar
Bernstein, J. N., Le ‘centre’ de Bernstein, in Representations of Reductive Groups Over a Local Field, Travaux en Cours (ed. Deligne, P.), pp. 1–32 (Hermann, Paris, 1984).Google Scholar
Bernstein, J. N. and Zelevinsky, A. V., Representations of the group GL (n, F), where F is a local non-Archimedean field, Uspehi Mat. Nauk 31 (1976), 5–70.Google Scholar
Boyarchenko, M. and Sabitova, M., The orbit method for profinite groups and a p-adic analogue of Brown’s theorem, Israel J. Math. 165 (2008), 67–91.Google Scholar
Casselman, W., The restriction of a representation of GL2(k) to GL2(𝔬), Math. Ann. 206 (1973), 311–318.Google Scholar
Chen, Z. and Stasinski, A., The algebraisation of higher Deligne–Lusztig representations, Sel. Math. New Ser. (2017). https://doi.org/10.1007/s00029-017-0349-z.Google Scholar
Crisp, T., Parahoric induction and chamber homology for SL2 , J. Lie Theory 25 (2015), 657–676.Google Scholar
Crisp, T., Meir, E. and Onn, U., Induced representations of $\text{GL}_{n}({\mathcal{O}})$ . In preparation, 2017.Google Scholar
Crisp, T., Meir, E. and Onn, U., Principal series for general linear groups over finite commutative rings, Preprint, 2017, arXiv:1704.05575 [math.RT].Google Scholar
Dat, J.-F., Finitude pour les représentations lisses de groupes p-adiques, J. Inst. Math. Jussieu 8 (2009), 261–333.Google Scholar
Deligne, P., Le support du caractère d’une représentation supercuspidale, C. R. Acad. Sci. Paris Sér. A-B 283 (1976), Aii, A155–A157.Google Scholar
Deligne, P. and Lusztig, G., Representations of reductive groups over finite fields, Ann. of Math. (2) 103 (1976), 103–161.Google Scholar
Digne, F. and Michel, J., Representations of Finite Groups of Lie Type, London Mathematical Society Student Texts, Volume 21 (Cambridge University Press, Cambridge, 1991).Google Scholar
Dixon, J. D., du Sautoy, M. P. F., Mann, A. and Segal, D., Analytic Pro-p Groups, 2nd edn, Cambridge Studies in Advanced Mathematics, Volume 61 (Cambridge University Press, Cambridge, 1999).Google Scholar
Glöckner, H., Scale functions on p-adic Lie groups, Manuscripta Math. 97 (1998), 205–215.Google Scholar
Glöckner, H., Contraction groups for tidy automorphisms of totally disconnected groups, Glasg. Math. J. 47 (2005), 329–333.Google Scholar
Harish-Chandra, Eisenstein series over finite fields, in Functional Analysis and Related fields (Proc. Conf. M. Stone, Univ. Chicago, Chicago, Ill., 1968, pp. 76–88(Springer, New York, 1970).Google Scholar
Hill, G., A Jordan decomposition of representations for GLn(O), Comm. Algebra 21 (1993), 3529–3543.Google Scholar
Hill, G., On the nilpotent representations of GLn(𝓞), Manuscripta Math. 82 (1994), 293–311.Google Scholar
Hill, G., Regular elements and regular characters of GLn(𝓞), J. Algebra 174 (1995), 610–635.Google Scholar
Hill, G., Semisimple and cuspidal characters of GLn(𝓞), Comm. Algebra 23 (1995), 7–25.Google Scholar
Howe, R., Kirillov theory for compact p-adic groups, Pacific J. Math. 73 (1977), 365–381.Google Scholar
Howe, R., Harish-Chandra homomorphisms for 𝔭-adic groups, CBMS Regional Conference Series in Mathematics, Volume 59 (American Mathematical Society, Providence, RI, 1985). Published for the Conference Board of the Mathematical Sciences, Washington, DC. With the collaboration of Allen Moy.Google Scholar
Howlett, R. B. and Lehrer, G. I., Induced cuspidal representations and generalised Hecke rings, Invent. Math. 58 (1980), 37–64.Google Scholar
Howlett, R. B. and Lehrer, G. I., On Harish-Chandra induction and restriction for modules of Levi subgroups, J. Algebra 165 (1994), 172–183.Google Scholar
Iwahori, N. and Matsumoto, H., On some Bruhat decomposition and the structure of the Hecke rings of 𝔭-adic Chevalley groups, Publ. Math. Inst. Hautes Études Sci. 25 (1965), 5–48.Google Scholar
Jaikin-Zapirain, A., Zeta function of representations of compact p-adic analytic groups, J. Amer. Math. Soc. 19 (2006), 91–118 (electronic).Google Scholar
Karpilovsky, G., Group Representations, Vol. 2, North-Holland Mathematics Studies, Volume 177 (North-Holland Publishing Company, Amsterdam, 1993).Google Scholar
Khukhro, E. I., p-Automorphisms of Finite p-Groups, London Mathematical Society Lecture Note Series, Volume 246 (Cambridge University Press, Cambridge, 1998).Google Scholar
Lazard, M., Sur les groupes nilpotents et les anneaux de Lie, Ann. Sci. Éc. Norm. Supér. 71 (1954), 101–190.Google Scholar
Lazard, M., Groupes analytiques p-adiques, Publ. Math. Inst. Hautes Études Sci. 26 (1965), 389–603.Google Scholar
van Leeuwen, M. A., An application of Hopf-algebra techniques to representations of finite classical groups, J. Algebra 140 (1991), 210–246.Google Scholar
Lusztig, G., Characters of Reductive Groups Over a Finite Field, Annals of Mathematics Studies, Volume 107 (Princeton University Press, Princeton, NJ, 1984).Google Scholar
Lusztig, G., Representations of reductive groups over finite rings, Represent. Theory 8 (2004), 1–14.Google Scholar
Lusztig, G., Generic character sheaves on groups over k[𝜖]/(𝜖 r ), in Categorification and Higher Representation Theory, Contemporary Mathematics, Volume 683, pp. 227–246 (2017).Google Scholar
Onn, U., Representations of automorphism groups of finite 𝔬-modules of rank two, Adv. Math. 219 (2008), 2058–2085.Google Scholar
Onn, U. and Singla, P., On the unramified principal series of GL(3) over non-Archimedean local fields, J. Algebra 397 (2014), 1–17.Google Scholar
Renard, D., Représentations des groupes réductifs p-adiques, Cours Spécialisés [Specialized Courses], Volume 17 (Société Mathématique de France, Paris, 2010).Google Scholar
Schneider, P. and Stuhler, U., Representation theory and sheaves on the Bruhat–Tits building, Publ. Math. Inst. Hautes Études Sci. 85 (1997), 97–191.Google Scholar
Singla, P., On representations of general linear groups over principal ideal local rings of length two, J. Algebra 324 (2010), 2543–2563.Google Scholar
Springer, T. A., Cusp forms for finite groups, in Seminar on Algebraic Groups and Related Finite Groups (The Institute for Advanced Study, Princeton, NJ, 1968/69), Lecture Notes in Mathematics, Volume 131, pp. 97–120 (Springer, Berlin, 1970).Google Scholar
Srinivasan, B., The characters of the finite symplectic group Sp(4, q), Trans. Amer. Math. Soc. 131 (1968), 488–525.Google Scholar
Stasinski, A., The smooth representations of GL2(𝔬), Comm. Algebra 37 (2009), 4416–4430.Google Scholar
Stasinski, A., Extended Deligne-Lusztig varieties for general and special linear groups, Adv. Math. 226 (2011), 2825–2853.Google Scholar
Wang, J. S. P., The Mautner phenomenon for p-adic Lie groups, Math. Z. 185 (1984), 403–412.Google Scholar
Zelevinsky, A. V., Representations of Finite Classical Groups, Lecture Notes in Mathematics, Volume 869 (Springer, Berlin–New York, 1981). A Hopf algebra approach.Google Scholar