Article contents
SUM THEOREMS FOR MAXIMALLY MONOTONE OPERATORS OF TYPE (FPV)
Published online by Cambridge University Press: 08 July 2014
Abstract
The most important open problem in monotone operator theory concerns the maximal monotonicity of the sum of two maximally monotone operators provided that the classical Rockafellar’s constraint qualification holds. In this paper, we establish the maximal monotonicity of 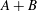 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}A+B$ provided that
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}A+B$ provided that 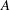 $A$ and
$A$ and 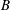 $B$ are maximally monotone operators such that
$B$ are maximally monotone operators such that 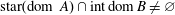 ${\rm star}({\rm dom}\ A)\cap {\rm int}\, {\rm dom}\, B\neq \varnothing $, and
${\rm star}({\rm dom}\ A)\cap {\rm int}\, {\rm dom}\, B\neq \varnothing $, and 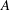 $A$ is of type (FPV). We show that when also
$A$ is of type (FPV). We show that when also 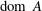 ${\rm dom}\ A$ is convex, the sum operator
${\rm dom}\ A$ is convex, the sum operator 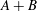 $A+B$ is also of type (FPV). Our result generalizes and unifies several recent sum theorems.
$A+B$ is also of type (FPV). Our result generalizes and unifies several recent sum theorems.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © 2014 Australian Mathematical Publishing Association Inc.
References
- 1
- Cited by




