Article contents
Effects of mechanical properties on the contact profile in Berkovich nanoindentation of elastoplastic materials
Published online by Cambridge University Press: 17 November 2011
Abstract
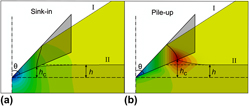
Pile-up or sink-in is always a concern in a nanoindentation test because it gives rise to errors in the estimation of the projected contact area when it is theoretically analyzed with the classic Oliver–Pharr method. In this study, a three-dimensional finite element model is developed to simulate nanoindentation with a perfect Berkovich tip. The variation of the contact profile with respect to the work-hardening rate n and the ratio of yield stress to elastic modulus σy/E has been studied for a wide range of elastoplastic materials. The numerical results show that a low σy/E not only facilitates the pile-up for materials with little or no work-hardening but also enhances the sink-in for materials with a high work-hardening rate. It is attributed to the lateral-flow dominated plastic deformation in low work-hardening materials and the normal-flow dominated plastic deformation in high work-hardening materials, respectively. Because of the large sink-in, for the materials with high n and low σy/E, significant errors in the calculation of the projected contact area can be generated by using the classic Oliver–Pharr method.
Keywords
- Type
- Articles
- Information
- Journal of Materials Research , Volume 27 , Issue 1: Focus Issue: Instrumented Indentation , 14 January 2012 , pp. 313 - 319
- Copyright
- Copyright © Materials Research Society 2011
References
REFERENCES
- 9
- Cited by


