Hostname: page-component-8448b6f56d-xtgtn Total loading time: 0 Render date: 2024-04-18T22:20:38.644Z Has data issue: false hasContentIssue false
  • English
  • Français

Tilting at wave beams: a new perspective on the St. Andrew’s Cross

Published online by Cambridge University Press:  05 October 2017

T. Kataoka Open the ORCID record for T. Kataoka [Opens in a new window] ,
S. J. Ghaemsaidi ,
N. Holzenberger ,
T. Peacock  and
T. R. Akylas
T. Kataoka
Affiliation:
Department of Mechanical Engineering, Graduate School of Engineering, Kobe University, Rokkodai, Nada, Kobe 657-8501, Japan
S. J. Ghaemsaidi
Affiliation:
Department of Mechanical Engineering, Massachusetts Institute of Technology, Cambridge, MA 02139, USA
N. Holzenberger
Affiliation:
Department of Mechanical Engineering, Massachusetts Institute of Technology, Cambridge, MA 02139, USA
T. Peacock
Affiliation:
Department of Mechanical Engineering, Massachusetts Institute of Technology, Cambridge, MA 02139, USA
T. R. Akylas*
Affiliation:
Department of Mechanical Engineering, Massachusetts Institute of Technology, Cambridge, MA 02139, USA
*
†Email address for correspondence: trakylas@mit.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

The generation of internal gravity waves by a vertically oscillating cylinder that is tilted to the horizontal in a stratified Boussinesq fluid of constant buoyancy frequency, $N$, is investigated. This variant of the widely studied horizontal configuration – where a cylinder aligned with a plane of constant gravitational potential induces four wave beams that emanate from the cylinder, forming a cross pattern known as the ‘St. Andrew’s Cross’ – brings out certain unique features of radiated internal waves from a line source tilted to the horizontal. Specifically, simple kinematic considerations reveal that for a cylinder inclined by a given angle 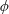$\unicode[STIX]{x1D719}$ to the horizontal, there is a cutoff frequency, 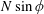$N\sin \unicode[STIX]{x1D719}$, below which there is no longer a radiated wave field. Furthermore, three-dimensional effects due to the finite length of the cylinder, which are minor in the horizontal configuration, become a significant factor and eventually dominate the wave field as the cutoff frequency is approached; these results are confirmed by supporting laboratory experiments. The kinematic analysis, moreover, suggests a resonance phenomenon near the cutoff frequency as the group-velocity component perpendicular to the cylinder direction vanishes at cutoff; as a result, energy cannot be easily radiated away from the source, and nonlinear and viscous effects are likely to come into play. This scenario is examined by adapting the model for three-dimensional wave beams developed in Kataoka & Akylas (J. Fluid Mech., vol. 769, 2015, pp. 621–634) to the near-resonant wave field due to a tilted line source of large but finite length. According to this model, the combination of three-dimensional, nonlinear and viscous effects near cutoff triggers transfer of energy, through the action of Reynolds stresses, to a circulating horizontal mean flow. Experimental evidence of such an induced mean flow near cutoff is also presented.

JFM classification

Geophysical and Geological Flows: Geophysical and Geological Flows Geophysical and Geological Flows: Internal waves
Type
Papers
Information
Journal of Fluid Mechanics , Volume 830 , 10 November 2017 , pp. 660 - 680
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Akylas, T. R. 1984 On the excitation of nonlinear water waves by a moving pressure distribution oscillating at resonant frequency. Phys. Fluids 27, 2803–2807.Google Scholar
Akylas, T. R., Grimshaw, R. H. J., Clarke, S. R. & Tabaei, A. 2007 Reflecting tidal wave beams and local generation of solitary waves in the ocean thermocline. J. Fluid Mech. 593, 297–313.Google Scholar
Akylas, T. R. & Karimi, H. H. 2012 Oblique collisions of internal wave beams and associated resonances. J. Fluid Mech. 711, 337–363.Google Scholar
Alexander, M. J., Holton, J. R. & Durran, D. R. 1995 The gravity wave response above deep convection in a squall line simulation. J. Atmos. Sci. 52, 2212–2226.Google Scholar
Appleby, J. C. & Crighton, D. G. 1986 Non-Boussinesq effects in the diffraction of internal waves from an oscillating cylinder. Q. J. Mech. Appl. Maths 39, 209–231.Google Scholar
Aranha, J. A., Yue, D. K. P. & Mei, C. C. 1982 Nonlinear waves near a cut-off frequency in an acoustic duct – a numerical study. J. Fluid Mech. 121, 465–478.Google Scholar
Bordes, G., Venaille, A., Joubaud, S., Odier, P. & Dauxois, T. 2012 Experimental observation of a strong mean flow induced by internal gravity waves. Phys. Fluids 24, 086602.Google Scholar
Cole, S. T., Rudnick, D. L., Hodges, B. A. & Martin, J. P. 2009 Observations of tidal internal wave beams at Kauai Channel, Hawaii. J. Phys. Oceanogr. 39, 421–436.Google Scholar
Dalziel, S. B. 2000 Synthetic schlieren measurements of internal waves generated by oscillating a square cylinder. In Proc. 5th Intl Symp. on Stratified Flows, Vancouver, Canada, 10–13, July 2000 (ed. Lawrence, G. A., Pieters, R. & Yonemitsu, N.), vol. 2, pp. 743–748. University of British Columbia.Google Scholar
Dewan, E. M., Picard, R. H., O’Neil, R. R., Gardiner, H. A. & Gibson, J. 1998 MSX satellite observations of thunderstorm-generated gravity waves in mid-wave infrared images of the upper stratosphere. Geophys. Res. Lett. 25, 939–942.Google Scholar
Ermanyuk, E. V. 2000 The use of impulse response functions for evaluation of added mass and damping coefficient of a circular cylinder oscillating in linearly stratified fluid. Exp. Fluids 28, 152–159.Google Scholar
Ermanyuk, E. V. & Gavrilov, N. V. 2002 Force on a body in a continuously stratified fluid. Part 1. Circular cylinder. J. Fluid Mech. 451, 421–443.CrossRefGoogle Scholar
Ermanyuk, E. V. & Gavrilov, N. V. 2005 Duration of transient processes in the formation of internal-wave beams. Dokl. Phys. 50, 548–550.CrossRefGoogle Scholar
Ermanyuk, E. V. & Gavrilov, N. V. 2008 On internal waves generated by large-amplitude circular and rectilinear oscillations of a circular cylinder in a uniformly stratified fluid. J. Fluid Mech. 613, 329–356.Google Scholar
Flynn, M. R., Onu, K. & Sutherland, B. R. 2003 Internal wave excitation by a vertically oscillating sphere. J. Fluid Mech. 494, 65–93.Google Scholar
Fortuin, J. M. H. 1960 Theory and application of two supplementary methods of constructing density gradient columns. J. Polym. Sci. 44, 505–515.CrossRefGoogle Scholar
Fovell, R., Durran, D. & Holton, J. R. 1992 Numerical simulations of convectively generated stratospheric gravity waves. J. Atmos. Sci. 49, 1427–1442.Google Scholar
Gavrilov, N. V. & Ermanyuk, E. V. 1997 Internal waves generated by circular translational motion of a cylinder in a linearly stratified fluid. J. Appl. Mech. Tech. Phys. 38, 224–227.Google Scholar
Ghaemsaidi, S. J. & Peacock, T. 2013 3D Stereoscopic PIV visualization of the axisymmetric conical internal wave field generated by an oscillating sphere. Exp. Fluids 54, 1454.CrossRefGoogle Scholar
Gordon, D. & Stevenson, T. N. 1972 Viscous effects in a vertically propagating internal wave. J. Fluid Mech. 56, 629–639.CrossRefGoogle Scholar
Görtler, H. 1943 On an oscillatory phenomenon in fluids of a stable distribution of density. Z. Angew. Math. Mech. 23, 65–71.Google Scholar
Gostiaux, L. & Dauxois, T. 2007 Laboratory experiments on the generation of internal tidal beams over steep slopes. Phys. Fluids 19, 028102.Google Scholar
Grisouard, N., Staquet, C. & Gerkema, T. 2011 Generation of internal solitary waves in a pycnocline by an internal wave beam: a numerical study. J. Fluid Mech. 676, 491–513.Google Scholar
Holbrook, W. S. & Fer, I. 2005 Ocean internal wave spectra inferred from seismic reflection transects. Geophys. Res. Lett. 32, L15604.CrossRefGoogle Scholar
Hurley, D. G. 1997 The generation of internal waves by vibrating elliptic cylinders. Part 1. Inviscid solution. J. Fluid Mech. 351, 105–118.Google Scholar
Hurley, D. G. & Keady, G. 1997 The generation of internal waves by vibrating elliptic cylinders. Part 2. Approximate viscous solution. J. Fluid Mech. 351, 119–138.CrossRefGoogle Scholar
Jiang, C.-H. & Marcus, P. S. 2009 Selection rules for the nonlinear interaction of internal gravity waves. Phys. Rev. Lett. 102, 124502.Google Scholar
Kataoka, T. & Akylas, T. R. 2015 On three-dimensional internal gravity wave beams and induced large-scale mean flows. J. Fluid Mech. 769, 621–634.Google Scholar
Lamb, H. 1932 Hydrodynamics, Art 235. Cambridge University Press.Google Scholar
Lane, T. P., Reeder, M. J. & Clark, T. L. 2001 Numerical modeling of gravity wave generation by deep tropical convection. J. Atmos. Sci. 58, 1249–1274.Google Scholar
Lien, R.-C. & Gregg, M. C. 2001 Observations of turbulence in a tidal beam and across a coastal ridge. J. Geophys. Res. 106, 4575–4591.Google Scholar
Lighthill, M. J. 1965 Group velocity. J. Inst. Maths Applics. 1, 1–28.CrossRefGoogle Scholar
Makarov, S. A., Neklyudov, V. I. & Chashechkin, Y. D. 1990 Spatial structure of two-dimensional monochromatic internal-wave beams in an exponentially stratified liquid. Izv. Atmos. Ocean. Phys. 26, 548–554.Google Scholar
Merzkirch, W. & Peters, F. 1992 Optical visualization of internal gravity waves in stratified fluid. Optics Lasers Engng 16, 411–425.Google Scholar
Mowbray, D. E. & Rarity, B. S. H. 1967 A theoretical and experimental investigation of the phase configuration of internal waves of small amplitude in a density stratified fluid. J. Fluid Mech. 28, 1–16.Google Scholar
Oster, G. 1965 Density gradients. Sci. Am. 213, 70–76.Google Scholar
Peacock, T. & Tabaei, A. 2005 Visualization of nonlinear effects in reflecting internal wave beams. Phys. Fluids 17, 061702.CrossRefGoogle Scholar
Peacock, T. & Weidman, P. 2005 The effect of rotation on conical wave beams in a stratified fluid. Exp. Fluids 39, 32–37.CrossRefGoogle Scholar
Peters, F. 1985 Schlieren interferometry applied to a gravity wave in a density-stratified liquid. Exp. Fluids 3, 261–269.CrossRefGoogle Scholar
Piani, C., Durran, D., Alexander, M. J. & Holton, J. R. 2000 A numerical study of three-dimensional gravity waves triggered by deep tropical convection and their role in the dynamics of the QBO. J. Atmos. Sci. 57, 3689–3702.Google Scholar
Pingree, R. D. & New, A. L. 1989 Downward propagation of internal tidal energy into the Bay of Biscay. Deep-Sea Res. 36, 735–758.Google Scholar
Rodenborn, B., Kiefer, D., Zhang, H. P. & Swinney, H. L. 2011 Harmonic generation by reflecting internal waves. Phys. Fluids 23, 026601.Google Scholar
Smith, S. & Crockett, J. 2014 Experiments on nonlinear harmonic wave generation from colliding internal wave beams. Exp. Therm. Fluid Sci. 54, 93–101.Google Scholar
Sutherland, B. R., Dalziel, S. B., Hughes, G. O. & Linden, P. F. 1999 Visualization and measurement of internal waves by ‘synthetic schlieren’. Part 1. Vertically oscillating cylinder. J. Fluid Mech. 390, 93–126.Google Scholar
Sutherland, B. R., Hughes, G. O., Dalziel, S. B. & Linden, P. F. 2000 Internal waves revisited. Dyn. Atmos. Oceans 31, 209–232.Google Scholar
Sutherland, B. R. & Linden, P. F. 2002 Internal wave excitation by a vertically oscillating elliptical cylinder. Phys. Fluids 14, 721–731.Google Scholar
Tabaei, A., Akylas, T. R. & Lamb, K. G. 2005 Nonlinear effects in reflecting and colliding internal wave beams. J. Fluid Mech. 526, 217–243.Google Scholar
Thomas, N. H. & Stevenson, T. N. 1972 A similarity solution for viscous internal waves. J. Fluid Mech. 54, 495–506.Google Scholar
Voisin, B. 1991 Internal wave generation in uniformly stratified fluids. Part 1. Green’s function and point sources. J. Fluid Mech. 231, 439–480.Google Scholar
Voisin, B. 2003 Limit states of internal wave beams. J. Fluid Mech. 496, 243–293.CrossRefGoogle Scholar
Voisin, B., Ermanyuk, E. V. & Flor, J. B. 2011 Internal wave generation by oscillation of a sphere, with application to internal tides. J. Fluid Mech. 666, 308–357.Google Scholar
Yih, C.-S. 1965 Dynamics of Nonhomogeneous Fluids, chap. 2. Macmillan.Google Scholar