Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bauerheim, M.
Nicoud, F.
and
Poinsot, T.
2016.
Progress in analytical methods to predict and control azimuthal combustion instability modes in annular chambers.
Physics of Fluids,
Vol. 28,
Issue. 2,
Bauerheim, M.
Ndiaye, A.
Constantine, P.
Moreau, S.
and
Nicoud, F.
2016.
Symmetry breaking of azimuthal thermoacoustic modes: the UQ perspective.
Journal of Fluid Mechanics,
Vol. 789,
Issue. ,
p.
534.
Magri, Luca
Bauerheim, Michael
and
Juniper, Matthew P.
2016.
Stability analysis of thermo-acoustic nonlinear eigenproblems in annular combustors. Part I. Sensitivity.
Journal of Computational Physics,
Vol. 325,
Issue. ,
p.
395.
Ghirardo, G.
Juniper, M. P.
and
Moeck, J. P.
2016.
Weakly nonlinear analysis of thermoacoustic instabilities in annular combustors.
Journal of Fluid Mechanics,
Vol. 805,
Issue. ,
p.
52.
Rouwenhorst, Driek
Hermann, Jakob
and
Polifke, Wolfgang
2017.
Bifurcation study of azimuthal bulk flow in annular combustion systems with cylindrical symmetry breaking.
International Journal of Spray and Combustion Dynamics,
Vol. 9,
Issue. 4,
p.
438.
Laera, Davide
Schuller, Thierry
Prieur, Kevin
Durox, Daniel
Camporeale, Sergio M.
and
Candel, Sébastien
2017.
Flame Describing Function analysis of spinning and standing modes in an annular combustor and comparison with experiments.
Combustion and Flame,
Vol. 184,
Issue. ,
p.
136.
Laera, D.
Campa, G.
and
Camporeale, S.M.
2017.
A finite element method for a weakly nonlinear dynamic analysis and bifurcation tracking of thermo-acoustic instability in longitudinal and annular combustors.
Applied Energy,
Vol. 187,
Issue. ,
p.
216.
Worth, Nicholas A.
Dawson, James R.
Sidey, Jenni AM
and
Mastorakos, Epaminondas
2017.
Azimuthally forced flames in an annular combustor.
Proceedings of the Combustion Institute,
Vol. 36,
Issue. 3,
p.
3783.
Mejia, D.
Bauerheim, M.
Xavier, P.
Ferret, B.
Selle, L.
and
Poinsot, T.
2017.
Stabilization of a premixed laminar flame on a rotating cylinder.
Proceedings of the Combustion Institute,
Vol. 36,
Issue. 1,
p.
1447.
Schulze, Moritz
Hummel, Tobias
Klarmann, Noah
Berger, Frederik
Schuermans, Bruno
and
Sattelmayer, Thomas
2017.
Linearized Euler Equations for the Prediction of Linear High-Frequency Stability in Gas Turbine Combustors.
Journal of Engineering for Gas Turbines and Power,
Vol. 139,
Issue. 3,
Poinsot, T.
2017.
Prediction and control of combustion instabilities in real engines.
Proceedings of the Combustion Institute,
Vol. 36,
Issue. 1,
p.
1.
Prieur, K.
Durox, D.
Schuller, T.
and
Candel, S.
2017.
A hysteresis phenomenon leading to spinning or standing azimuthal instabilities in an annular combustor.
Combustion and Flame,
Vol. 175,
Issue. ,
p.
283.
Laera, Davide
Prieur, Kevin
Durox, Daniel
Schuller, Thierry
Camporeale, Sergio M.
and
Candel, Sébastien
2017.
Impact of Heat Release Distribution on the Spinning Modes of an Annular Combustor With Multiple Matrix Burners.
Journal of Engineering for Gas Turbines and Power,
Vol. 139,
Issue. 5,
Prieur, Kevin
Durox, Daniel
Schuller, Thierry
and
Candel, Sébastien
2018.
Strong Azimuthal Combustion Instabilities in a Spray Annular Chamber With Intermittent Partial Blow-Off.
Journal of Engineering for Gas Turbines and Power,
Vol. 140,
Issue. 3,
Pant, Tejas
and
Wang, Haifeng
2019.
Flamelet Modeling of Transverse Thermo-Acoustic Instability in a Multi-Element Combustor.
Mensah, Georg A.
Magri, Luca
Orchini, Alessandro
and
Moeck, Jonas P.
2019.
Effects of Asymmetry on Thermoacoustic Modes in Annular Combustors: A Higher-Order Perturbation Study.
Journal of Engineering for Gas Turbines and Power,
Vol. 141,
Issue. 4,
Bauerheim, M.
Ndiaye, A.
and
Nicoud, F.
2019.
Uncertainty Management for Robust Industrial Design in Aeronautics.
Vol. 140,
Issue. ,
p.
247.
Yao, Wei
Wu, Kun
and
Fan, Xuejun
2019.
Influences of Domain Symmetry on Supersonic Combustion Modeling.
Journal of Propulsion and Power,
Vol. 35,
Issue. 2,
p.
451.
Yang, Dong
Laera, Davide
and
Morgans, Aimee S.
2019.
A systematic study of nonlinear coupling of thermoacoustic modes in annular combustors.
Journal of Sound and Vibration,
Vol. 456,
Issue. ,
p.
137.
Li, Chunyan
Yang, Dong
Li, Suhui
and
Zhu, Min
2019.
An analytical study of the effect of flame response to simultaneous axial and transverse perturbations on azimuthal thermoacoustic modes in annular combustors.
Proceedings of the Combustion Institute,
Vol. 37,
Issue. 4,
p.
5279.
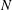 $N$ of flames prone to azimuthal thermo-acoustic instabilities. A general dispersion relation for non-perfectly-axisymmetric cavities is obtained and analytically solved to provide an explicit expression for the frequencies and growth rates of all azimuthal modes of the configuration. This analytical study unveils two parameters affecting the stability of the mode: (i) a coupling strength corresponding to the cumulative effects of the
$N$ of flames prone to azimuthal thermo-acoustic instabilities. A general dispersion relation for non-perfectly-axisymmetric cavities is obtained and analytically solved to provide an explicit expression for the frequencies and growth rates of all azimuthal modes of the configuration. This analytical study unveils two parameters affecting the stability of the mode: (i) a coupling strength corresponding to the cumulative effects of the  $N$ flames and (ii) a splitting strength due to the symmetry breaking when the flames are different. This theory has been validated using a 3D Helmholtz solver and good agreement is found. When only two types of flames are introduced into the annular cavity, the splitting strength is found to depend on two parameters: the difference between the two burner types and the pattern used to distribute the flames along the azimuthal direction. To first order, this theory suggests that the most stable configuration is obtained for a perfectly axisymmetric configuration. Therefore, breaking the symmetry by mixing different flames cannot improve the stability of an annular combustor independently of the flame distribution pattern.
$N$ flames and (ii) a splitting strength due to the symmetry breaking when the flames are different. This theory has been validated using a 3D Helmholtz solver and good agreement is found. When only two types of flames are introduced into the annular cavity, the splitting strength is found to depend on two parameters: the difference between the two burner types and the pattern used to distribute the flames along the azimuthal direction. To first order, this theory suggests that the most stable configuration is obtained for a perfectly axisymmetric configuration. Therefore, breaking the symmetry by mixing different flames cannot improve the stability of an annular combustor independently of the flame distribution pattern.


