Hostname: page-component-848d4c4894-2xdlg Total loading time: 0 Render date: 2024-06-18T23:18:27.408Z Has data issue: false hasContentIssue false
  • English
  • Français

Rheology of a dense suspension of spherical capsules under simple shear flow

Published online by Cambridge University Press:  30 November 2015

D. Matsunaga ,
Y. Imai ,
T. Yamaguchi  and
T. Ishikawa
D. Matsunaga
Affiliation:
Department of Bioengineering and Robotics, Tohoku University, 6-6-01 Aoba, Aoba, Sendai 980-8579, Japan
Y. Imai*
Affiliation:
School of Engineering, Tohoku University, 6-6-01 Aoba, Aoba, Sendai 980-8579, Japan
T. Yamaguchi
Affiliation:
Department of Biomedical Engineering, Tohoku University, 6-6-01 Aoba, Aoba, Sendai 980-8579, Japan
T. Ishikawa
Affiliation:
Department of Bioengineering and Robotics, Tohoku University, 6-6-01 Aoba, Aoba, Sendai 980-8579, Japan Department of Biomedical Engineering, Tohoku University, 6-6-01 Aoba, Aoba, Sendai 980-8579, Japan
*
†Email address for correspondence: yimai@pfsl.mech.tohoku.ac.jp
Get access Rights & Permissions [Opens in a new window]

Abstract

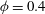We present a numerical analysis of the rheology of a dense suspension of spherical capsules in simple shear flow in the Stokes flow regime. The behaviour of neo-Hookean capsules is simulated for a volume fraction up to ${\it\phi}=0.4$ by graphics processing unit computing based on the boundary element method with a multipole expansion. To describe the specific viscosity using a polynomial equation of the volume fraction, the coefficients of the equation are calculated by least-squares fitting. The results suggest that the effect of higher-order terms is much smaller for capsule suspensions than rigid sphere suspensions; for example, 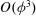$O({\it\phi}^{3})$ terms account for only 8 % of the specific viscosity even at ${\it\phi}=0.4$ for capillary numbers 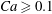$Ca\geqslant 0.1$. We also investigate the relationship between the deformation and orientation of the capsules and the suspension rheology. When the volume fraction increases, the deformation of the capsules increases while the orientation angle of the capsules with respect to the flow direction decreases. Therefore, both the specific viscosity and the normal stress difference increase with volume fraction due to the increased deformation, whereas the decreased orientation angle suppresses the specific viscosity, but amplifies the normal stress difference.

JFM classification

Low-Reynolds-number flows: Boundary integral methods Biological Fluid Dynamics: Capsule/cell dynamics Complex Fluids: Suspensions
Type
Papers
Information
Journal of Fluid Mechanics , Volume 786 , 10 January 2016 , pp. 110 - 127
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bagchi, P. & Kalluri, R. M. 2010 Rheology of a dilute suspension of liquid-filled elastic capsules. Phys. Rev. E 81, 056320.CrossRefGoogle ScholarPubMed
Barthès-Biesel, D. & Chhim, V. 1981 The constitutive equation of a dilute suspension of spherical microcapsules. Intl J. Multiphase Flow 7, 493–505.Google Scholar
Barthès-Biesel, D. & Rallison, J. M. 1981 The time-dependent deformation of a capsule freely suspended in a linear shear flow. J. Fluid Mech. 113, 251–267.CrossRefGoogle Scholar
Batchelor, G. K. 1970 The stress system in a suspension of force-free particles. J. Fluid Mech. 41, 545–570.Google Scholar
Batchelor, G. K. & Green, J. T. 1972 The determination of the bulk stress in a suspension of spherical particles to order c2. J. Fluid Mech. 56, 401–427.Google Scholar
Beenakker, C. W. J. 1986 Ewald sum of the Rotne–Prager tensor. J. Chem. Phys. 85 (3), 1581–1582.CrossRefGoogle Scholar
Clausen, J. R. & Aidun, C. K. 2010 Capsule dynamics and rheology in shear flow: particle pressure and normal stress. Phys. Fluids 22 (12), 123302.CrossRefGoogle Scholar
Clausen, J. R., Reasor, D. A. & Aidun, C. K. 2010 Parallel performance of a lattice-boltzmann/finite element cellular blood flow solver on the IBM blue gene/p architecture. Comput. Phys. Commun. 181 (6), 1013–1020.CrossRefGoogle Scholar
Clausen, J. R., Reasor, D. A. & Aidun, C. K. 2011 The rheology and microstructure of concentrated non-colloidal suspensions of deformable capsules. J. Fluid Mech. 685, 202–234.CrossRefGoogle Scholar
Durlofsky, L. J. & Brady, J. F. 1989 Dynamic simulation of bounded suspensions of hydrodynamically interacting particles. J. Fluid Mech. 200, 39–67.CrossRefGoogle Scholar
Einstein, A. 1906 Eine neue bestimmung der moleküldimensionen. Ann. Phys. 19, 289–306.Google Scholar
Freund, J. B. 2007 Leukocyte margination in a model microvessel. Phys. Fluids 19 (2), 023301.Google Scholar
Greengard, L. & Rokhlin, V. 1987 A fast algorithm for particle simulations. J. Comput. Phys. 73 (2), 325–348.Google Scholar
Gross, M., Kruger, T. & Varnik, F. 2014 Rheology of dense suspensions of elastic capsules: normal stresses, yield stress, jamming and confinement effects. Soft Matt. 10, 4360–4372.Google Scholar
Guazzelli, E. & Morris, F. J. 2012 A Physical Introduction to Suspension Dynamics. Cambridge University Press.Google Scholar
Krieger, I. M. & Dougherty, T. J. 1959 A mechanism for non-newtonian flow in suspensions of rigid spheres. Trans. Soc. Rheol. 3 (1), 137–152.Google Scholar
Kruger, T., Gross, M., Raabe, D. & Varnik, F. 2013 Crossover from tumbling to tank-treading-like motion in dense simulated suspensions of red blood cells. Soft Matt. 9, 9008–9015.Google Scholar
Li, X., Charles, R. & Pozrikidis, C. 1996 Simple shear flow of suspensions of liquid drops. J. Fluid Mech. 320, 395–416.CrossRefGoogle Scholar
Loewenberg, M. & Hinch, E. J. 1996 Numerical simulation of a concentrated emulsion in shear flow. J. Fluid Mech. 321, 395–419.Google Scholar
Matsunaga, D., Imai, Y., Omori, T., Ishikawa, T. & Yamaguchi, T. 2014 A full GPU implementation of a numerical method for simulating capsule suspensions. J. Biomech. Sci. Engng 14, 00039.Google Scholar
Onoda, G. Y. & Liniger, E. G. 1990 Random loose packings of uniform spheres and the dilatancy onset. Phys. Rev. Lett. 64, 2727–2730.CrossRefGoogle ScholarPubMed
Pozrikidis, C. 1992 Boundary Integral and Singularity Methods for Linearized Viscous Flow. Cambridge University Press.Google Scholar
Pozrikidis, C. 1995 Finite deformation of liquid capsules enclosed by elastic membranes in simple shear flow. J. Fluid Mech. 297, 123–152.Google Scholar
Pozrikidis, C. 2003 Modeling and Simulation of Capsules and Biological Cells. Chapman and Hall/CRC.CrossRefGoogle Scholar
Ramanujan, S. & Pozrikidis, C. 1998 Deformation of liquid capsules enclosed by elastic membranes in simple shear flow: large deformations and the effect of fluid viscosities. J. Fluid Mech. 361, 117–143.CrossRefGoogle Scholar
Tan, M. H.-Y., Le, D.-V. & Chiam, K.-H. 2012 Hydrodynamic diffusion of a suspension of elastic capsules in bounded simple shear flow. Soft Matt. 8, 2243–2251.CrossRefGoogle Scholar
Veerapaneni, K. S., Rahimian, A., Biros, G. & Zorin, D. 2011 A fast algorithm for simulating vesicle flows in three dimensions. J. Comput. Phys. 230 (14), 5610–5634.Google Scholar
Walter, J., Salsac, A.-V., Barthès-Biesel, D. & Le Tallec, P. 2010 Coupling of finite element and boundary integral methods for a capsule in a Stokes flow. Intl J. Numer. Meth. Engng 83 (7), 829–850.Google Scholar
Ying, L., Biros, G. & Zorin, D. 2004 A kernel-independent adaptive fast multipole algorithm in two and three dimensions. J. Comput. Phys. 196 (2), 591–626.Google Scholar
Zhao, H. & Shaqfeh, E. S. G. 2013 The dynamics of a non-dilute vesicle suspension in a simple shear flow. J. Fluid Mech. 725, 709–731.Google Scholar
Zinchenko, A. Z. & Davis, R. H. 2000 An efficient algorithm for hydrodynamical interaction of many deformable drops. J. Comput. Phys. 157 (2), 539–587.Google Scholar
Zinchenko, A. Z. & Davis, R. H. 2005 A multipole-accelerated algorithm for close interaction of slightly deformable drops. J. Comput. Phys. 207 (2), 695–735.Google Scholar