Hostname: page-component-8448b6f56d-jr42d Total loading time: 0 Render date: 2024-04-23T22:13:57.190Z Has data issue: false hasContentIssue false
  • English
  • Français

A physically motivated approach for filtering acoustic waves from the equations governing compressible stratified flow

Published online by Cambridge University Press:  25 April 2008

DALE R. DURRAN
DALE R. DURRAN*
Affiliation:
Department of Atmospheric Sciences, University of Washington, Seattle, WA, 98125, USA
Get access Rights & Permissions [Opens in a new window]

Abstract

An incompressibility approximation is formulated for isentropic motions in a compressible stratified fluid by defining a pseudo-density ρ* and enforcing mass conservation with respect to ρ* instead of the true density. Using this approach, sound waves will be eliminated from the governing equations provided ρ* is an explicit function of the space and time coordinates and of entropy. By construction, isentropic pressure perturbations have no influence on the pseudo-density.

A simple expression for ρ* is available for perfect gases that allows the approximate mass conservation relation to be combined with the unapproximated momentum and thermodynamic equations to yield a closed system with attractive energy conservation properties. The influence of pressure on the pseudo-density, along with the explicit (x,t) dependence of ρ* is determined entirely by the hydrostatically balanced reference state.

Scale analysis shows that the pseudo-incompressible approximation is applicable to motions for which 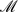2 ≪ min(1,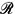2, where 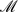is the Mach number and 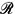the Rossby number. This assumption is easy to satisfy for small-scale atmospheric motions in which the Earth's rotation may be neglected and is also satisfied for quasi-geostrophic synoptic-scale motions, but not planetary-scale waves. This scaling assumption can, however, be relaxed to allow the accurate representation of planetary-scale motions if the pressure in the time-evolving reference state is computed with sufficient accuracy that the large-scale components of the pseudo-incompressible pressure represent small corrections to the total pressure, in which case the full solution to both the pseudo-incompressible and reference-state equations has the potential to accurately model all non-acoustic atmospheric motions.

Type
Papers
Information
Journal of Fluid Mechanics , Volume 601 , 25 April 2008 , pp. 365 - 379
Copyright
Copyright © Cambridge University Press 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Almgren, A. 2000 A new look at the pseudo-incompressible solution to Lamb's problem of hydrostatic adjustment. J. Atmos. Sci. 57, 995–998.2.0.CO;2>CrossRefGoogle Scholar
Almgren, A. S., Bell, J. B., Rendleman, C. A. & Zingale, M. 2006 Low Mach number modeling of type Ia supernovae. I. Hydrodynamics. Astrophys. J. 637, 922–936.CrossRefGoogle Scholar
Bannon, P. R. 1996 a Nonlinear hydrostatic adjustment. J. Atmos. Sci. 53, 3606–3617.2.0.CO;2>CrossRefGoogle Scholar
Bannon, P. R. 1996 b On the anelastic approximation for a compressible atmosphere. J. Atmos. Sci. 53, 3618–3628.2.0.CO;2>CrossRefGoogle Scholar
Batchelor, G. K. 1953 The conditions for dynamical similarity of motions of a frictionless perfect-gas atmosphere. Q. J. R. Met. Soc. 79, 224–235.CrossRefGoogle Scholar
Batchelor, G. K. 1967 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
Bois, P.-A. 2006 A unified asymptotic theory of the anelastic approximation in geophysical gases and liquids. Mech. Res. Commun. 33, 628–635.CrossRefGoogle Scholar
Chorin, A. J. 1968 Numerical solution of the Navier-Stokes equations. Math. Comput. 22, 745–762.CrossRefGoogle Scholar
Davies, T., Staniforth, A., Wood, N. & Thuburn, J. 2003 Validity of anelastic and other equation sets as inferred from normal-mode analysis. Q. J. R. Met. Soc. 129, 2761–2775.Google Scholar
Durran, D. R. 1989 Improving the anelastic approximation. J. Atmos. Sci. 46, 1453–1461.2.0.CO;2>CrossRefGoogle Scholar
Durran, D. R. 1999 Numerical Methods for Wave Equations in Geophysical Fluid Dynamics. Springer.CrossRefGoogle Scholar
Gill, A. E. 1982 Atmosphere—Ocean Dynamics. Academic.Google Scholar
Klein, R. 2000 Asymptotic analyses for atmospheric flows and the construction of asymptotically adaptive numerical methods. Z. Angew. Math. Mech. 80, 765–777.3.0.CO;2-1>CrossRefGoogle Scholar
Klein, R. & Majda, A. 2006 Systematic multiscale models for deep convection on mesoscales. Theor. Comput. Fluid Dyn. 20, 525–551.CrossRefGoogle Scholar
Lipps, F. 1990 On the anelastic approximation for deep convection. J. Atmos. Sci. 47, 1794–1798.2.0.CO;2>CrossRefGoogle Scholar
Lipps, F. & Hemler, R. 1982 A scale analysis of deep moist convection and some related numerical calculations. J. Atmos. Sci. 29, 2192–2210.2.0.CO;2>CrossRefGoogle Scholar
Majda, A. 1984 Compressible fluid flow and systems of conservation laws in several space variables. Appl. Math. Sci. 53.CrossRefGoogle Scholar
McMurty, P. A., Jou, W.-H., Riley, J. J. & Metcalfe, R. W. 1986 Direct numerical simulations of a reacting mixing layer with chemical heat release. AIAA J. 24, 962–970.CrossRefGoogle Scholar
Nance, L. B. 1997 On the inclusion of compressibility effects in the scorer parameter. J. Atmos. Sci. 54, 362–367.2.0.CO;2>CrossRefGoogle Scholar
Nance, L. B. & Durran, D. R. 1994 A comparison of three anelastic systems and the pseudo-incompressible system. J. Atmos. Sci. 51, 3549–3565.2.0.CO;2>CrossRefGoogle Scholar
Ogura, Y. & Phillips, N. 1962 Scale analysis for deep and shallow convection in the atmosphere. J. Atmos. Sci. 19, 173–179.2.0.CO;2>CrossRefGoogle Scholar
Pedlosky, J. 1987 Geophysical Fluid Dynamics, 2nd edn. Springer.CrossRefGoogle Scholar
Shirgaonkar, A. A. & Lele, S. K. 2006 On the extension of the Boussinesq approximation for inertia dominated flows. Phys. Fluids 18, 066601.CrossRefGoogle Scholar
Smolarkiewicz, P. K. & Dörnbrack, A. 2008 Conservative integrals of adiabatic Durran's equations. Intl J. Numer. Meth. Fluids. In press, DOI: 10.1002/fld.1601.CrossRefGoogle Scholar
Spiegel, E. A. & Veronis, G. K. 1960 On the Boussinesq approximation for a compressible fluid. Astrophys. J. 131, 442–447.CrossRefGoogle Scholar
Témam, R. 1969 Sur l'approximation de la solution des équations Navier-Stokes par la méthode des pas fractionnaires. Arch. Rat. Mech. Anal. 33, 377–385.CrossRefGoogle Scholar