Article contents
Mean dynamics of transitional boundary-layer flow
Published online by Cambridge University Press: 19 July 2011
Abstract
The dynamical mechanisms underlying the redistribution of mean momentum and vorticity are explored for transitional two-dimensional boundary-layer flow at nominally zero pressure gradient. The analyses primarily employ the direct numerical simulation database of Wu & Moin (J. Fluid Mech., vol. 630, 2009, p. 5), but are supplemented with verifications utilizing subsequent similar simulations. The transitional regime is taken to include both an instability stage, which effectively generates a finite Reynolds stress profile, −ρuv(y), and a nonlinear development stage, which progresses until the terms in the mean momentum equation attain the magnitude ordering of the four-layer structure revealed by Wei et al. (J. Fluid Mech., vol. 522, 2005, p. 303). Self-consistently applied criteria reveal that the third layer of this structure forms first, followed by layers IV and then II and I. For the present flows, the four-layer structure is estimated to be first realized at a momentum thickness Reynolds number Rθ = U∞ θ/ν ≃ 780. The first-principles-based theory of Fife et al. (J. Disc. Cont. Dyn. Syst. A, vol. 24, 2009, p. 781) is used to describe the mean dynamics in the laminar, transitional and four-layer regimes. As in channel flow, the transitional regime is marked by a non-negligible influence of all three terms in the mean momentum equation at essentially all positions in the boundary layer. During the transitional regime, the action of the Reynolds stress gradient rearranges the mean viscous force and mean advection profiles. This culminates with the segregation of forces characteristic of the four-layer regime. Empirical and theoretical evidence suggests that the formation of the four-layer structure also underlies the emergence of the mean dynamical properties characteristic of the high-Reynolds-number flow. These pertain to why and where the mean velocity profile increasingly exhibits logarithmic behaviour, and how and why the Reynolds stress distribution develops such that the inner normalized position of its peak value, ym+, exhibits a Reynolds number dependence according to 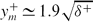 .
.
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2011
References
REFERENCES
- 26
- Cited by


