Published online by Cambridge University Press: 17 December 2019
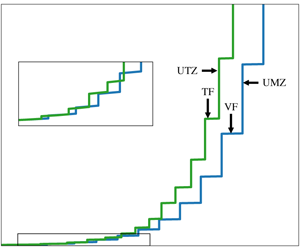
Experimental and numerical studies over the past two decades indicate that as the Reynolds number becomes large the turbulent boundary layer is increasingly composed of zones of uniform streamwise momentum, segregated by narrow regions of high shear. Recent experimental evidence suggests that passive scalar fields (for example, temperature) in turbulent boundary layers at high Reynolds number show similar characteristics; namely, large uniform temperature zones (UTZs) separated by narrow regions of high gradient, which we term thermal fissures (TFs). Herein, a model informed by analysis of the mean scalar transport equation, and that leverages the dynamical model recently developed by the authors (Cuevas Bautista et al., J. Fluid Mech., vol. 858, 2019, pp. 609–633), is formulated to predict passive scalar transport using the UTZ/TF concept. First, a finite number of TFs are distributed across the boundary layer. In analogy with the aforementioned dynamical model, the wall-normal positions of the TFs and their characteristic temperatures are then perturbed to generate independent ensembles, from which statistical moments are computed. The model successfully reproduces the statistical profiles of the temperature field as well as the streamwise turbulent heat flux. Lastly, the Prandtl number dependency of the empirically chosen parameters is investigated. It is concluded that the higher-order statistics, especially the kurtosis, produced by the model are sensitive to the Prandtl number, while the mean temperature and turbulent heat flux do not show noticeable Prandtl number dependency.