Article contents
Geometry of enstrophy and dissipation, grid resolution effects and proximity issues in turbulence
Published online by Cambridge University Press: 10 February 2009
Abstract
We perform a multi-scale non-local geometrical analysis of the structures extracted from the enstrophy and kinetic energy dissipation-rate, instantaneous fields of a numerical database of incompressible homogeneous isotropic turbulence decaying in time obtained by DNS in a periodic box. Three different resolutions are considered: 2563, 5123 and 10243 grid points, with kmax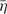 approximately 1, 2 and 4, respectively, the same initial conditions and Reλ ≈ 77. This allows a comparison of the geometry of the structures obtained for different resolutions. For the highest resolution, structures of enstrophy and dissipation evolve in a continuous distribution from blob-like and moderately stretched tube-like shapes at the large scales to highly stretched sheet-like structures at the small scales. The intermediate scales show a predominance of tube-like structures for both fields, much more pronounced for the enstrophy field. The dissipation field shows a tendency towards structures with lower curvedness than those of the enstrophy, for intermediate and small scales. The 2563 grid resolution case (kmax
approximately 1, 2 and 4, respectively, the same initial conditions and Reλ ≈ 77. This allows a comparison of the geometry of the structures obtained for different resolutions. For the highest resolution, structures of enstrophy and dissipation evolve in a continuous distribution from blob-like and moderately stretched tube-like shapes at the large scales to highly stretched sheet-like structures at the small scales. The intermediate scales show a predominance of tube-like structures for both fields, much more pronounced for the enstrophy field. The dissipation field shows a tendency towards structures with lower curvedness than those of the enstrophy, for intermediate and small scales. The 2563 grid resolution case (kmax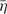 ≈ 1) was unable to detect the predominance of highly stretched sheet-like structures at the smaller scales in both fields. The same non-local methodology for the study of the geometry of structures, but without the multi-scale decomposition, is applied to two scalar fields used by existing local criteria for the eduction of tube- and sheet-like structures in turbulence, Q and [Aij]+, respectively, obtained from invariants of the velocity-gradient tensor and alike in the 10243 case. This adds the non-local geometrical characterization and classification to those local criteria, assessing their validity in educing particular geometries. Finally, we introduce a new methodology for the study of proximity issues among structures of different fields, based on geometrical considerations and non-local analysis, by taking into account the spatial extent of the structures. We apply it to the four fields previously studied. Tube-like structures of Q are predominantly surrounded by sheet-like structures of [Aij]+, which appear at closer distances. For the enstrophy, tube-like structures at an intermediate scale are primarily surrounded by sheets of smaller scales of the enstrophy and structures of dissipation at the same and smaller scales. A secondary contribution results from tubes of enstrophy at smaller scales appearing at farther distances. Different configurations of composite structures are presented.
≈ 1) was unable to detect the predominance of highly stretched sheet-like structures at the smaller scales in both fields. The same non-local methodology for the study of the geometry of structures, but without the multi-scale decomposition, is applied to two scalar fields used by existing local criteria for the eduction of tube- and sheet-like structures in turbulence, Q and [Aij]+, respectively, obtained from invariants of the velocity-gradient tensor and alike in the 10243 case. This adds the non-local geometrical characterization and classification to those local criteria, assessing their validity in educing particular geometries. Finally, we introduce a new methodology for the study of proximity issues among structures of different fields, based on geometrical considerations and non-local analysis, by taking into account the spatial extent of the structures. We apply it to the four fields previously studied. Tube-like structures of Q are predominantly surrounded by sheet-like structures of [Aij]+, which appear at closer distances. For the enstrophy, tube-like structures at an intermediate scale are primarily surrounded by sheets of smaller scales of the enstrophy and structures of dissipation at the same and smaller scales. A secondary contribution results from tubes of enstrophy at smaller scales appearing at farther distances. Different configurations of composite structures are presented.
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2009
References
REFERENCES
- 24
- Cited by




