Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-18T18:49:42.720Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamic 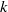-equation model for large-eddy simulation of compressible flows

Published online by Cambridge University Press:  16 April 2012

Xiaochuan Chai  and
Krishnan Mahesh
Xiaochuan Chai
Affiliation:
Aerospace Engineering and Mechanics, University of Minnesota, Minneapolis, MN 55108, USA
Krishnan Mahesh*
Affiliation:
Aerospace Engineering and Mechanics, University of Minnesota, Minneapolis, MN 55108, USA
*
†Email address for correspondence: mahesh@aem.umn.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper presents a dynamic one-equation eddy viscosity model for large-eddy simulation (LES) of compressible flows. The transport equation for subgrid-scale (SGS) kinetic energy is introduced to predict SGS kinetic energy. The exact SGS kinetic energy transport equation for compressible flows is derived formally. Each of the unclosed terms in the SGS kinetic energy equation is modelled separately and dynamically closed, instead of being grouped into production and dissipation terms, as in the Reynolds averaged Navier–Stokes equations. All of the SGS terms in the filtered total energy equation are found to reappear in the SGS kinetic energy equation. Therefore, these terms can be included in the total energy equation without adding extra computational cost. A priori tests using direct numerical simulation (DNS) of decaying isotropic turbulence show that, for a Smagorinsky-type eddy viscosity model, the correlation between the SGS stress and the model is comparable to that from the original model. Also, the suggested model for the pressure dilatation term in the SGS kinetic energy equation is found to have a high correlation with its actual value. In a posteriori tests, the proposed dynamic 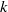-equation model is applied to decaying isotropic turbulence and normal shock–isotropic turbulence interaction, and yields good agreement with available experimental and DNS data. Compared with the results of the dynamic Smagorinsky model (DSM), the 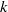-equation model predicts better energy spectra at high wavenumbers, similar kinetic energy decay and fluctuations of thermodynamic quantities for decaying isotropic turbulence. For shock–turbulence interaction, the 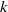-equation model and the DSM predict similar evolutions of turbulent intensities across shocks, owing to the dominant effect of linear interaction. The proposed -equation model is more robust in that local averaging over neighbouring control volumes is sufficient to regularize the dynamic procedure. The behaviour of pressure dilatation and dilatational dissipation is discussed through the budgets of the SGS kinetic energy equation, and the importance of the dilatational dissipation term is addressed.

JFM classification

Turbulent Flows: Compressible turbulence Aerodynamics: High-speed flow Compressible Flows: Shock waves
Type
Papers
Information
Journal of Fluid Mechanics , Volume 699 , 25 May 2012 , pp. 385 - 413
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Bedford, K. W. & Yeo, W. K. 1993 Conjunctive filtering procedures in surface water flow and transport. In Large Eddy Simulation of Complex Engineering and Geophysical Flows (ed. Galperin, B. & Orszag, S. A. ), pp. 513–539. Cambridge University Press.Google Scholar
2. Chai, X. & Mahesh, K. 2011 Simulations of high speed jets in cross-flows. AIAA Paper 2011-650.CrossRefGoogle Scholar
3. Chollet, J. P. & Lesieur, M. 1981 Parametrization of small scales of three-dimensional isotropic turbulence utilizing spectral closures. J. Atmos. Sci. 38, 2747–2757.2.0.CO;2>CrossRefGoogle Scholar
4. Comte-Bellot, G. & Corrsin, S. 1971 Simple Eulerian time correlation of full- and narrow-band velocity signals in grid-generated isotropic turbulence. J. Fluid Mech. 48, 273.Google Scholar
5. Deardorff, J. W. 1973 Three-dimensional numerical modeling of the planetary boundary layer. In Workshop on Micrometeorology (ed. Haugen, D. A. ), pp. 271–311. American Meteorological Society.Google Scholar
6. Dubois, T., Domaradzki, J. A. & Honein, A. 2002 The subgrid-scale estimation model applied to large eddy simulations of compressible turbulence. Phys. Fluids 14 (5), 1781–1801.CrossRefGoogle Scholar
7. Erlebacher, G., Hussaini, M. Y., Kreiss, H. O. & Sarkar, S. 1990 The analysis and simulation of compressible turbulence. Theor. Comput. Fluid Dyn. 2, 73–95.CrossRefGoogle Scholar
8. Garnier, E., Adams, N. & Sagaut, P. 2009 Large Eddy Simulation for Compressible Flows (Scientific Computation), 1st edn. Springer.Google Scholar
9. Génin, F. & Menon, S. 2010 Dynamics of sonic jet injection into supersonic cross-flow. J. Turbul. 11, 1–13.CrossRefGoogle Scholar
10. Germano, M., Piomelli, U., Moin, P. & Cabot, M. 1991 A dynamic subgrid-scale eddy viscosity model. Phys. Fluids 3, 1760.CrossRefGoogle Scholar
11. Ghosal, S., Lund, T. S., Moin, P. & Akselvoll, K. 1995 A dynamic localization model for large-eddy simulation of turbulent flows. J. Fluid Mech. 286, 229.CrossRefGoogle Scholar
12. Ghosh, S. & Mahesh, K. 2008 Numerical simulation of the fluid dynamic effects of laser energy deposition in air. J. Fluid Mech. 605, 329–354.Google Scholar
13. Horiuti, K. 1985 Large eddy simulation of turbulent channel flow by one-equation modeling. J. Phys. Soc. Japan 54, 2855–2865.CrossRefGoogle Scholar
14. Kraichnan, R. H. 1964 Direct-interaction approximation for shear and thermally driven turbulence. Phys. Fluids 7 (7), 1048–1062.Google Scholar
15. Kraichnan, R. H. 1976 Eddy viscosity in two and three dimensions. J. Atmos. Sci. 33, 1521–1536.2.0.CO;2>CrossRefGoogle Scholar
16. Larsson, J. & Lele, S. K. 2009 Direct numerical simulation of canonical shock/turbulence interaction. Phys. Fluids 21, 126101.CrossRefGoogle Scholar
17. Lee, S., Lele, S. K. & Moin, P. 1993 Direct numerical simulation of isotropic turbulence interacting with a weak shock wave. J. Fluid Mech. 251, 533–562.CrossRefGoogle Scholar
18. Lesieur, M. & Métais, O. 1996 New trends in large-eddy simulations of turbulence. Annu. Rev. Fluid Mech. 28, 45–82.CrossRefGoogle Scholar
19. Lilly, D. K. 1967 The representation of small-scale turbulence in numerical simulation experiments. In IBM Scientific Computing Symposium on Environmental Sciences, Yorktown Heights, pp. 195–210. IBM.Google Scholar
20. Lilly, D. K. 1991 A proposed modification of the Germano subgrid-scale closure method. Phys. Fluids A 3, 1760.Google Scholar
21. Mahesh, K., Lele, S. K. & Moin, P. 1997 The influence of entropy fluctuations on the interaction of turbulence with a shock wave. J. Fluid Mech. 334, 353–379.CrossRefGoogle Scholar
22. Mason, P. J. 1994 Large-eddy simulation: a critical review of the technique. Q. J. R. Meteorol. Soc. 120 (515), 1–26.Google Scholar
23. Meneveau, C. 1994 Statistics of turbulence subgrid-scale stresses: necessary conditions and experimental tests. Phys. Fluids 6 (2), 815–833.CrossRefGoogle Scholar
24. Meneveau, C. & Katz, J. 2000 Scale-invariance and turbulence models for large-eddy simulation. Annu. Rev. Fluid Mech. 32 (1), 1–32.Google Scholar
25. Menon, S. & Kim, W. W. 1996 High Reynolds number flow simulations using the localized dynamic subgrid-scale model. In AIAA 34th Aerospace Sciences Meeting and Exhibit. AIAA Paper 1996-0425.Google Scholar
26. Métais, O. & Lesieur, M. 1992 Spectral large-eddy simulation of isotropic and stably stratified turbulence. J. Fluid Mech. 239, 157–194.Google Scholar
27. Moeng, C.-H. 1984 A large-eddy-simulation model for the study of planetary boundary-layer turbulence. J. Atmos. Sci. 41, 2052–2062.Google Scholar
28. Moin, P., Squires, K., Cabot, W. & Lee, S. 1991 A dynamic subgrid-scale model for compressible turbulence and scalar transport. Phys. Fluids A 3 (11), 2746–2757.CrossRefGoogle Scholar
29. Muppidi, S. & Mahesh, K. 2011 DNS of roughness-induced transition in supersonic boundary layers. J. Fluid Mech. 693, 28–56.Google Scholar
30. Park, N. & Mahesh, K. 2007 Numerical and modeling issues in LES of compressible turbulent flows on unstructured grids. AIAA Paper 2007-0722.CrossRefGoogle Scholar
31. Piomelli, U., Cabot, W. H., Moin, P. & Lee, S. 1991 Subgrid-scale backscatter in turbulent and transitional flows. Phys. Fluids A 3, 1766–1771.Google Scholar
32. Pomraning, E. & Rutland, C. J. 2002 Dynamic one-equation nonviscosity large-eddy simulation model. AIAA J. 40, 689–701.Google Scholar
33. Ristorcelli, J. R. 1997 A pseudo-sound constitutive relationship for the dilatational covariances in compressible turbulence. J. Fluid Mech. 347, 37–70.CrossRefGoogle Scholar
34. Ristorcelli, J. R. & Blaisdell, G. A. 1997 Consistent initial conditions for the DNS of compressible turbulence. Phys. Fluids 9 (1), 4–6.CrossRefGoogle Scholar
35. Sarkar, S., Erlebacher, G., Hussaini, M. Y. & Kreiss, H. O. 1991 The analysis and modeling of dilatational terms in compressible turbulence. J. Fluid Mech. 227, 473–493.CrossRefGoogle Scholar
36. Schumann, U. 1975 Subgrid scale model for finite difference simulations of turbulent flows in plane channels and annuli. J. Comput. Phys. 18 (4), 376–404.Google Scholar
37. Shaw, R. H. & Schumann, U. 1992 Large-eddy simulation of turbulent flow above and within a forest. Boundary-Layer Meteorol. 61, 47–64.CrossRefGoogle Scholar
38. Smagorinsky, J. 1963 General circulation experiments with the primitive equations. I. The basic experiment. Mon. Weath. Rev. 91, 99–165.2.3.CO;2>CrossRefGoogle Scholar
39. Smith, L. M. & Woodruff, S. L. 1998 Renormaliztion-group analysis of turbulence. Annu. Rev. Fluid Mech. 30, 275–310.Google Scholar
40. Speziale, C. G. 1991 Analytic methods for the development of Reynolds-stress closures in turbulence. Annu. Rev. Fluid Mech. 23, 107–157.CrossRefGoogle Scholar
41. Speziale, C. G., Erlebacher, G., Zang, T. A. & Hussaini, M. Y. 1988 The subgrid-scale modeling of compressible turbulence. Phys. Fluids 31 (4), 940–942.CrossRefGoogle Scholar
42. Spyropoulos, E. T. & Blaisdell, G. A. 1996 Evaluation of the dynamic model for simulations of compressible decaying isotropic turbulence. AIAA J. 34 (5), 990–998.CrossRefGoogle Scholar
43. Vreman, B., Geurts, B. & Kuerten, H. 1995 Subgrid-modeling in LES of compressible flow. Appl. Sci. Res. 54, 191–203.Google Scholar
44. Yakhot, A., Orszag, S. A. & Yakhot, Y. 1989 Renormalization-group formulation of large-eddy simulations. J. Sci. Comput. 4, 139.CrossRefGoogle Scholar
45. Yee, H. C, Sandham, N. D & Djomehri, M. J 1999 Low-dissipative high-order shock-capturing methods using characteristic-based filters. J. Comput. Phys. 150 (1), 199–238.CrossRefGoogle Scholar
46. Yoshizawa, A. 1986 Statistical theory for compressible turbulent shear flows, with the application to subgrid modeling. Phys. Fluids 29, 2152.Google Scholar
47. Yoshizawa, A. & Horiuti, K. 1985 A statistically-derived subgrid-scale kinetic energy model for the large-eddy simulation of turbulent flows. J. Phys. Soc. Japan 54, 2834–2839.Google Scholar