Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ganguli, Swetava
and
Lele, Sanjiva K.
2019.
Low Mach, compressibility, and finite size effects of localized uniform heat sources in a gas.
Theoretical and Computational Fluid Dynamics,
Vol. 33,
Issue. 3-4,
p.
341.
Fairbanks, Hillary R.
Jofre, Lluís
Geraci, Gianluca
Iaccarino, Gianluca
and
Doostan, Alireza
2020.
Bi-fidelity approximation for uncertainty quantification and sensitivity analysis of irradiated particle-laden turbulence.
Journal of Computational Physics,
Vol. 402,
Issue. ,
p.
108996.
Mouallem, Joseph
and
Hickey, Jean-Pierre
2020.
Induction heating of dispersed metallic particles in a turbulent flow.
International Journal of Multiphase Flow,
Vol. 132,
Issue. ,
p.
103414.
Banko, Andrew J.
Villafañe, Laura
Kim, Ji Hoon
and
Eaton, John K.
2020.
Temperature statistics in a radiatively heated particle-laden turbulent square duct flow.
International Journal of Heat and Fluid Flow,
Vol. 84,
Issue. ,
p.
108618.
Horwitz, Jeremy A. K.
Ganguli, Swetava
Lele, Sanjiva K.
and
Mani, Ali
2021.
Settling of two-way momentum and energy coupled particles subject to Boussinesq and non-Boussinesq heating.
Theoretical and Computational Fluid Dynamics,
Vol. 35,
Issue. 4,
p.
539.
Wang, Yingdong
Wang, Huibo
Jin, Hui
and
Guo, Liejin
2021.
Numerical simulation of adiabatic/cooled/heated spherical particles with Stefan flow in supercritical water.
Physics of Fluids,
Vol. 33,
Issue. 5,
Ou, Zhisong
Chi, Cheng
Guo, Liejin
and
Thévenin, Dominique
2022.
A directional ghost-cell immersed boundary method for low Mach number reacting flows with interphase heat and mass transfer.
Journal of Computational Physics,
Vol. 468,
Issue. ,
p.
111447.
Shaw, B. D.
and
Vang, C. L.
2022.
Evaluation of the Nusselt number for a fluid sphere in Stokes flow.
Physics of Fluids,
Vol. 34,
Issue. 9,
Ou, Zhisong
Guo, Liejin
Chi, Cheng
Zhu, Shixing
Ren, Changsheng
Jin, Hui
and
Thévenin, Dominique
2022.
Interface-resolved direct numerical simulations of interphase momentum, heat, and mass transfer in supercritical water gasification of coal.
Physics of Fluids,
Vol. 34,
Issue. 10,
Kiwitt, Thede
Fröhlich, Konstantin
Meinke, Matthias
and
Schröder, Wolfgang
2022.
Nusselt correlation for ellipsoidal particles.
International Journal of Multiphase Flow,
Vol. 149,
Issue. ,
p.
103941.
Yuan, Xuyao
Wei, Wei
Fang, Zhenlong
and
Chen, Yong
2022.
Lattice Boltzmann model for the low-Mach number variable-density flow.
Physics of Fluids,
Vol. 34,
Issue. 6,
Sundmacher, Kai
and
Thévenin, Dominique
2023.
Direct numerical simulations of polypropylene gasification in supercritical water.
Physics of Fluids,
Vol. 35,
Issue. 6,
Keane, Nathan A.
Apte, Sourabh V.
Jain, Suhas S.
and
Khanwale, Makrand A.
2023.
Effect of interpolation kernels and grid refinement on two way-coupled point-particle simulations.
International Journal of Multiphase Flow,
Vol. 166,
Issue. ,
p.
104517.
Rodriguez, I.
and
Campo, A.
2023.
Numerical investigation of forced convection heat transfer from a sphere at low Prandtl numbers.
International Journal of Thermal Sciences,
Vol. 184,
Issue. ,
p.
107970.
Zhang, Yunxin
Li, Chenggong
and
Ye, Mao
2024.
The role of permeability in lid-driven cavity flow containing a cluster of hot solids.
Physics of Fluids,
Vol. 36,
Issue. 4,
Mahato, Bikash
Saxena, Saurabh
and
Yaghoobian, Neda
2024.
Aerodynamic force modifications of a spherical particle with varying temperature: a study of an idealized firebrand.
Theoretical and Computational Fluid Dynamics,
Vol. 38,
Issue. 2,
p.
251.
Briney, Sam
Osnes, Andreas N.
Vartdal, Magnus
Jackson, Thomas L.
and
Balachandar, S.
2024.
Neighbor-induced unsteady force in the interaction of a cylindrical shock wave with an annular particle cloud.
Physical Review Fluids,
Vol. 9,
Issue. 2,
Berti, Zavier
and
Flynn, M. R.
2024.
A Nusselt number correlation for a superhydrophobic solid sphere encapsulated in a perfect plastron.
Journal of Engineering Mathematics,
Vol. 147,
Issue. 1,
Shin, Yeongho
Kim, Sanghun
and
Jun, Eunji
2024.
An approach for multiscale two-phase flow simulation in the direct simulation Monte Carlo framework.
Physics of Fluids,
Vol. 36,
Issue. 6,
 $Re$) in the range
$Re$) in the range 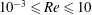 $10^{-3}\leqslant Re\leqslant 10$ in a variable-property fluid. The case where buoyancy is present is analysed in a subsequent paper. This analysis is carried out without making any assumptions on the amount of heat addition from the sphere and thus encompasses both the heating regime where the Boussinesq approximation holds and the regime where it breaks down. The particle is assumed to have a low Biot number, which means that the particle is uniformly at the same temperature and has no internal temperature gradients. Large deviations in the value of the drag coefficient as the temperature of the sphere increases are observed. When
$10^{-3}\leqslant Re\leqslant 10$ in a variable-property fluid. The case where buoyancy is present is analysed in a subsequent paper. This analysis is carried out without making any assumptions on the amount of heat addition from the sphere and thus encompasses both the heating regime where the Boussinesq approximation holds and the regime where it breaks down. The particle is assumed to have a low Biot number, which means that the particle is uniformly at the same temperature and has no internal temperature gradients. Large deviations in the value of the drag coefficient as the temperature of the sphere increases are observed. When  $Re<O(10^{-2})$, these deviations are explained using a low-Mach-number perturbation analysis as irrotational corrections to a Stokes–Oseen base flow. Correlations for the drag and Nusselt number of a heated sphere are proposed for the range of Reynolds numbers
$Re<O(10^{-2})$, these deviations are explained using a low-Mach-number perturbation analysis as irrotational corrections to a Stokes–Oseen base flow. Correlations for the drag and Nusselt number of a heated sphere are proposed for the range of Reynolds numbers 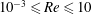 $10^{-3}\leqslant Re\leqslant 10$ which fit the computationally obtained values with less than 1 % and 3 % errors, respectively. These correlations can be used in simulations of gas–solid flows where the accuracy of the drag law affects the prediction of the overall flow behaviour. Finally, an analogy to incompressible flow over a modified sphere is demonstrated.
$10^{-3}\leqslant Re\leqslant 10$ which fit the computationally obtained values with less than 1 % and 3 % errors, respectively. These correlations can be used in simulations of gas–solid flows where the accuracy of the drag law affects the prediction of the overall flow behaviour. Finally, an analogy to incompressible flow over a modified sphere is demonstrated.