Hostname: page-component-76fb5796d-25wd4 Total loading time: 0 Render date: 2024-04-26T10:36:55.365Z Has data issue: false hasContentIssue false
  • English
  • Français

Convective heat transfer along ratchet surfaces in vertical natural convection

Published online by Cambridge University Press:  28 June 2019

Hechuan Jiang Open the ORCID record for Hechuan Jiang [Opens in a new window] ,
Xiaojue Zhu Open the ORCID record for Xiaojue Zhu [Opens in a new window] ,
Varghese Mathai Open the ORCID record for Varghese Mathai [Opens in a new window] ,
Xianjun Yang ,
Roberto Verzicco Open the ORCID record for Roberto Verzicco [Opens in a new window] ,
Detlef Lohse Open the ORCID record for Detlef Lohse [Opens in a new window]  and
Chao Sun Open the ORCID record for Chao Sun [Opens in a new window]
Hechuan Jiang
Affiliation:
Center for Combustion Energy, Key Laboratory for Thermal Science and Power Engineering of Ministry of Education, Department of Energy and Power Engineering, Tsinghua University, 100084 Beijing, China
Xiaojue Zhu
Affiliation:
Center of Mathematical Sciences and Applications, and School of Engineering and Applied Sciences, Harvard University, Cambridge, MA 02138, USA
Varghese Mathai
Affiliation:
School of Engineering, Brown University, Providence, RI 02912, USA
Xianjun Yang
Affiliation:
Center for Combustion Energy, Key Laboratory for Thermal Science and Power Engineering of Ministry of Education, Department of Energy and Power Engineering, Tsinghua University, 100084 Beijing, China
Roberto Verzicco
Affiliation:
Dipartimento di Ingegneria Industriale, University of Rome ‘Tor Vergata’, Via del Politecnico 1, 00133 Roma, Italy Physics of Fluids Group and Max Planck Center Twente, University of Twente, PO Box 217, 7500AE Enschede, The Netherlands
Detlef Lohse
Affiliation:
Physics of Fluids Group and Max Planck Center Twente, University of Twente, PO Box 217, 7500AE Enschede, The Netherlands Max Planck Institute for Dynamics and Self-Organization, 37077 Göttingen, Germany
Chao Sun*
Affiliation:
Center for Combustion Energy, Key Laboratory for Thermal Science and Power Engineering of Ministry of Education, Department of Energy and Power Engineering, Tsinghua University, 100084 Beijing, China Physics of Fluids Group and Max Planck Center Twente, University of Twente, PO Box 217, 7500AE Enschede, The Netherlands
*
†Email address for correspondence: chaosun@tsinghua.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

We report on a combined experimental and numerical study of convective heat transfer along ratchet surfaces in vertical natural convection (VC). Due to the asymmetry of the convection system caused by the asymmetric ratchet-like wall roughness, two distinct states exist, with markedly different orientations of the large-scale circulation roll (LSCR) and different heat transport efficiencies. Statistical analysis shows that the heat transport efficiency depends on the strength of the LSCR. When a large-scale wind flows along the ratchets in the direction of their smaller slopes, the convection roll is stronger and the heat transport is larger than the case in which the large-scale wind is directed towards the steeper slope side of the ratchets. Further analysis of the time-averaged temperature profiles indicates that the stronger LSCR in the former case triggers the formation of a secondary vortex inside the roughness cavity, which promotes fluid mixing and results in a higher heat transport efficiency. Remarkably, this result differs from classical Rayleigh–Bénard convection (RBC) with asymmetric ratchets (Jiang et al., Phys. Rev. Lett., vol. 120, 2018, 044501), wherein the heat transfer is stronger when the large-scale wind faces the steeper side of the ratchets. We reveal that the reason for the reversed trend for VC as compared to RBC is that the flow is less turbulent in VC at the same 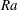$Ra$. Thus, in VC the heat transport is driven primarily by the coherent LSCR, while in RBC the ejected thermal plumes aided by gravity are the essential carrier of heat. The present work provides opportunities for control of heat transport in engineering and geophysical flows.

JFM classification

Turbulent Flows: Turbulent convection
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 873 , 25 August 2019 , pp. 1055 - 1071
Check for updates
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–537.Google Scholar
Batchelor, G. K. 1954 Heat transfer by free convection across a closed cavity between vertical boundaries at different temperatures. Q. Appl. Maths 12 (3), 209–233.Google Scholar
Belleoud, P., Saury, D. & Lemonnier, D. 2018 Coupled velocity and temperature measurements in an air-filled differentially heated cavity at Ra = 1. 2 × 1011 . Intl J. Therm. Sci. 123, 151–161.Google Scholar
Belmonte, A., Tilgner, A. & Libchaber, A. 1994 Temperature and velocity boundary layers in turbulent convection. Phys. Rev. E 50, 269–279.Google Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35, 58.Google Scholar
Ciliberto, S., Cioni, S. & Laroche, C. 1996 Large-scale flow properties of turbulent thermal convection. Phys. Rev. E 54, R5901–R5904.Google Scholar
Corcione, M. 2010 Heat transfer features of buoyancy-driven nanofluids inside rectangular enclosures differentially heated at the sidewalls. Intl J. Therm. Sci. 49 (9), 1536–1546.Google Scholar
Dol, H. & Hanjalic, K. 2001 Computational study of turbulent natural convection in a side-heated near-cubic enclosure at a high Rayleigh number. Intl J. Heat Mass Transfer 44, 2323–2344.Google Scholar
Dou, H.-S. & Jiang, G. 2016 Numerical simulation of flow instability and heat transfer of natural convection in a differentially heated cavity. Intl J. Heat Mass Transfer 103, 370–381.Google Scholar
Du, Y. B. & Tong, P. 1998 Enhanced heat transport in turbulent convection over a rough surface. Phys. Rev. Lett. 81, 987–990.Google Scholar
Du, Y. B. & Tong, P. 2000 Turbulent thermal convection in a cell with ordered rough boundaries. J. Fluid Mech. 407, 57–84.Google Scholar
Fadlun, E. A., Verzicco, R., Orlandi, P. & Mohd-Yusof, J. 2000 Combined immersed-boundary finite-difference methods for three-dimensional complex flow simulations. J. Comput. Phys. 161, 35–60.Google Scholar
Feynman, R. P., Leighton, R. B. & Sands, M. 1963 The Feynman Lectures On Physics, vol. 1. Addison-Wesley.Google Scholar
Goluskin, D. & Doering, C. R. 2016 Bounds for convection between rough boundaries. J. Fluid Mech. 804, 370–386.Google Scholar
Grossmann, S. & Lohse, D. 2001 Thermal convection for large Prandtl number. Phys. Rev. Lett. 86, 3316–3319.Google Scholar
Gvozdić, B., Almras, E., Mathai, V., Zhu, X.-J., van Gils, D. P. M., Verzicco, R., Huisman, S. G., Sun, C. & Lohse, D. 2018 Experimental investigation of heat transport in homogeneous bubbly flow. J. Fluid Mech. 845, 226–244.Google Scholar
Gvozdić, B., Dung, O.-Y., Almras, E., van Gils, D. P. M., Lohse, D., Huisman, S. G. & Sun, C. 2019 Experimental investigation of heat transport in inhomogeneous bubbly flow. Chem. Engng Sci. 198, 260–267.Google Scholar
Hänggi, P. & Marchesoni, F. 2009 Artificial Brownian motors: controlling transport on the nanoscale. Rev. Mod. Phys. 81, 387–442.Google Scholar
Huang, S.-D., Wang, F., Xi, H.-D. & Xia, K.-Q. 2015 Comparative experimental study of fixed temperature and fixed heat flux boundary conditions in turbulent thermal convection. Phys. Rev. Lett. 115, 154502.Google Scholar
Jiang, H.-C., Zhu, X.-J., Mathai, V., Verzicco, R., Lohse, D. & Sun, C. 2018 Controlling heat transport and flow structures in thermal turbulence using ratchet surfaces. Phys. Rev. Lett. 120, 044501.Google Scholar
Johnston, H. & Doering, C. R. 2009 Comparison of turbulent thermal convection between conditions of constant temperature and constant flux. Phys. Rev. Lett. 102, 064501.Google Scholar
Keating, A., Piomelli, U., Bremhorst, K. & Nesic, S. 2004 Large-eddy simulation of heat transfer downstream of a backward-facing step. J. Turbul. 5, N20.Google Scholar
Lagubeau, G., Merrer, M. L., Clanet, C. & Quéré, D. 2011 Leidenfrost on a ratchet. Nature Phys. 7, 395–398.Google Scholar
Lavenuta, G. 1997 Negative temperature coefficient thermistors. Sens. J. Appl. Sens. Tech. 14 (5), 46–55.Google Scholar
Linke, H., Alemán, B. J., Melling, L. D., Taormina, M. J., Francis, M. J., Dow-Hygelund, C. C., Narayanan, V., Taylor, R. P. & Stout, A. 2006 Self-propelled Leidenfrost droplets. Phys. Rev. Lett. 96, 154502.Google Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Ann. Rev. Fluid Mech. 42, 335–364.Google Scholar
Narezo Guzman, D., Xie, Y.-B., Chen, S.-Y., Fernandez Rivas, D., Sun, C., Lohse, D. & Ahlers, G. 2016 Heat-flux enhancement by vapour-bubble nucleation in Rayleigh–Bénard turbulence. J. Fluid Mech. 787, 331–366.Google Scholar
Ng, C. S., Ooi, A., Lohse, D. & Chung, D. 2015 Vertical natural convection: application of the unifying theory of thermal convection. J. Fluid Mech. 764, 349–361.Google Scholar
Ng, C. S., Ooi, A., Lohse, D. & Chung, D. 2017 Changes in the boundary-layer structure at the edge of the ultimate regime in vertical natural convection. J. Fluid Mech. 825, 550–572.Google Scholar
Ng, C. S., Ooi, A., Lohse, D. & Chung, D. 2018 Bulk scaling in wall-bounded and homogeneous vertical natural convection. J. Fluid Mech. 841, 825–850.Google Scholar
Paolucci, S. & Chenoweth, D. R. 1989 Transition to chaos in a differentially heated vertical cavity. J. Fluid Mech. 201, 379–410.Google Scholar
Patterson, J. & Imberger, J. 1980 Unsteady natural convection in a rectangular cavity. J. Fluid Mech. 100 (1), 65–86.Google Scholar
van der Poel, E. P., Ostilla-Mónico, R., Donners, J. & Verzicco, R. 2015 A pencil distributed finite difference code for strongly turbulent wall-bounded flows. Comput. Fluids 116, 10–16.Google Scholar
Prakash, M., Quéré, D. & Bush, J. W. M. 2008 Surface tension transport of prey by feeding shorebirds: the capillary ratchet. Science 320, 931–934.Google Scholar
Qiu, X. L., Xia, K.-Q. & Tong, P. 2005 Experimental study of velocity boundary layer near a rough conducting surface in turbulent natural convection. J. Turbul. 6, 1–13.Google Scholar
Roche, P. E., Castaing, B., Chabaud, B. & Hebral, B. 2001 Observation of the 1/2 power law in Rayleigh–Bénard convection. Phys. Rev. E 63, 045303.Google Scholar
Salort, J., Liot, O., Rusaouen, E., Seychelles, F., Tisserand, J.-C., Creyssels, M., Castaing, B. & Chilla, F. 2014 Thermal boundary layer near roughnesses in turbulent Rayleigh–Bénard convection: flow structure and multistability. Phys. Fluids 26, 015112.Google Scholar
Shakerin, S., Bohn, M. & Loehrke, R. I. 1988 Natural convection in an enclosure with discrete roughness elements on a vertical heated wall. Intl J. Heat Mass Transfer 31 (7), 1423–1430.Google Scholar
Shen, Y., Tong, P. & Xia, K.-Q. 1996 Turbulent convection over rough surfaces. Phys. Rev. Lett. 76, 908–911.Google Scholar
Shishkina, O. 2016 Momentum and heat transport scalings in laminar vertical convection. Phys. Rev. E 93, 051102.Google Scholar
Shishkina, O. & Wagner, C. 2011 Modelling the influence of wall roughness on heat transfer in thermal convection. J. Fluid Mech. 686, 568–582.Google Scholar
Smoluchowski, M. v. 1912 Experimentally demonstrable molecular phenomena contradicting convectional thermodynamics. Phys. Z. 13, 1069–1080.Google Scholar
Stevens, R. J. A. M., Lohse, D. & Verzicco, R. 2011 Prandtl and Rayleigh number dependence of heat transport in high Rayleigh number thermal convection. J. Fluid Mech. 688, 31–43.Google Scholar
Stringano, G., Pascazio, G. & Verzicco, R. 2006 Turbulent thermal convection over grooved plates. J. Fluid Mech. 557, 307–336.Google Scholar
Tisserand, J. C., Creyssels, M., Gasteuil, Y., Pabiou, H., Gibert, M., Castaing, B. & Chilla, F. 2011 Comparison between rough and smooth plates within the same Rayleigh–Bénard cell. Phys. Fluids 23 (1), 015105.Google Scholar
Tiwari, R. K. & Das, M. K. 2007 Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Intl J. Heat Mass Transfer 50 (9), 2002–2018.Google Scholar
Toppaladoddi, S., Succi, S. & Wettlaufer, J. S. 2017 Roughness as a route to the ultimate regime of thermal convection. Phys. Rev. Lett. 118, 074503.Google Scholar
Van Oudenaarden, A. & Boxer, S. G. 1999 Brownian ratchets: molecular separations in lipid bilayers supported on patterned arrays. Science 285 (5430), 1046–1048.Google Scholar
Verzicco, R. & Orlandi, P. 1996 A finite-difference scheme for three-dimensional incompressible flow in cylindrical coordinates. J. Comput. Phys. 123, 402–413.Google Scholar
Villermaux, E. 1998 Transfer at rough sheared interfaces. Phys. Rev. Lett. 81, 4859–4862.Google Scholar
Wagner, S. & Shishkina, O. 2015 Heat flux enhancement by regular surface roughness in turbulent thermal convection. J. Fluid Mech. 763, 109–135.Google Scholar
Xie, Y.-C. & Xia, K.-Q. 2017 Turbulent thermal convection over rough plates with varying roughness geometries. J. Fluid Mech. 825, 573–599.Google Scholar
Xin, S.-h & Le Qur, P. 1995 Direct numerical simulations of two-dimensional chaotic natural convection in a differentially heated cavity of aspect ratio 4. J. Fluid Mech. 304, 87–118.Google Scholar
Xu, F., Patterson, J. C. & Lei, C.-W. 2009 Transient natural convection flows around a thin fin on the sidewall of a differentially heated cavity. J. Fluid Mech. 639, 261–290.Google Scholar
Yousaf, M. & Usman, S. 2015 Natural convection heat transfer in a square cavity with sinusoidal roughness elements. Intl J. Heat Mass Transfer 90, 180–190.Google Scholar
Zhang, Y.-Z., Sun, C., Bao, Y. & Zhou, Q. 2018 How surface roughness reduces heat transport for small roughness heights in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 836, R2.Google Scholar
Zhou, Q. & Xia, K.-Q. 2013 Thermal boundary layer structure in turbulent Rayleigh–Bénard convection in a rectangular cell. J. Fluid Mech. 721, 199–224.Google Scholar
Zhu, X., Ostilla-Monico, R., Verzicco, R. & Lohse, D. 2016 Direct numerical simulation of Taylor–Couette flow with grooved walls: torque scaling and flow structure. J. Fluid Mech. 794, 746–774.Google Scholar
Zhu, X.-J., Phillips, E., Spandan, V., Donners, J., Ruetsch, G., Romero, J., Ostilla-Mónico, R., Yang, Y., Lohse, D., Verzicco, R., Fatica, M. & Stevens, R. A. J. M. 2018 AFiD-GPU: a versatile Navier–Stokes solver for wall-bounded turbulent flows on GPU clusters. Comput. Phys. Commun. 229, 199–210.Google Scholar
Zhu, X.-J., Stevens, R. J. A. M., Verzicco, R. & Lohse, D. 2017 Roughness-facilitated local 1/2 scaling does not imply the onset of the ultimate regime of thermal convection. Phys. Rev. Lett. 119, 154501.Google Scholar

Jiang et al. supplementary movie 1

Shadowgraph visualization for VC-case A

Download Jiang et al. supplementary movie 1(Video)
Video 2.8 MB

Jiang et al. supplementary movie 2

Shadowgraph visualization for VC-case B

Download Jiang et al. supplementary movie 2(Video)
Video 3 MB