Article contents
Analysis of complex singularities in high-Reynolds-number Navier–Stokes solutions
Published online by Cambridge University Press: 17 April 2014
Abstract
Numerical solutions of the laminar Prandtl boundary-layer and Navier–Stokes equations are considered for the case of the two-dimensional uniform flow past an impulsively-started circular cylinder. The various viscous–inviscid interactions that occur during the unsteady separation process are investigated by applying complex singularity analysis to the wall shear and streamwise velocity component of the two solutions. This is carried out using two different methodologies, namely a singularity-tracking method and the Padé approximation. It is shown how the van Dommelen and Shen singularity that occurs in solutions of the Prandtl boundary-layer equations evolves in the complex plane before leading to a separation singularity in finite time. Navier–Stokes solutions, computed at different Reynolds numbers in the range 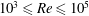 $10^3 \leq Re \leq 10^5$, are characterized by the presence of various complex singularities that can be related to different physical interactions acting over multiple spatial scales. The first interaction developing in the separation process is large-scale interaction that is visible for all the Reynolds numbers considered, and it signals the first relevant differences between the Prandtl and Navier–Stokes solutions. For
$10^3 \leq Re \leq 10^5$, are characterized by the presence of various complex singularities that can be related to different physical interactions acting over multiple spatial scales. The first interaction developing in the separation process is large-scale interaction that is visible for all the Reynolds numbers considered, and it signals the first relevant differences between the Prandtl and Navier–Stokes solutions. For  $Re\geq O(10^4)$, a small-scale interaction follows the large-scale interaction. The onset of these interactions is related to the characteristic changes of the streamwise pressure gradient on the circular cylinder. Even if these interactions physically differ from that prescribed by the Prandtl solution, and they set a possible limit on the comparison of Prandtl solutions with Navier–Stokes solutions, it is shown how the asymptotic validity of boundary-layer theory is strongly supported by the results that have been obtained through the complex singularity analysis.
$Re\geq O(10^4)$, a small-scale interaction follows the large-scale interaction. The onset of these interactions is related to the characteristic changes of the streamwise pressure gradient on the circular cylinder. Even if these interactions physically differ from that prescribed by the Prandtl solution, and they set a possible limit on the comparison of Prandtl solutions with Navier–Stokes solutions, it is shown how the asymptotic validity of boundary-layer theory is strongly supported by the results that have been obtained through the complex singularity analysis.
JFM classification
- Type
- Papers
- Information
- Copyright
- © 2014 Cambridge University Press
References
- 25
- Cited by


