Article contents
On first exit times and their means for Brownian bridges
Published online by Cambridge University Press: 01 October 2019
Abstract
For a Brownian bridge from 0 to y, we prove that the mean of the first exit time from the interval 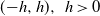 $\left( -h,h \right),h>0$
, behaves as
$\left( -h,h \right),h>0$
, behaves as 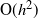 ${\mathrm{O}}(h^2)$
when
${\mathrm{O}}(h^2)$
when 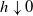 $h
\downarrow 0$
. Similar behaviour is also seen to hold for the three-dimensional Bessel bridge. For the Brownian bridge and three-dimensional Bessel bridge, this mean of the first exit time has a puzzling representation in terms of the Kolmogorov distribution. The result regarding the Brownian bridge is applied to provide a detailed proof of an estimate needed by Walsh to determine the convergence of the binomial tree scheme for European options.
$h
\downarrow 0$
. Similar behaviour is also seen to hold for the three-dimensional Bessel bridge. For the Brownian bridge and three-dimensional Bessel bridge, this mean of the first exit time has a puzzling representation in terms of the Kolmogorov distribution. The result regarding the Brownian bridge is applied to provide a detailed proof of an estimate needed by Walsh to determine the convergence of the binomial tree scheme for European options.
Keywords
MSC classification
- Type
- Research Papers
- Information
- Copyright
- © Applied Probability Trust 2019
References
- 1
- Cited by


