Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-01T16:55:45.739Z Has data issue: false hasContentIssue false
  • English
  • Français

Multivariate Poisson flows on Markov step processes

Published online by Cambridge University Press:  14 July 2016

Frederick J. Beutler  and
Benjamin Melamed
Frederick J. Beutler*
Affiliation:
University of Michigan
Benjamin Melamed*
Affiliation:
Bell Laboratories
*
∗ Postal address: Computer, Information and Control Engineering Program, University of Michigan, Ann Arbor, MI 48109, U.S.A.
∗∗ Postal address: Bell Telephone Laboratories, Holmdel, NJ 07733, U.S.A.
Get access Rights & Permissions [Opens in a new window]

Abstract

A Markov step process Z equipped with a possibly non-denumerable state space X can model a variety of queueing, communication and computer networks. The analysis of such networks can be facilitated if certain traffic flows consist of mutually independent Poisson processes with respective deterministic intensities λi (t). Accordingly, we define the multivariate counting process N = (N1, N2, · ·· Nc) induced by Z; a count in Ni occurs whenever Z jumps from x (χ into a (possibly empty) target set 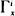. We study N through the infinitesimal operator à of the augmented Markov process W = (Z, N), and the integral relationship connecting à with the transition operator Tt of W. It is then shown that Ni depends on a non-negative function ri defined on χ; ri (x) may be interpreted as the expected rate of increase in Ni, given that Z is in state x.

A multivariate N is Poisson (i.e., composed of mutually independent Poisson streams Ni) if and only if simultaneous jumps are impossible in a certain sense, and if the conditional expectation E[ri (Z(t) | 𝒩i] = E[ri (Z(t))] for i = 1, 2, ···, c and each t ≧ 0, where 𝒩i is the σ-algebra σ{N(s), s ≦ t}. Necessary and sufficient conditions are also specified that, for each s ≦ t, the variates [Ni(t)-Ni(s)] are mutually independent Poisson distributed; this involves a weakened version of E[ri(Z(v)) | N(v) – N(u)] = E [ri(Z(v))] for i = 1, 2, ···, c and all 0 ≦ u ≦ v.

It is shown that the above criteria are automatically met by the more stringent classical requirement that N(t) and Z(t) be independent for each t ≧ 0.

Keywords

POISSON PROCESSMULTIVARIATE POISSON PROCESSMARKOV STEP PROCESSMARKOV PROCESSFLOWS IN QUEUEING SYSTEMSQUEUEING SYSTEMSQUEUEING NETWORKSCOUNTING PROCESSES
Type
Research Papers
Information
Journal of Applied Probability , Volume 19 , Issue 2 , June 1982 , pp. 289 - 300
Copyright
Copyright © Applied Probability Trust 1982 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Research sponsored by the Air Force Office of Scientific Research, AFSC, USAF, under Grant No. AFOSR-76-2903E, and by the National Science Foundation under Grant No. ENG-75-20223.

References

[1] Beutler, F. J. and Melamed, B. (1978) Decomposition and customer streams of feedback networks in equilibrium. Operat. Res. 26, 1059–1072.CrossRefGoogle Scholar
[2] Beutler, F. J., Melamed, B. and Zeigler, B. (1977) Equilibrium properties of arbitrarily interconnected networks. In Multivariate Analysis IV, ed. Krishnaiah, P. R., North-Holland, Amsterdam, 351–370.Google Scholar
[3] Brémaud, P. (1980) Point Processes and Queues: Martingale Dynamics. Ecole Nationale Supérieure de Techniques Avancés, Paris.Google Scholar
[4] Burke, P. J. (1956) Output of a queueing system. Operat. Res. 4, 699–704.CrossRefGoogle Scholar
[5] Chandy, K. M., Howard, J. H. Jr and Towsley, D. F. (1977) Product form and local balance in queueing systems. J. Assoc. Comput. Mach. 24, 250–263.Google Scholar
[6] Dynkin, E. B. (1965) Markov Processes, I. Academic Press, New York.Google Scholar
[7] Gelenbe, E. and Muntz, R. R. (1976) Probabilistic models of computer systems — Part I (exact results). Acta Informatica 7, 35–60.Google Scholar
[8] Jackson, J. R. (1957) Networks of waiting lines. Operat. Res. 5, 518–521.Google Scholar
[9] Keilson, J. (1979) Markov Chain Models — Rarity and Exponentiality. Springer-Verlag, New York.CrossRefGoogle Scholar
[10] Kelly, F. P. (1976) Networks of queues. Adv. Appl. Prob. 9, 416–432.CrossRefGoogle Scholar
[11] Kobayashi, H. and Konheim, A. G. (1977) Queueing models for computer communication system analysis. IEEE Trans. Commun. COM-25, 2–29.Google Scholar
[12] Loève, M. (1963) Probability Theory, 3rd edn. Van Nostrand, New York.Google Scholar
[13] Melamed, B. (1979) On Poisson traffic processes in discrete-state Markovian systems with applications to queueing theory. Adv. Appl. Prob. 11, 218–239.Google Scholar
[14] Melamed, B. (1979) Characterization of Poisson traffic streams in Jackson queueing networks. Adv. Appl. Prob. 11, 422–438.Google Scholar
[15] Muntz, R. (1972) Poisson departure processes and queueing networks. IBM Research Report RC-4145, IBM Thomas J. Watson Research Center, Yorktown Heights, NY.Google Scholar
[16] Reich, E. (1957) Waiting lines when queues are in tandem. Ann. Math. Statist. 28, 768–773.Google Scholar