Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-17T17:21:58.249Z Has data issue: false hasContentIssue false
  • English
  • Français

A dichotomy of sets via typical differentiability

Part of: Classical measure theory Real functions Functions of several variables Set theory

Published online by Cambridge University Press:  04 November 2020

Michael Dymond  and
Olga Maleva
Michael Dymond
Affiliation:
Institut für Mathematik, Universität Innsbruck, Technikerstraße 13, 6020 Innsbruck, Austria; E-mail: Michael.Dymond@uibk.ac.at
Olga Maleva
Affiliation:
School of Mathematics, University of Birmingham, Birmingham, B15 2TTUnited Kingdom; E-mail: O.Maleva@bham.ac.uk

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We obtain a criterion for an analytic subset of a Euclidean space to contain points of differentiability of a typical Lipschitz function: namely, that it cannot be covered by countably many sets, each of which is closed and purely unrectifiable (has a zero-length intersection with every 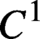$C^1$ curve). Surprisingly, we establish that any set failing this criterion witnesses the opposite extreme of typical behaviour: in any such coverable set, a typical Lipschitz function is everywhere severely non-differentiable.

Keywords

differentiability of Lipschitz functionsBaire categorypurely unrectifiableBanach-Mazur game

MSC classification

Primary: 26B05: Continuity and differentiation questions 26A16: Lipschitz (Hölder) classes
Secondary: 28A05: Classes of sets (Borel fields, $sigma$-rings, etc.), measurable sets, Suslin sets, analytic sets 26A21: Classification of real functions; Baire classification of sets and functions 03E15: Descriptive set theory 28A75: Length, area, volume, other geometric measure theory
Type
Analysis
Information
Forum of Mathematics, Sigma , Volume 8 , 2020 , e41
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s), 2020. Published by Cambridge University Press

References

Alberti, G., Csörnyei, M., and Preiss, D., ‘Differentiability of Lipschitz functions, structure of null sets, and other problems’, in Proceedings of the International Congress of Mathematicians 2010 (ICM 2010) (In 4 Volumes) Vol. I: Plenary Lectures and Ceremonies Vols. II–IV: Invited Lectures (World Scientific, 2010), 1379–1394.Google Scholar
Banach, S., ‘Über die Baire’sche Kategorie gewisser Funktionenmengen’, Studia Mathematica 3(1):174–179, 1931.CrossRefGoogle Scholar
Choquet, G., ‘Application des propriétés descriptives de la fonction contingent à la théorie des fonctions de variable réelle et à la géométrie différentielle des variétés cartésiennes’, J. Math. Pures Appl . 9(26):115–226 (1948), 1947.Google Scholar
Csörnyei, M., Preiss, D., and Tiser, J., ‘Lipschitz functions with unexpectedly large sets of nondifferentiability points’, 2005, Abstract and Applied Analysis, (4), 361–373, 2005.CrossRefGoogle Scholar
Doré, M. and Maleva, O., ‘A universal differentiability set in Banach spaces with separable dual’, Journal of Functional Analysis 261(6):1674–1710, 2011.CrossRefGoogle Scholar
Dymond, M., ‘Typical differentiability within an exceptionally small set’, J. Math. Anal. Appl. 490 (2020), no. 2.CrossRefGoogle Scholar
Dymond, M. and Maleva, O., ‘Differentiability inside sets with Minkowski dimension one’, Michigan Math. J . 65(3):613–636, 08 2016.CrossRefGoogle Scholar
Federer, H., Geometric Measure Theory, Classics in Mathematics, (Springer, 1996).CrossRefGoogle Scholar
Fitzpatrick, S., ‘Differentiation of real-valued functions and continuity of metric projections’, Proceedings of the American Mathematical Society 91(4):544–548, 1984.CrossRefGoogle Scholar
Hewitt, E. and Stromberg, K., Real and Abstract Analysis, (Springer-Verlag, New York-Heidelberg, 1975).Google Scholar
Kechris, A., Classical Descriptive Set Theory, vol. 156 (Springer Science & Business Media, 2012).Google Scholar
Kirszbraun, M., ‘Über die zusammenziehende und Lipschitzsche Transformationen’, Fundamenta Mathematicae 22(1):77–108, 1934.CrossRefGoogle Scholar
Kuratowski, C., Topology I, (Academic Press New York and London, 1966).Google Scholar
Le Donne, E., Pinamonti, A., and Speight, G., ‘Universal differentiability sets and maximal directional derivatives in Carnot groups’, J. Math. Pures Appl. 9(121):83–112, 2019.CrossRefGoogle Scholar
Maleva, O. and Preiss, D., ‘Cone unrectifiable sets and non-differentiability of Lipschitz functions’, Israel Journal of Mathematics 232 (2019), no. 1, 75–108.CrossRefGoogle Scholar
Mattila, P., Geometry of Sets and Measures in Euclidean Spaces: Fractals and Rectifiability, Cambridge Studies in Advanced Mathematics (Cambridge University Press, 1995).CrossRefGoogle Scholar
Merlo, A., ‘Full non-differentiability of typical Lipschitz functions’, arXiv:1906.08366, 2019.Google Scholar
Petruska, G., ‘On Borel sets with small cover: A problem of M. Laczkovich’, Real Analysis Exchange 18(2):330–338, 1992.CrossRefGoogle Scholar
Pinamonti, A. and Speight, G., ‘A measure zero universal differentiability set in the Heisenberg group’, Math. Ann. 368(1–2):233–278, 2017.CrossRefGoogle Scholar
Preiss, D. ‘Differentiability of Lipschitz functions on Banach spaces’, Journal of Functional Analysis 91(2):312–345, 1990.CrossRefGoogle Scholar
Preiss, D. and Speight, G., Differentiability of Lipschitz functions in Lebesgue null sets’, Invent. Math. 199(2):517–559, 2015.CrossRefGoogle Scholar
Preiss, D. and Tišer, J., ‘Points of non-differentiability of typical Lipschitz functions’, Real Analysis Exchange 20(1):219–226, 1994.CrossRefGoogle Scholar
Rădulescu, S. and Rădulescu, M., ‘Local inversion theorems without assuming continuous differentiability’, J. Math. Anal. Appl. 138(2):581–590, 1989.CrossRefGoogle Scholar
Solecki, S., ‘Covering analytic sets by families of closed sets’, J. Symbolic Logic 59(3):1022–1031, (09) 1994.CrossRefGoogle Scholar
Zahorski, Z., ‘ Sur les ensembles des points de divergence de certaines intégrales singulières’, Ann. Soc. Polon. Math. 19:66–105 (1947), 1946.Google Scholar