Hostname: page-component-7bb8b95d7b-l4ctd Total loading time: 0 Render date: 2024-09-20T07:49:55.690Z Has data issue: false hasContentIssue false
  • English
  • Français

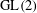THE WEIGHT PART OF SERRE’S CONJECTURE FOR $\text{GL}(2)$

Part of: Discontinuous groups and automorphic forms

Published online by Cambridge University Press:  05 February 2015

TOBY GEE ,
TONG LIU  and
DAVID SAVITT
TOBY GEE
Affiliation:
Department of Mathematics, Imperial College London SW7 2RH, UK; toby.gee@imperial.ac.uk
TONG LIU
Affiliation:
Department of Mathematics, Purdue University, West Lafayette, IN 47907, USA; tongliu@math.purdue.edu
DAVID SAVITT
Affiliation:
Department of Mathematics, University of Arizona, Tucson, AZ 85721, USA; savitt@math.arizona.edu

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

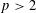Let $p>2$ be prime. We use purely local methods to determine the possible reductions of certain two-dimensional crystalline representations, which we call pseudo-Barsotti–Tate representations, over arbitrary finite extensions of 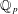$\mathbb{Q}_{p}$. As a consequence, we establish (under the usual Taylor–Wiles hypothesis) the weight part of Serre’s conjecture for 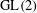$\text{GL}(2)$ over arbitrary totally real fields.

MSC classification

Primary: 11F33: Congruences for modular and $p$-adic modular forms
Secondary: 11F80: Galois representations
Type
Research Article
Information
Forum of Mathematics, Pi , Volume 3 , 2015 , e2
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/3.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s) 2015

References

Buzzard, K., Diamond, F. and Jarvis, F., ‘On Serre’s conjecture for mod l Galois representations over totally real fields’, Duke Math. J. 155(1) (2010), 105–161.CrossRefGoogle Scholar
Barnet-Lamb, T., Gee, T. and Geraghty, D., ‘Serre weights for rank two unitary groups’, Math. Ann. 356(4) (2013), 1551–1598.Google Scholar
Barnet-Lamb, T., Gee, T., Geraghty, D. and Taylor, R., ‘Potential automorphy and change of weight’, Ann. of Math. (2) 179(2) (2014), 501–609.CrossRefGoogle Scholar
Breuil, C., ‘Représentations p-adiques semi-stables et transversalité de Griffiths’, Math. Ann. 307(2) (1997), 191–224.CrossRefGoogle Scholar
Breuil, C., ‘Sur un problème de compatibilité local-global modulo p pour GL2’, J. Reine Angew. Math. 92 (2014), 1–76.CrossRefGoogle Scholar
Breuil, C. and Mézard, A., ‘Multiplicités modulaires et représentations de GL2(Zp) et de Gal(ℚp∕ℚp) en l = p’, Duke Math. J. 115(2) (2002), 205–310. With an appendix by G. Henniart.Google Scholar
Breuil, C. and Mézard, A., ‘Mulitplicités modulaires raffinées’, Bull. Soc. Math. France 142 (2012), 127–175.Google Scholar
Breuil, C. and Paškūnas, V., ‘Towards a modulo p Langlands correspondence for GL2’, Mem. Amer. Math. Soc. 216(1016) (2012), vi+114.Google Scholar
Conrad, B., ‘Lifting global representations with local properties’, Preprint, 2011.Google Scholar
Coleman, R. F. and Voloch, J. F., ‘Companion forms and Kodaira–Spencer theory’, Invent. Math. 110(2) (1992), 263–281.Google Scholar
Diamond, F. and Savitt, D., ‘Serre weights for locally reducible two-dimensional Galois representations’, J. Inst. Math. Jussieu, to appear.Google Scholar
Edixhoven, B., ‘The weight in Serre’s conjectures on modular forms’, Invent. Math. 109(3) (1992), 563–594.CrossRefGoogle Scholar
Emerton, M., Gee, T. and Savitt, D., ‘Lattices in the cohomology of Shimura curves’, Invent. Math., to appear.Google Scholar
Fontaine, J.-M., ‘Representations p-adiques semi-stables’, Astérisque 223 (1994), 113–184.Google Scholar
Gee, T., ‘A modularity lifting theorem for weight two Hilbert modular forms’, Math. Res. Lett. 13(5–6) (2006), 805–811.CrossRefGoogle Scholar
Gee, T., ‘Automorphic lifts of prescribed types’, Math. Ann. 350(1) (2011), 107–144.Google Scholar
Gee, T., ‘On the weights of mod p Hilbert modular forms’, Invent. Math. 184 (2011), 1–46. doi:10.1007/s00222-010-0284-5..CrossRefGoogle Scholar
Gee, T. and Kisin, M., ‘The Breuil–Mézard conjecture for potentially Barsotti–Tate representations’, Forum of Mathematics, Pi 2 (2014), e1 (56 pages).Google Scholar
Gee, T., Liu, T. and Savitt, D., ‘Crystalline extensions and the weight part of Serre’s conjecture’, Algebra Number Theory 6(7) (2012), 1537–1559.CrossRefGoogle Scholar
Gee, T., Liu, T. and Savitt, D., ‘The Buzzard–Diamond–Jarvis conjecture for unitary groups’, J. Amer. Math. Soc. 27(2) (2014), 389–435.CrossRefGoogle Scholar
Gee, T. and Savitt, D., ‘Serre weights for mod p Hilbert modular forms: the totally ramified case’, J. reine angew. Math. 660 (2011), 1–26.Google Scholar
Gross, B. H., ‘A tameness criterion for Galois representations associated to modular forms (mod p)’, Duke Math. J. 61(2) (1990), 445–517.Google Scholar
Kisin, M., ‘Crystalline representations and F-crystals’, inAlgebraic Geometry and Number Theory, Progress in Mathematics, 253 (Birkhäuser Boston, Boston, MA, 2006), 459–496.CrossRefGoogle Scholar
Kisin, M., ‘Moduli of finite flat group schemes, and modularity’, Ann. of Math. (2) 170(3) (2009), 1085–1180.Google Scholar
Liu, T., ‘On lattices in semi-stable representations: a proof of a conjecture of Breuil’, Compos. Math. 144(1) (2008), 61–88.Google Scholar
Newton, J., ‘Serre weights and Shimura curves’, Proc. Lond. Math. Soc. (3) 108(6) (2014), 1471–1500.CrossRefGoogle Scholar
Newton, J. and Yoshida, T., ‘Shimura curves, the Drinfeld curve and Serre weights’, Preprint, 2014.Google Scholar
Savitt, D., ‘On a conjecture of Conrad, Diamond, and Taylor’, Duke Math. J. 128(1) (2005), 141–197.Google Scholar
Schein, M. M., ‘Weights in Serre’s conjecture for Hilbert modular forms: the ramified case’, Israel J. Math. 166 (2008), 369–391.CrossRefGoogle Scholar
Serre, J.-P., ‘Sur les représentations modulaires de degré 2 de Gal(ℚ∕ℚ)’, Duke Math. J. 54(1) (1987), 179–230.CrossRefGoogle Scholar