Article contents
Impact of the variations of the mixing length in a first order turbulentclosure system
Published online by Cambridge University Press: 15 May 2002
Abstract
This paper is devoted to the study of a turbulent
circulation model. Equations are derived from the “Navier-Stokes turbulent
kinetic energy” system. Some simplifications are performed but attention
is focused on non linearities linked to turbulent eddy viscosity $\nu _{t}$ . The mixing length $\ell $
. The mixing length $\ell $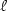 acts as a parameter which controls the
turbulent part in $\nu _{t}$
acts as a parameter which controls the
turbulent part in $\nu _{t}$ . The main theoretical results that we have
obtained concern the uniqueness of the solution for bounded eddy viscosities
and small values of $\ell $
. The main theoretical results that we have
obtained concern the uniqueness of the solution for bounded eddy viscosities
and small values of $\ell $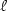 and its asymptotic decreasing as $\ell
\rightarrow \infty $
and its asymptotic decreasing as $\ell
\rightarrow \infty $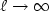 in more general cases. Numerical experiments
illustrate but also allow to extend these theoretical results: uniqueness is
proved only for $\ell $
in more general cases. Numerical experiments
illustrate but also allow to extend these theoretical results: uniqueness is
proved only for $\ell $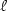 small enough while regular solutions are numerically
obtained for any values of $\ell $
small enough while regular solutions are numerically
obtained for any values of $\ell $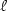 . A convergence theorem is proved for
turbulent kinetic energy: $k_{\ell }\rightarrow 0$
. A convergence theorem is proved for
turbulent kinetic energy: $k_{\ell }\rightarrow 0$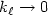 as $\ell \rightarrow
\infty ,$
as $\ell \rightarrow
\infty ,$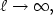 but for velocity $u_{\ell }$
but for velocity $u_{\ell }$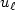 we obtain only weaker results.
Numerical results allow to conjecture that $k_{\ell }\rightarrow 0,$
we obtain only weaker results.
Numerical results allow to conjecture that $k_{\ell }\rightarrow 0,$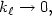 $\nu
_{t}\rightarrow \infty $
$\nu
_{t}\rightarrow \infty $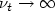 and $u_{\ell }\rightarrow 0$
and $u_{\ell }\rightarrow 0$ as $\ell \rightarrow
\infty .$
as $\ell \rightarrow
\infty .$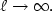 So we can conjecture that this classical turbulent model obtained
with one degree of closure regularizes the solution.
So we can conjecture that this classical turbulent model obtained
with one degree of closure regularizes the solution.
- Type
- Research Article
- Information
- ESAIM: Mathematical Modelling and Numerical Analysis , Volume 36 , Issue 2 , March 2002 , pp. 345 - 372
- Copyright
- © EDP Sciences, SMAI, 2002
References
- 12
- Cited by


