Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-18T08:42:35.700Z Has data issue: false hasContentIssue false
  • English
  • Français

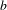Uniform Diophantine approximation related to $b$-ary and ${\it\beta}$-expansions

Published online by Cambridge University Press:  04 August 2014

YANN BUGEAUD  and
LINGMIN LIAO
YANN BUGEAUD
Affiliation:
Université de Strasbourg, IRMA, 7, rue René Descartes, 67084 Strasbourg, France email bugeaud@math.unistra.fr
LINGMIN LIAO
Affiliation:
Université Paris-Est Créteil, LAMA 61, av Général de Gaulle, 94000 Créteil, France email lingmin.liao@u-pec.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

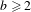Let $b\geq 2$ be an integer and 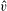$\hat{v}$ a real number. Among other results, we compute the Hausdorff dimension of the set of real numbers ${\it\xi}$ with the property that, for every sufficiently large integer 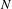$N$, there exists an integer $n$ such that $1\leq n\leq N$ and the distance between 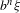$b^{n}{\it\xi}$ and its nearest integer is at most equal to 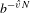$b^{-\hat{v}N}$. We further solve the same question when replacing 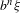$b^{n}{\it\xi}$ by 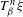$T_{{\it\beta}}^{n}{\it\xi}$, where $T_{{\it\beta}}$ denotes the classical 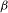${\it\beta}$-transformation.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 36 , Issue 1 , February 2016 , pp. 1 - 22
Copyright
© Cambridge University Press, 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Amou, M. and Bugeaud, Y.. Expansions in integer bases and exponents of Diophantine approximation. J. Lond. Math. Soc. 81 (2010), 297–316.CrossRefGoogle Scholar
Beresnevich, V. and Velani, S.. A mass transference principle and the Duffin–Schaeffer conjecture for Hausdorff measures. Ann. of Math. (2) 164 (2006), 971–992.CrossRefGoogle Scholar
Borosh, I. and Fraenkel, A. S.. A generalization of Jarník’s theorem on Diophantine approximations. Indag. Math. 34 (1972), 193–201.CrossRefGoogle Scholar
Bugeaud, Y.. Distribution Modulo One and Diophantine Approximation (Cambridge Tracts in Mathematics, 193). Cambridge University Press, Cambridge, 2012.CrossRefGoogle Scholar
Bugeaud, Y. and Laurent, M.. Exponents of Diophantine approximation and Sturmian continued fractions. Ann. Inst. Fourier (Grenoble) 55 (2005), 773–804.CrossRefGoogle Scholar
Bugeaud, Y. and Wang, B.-W.. Distribution of full cylinders and the Diophantine properties of the orbits in ${\it\beta}$-expansions, J. Fractal Geometry to appear.Google Scholar
Falconer, K.. Techniques in Fractal Geometry. John Wiley & Sons, Ltd, Chichester, 1997.Google Scholar
Fan, A.-H. and Wang, B.-W.. On the lengths of basic intervals in beta expansions. Nonlinearity 25 (2012), 1329–1343.CrossRefGoogle Scholar
Khintchine, A. Ya.. Über eine Klasse linearer diophantischer approximationen. Rend. Circ. Mat. Palermo 50 (1926), 170–195.CrossRefGoogle Scholar
Levesley, J., Salp, C. and Velani, S. L.. On a problem of K Mahler: Diophantine approximation and Cantor sets. Math. Ann. 338 (2007), 97–118.CrossRefGoogle Scholar
Li, B., Persson, T., Wang, B.-W. and Wu, J.. Diophantine approximation of the orbit of 1 in the dynamical system of beta expansions. Math. Z. 276 (2014), 799–827.CrossRefGoogle Scholar
Parry, W.. On the 𝛽-expansions of real numbers. Acta Math. Acad. Sci. Hung. 11 (1960), 401–416.CrossRefGoogle Scholar
Persson, T. and Schmeling, J.. Dyadic Diophantine approximation and Katok’s horseshoe approximation. Acta Arith. 132 (2008), 205–230.CrossRefGoogle Scholar
Rényi, A.. Representations for real numbers and their ergodic properties. Acta Math. Acad. Sci. Hung. 8 (1957), 477–493.CrossRefGoogle Scholar
Shen, L. M. and Wang, B. W.. Shrinking target problems for beta-dynamical system. Sci. China Math. 56 (2013), 91–104.CrossRefGoogle Scholar