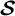 $\mathcal{\textbf{S}}$-adic characterization of minimal ternary dendric shifts
$\mathcal{\textbf{S}}$-adic characterization of minimal ternary dendric shiftsPublished online by Cambridge University Press: 02 September 2021
Dendric shifts are defined by combinatorial restrictions of the extensions of the words in their languages. This family generalizes well-known families of shifts such as Sturmian shifts, Arnoux–Rauzy shifts and codings of interval exchange transformations. It is known that any minimal dendric shift has a primitive 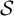 $\mathcal {S}$
-adic representation where the morphisms in
$\mathcal {S}$
-adic representation where the morphisms in 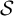 $\mathcal {S}$
are positive tame automorphisms of the free group generated by the alphabet. In this paper, we investigate those
$\mathcal {S}$
are positive tame automorphisms of the free group generated by the alphabet. In this paper, we investigate those 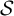 $\mathcal {S}$
-adic representations, heading towards an
$\mathcal {S}$
-adic representations, heading towards an 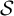 $\mathcal {S}$
-adic characterization of this family. We obtain such a characterization in the ternary case, involving a directed graph with two vertices.
$\mathcal {S}$
-adic characterization of this family. We obtain such a characterization in the ternary case, involving a directed graph with two vertices.