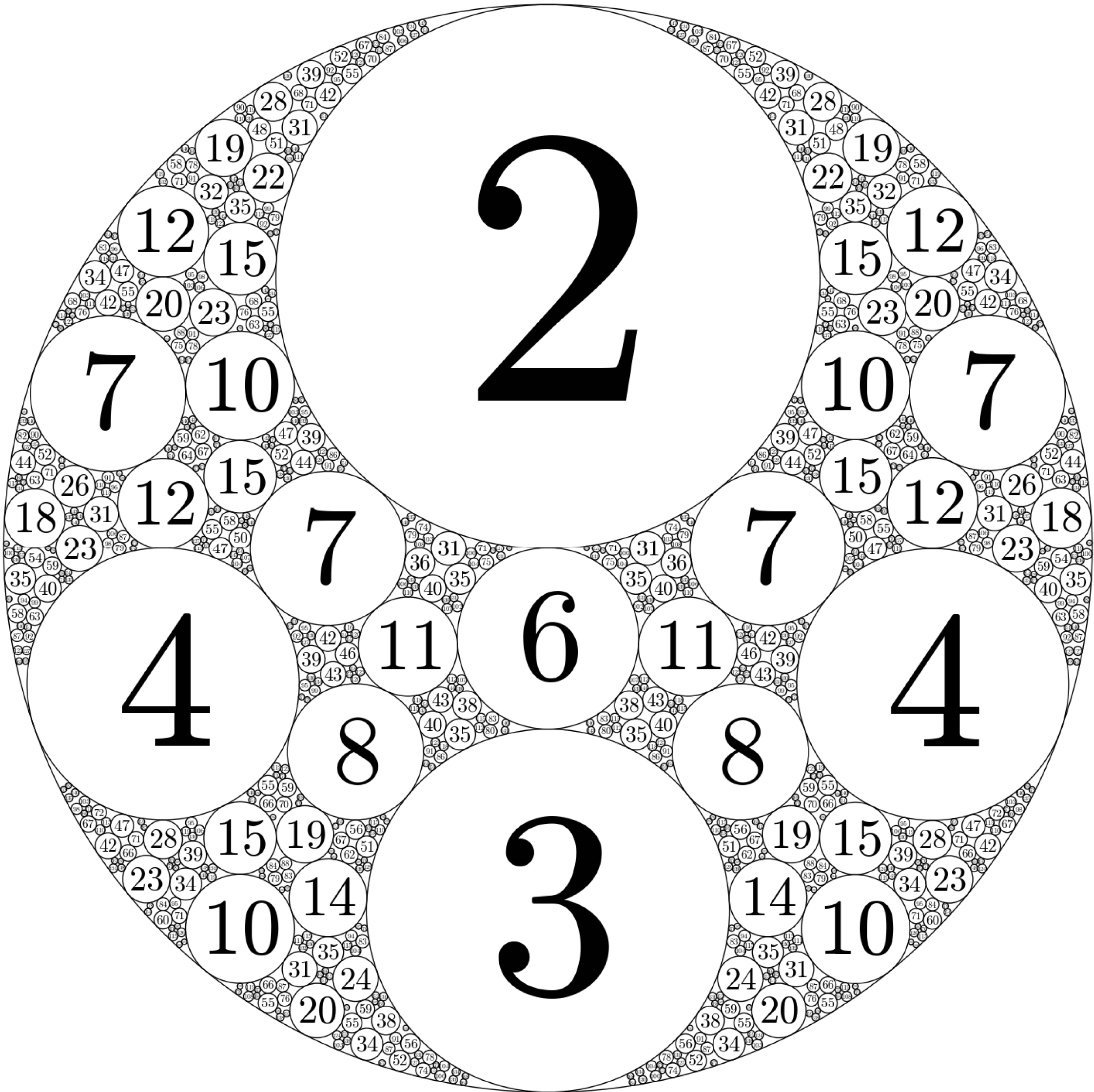1 Introduction
Local-to-global questions have been studied throughout the history of number theory. Here, we consider the set of curvatures appearing in circle packings which are orbits of thin Kleinian groups: when is the set of curvatures essentially characterized by congruence conditions alone? In this context, a thin Kleinian group is one commensurable to an infinite-index subgroup of a Bianchi group
![]() $\operatorname{PSL}_{2}({\mathcal{O}}_{K})$
, but simultaneously Zariski dense in
$\operatorname{PSL}_{2}({\mathcal{O}}_{K})$
, but simultaneously Zariski dense in
![]() $\operatorname{PGL}_{2}$
.
$\operatorname{PGL}_{2}$
.
This question was first considered in 2003 in a groundwork paper by Graham, Lagarias, Mallows, Wilks and Yan [Reference Graham, Lagarias, Mallows, Wilks and YanGLMWY03]. They observed that for several primitive integral Apollonian packings there appears to be a set of congruence classes modulo
![]() $24$
or
$24$
or
![]() $48$
such that any large enough integer having such a residue is indeed a curvature in that packing. They conjectured that this is the case for all packings. In 2011, the first-named author of the present paper made a detailed study of congruence conditions for Apollonian packings [Reference FuchsFuc11]. Together with Sanden, this author performed extensive numerical experiments and conjectured that in fact all primitive integral Apollonian packings can be described in terms of conditions modulo
$48$
such that any large enough integer having such a residue is indeed a curvature in that packing. They conjectured that this is the case for all packings. In 2011, the first-named author of the present paper made a detailed study of congruence conditions for Apollonian packings [Reference FuchsFuc11]. Together with Sanden, this author performed extensive numerical experiments and conjectured that in fact all primitive integral Apollonian packings can be described in terms of conditions modulo
![]() $24$
[Reference Fuchs and SandenFS11].
$24$
[Reference Fuchs and SandenFS11].
The first step towards trying to prove this conjecture is in [Reference Graham, Lagarias, Mallows, Wilks and YanGLMWY03], where it is shown that at least
![]() $cx^{1/2}$
integers less than
$cx^{1/2}$
integers less than
![]() $x$
appear as curvatures in a given integral Apollonian packing, where
$x$
appear as curvatures in a given integral Apollonian packing, where
![]() $c$
is a constant depending on the packing. Sarnak then made an observation in [Reference SarnakSar07] which became the basis for all future developments on this question. In that letter, Sarnak showed that in any primitive Apollonian packing there are, up to a constant, at least
$c$
is a constant depending on the packing. Sarnak then made an observation in [Reference SarnakSar07] which became the basis for all future developments on this question. In that letter, Sarnak showed that in any primitive Apollonian packing there are, up to a constant, at least
![]() $x/\sqrt{\log x}$
integers less than
$x/\sqrt{\log x}$
integers less than
![]() $x$
which appear as curvatures in the packing. His approach was to observe that if one fixes a circle in the packing and considers only those circles tangent to it, their curvatures, without multiplicity, are exactly the set of numbers that are primitively represented by a shifted binary quadratic form
$x$
which appear as curvatures in the packing. His approach was to observe that if one fixes a circle in the packing and considers only those circles tangent to it, their curvatures, without multiplicity, are exactly the set of numbers that are primitively represented by a shifted binary quadratic form
![]() $f(x,y)-a$
whose coefficients depend on the circle that is fixed. Sarnak’s idea was then expanded by Bourgain and Fuchs to prove that in fact a positive fraction of all integers appear in any primitive integral Apollonian packing [Reference Bourgain and FuchsBF11]. The methods of [Reference Bourgain and FuchsBF11] were then taken several steps further by Bourgain and Kontorovich in [Reference Bourgain and KontorovichBK14a] to prove an asymptotic local-to-global principal for Apollonian packings: they showed that, if
$f(x,y)-a$
whose coefficients depend on the circle that is fixed. Sarnak’s idea was then expanded by Bourgain and Fuchs to prove that in fact a positive fraction of all integers appear in any primitive integral Apollonian packing [Reference Bourgain and FuchsBF11]. The methods of [Reference Bourgain and FuchsBF11] were then taken several steps further by Bourgain and Kontorovich in [Reference Bourgain and KontorovichBK14a] to prove an asymptotic local-to-global principal for Apollonian packings: they showed that, if
![]() $A$
is the set of positive integers that are admissible as curvatures in a given primitive integral Apollonian packing according to their residue modulo
$A$
is the set of positive integers that are admissible as curvatures in a given primitive integral Apollonian packing according to their residue modulo
![]() $24$
, the subset of
$24$
, the subset of
![]() $A$
of integers which do not appear as curvatures in the packing make up a zero-density subset of all integers.
$A$
of integers which do not appear as curvatures in the packing make up a zero-density subset of all integers.
How far can one take the method in [Reference Bourgain and KontorovichBK14a] to prove asymptotic local-to-global principles in the thin setting? For example, the third-named author of this paper successfully used the tools of [Reference Bourgain and KontorovichBK14a] to prove an asymptotic local-to-global principle in so-called integral Apollonian 3-packings [Reference ZhangZha18b]. In this paper, we identify the key necessary conditions for these methods to work, which, when satisfied, guarantee an asymptotic local-to-global principle for an integral circle packing or, viewed differently, an orbit of a thin subgroup of
![]() $\text{PSL}_{2}(\mathbb{C})$
. As a consequence, we immediately have that an asymptotic local-to-global principle holds for the
$\text{PSL}_{2}(\mathbb{C})$
. As a consequence, we immediately have that an asymptotic local-to-global principle holds for the
![]() $K$
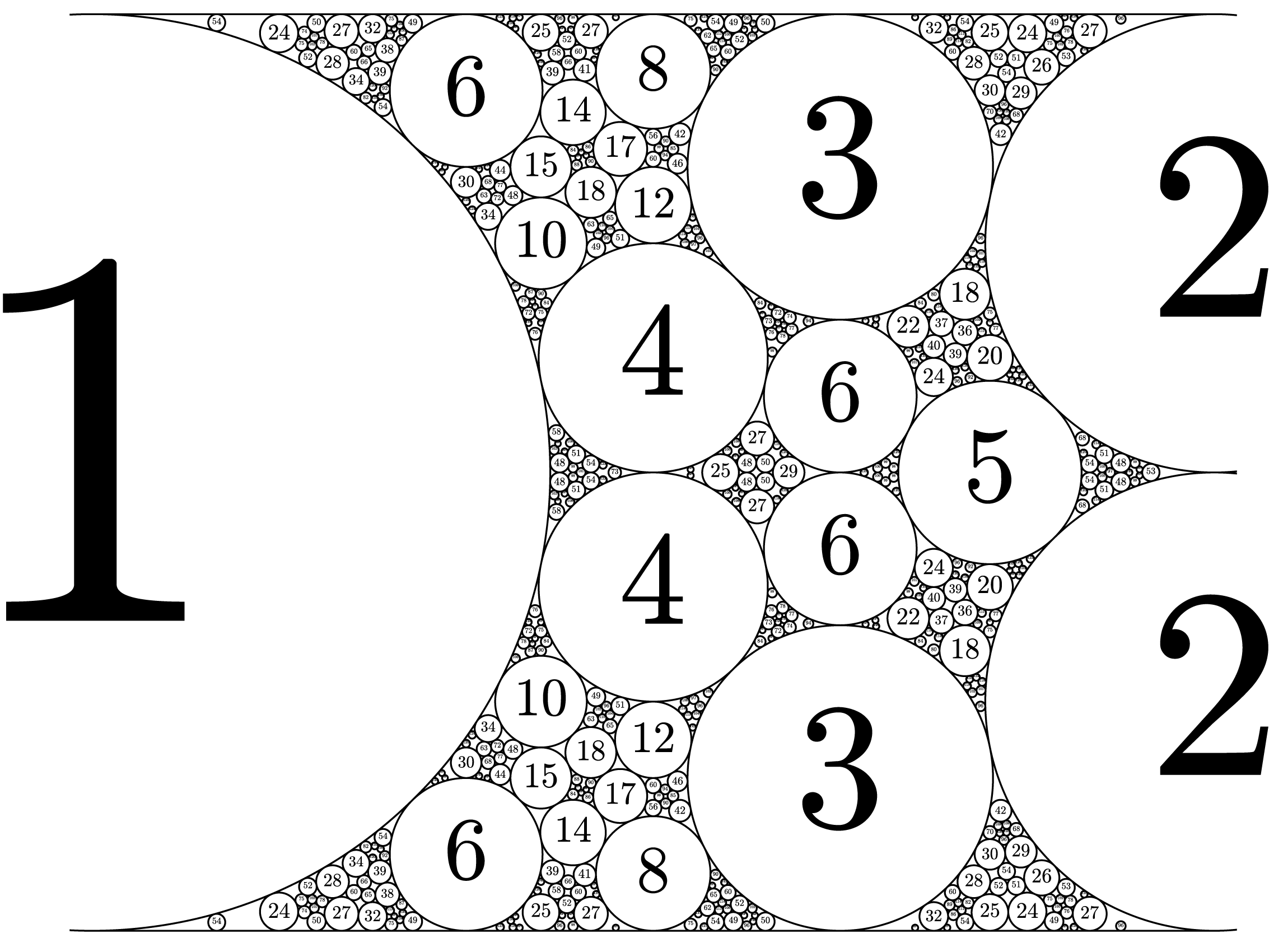
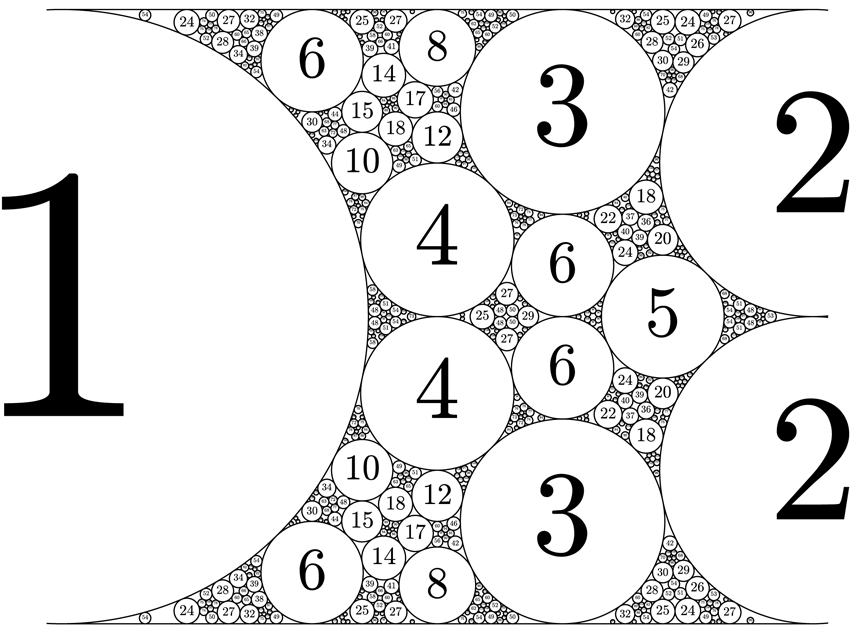
-Apollonian packings described by the second-named author [Reference StangeSta18a] and for superintegral polyhedral packings described by Kontorovich and Nakamura [Reference Kontorovich and NakamuraKN17]. We provide a concrete example of such a packing and give more details on the packings of Stange and of Kontorovich and Nakamura in § 9. See Figures 1 and 2.
$K$
-Apollonian packings described by the second-named author [Reference StangeSta18a] and for superintegral polyhedral packings described by Kontorovich and Nakamura [Reference Kontorovich and NakamuraKN17]. We provide a concrete example of such a packing and give more details on the packings of Stange and of Kontorovich and Nakamura in § 9. See Figures 1 and 2.
In the work on Apollonian packings by Bourgain, Fuchs, Kontorovich and Zhang, the curvatures in the packings were represented as coordinates of points in an orbit of a thin subgroup of
![]() $\text{O}_{Q}(\mathbb{Z})$
, where
$\text{O}_{Q}(\mathbb{Z})$
, where
![]() $Q$
is a signature
$Q$
is a signature
![]() $(3,1)$
quadratic form which is simply the Descartes form in the Apollonian case, and an analogue thereof in the 3-packing case. In both of these cases, one can view the curvatures as curvatures of circles obtained by considering the orbit via Möbius transformations of a fixed circle (or line) in the complex plane under the action of a thin (Kleinian) subgroup
$(3,1)$
quadratic form which is simply the Descartes form in the Apollonian case, and an analogue thereof in the 3-packing case. In both of these cases, one can view the curvatures as curvatures of circles obtained by considering the orbit via Möbius transformations of a fixed circle (or line) in the complex plane under the action of a thin (Kleinian) subgroup
![]() ${\mathcal{A}}$
of
${\mathcal{A}}$
of
![]() $\text{PSL}_{2}({\mathcal{O}}_{K})$
where
$\text{PSL}_{2}({\mathcal{O}}_{K})$
where
![]() $K$
is an imaginary quadratic field. In the original Apollonian case
$K$
is an imaginary quadratic field. In the original Apollonian case
![]() $K=\mathbb{Q}(i)$
, and in the 3-packing case
$K=\mathbb{Q}(i)$
, and in the 3-packing case
![]() $K=\mathbb{Q}(\sqrt{-2})$
. One can pass between these two interpretations of the set of curvatures via the spin homomorphism
$K=\mathbb{Q}(\sqrt{-2})$
. One can pass between these two interpretations of the set of curvatures via the spin homomorphism
![]() $\unicode[STIX]{x1D70C}:\text{PSL}_{2}(\mathbb{C})\rightarrow \text{O}_{\mathbb{R}}(3,1)$
, but the
$\unicode[STIX]{x1D70C}:\text{PSL}_{2}(\mathbb{C})\rightarrow \text{O}_{\mathbb{R}}(3,1)$
, but the
![]() $\text{PSL}_{2}$
setup is more convenient for several reasons: for example, there are numerous choices for the analogue of the Descartes form if one chooses to work in
$\text{PSL}_{2}$
setup is more convenient for several reasons: for example, there are numerous choices for the analogue of the Descartes form if one chooses to work in
![]() $\text{O}_{\mathbb{R}}(3,1)$
; also,
$\text{O}_{\mathbb{R}}(3,1)$
; also,
![]() $\text{SL}_{2}$
is simply connected, while the orthogonal group is not.
$\text{SL}_{2}$
is simply connected, while the orthogonal group is not.
Definition 1.1. Let
![]() ${\mathcal{A}}$
be a Kleinian group, and let
${\mathcal{A}}$
be a Kleinian group, and let
![]() $C_{1},\ldots ,C_{n}$
be circles in the extended complex plane. Write
$C_{1},\ldots ,C_{n}$
be circles in the extended complex plane. Write
![]() ${\mathcal{A}}C_{i}$
for the orbit of
${\mathcal{A}}C_{i}$
for the orbit of
![]() $C_{i}$
under
$C_{i}$
under
![]() ${\mathcal{A}}$
, as a subset of the plane (a union of circles). Then
${\mathcal{A}}$
, as a subset of the plane (a union of circles). Then
is called a Kleinian circle packing. Such a packing is called integral if, after a universal scaling factor is applied, the set of curvatures can be taken to be a subset of
![]() $\mathbb{Z}$
.
$\mathbb{Z}$
.
We define the curvature of a circle
![]() $N(\widehat{\mathbb{R}})$
, where
$N(\widehat{\mathbb{R}})$
, where
![]() $N=\big(\!\begin{smallmatrix}A & B\\ C & D\end{smallmatrix}\!\big)$
, to be
$N=\big(\!\begin{smallmatrix}A & B\\ C & D\end{smallmatrix}\!\big)$
, to be
![]() $2\Im (\overline{C}D)$
; then the radius is
$2\Im (\overline{C}D)$
; then the radius is
![]() $1/|2\Im (\overline{C}D)|$
, but the curvature contains some further information in the form of the sign, which can be interpreted as orientation. In general, the circles in a Kleinian circle packing may overlap, although they do not in the most famous cases, such as the Apollonian circle packing.
$1/|2\Im (\overline{C}D)|$
, but the curvature contains some further information in the form of the sign, which can be interpreted as orientation. In general, the circles in a Kleinian circle packing may overlap, although they do not in the most famous cases, such as the Apollonian circle packing.
Although one might conjecture a local-global principle for a larger class of integral Kleinian circle packings, our methods require that the packing contain ‘congruence families’ of circles, which give rise to integral binary quadratic forms as in the Apollonian case. Therefore we define a restricted class of groups.
Definition 1.2. A Kleinian group
![]() ${\mathcal{A}}$
is called familial if:
${\mathcal{A}}$
is called familial if:
(i)
 $\operatorname{PSL}_{2}(\mathbb{Z})\cap {\mathcal{A}}$
contains a principal congruence subgroup; and
$\operatorname{PSL}_{2}(\mathbb{Z})\cap {\mathcal{A}}$
contains a principal congruence subgroup; and(ii) the entries of
 ${\mathcal{A}}$
are contained in some fractional ideal
${\mathcal{A}}$
are contained in some fractional ideal
 $\mathfrak{a}$
of an imaginary quadratic field
$\mathfrak{a}$
of an imaginary quadratic field
 $K=\mathbb{Q}(\sqrt{-d})$
,
$K=\mathbb{Q}(\sqrt{-d})$
,
 $d>0$
.
$d>0$
.
Furthermore, the methods require that the group
![]() ${\mathcal{A}}$
has a spectral gap property: i.e. that the family of graphs
${\mathcal{A}}$
has a spectral gap property: i.e. that the family of graphs
![]() $\{\text{Cay}({\mathcal{A}}/{\mathcal{A}}(q),\overline{S})\}_{q}$
is an expander family. Here
$\{\text{Cay}({\mathcal{A}}/{\mathcal{A}}(q),\overline{S})\}_{q}$
is an expander family. Here
![]() ${\mathcal{A}}/{\mathcal{A}}(q)$
denotes
${\mathcal{A}}/{\mathcal{A}}(q)$
denotes
![]() ${\mathcal{A}}$
reduced modulo
${\mathcal{A}}$
reduced modulo
![]() $q$
, the set
$q$
, the set
![]() $S$
is a finite generating set of
$S$
is a finite generating set of
![]() ${\mathcal{A}}$
,
${\mathcal{A}}$
,
![]() $\overline{S}$
denotes its image under reduction, and
$\overline{S}$
denotes its image under reduction, and
![]() $q$
ranges over all positive integers. In § 8, we show that this is the case for a class of groups including those we intend to consider, i.e. we show the following theorem.
$q$
ranges over all positive integers. In § 8, we show that this is the case for a class of groups including those we intend to consider, i.e. we show the following theorem.
Theorem 1.3. Any infinite-covolume, geometrically finite, Zariski dense Kleinian group contained in
![]() $\text{PSL}_{2}({\mathcal{O}}_{K})$
, containing a Zariski dense subgroup of
$\text{PSL}_{2}({\mathcal{O}}_{K})$
, containing a Zariski dense subgroup of
![]() $\text{PSL}_{2}(\mathbb{Z})$
has a combinatorial spectral gap.
$\text{PSL}_{2}(\mathbb{Z})$
has a combinatorial spectral gap.
In our setting, the group
![]() ${\mathcal{A}}$
has critical exponent greater than 1. It then follows from the arguments in [Reference KimKim15, Theorem 11.2, Step II], which is an immediate generalization of [Reference Bourgain, Gamburd and SarnakBGS11, Theorem 1.2], that
${\mathcal{A}}$
has critical exponent greater than 1. It then follows from the arguments in [Reference KimKim15, Theorem 11.2, Step II], which is an immediate generalization of [Reference Bourgain, Gamburd and SarnakBGS11, Theorem 1.2], that
![]() ${\mathcal{A}}$
also enjoys a geometric spectral gap (see [Reference Bourgain, Gamburd and SarnakBGS11, p. 39] for a definition).
${\mathcal{A}}$
also enjoys a geometric spectral gap (see [Reference Bourgain, Gamburd and SarnakBGS11, p. 39] for a definition).
Previously, Sarnak established the spectral gap property for finite-index subgroups of
![]() $\text{PSL}_{2}({\mathcal{O}}_{K})$
[Reference SarnakSar83], and Magee established this property for geometrically finite subgroups of
$\text{PSL}_{2}({\mathcal{O}}_{K})$
[Reference SarnakSar83], and Magee established this property for geometrically finite subgroups of
![]() $\text{PSL}_{2}({\mathcal{O}}_{K})$
with critical exponents greater than
$\text{PSL}_{2}({\mathcal{O}}_{K})$
with critical exponents greater than
![]() $\frac{11}{6}$
[Reference MageeMag15]. Salehi-Golsefidy and Zhang, the third named author, generalize Theorem 1.3 even further in an upcoming preprint [Reference Salehi Golsefidy and ZhangSal18]. The existence of a geometric spectral gap is a crucial ingredient both in [Reference Bourgain and KontorovichBK14a] and in [Reference ZhangZha18b], and indeed in almost all works that have investigated arithmetic aspects of thin groups. We indicate exactly how this spectral gap is relevant in § 5. Note that, as explained below, every familial group
$\frac{11}{6}$
[Reference MageeMag15]. Salehi-Golsefidy and Zhang, the third named author, generalize Theorem 1.3 even further in an upcoming preprint [Reference Salehi Golsefidy and ZhangSal18]. The existence of a geometric spectral gap is a crucial ingredient both in [Reference Bourgain and KontorovichBK14a] and in [Reference ZhangZha18b], and indeed in almost all works that have investigated arithmetic aspects of thin groups. We indicate exactly how this spectral gap is relevant in § 5. Note that, as explained below, every familial group
![]() ${\mathcal{A}}$
does satisfy the Hausdorff dimension hypothesis in Theorem 1.3.
${\mathcal{A}}$
does satisfy the Hausdorff dimension hypothesis in Theorem 1.3.
We now state the general setup of the paper. Let
![]() $K$
be an imaginary quadratic field. Henceforth, we will assume that
$K$
be an imaginary quadratic field. Henceforth, we will assume that
![]() ${\mathcal{A}}$
denotes an infinite-covolume, geometrically finite, Zariski-dense, familial Kleinian group in
${\mathcal{A}}$
denotes an infinite-covolume, geometrically finite, Zariski-dense, familial Kleinian group in
![]() $\operatorname{PSL}_{2}(K)$
. We will consider an associated packing
$\operatorname{PSL}_{2}(K)$
. We will consider an associated packing
![]() ${\mathcal{P}}:=M{\mathcal{A}}C$
, where
${\mathcal{P}}:=M{\mathcal{A}}C$
, where
![]() $C$
is any circle tangent to the real line and having the form
$C$
is any circle tangent to the real line and having the form
![]() $C=N(\widehat{\mathbb{R}})$
, where
$C=N(\widehat{\mathbb{R}})$
, where
![]() $N,M\in \operatorname{PSL}_{2}(K)$
.
$N,M\in \operatorname{PSL}_{2}(K)$
.
This last condition, on the tangency of
![]() $C$
to the real line, is crucial to the methods of the paper, as it guarantees, together with the congruence subgroup condition of Definition 1.2, that a collection of integral binary quadratic forms govern the curvatures of the packing.
$C$
to the real line, is crucial to the methods of the paper, as it guarantees, together with the congruence subgroup condition of Definition 1.2, that a collection of integral binary quadratic forms govern the curvatures of the packing.
Under these conditions, the packing
![]() ${\mathcal{P}}$
is necessarily integral as in Definition 1.2 (see § 3). We let
${\mathcal{P}}$
is necessarily integral as in Definition 1.2 (see § 3). We let
![]() ${\mathcal{K}}\subset \mathbb{Z}$
be the set of curvatures, after some universal scaling factor is applied as in the definition of integrality.
${\mathcal{K}}\subset \mathbb{Z}$
be the set of curvatures, after some universal scaling factor is applied as in the definition of integrality.
Let
![]() ${\mathcal{K}}_{a}$
be the set of integers passing all the local obstructions by
${\mathcal{K}}_{a}$
be the set of integers passing all the local obstructions by
![]() ${\mathcal{K}}$
. In other words,
${\mathcal{K}}$
. In other words,
We call the integers in
![]() ${\mathcal{K}}_{a}$
admissible.
${\mathcal{K}}_{a}$
admissible.
We now state an immediate corollary of the spectral gap statement in Theorem 1.3.
Corollary 1.4. There exists a positive integer
![]() $L_{0}$
such that
$L_{0}$
such that
![]() ${\mathcal{K}}_{a}$
is the union of some congruence classes
${\mathcal{K}}_{a}$
is the union of some congruence classes
![]() $\text{mod}\,L_{0}$
.
$\text{mod}\,L_{0}$
.
Of course, this also follows by strong approximation (see [Reference Rapinchuk, Breuillard and OhRap14]) for
![]() $\text{SL}_{2}$
. However, the proof of our Theorem 1.3 gives not only the existence of
$\text{SL}_{2}$
. However, the proof of our Theorem 1.3 gives not only the existence of
![]() $L_{0}$
but also an algorithm to quickly determine its exact value: in particular, the prime factors of
$L_{0}$
but also an algorithm to quickly determine its exact value: in particular, the prime factors of
![]() $L_{0}$
will come from the level of the congruence subgroup contained in
$L_{0}$
will come from the level of the congruence subgroup contained in
![]() ${\mathcal{A}}$
, any failure of primitivity of the packing
${\mathcal{A}}$
, any failure of primitivity of the packing
![]() ${\mathcal{P}}$
, and the primes
${\mathcal{P}}$
, and the primes
![]() $2$
and
$2$
and
![]() $3$
, as well as the matrix
$3$
, as well as the matrix
![]() $M$
if
$M$
if
![]() $M$
is fractional. See Theorem 8.1 and (6.21) for details.
$M$
is fractional. See Theorem 8.1 and (6.21) for details.
Now let
![]() ${\mathcal{K}}_{a}(N)={\mathcal{K}}_{a}\cap [0,N]$
be the set of admissible integers up to
${\mathcal{K}}_{a}(N)={\mathcal{K}}_{a}\cap [0,N]$
be the set of admissible integers up to
![]() $N$
, and similarly denote
$N$
, and similarly denote
![]() ${\mathcal{K}}(N)={\mathcal{K}}\cap [0,N]$
. Then Corollary 1.4 directly implies that
${\mathcal{K}}(N)={\mathcal{K}}\cap [0,N]$
. Then Corollary 1.4 directly implies that
where
![]() $c_{M,{\mathcal{A}},C}$
is the proportion of admissible congruence classes. We predict that all sufficiently large admissible integers are actually curvatures; in other words, we make the following conjecture.
$c_{M,{\mathcal{A}},C}$
is the proportion of admissible congruence classes. We predict that all sufficiently large admissible integers are actually curvatures; in other words, we make the following conjecture.
Conjecture 1.5.
In place of the full conjecture, we prove the following theorem.
Theorem 1.6. Let
![]() ${\mathcal{A}}$
and
${\mathcal{A}}$
and
![]() ${\mathcal{P}}=M{\mathcal{A}}C$
be as above. There exists a positive number
${\mathcal{P}}=M{\mathcal{A}}C$
be as above. There exists a positive number
![]() $\unicode[STIX]{x1D702}$
, depending only on
$\unicode[STIX]{x1D702}$
, depending only on
![]() $M$
,
$M$
,
![]() ${\mathcal{A}}$
and
${\mathcal{A}}$
and
![]() $C$
, such that
$C$
, such that
We feel that it is unlikely that our method can prove Conjecture 1.5 without significant new ideas.
We mention a remark by Chris Leininger: in fact our geometric finiteness assumption can be relaxed to be finitely generated. It is a corollary of the tameness theorem [Reference AgolAgo04] that any finitely generated Zariski dense subgroup of the Bianchi group containing a congruence subgroup of
![]() $\text{PSL}_{2}(\mathbb{Z})$
must be geometrically finite.
$\text{PSL}_{2}(\mathbb{Z})$
must be geometrically finite.
Kontorovich and Nakamura define a family of dense circle packings of the plane defined by hyperbolic reflection groups built from uniform polyhedra and their growths [Reference Kontorovich and NakamuraKN17]. For infinitely many of their examples, Kontorovich and Nakamura verify in their paper that such packings satisfy the hypotheses of Theorem 1.6, and hence have a local-to-global principle.
Of course, it is possible to construct examples of integral Kleinian packings which fail to satisfy the hypotheses of Theorem 1.6. For example, one may take a non-congruence subgroup of
![]() $\operatorname{PSL}_{2}(\mathbb{Z})$
, and adjoin another element to obtain a non-Fuchsian group; then consider the orbit of a
$\operatorname{PSL}_{2}(\mathbb{Z})$
, and adjoin another element to obtain a non-Fuchsian group; then consider the orbit of a
![]() $K$
-rational circle tangent to
$K$
-rational circle tangent to
![]() $\widehat{\mathbb{R}}$
. In such a case, one cannot guarantee the existence of a suitable family of binary quadratic forms: one only obtains quadratic forms in four related variables. It is therefore an interesting open question to develop methods which will prove an analogue to Theorem 1.6 for such packings.
$\widehat{\mathbb{R}}$
. In such a case, one cannot guarantee the existence of a suitable family of binary quadratic forms: one only obtains quadratic forms in four related variables. It is therefore an interesting open question to develop methods which will prove an analogue to Theorem 1.6 for such packings.
In § 9, in order the demonstrate the variety of examples to which our work applies, we verify that the hypotheses of Theorem 1.6 hold for the
![]() $K$
-Apollonian packings of the second-named author [Reference StangeSta18a], and also for an explicit example of a cuboctahedral packing (which also arises in the work of Kontorovich and Nakamura; Figure 1).
$K$
-Apollonian packings of the second-named author [Reference StangeSta18a], and also for an explicit example of a cuboctahedral packing (which also arises in the work of Kontorovich and Nakamura; Figure 1).
The main method in the proof of this theorem is the Hardy–Littlewood circle method. In the major arc analysis of the circle method, the main ingredient is an effective counting of group elements for
![]() ${\mathcal{A}}$
and its congruence subgroups originally achieved by Vinogradov [Reference VinogradovVin14]. In doing this, we require a geometric spectral gap for
${\mathcal{A}}$
and its congruence subgroups originally achieved by Vinogradov [Reference VinogradovVin14]. In doing this, we require a geometric spectral gap for
![]() ${\mathcal{A}}$
in order to have a uniform control over the error terms. In § 8 we establish a combinatorial spectral gap for
${\mathcal{A}}$
in order to have a uniform control over the error terms. In § 8 we establish a combinatorial spectral gap for
![]() ${\mathcal{A}}$
, which in turn implies a geometric spectral gap for
${\mathcal{A}}$
, which in turn implies a geometric spectral gap for
![]() ${\mathcal{A}}$
by the methods in [Reference Bourgain, Gamburd and SarnakBGS11], proving Theorem 1.3. Moreover, we require that
${\mathcal{A}}$
by the methods in [Reference Bourgain, Gamburd and SarnakBGS11], proving Theorem 1.3. Moreover, we require that
![]() $\unicode[STIX]{x1D6FF}=\unicode[STIX]{x1D6FF}({\mathcal{A}})$
, the critical exponent of
$\unicode[STIX]{x1D6FF}=\unicode[STIX]{x1D6FF}({\mathcal{A}})$
, the critical exponent of
![]() ${\mathcal{A}}$
, which is also the Hausdorff dimension of the limit set of
${\mathcal{A}}$
, which is also the Hausdorff dimension of the limit set of
![]() ${\mathcal{A}}$
, is strictly greater than 1, which is guaranteed by our assumption that
${\mathcal{A}}$
, is strictly greater than 1, which is guaranteed by our assumption that
![]() ${\mathcal{A}}$
is familial, and a limit set classification theorem of Bishop and Jones [Reference Bishop and JonesBJ97, Corollary 1.8].
${\mathcal{A}}$
is familial, and a limit set classification theorem of Bishop and Jones [Reference Bishop and JonesBJ97, Corollary 1.8].
Besides the existence of the spectral gap, which is crucial for minor arcs as well as major arcs, the main ingredient in the minor arc analysis is the quadratic form structure, which allows us to do abelian harmonic analysis of two free variables. Certain Kloosterman-type sums naturally appear here, where we apply standard methods to gain power savings. In fact the power saving here, as well as in [Reference Bourgain and KontorovichBK14a, Reference ZhangZha18b], is so significant that one does not need further restriction on the critical exponent
![]() $\unicode[STIX]{x1D6FF}$
(besides
$\unicode[STIX]{x1D6FF}$
(besides
![]() $\unicode[STIX]{x1D6FF}>1$
), in contrast to the works [Reference Bourgain and KontorovichBK10, Reference Bourgain and KontorovichBK14b, Reference ZhangZha18a], which require the critical exponent to be very big in order to get enough cancelation in the minor arc analysis.
$\unicode[STIX]{x1D6FF}>1$
), in contrast to the works [Reference Bourgain and KontorovichBK10, Reference Bourgain and KontorovichBK14b, Reference ZhangZha18a], which require the critical exponent to be very big in order to get enough cancelation in the minor arc analysis.
Note that our methods, while similar to that in [Reference Bourgain and KontorovichBK14a, Reference ZhangZha18b], require several new ingredients and careful generalizations to work. One crucial such ingredient is the spectral gap of Theorem 1.3. This theorem applies to a much wider class of groups than our local-to-global analysis, and generalizes the case of the Apollonian group, proven by Varjú in the appendix of [Reference Bourgain and KontorovichBK14a]. In proving this theorem, we do not, for instance, have any concrete information about the generators of the group we work with, or exactly at which primes and to what level there are local obstructions for the group. Indeed, in the proof of Theorem 1.3, we are able to derive, in the case of the groups considered within this paper, exactly what the local obstructions should be: something that was done explicitly for the Apollonian group in [Reference FuchsFuc11].
Secondly, the fact that we work with an arbitrary imaginary quadratic field
![]() $K$
(as opposed to
$K$
(as opposed to
![]() $\mathbb{Q}(i)$
and
$\mathbb{Q}(i)$
and
![]() $\mathbb{Q}(\sqrt{-2})$
as in [Reference Bourgain and KontorovichBK14a] and [Reference ZhangZha18b], respectively), and an abstract subgroup of
$\mathbb{Q}(\sqrt{-2})$
as in [Reference Bourgain and KontorovichBK14a] and [Reference ZhangZha18b], respectively), and an abstract subgroup of
![]() $\text{PSL}_{2}({\mathcal{O}}_{K})$
makes the local analysis in the major arcs section (§ 6) much less straightforward: where the authors of [Reference Bourgain and KontorovichBK14a, Reference ZhangZha18b] could depend on concrete local information about the groups they work with, we derive this without relying on explicit information about the local obstructions.
$\text{PSL}_{2}({\mathcal{O}}_{K})$
makes the local analysis in the major arcs section (§ 6) much less straightforward: where the authors of [Reference Bourgain and KontorovichBK14a, Reference ZhangZha18b] could depend on concrete local information about the groups they work with, we derive this without relying on explicit information about the local obstructions.
Thirdly, in both [Reference Bourgain and KontorovichBK14a, Reference ZhangZha18b], the level of the congruence subgroup contained in the Apollonian group in question is
![]() $2$
, which means that the curvatures of the circles are exactly the set of integers represented by a corresponding class of shifted binary quadratic forms. In our paper this is no longer the case and it is possible that the curvatures we consider (after appropriate scaling to make them integral) comprise a subset of values of the corresponding class of shifted forms. In fact, while the methods here deal with this nicely, this would make executing the positive density proof in [Reference Bourgain and FuchsBF11] significantly more cumbersome in our setting than in the original setup of the classical Apollonian group.
$2$
, which means that the curvatures of the circles are exactly the set of integers represented by a corresponding class of shifted binary quadratic forms. In our paper this is no longer the case and it is possible that the curvatures we consider (after appropriate scaling to make them integral) comprise a subset of values of the corresponding class of shifted forms. In fact, while the methods here deal with this nicely, this would make executing the positive density proof in [Reference Bourgain and FuchsBF11] significantly more cumbersome in our setting than in the original setup of the classical Apollonian group.
We have made a special effort to make our exposition of these methods particularly accessible, in the hope that it may benefit students and experts alike.
Notation
Sections 2–7 are notation-heavy. For ease of reading, we include a table of the major notation used in those sections in § 10. We also note that whenever the constant
![]() $\unicode[STIX]{x1D702}$
appears, it is assumed to satisfy not only the current claim, but also all claims in previous contexts.
$\unicode[STIX]{x1D702}$
appears, it is assumed to satisfy not only the current claim, but also all claims in previous contexts.
Figures
The figures were produced with Sage Mathematics Software [Sage16].
2 Integrality of
 ${\mathcal{A}}$
${\mathcal{A}}$
For the purpose of our methods, we intend to replace
![]() ${\mathcal{A}}$
with
${\mathcal{A}}$
with
![]() $\tilde{{\mathcal{A}}}:={\mathcal{A}}\cap \text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
, where
$\tilde{{\mathcal{A}}}:={\mathcal{A}}\cap \text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
, where
![]() $d$
is as in Definition 1.2, since we would like to work with an integral group. The next lemma asserts that
$d$
is as in Definition 1.2, since we would like to work with an integral group. The next lemma asserts that
![]() $\tilde{{\mathcal{A}}}$
is finite index in
$\tilde{{\mathcal{A}}}$
is finite index in
![]() ${\mathcal{A}}$
.
${\mathcal{A}}$
.
Without loss of generality, we can replace
![]() ${\mathcal{A}}$
with any finite-index subgroup for the purposes of Theorem 1.6. This is because a finite number of orbits of the subgroup comprise the full orbit of
${\mathcal{A}}$
with any finite-index subgroup for the purposes of Theorem 1.6. This is because a finite number of orbits of the subgroup comprise the full orbit of
![]() ${\mathcal{A}}$
, and the congruence obstructions from these orbits can be combined to give the obstruction for the union.
${\mathcal{A}}$
, and the congruence obstructions from these orbits can be combined to give the obstruction for the union.
For this reason, we are free to assume throughout the paper that
![]() ${\mathcal{A}}$
is torsion-free, by Selberg’s theorem, saying that any matrix group contains a finite-index torsion-free subgroup [Reference SelbergSel60], and, by the following lemma, that it is a subgroup of
${\mathcal{A}}$
is torsion-free, by Selberg’s theorem, saying that any matrix group contains a finite-index torsion-free subgroup [Reference SelbergSel60], and, by the following lemma, that it is a subgroup of
![]() $\text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
.
$\text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
.
Lemma 2.1. Let
![]() ${\mathcal{A}}$
be as defined in the introduction, and let
${\mathcal{A}}$
be as defined in the introduction, and let
![]() $\tilde{{\mathcal{A}}}={\mathcal{A}}\cap \text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
. Then
$\tilde{{\mathcal{A}}}={\mathcal{A}}\cap \text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
. Then
![]() $[{\mathcal{A}}:\tilde{{\mathcal{A}}}]$
is finite.
$[{\mathcal{A}}:\tilde{{\mathcal{A}}}]$
is finite.
Proof. Recall that
![]() $K=\mathbb{Q}(\sqrt{-d})$
. If
$K=\mathbb{Q}(\sqrt{-d})$
. If
![]() ${\mathcal{A}}\subset \text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
, then the statement is trivial. Hence, suppose
${\mathcal{A}}\subset \text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
, then the statement is trivial. Hence, suppose
![]() ${\mathcal{A}}\not \subset \text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
, such that the denominators featured in its elements are bounded above, as assumed in the previous section. Let
${\mathcal{A}}\not \subset \text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
, such that the denominators featured in its elements are bounded above, as assumed in the previous section. Let
![]() $q=p_{1}^{e_{1}}\cdots p_{k}^{e_{k}}$
, where
$q=p_{1}^{e_{1}}\cdots p_{k}^{e_{k}}$
, where
![]() $p_{1},\ldots ,p_{k}$
are distinct primes, be the least common multiple of all denominators featured among entries of elements of
$p_{1},\ldots ,p_{k}$
are distinct primes, be the least common multiple of all denominators featured among entries of elements of
![]() ${\mathcal{A}}$
.
${\mathcal{A}}$
.
Let
![]() $H_{1}=\text{PSL}_{2}((1/q)\mathbb{Z}[\sqrt{-d}])$
, let
$H_{1}=\text{PSL}_{2}((1/q)\mathbb{Z}[\sqrt{-d}])$
, let
![]() $H_{2}={\mathcal{A}}$
, and let
$H_{2}={\mathcal{A}}$
, and let
![]() $H_{3}=\text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
. Note that
$H_{3}=\text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
. Note that
![]() $H_{1}$
is not a group, but contains both
$H_{1}$
is not a group, but contains both
![]() $H_{2}$
and
$H_{2}$
and
![]() $H_{3}$
. Furthermore, it is covered by some union of cosets of
$H_{3}$
. Furthermore, it is covered by some union of cosets of
![]() $H_{3}$
in
$H_{3}$
in
![]() $\text{PSL}_{2}(K)$
. If
$\text{PSL}_{2}(K)$
. If
![]() $H_{1}$
is covered by a finite union of cosets of
$H_{1}$
is covered by a finite union of cosets of
![]() $H_{3}$
, then
$H_{3}$
, then
![]() $H_{2}=H_{2}\cap H_{1}$
is covered by a finite union of cosets of
$H_{2}=H_{2}\cap H_{1}$
is covered by a finite union of cosets of
![]() $H_{2}\cap H_{3}$
, i.e.
$H_{2}\cap H_{3}$
, i.e.
![]() $[{\mathcal{A}}:\tilde{{\mathcal{A}}}]$
is finite.
$[{\mathcal{A}}:\tilde{{\mathcal{A}}}]$
is finite.
Therefore, we will cover
![]() $H_{1}$
by a finite union of cosets of
$H_{1}$
by a finite union of cosets of
![]() $H_{3}$
. To show this, note that if the
$H_{3}$
. To show this, note that if the
![]() $p_{i}$
-adic expansions of
$p_{i}$
-adic expansions of
![]() $\unicode[STIX]{x1D6FE}_{1},\unicode[STIX]{x1D6FE}_{2}\in \text{PSL}_{2}((1/q)\mathbb{Z}[\sqrt{-d}])$
agree in the
$\unicode[STIX]{x1D6FE}_{1},\unicode[STIX]{x1D6FE}_{2}\in \text{PSL}_{2}((1/q)\mathbb{Z}[\sqrt{-d}])$
agree in the
![]() $p_{i}^{-e_{i}},p_{i}^{-e_{i}+1},\ldots ,p_{i}^{e_{i}}$
terms for all
$p_{i}^{-e_{i}},p_{i}^{-e_{i}+1},\ldots ,p_{i}^{e_{i}}$
terms for all
![]() $1\leqslant i\leqslant k$
, then the ‘coefficients’ of the entries of
$1\leqslant i\leqslant k$
, then the ‘coefficients’ of the entries of
![]() $\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FE}_{2}^{-1}$
are
$\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FE}_{2}^{-1}$
are
![]() $p_{i}$
-adic integers for all
$p_{i}$
-adic integers for all
![]() $i$
. Here, what we mean by
$i$
. Here, what we mean by
![]() $p_{i}$
-adic expansions of
$p_{i}$
-adic expansions of
![]() $\unicode[STIX]{x1D6FE}$
is what one gets when one considers for each entry of
$\unicode[STIX]{x1D6FE}$
is what one gets when one considers for each entry of
![]() $\unicode[STIX]{x1D6FE}$
of the form
$\unicode[STIX]{x1D6FE}$
of the form
![]() $a+b\sqrt{-d}$
the
$a+b\sqrt{-d}$
the
![]() $p_{i}$
-adic expansion of
$p_{i}$
-adic expansion of
![]() $a$
and
$a$
and
![]() $b$
. By ‘coefficients’ of an entry
$b$
. By ‘coefficients’ of an entry
![]() $a+b\sqrt{-d}$
of
$a+b\sqrt{-d}$
of
![]() $\unicode[STIX]{x1D6FE}$
we mean precisely
$\unicode[STIX]{x1D6FE}$
we mean precisely
![]() $a$
and
$a$
and
![]() $b$
. Since
$b$
. Since
![]() $\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FE}_{2}^{-1}\in \text{PSL}_{2}((1/q^{2})\mathbb{Z}[\sqrt{-d}])$
, this in fact implies that
$\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FE}_{2}^{-1}\in \text{PSL}_{2}((1/q^{2})\mathbb{Z}[\sqrt{-d}])$
, this in fact implies that
![]() $\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FE}_{2}^{-1}\in \text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
. Since there are only finitely many possibilities for the
$\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FE}_{2}^{-1}\in \text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
. Since there are only finitely many possibilities for the
![]() $p_{i}^{-e_{i}},p_{i}^{-e_{i}+1},\ldots ,p_{i}^{e_{i}}$
terms in the
$p_{i}^{-e_{i}},p_{i}^{-e_{i}+1},\ldots ,p_{i}^{e_{i}}$
terms in the
![]() $p_{i}$
-adic expansion of any number, where
$p_{i}$
-adic expansion of any number, where
![]() $i$
ranges over finitely many indices, we have that there are in fact finitely many cosets of
$i$
ranges over finitely many indices, we have that there are in fact finitely many cosets of
![]() $\text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
in
$\text{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
in
![]() $\text{PSL}_{2}((1/q)\mathbb{Z}[\sqrt{-d}])$
, as desired.◻
$\text{PSL}_{2}((1/q)\mathbb{Z}[\sqrt{-d}])$
, as desired.◻
We remark that a converse also holds: if
![]() ${\mathcal{A}}$
has its intersection with the Bianchi group as a subgroup of finite index, then
${\mathcal{A}}$
has its intersection with the Bianchi group as a subgroup of finite index, then
![]() ${\mathcal{A}}$
has bounded denominators.
${\mathcal{A}}$
has bounded denominators.
Therefore, from this point on we assume
![]() ${\mathcal{A}}$
is a torsion-free subgroup of
${\mathcal{A}}$
is a torsion-free subgroup of
![]() $\operatorname{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
.
$\operatorname{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
.
3 Families of quadratic forms
We now describe the set of curvatures
![]() ${\mathcal{K}}$
as a union of values of a family of quadratic forms. Write
${\mathcal{K}}$
as a union of values of a family of quadratic forms. Write
![]() $\unicode[STIX]{x1D6E5}$
for the discriminant of
$\unicode[STIX]{x1D6E5}$
for the discriminant of
![]() ${\mathcal{O}}_{K}$
. If
${\mathcal{O}}_{K}$
. If
![]() $d\equiv 1,2(\text{mod}\;4)$
, then
$d\equiv 1,2(\text{mod}\;4)$
, then
![]() $\unicode[STIX]{x1D6E5}=-4d$
, and if
$\unicode[STIX]{x1D6E5}=-4d$
, and if
![]() $d\equiv 3(\text{mod}\;4)$
, then
$d\equiv 3(\text{mod}\;4)$
, then
![]() $\unicode[STIX]{x1D6E5}=-d$
. Letting
$\unicode[STIX]{x1D6E5}=-d$
. Letting
![]() $\unicode[STIX]{x1D6FE}=\big(\!\begin{smallmatrix}A_{\unicode[STIX]{x1D6FE}} & B_{\unicode[STIX]{x1D6FE}}\\ C_{\unicode[STIX]{x1D6FE}} & D_{\unicode[STIX]{x1D6FE}}\end{smallmatrix}\!\big)\in \operatorname{PSL}_{2}(\mathbb{C})$
, direct computation shows that
$\unicode[STIX]{x1D6FE}=\big(\!\begin{smallmatrix}A_{\unicode[STIX]{x1D6FE}} & B_{\unicode[STIX]{x1D6FE}}\\ C_{\unicode[STIX]{x1D6FE}} & D_{\unicode[STIX]{x1D6FE}}\end{smallmatrix}\!\big)\in \operatorname{PSL}_{2}(\mathbb{C})$
, direct computation shows that
![]() $\unicode[STIX]{x1D6FE}$
sends the horizontal line
$\unicode[STIX]{x1D6FE}$
sends the horizontal line
![]() $\widehat{\mathbb{R}}$
to a circle of curvature
$\widehat{\mathbb{R}}$
to a circle of curvature
If
![]() $\unicode[STIX]{x1D6FE}\in \operatorname{PSL}_{2}({\mathcal{O}}_{K})$
, then
$\unicode[STIX]{x1D6FE}\in \operatorname{PSL}_{2}({\mathcal{O}}_{K})$
, then
![]() $\unicode[STIX]{x1D705}(\unicode[STIX]{x1D6FE}(\widehat{\mathbb{R}}))\in \sqrt{-\unicode[STIX]{x1D6E5}}\mathbb{Z}$
.
$\unicode[STIX]{x1D705}(\unicode[STIX]{x1D6FE}(\widehat{\mathbb{R}}))\in \sqrt{-\unicode[STIX]{x1D6E5}}\mathbb{Z}$
.
We may assume without loss of generality that
![]() $N(\widehat{\mathbb{R}})=\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2$
. For
$N(\widehat{\mathbb{R}})=\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2$
. For
![]() $\operatorname{PSL}_{2}(\mathbb{Q})$
is transitive on circles of
$\operatorname{PSL}_{2}(\mathbb{Q})$
is transitive on circles of
![]() $\operatorname{PSL}_{2}(K)\widehat{\mathbb{R}}$
tangent to
$\operatorname{PSL}_{2}(K)\widehat{\mathbb{R}}$
tangent to
![]() $\widehat{\mathbb{R}}$
. Therefore we may choose
$\widehat{\mathbb{R}}$
. Therefore we may choose
![]() $N_{0}$
satisfying
$N_{0}$
satisfying
![]() $N_{0}(\widehat{\mathbb{R}})=\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2$
, and
$N_{0}(\widehat{\mathbb{R}})=\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2$
, and
![]() $NN_{0}^{-1}\in \operatorname{PSL}_{2}(\mathbb{Q})$
. Then we have
$NN_{0}^{-1}\in \operatorname{PSL}_{2}(\mathbb{Q})$
. Then we have
But
![]() $M^{\prime }=MNN_{0}^{-1}\in \operatorname{PSL}_{2}(K)$
,
$M^{\prime }=MNN_{0}^{-1}\in \operatorname{PSL}_{2}(K)$
,
![]() $N_{0}\in \operatorname{PSL}_{2}({\mathcal{O}}_{K})$
, and
$N_{0}\in \operatorname{PSL}_{2}({\mathcal{O}}_{K})$
, and
![]() ${\mathcal{A}}^{\prime }=N_{0}N^{-1}{\mathcal{A}}NN_{0}^{-1}$
is again Zariski dense, infinite covolume, geometrically finite and familial. Therefore let us assume
${\mathcal{A}}^{\prime }=N_{0}N^{-1}{\mathcal{A}}NN_{0}^{-1}$
is again Zariski dense, infinite covolume, geometrically finite and familial. Therefore let us assume
![]() $N(\widehat{\mathbb{R}})=\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2$
. By Lemma 2.1, we may again pass to a finite-index subgroup
$N(\widehat{\mathbb{R}})=\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2$
. By Lemma 2.1, we may again pass to a finite-index subgroup
![]() ${\mathcal{A}}$
of
${\mathcal{A}}$
of
![]() ${\mathcal{A}}^{\prime }$
and work with this group in order to prove Theorem 1.6.
${\mathcal{A}}^{\prime }$
and work with this group in order to prove Theorem 1.6.
With this choice of
![]() $N$
, for any
$N$
, for any
![]() $\unicode[STIX]{x1D6FE}\in {\mathcal{A}}$
, and
$\unicode[STIX]{x1D6FE}\in {\mathcal{A}}$
, and
![]() $M$
as above, the curvatures of the orbit
$M$
as above, the curvatures of the orbit
are given by the shifted quadratic form
in terms of the entries
![]() $a$
and
$a$
and
![]() $c$
of
$c$
of
![]() $\big(\!\begin{smallmatrix}a & b\\ c & d\end{smallmatrix}\!\big)\in \operatorname{PSL}_{2}(\mathbb{Z})$
. Therefore the packing
$\big(\!\begin{smallmatrix}a & b\\ c & d\end{smallmatrix}\!\big)\in \operatorname{PSL}_{2}(\mathbb{Z})$
. Therefore the packing
![]() $M{\mathcal{A}}(\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2)$
contains the curvatures of
$M{\mathcal{A}}(\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2)$
contains the curvatures of
where
![]() $L$
is the level of the congruence subgroup contained in
$L$
is the level of the congruence subgroup contained in
![]() ${\mathcal{A}}$
. Write
${\mathcal{A}}$
. Write
Then
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(a,c)$
is a shifted binary rational quadratic form, i.e.
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(a,c)$
is a shifted binary rational quadratic form, i.e.
where
In particular,
![]() $\widetilde{f}_{M\unicode[STIX]{x1D6FE}}$
has discriminant
$\widetilde{f}_{M\unicode[STIX]{x1D6FE}}$
has discriminant
![]() $\unicode[STIX]{x1D6E5}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}=-4(\Im (\overline{C_{M\unicode[STIX]{x1D6FE}}}D_{M\unicode[STIX]{x1D6FE}}))^{2}<0$
.
$\unicode[STIX]{x1D6E5}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}=-4(\Im (\overline{C_{M\unicode[STIX]{x1D6FE}}}D_{M\unicode[STIX]{x1D6FE}}))^{2}<0$
.
Unlike in the Apollonian case, it is possible that not all of these forms are primitive integral binary quadratic forms. However, their deviation from such forms, which is a function of the denominators introduced by
![]() $M$
, is uniformly bounded.
$M$
, is uniformly bounded.
Lemma 3.1. Let
![]() $M\in \operatorname{PSL}_{2}(K)$
and let
$M\in \operatorname{PSL}_{2}(K)$
and let
![]() $d_{1}$
be such that
$d_{1}$
be such that
![]() $d_{1}M\in \operatorname{PGL}_{2}({\mathcal{O}}_{K})$
. Up to multiplying and/or dividing by integers dividing
$d_{1}M\in \operatorname{PGL}_{2}({\mathcal{O}}_{K})$
. Up to multiplying and/or dividing by integers dividing
![]() $d_{1}^{4}$
, the form
$d_{1}^{4}$
, the form
![]() $\widetilde{f}_{M\unicode[STIX]{x1D6FE}}$
becomes a primitive integral binary quadratic form.
$\widetilde{f}_{M\unicode[STIX]{x1D6FE}}$
becomes a primitive integral binary quadratic form.
Proof. We have that
![]() $M\unicode[STIX]{x1D6FE}\in \operatorname{PSL}_{2}(K)$
. In particular, we have
$M\unicode[STIX]{x1D6FE}\in \operatorname{PSL}_{2}(K)$
. In particular, we have
By assumption,
![]() $C_{M\unicode[STIX]{x1D6FE}},D_{M\unicode[STIX]{x1D6FE}}\in (1/d_{1}){\mathcal{O}}_{K}$
. Write
$C_{M\unicode[STIX]{x1D6FE}},D_{M\unicode[STIX]{x1D6FE}}\in (1/d_{1}){\mathcal{O}}_{K}$
. Write
where
![]() $C_{M\unicode[STIX]{x1D6FE}}^{\prime },D_{M\unicode[STIX]{x1D6FE}}^{\prime }\in {\mathcal{O}}_{K}$
. In particular, the ideal generated by
$C_{M\unicode[STIX]{x1D6FE}}^{\prime },D_{M\unicode[STIX]{x1D6FE}}^{\prime }\in {\mathcal{O}}_{K}$
. In particular, the ideal generated by
![]() $C_{M\unicode[STIX]{x1D6FE}}^{\prime }$
and
$C_{M\unicode[STIX]{x1D6FE}}^{\prime }$
and
![]() $D_{M\unicode[STIX]{x1D6FE}}^{\prime }$
has norm at most
$D_{M\unicode[STIX]{x1D6FE}}^{\prime }$
has norm at most
![]() $d_{1}^{4}$
by (3.4).
$d_{1}^{4}$
by (3.4).
For any
![]() $C,D\in {\mathcal{O}}_{K}$
, if the integral form
$C,D\in {\mathcal{O}}_{K}$
, if the integral form
is imprimitive by a factor of, say,
![]() $e$
dividing all its coefficients, then
$e$
dividing all its coefficients, then
![]() $e\mid N(C,D)$
(the norm of the ideal). To see this, suppose
$e\mid N(C,D)$
(the norm of the ideal). To see this, suppose
![]() $p$
is prime and
$p$
is prime and
![]() $p^{k}\mid |Cx+Dy|^{2}$
for all
$p^{k}\mid |Cx+Dy|^{2}$
for all
![]() $(x,y)$
. If
$(x,y)$
. If
![]() $p$
is inert, then this implies
$p$
is inert, then this implies
![]() $(C,D)\subset p^{\lceil k/2\rceil }{\mathcal{O}}_{K}$
, so
$(C,D)\subset p^{\lceil k/2\rceil }{\mathcal{O}}_{K}$
, so
![]() $p^{k}\mid N(C,D)$
. If
$p^{k}\mid N(C,D)$
. If
![]() $p=\mathfrak{p}\overline{\mathfrak{p}}$
is split, then
$p=\mathfrak{p}\overline{\mathfrak{p}}$
is split, then
![]() $C$
,
$C$
,
![]() $D$
and
$D$
and
![]() $C+D$
are each contained in some ideal
$C+D$
are each contained in some ideal
![]() $\mathfrak{p}^{s}\overline{\mathfrak{p}}^{t}$
, where
$\mathfrak{p}^{s}\overline{\mathfrak{p}}^{t}$
, where
![]() $s+t=k$
. Call these three pairs
$s+t=k$
. Call these three pairs
![]() $(s,t)=(s_{1},t_{1}),(s_{2},t_{2}),(s_{3},t_{3})$
, ordered so that
$(s,t)=(s_{1},t_{1}),(s_{2},t_{2}),(s_{3},t_{3})$
, ordered so that
![]() $s_{1}<s_{2}<s_{3}$
. Then
$s_{1}<s_{2}<s_{3}$
. Then
since any two of
![]() $C,D,C+D$
generate
$C,D,C+D$
generate
![]() $(C,D)$
. Hence,
$(C,D)$
. Hence,
![]() $p^{k}\mid N(C,D)$
.
$p^{k}\mid N(C,D)$
.
Therefore
![]() $|C_{M\unicode[STIX]{x1D6FE}}^{\prime }x+D_{M\unicode[STIX]{x1D6FE}}^{\prime }y|^{2}$
is imprimitive by a factor dividing
$|C_{M\unicode[STIX]{x1D6FE}}^{\prime }x+D_{M\unicode[STIX]{x1D6FE}}^{\prime }y|^{2}$
is imprimitive by a factor dividing
![]() $d_{1}^{4}$
.
$d_{1}^{4}$
.
This shows that the integrality and/or primitivity of
![]() $\widetilde{f}_{M\unicode[STIX]{x1D6FE}}$
is achieved by multiplication and/or division by a factor of at most
$\widetilde{f}_{M\unicode[STIX]{x1D6FE}}$
is achieved by multiplication and/or division by a factor of at most
![]() $d_{1}^{4}$
, where
$d_{1}^{4}$
, where
![]() $d_{1}$
is independent of
$d_{1}$
is independent of
![]() $\unicode[STIX]{x1D6FE}$
.◻
$\unicode[STIX]{x1D6FE}$
.◻
Finally, the integral curvatures we seek to study are given by the union of the integers represented by these shifted forms, i.e.
4 Setup of the circle method
Throughout the circle method, there are the following growing parameters:
Their precise relationships, used to tune the result, are boxed throughout the paper, and these are: (4.1), (4.2), (4.11), (5.1) and (7.53). We collect these equations here for reference:

Each element
![]() $\unicode[STIX]{x1D6FE}\in {\mathcal{A}}$
corresponds to a shifted quadratic form of two variables that represents curvatures of circles tangent to
$\unicode[STIX]{x1D6FE}\in {\mathcal{A}}$
corresponds to a shifted quadratic form of two variables that represents curvatures of circles tangent to
![]() $M\unicode[STIX]{x1D6FE}(\mathbb{R}+\sqrt{\unicode[STIX]{x1D6E5}}/2)$
, given in (3.3). Note that
$M\unicode[STIX]{x1D6FE}(\mathbb{R}+\sqrt{\unicode[STIX]{x1D6E5}}/2)$
, given in (3.3). Note that
![]() $M$
is fixed throughout the paper.
$M$
is fixed throughout the paper.
Our goal is to show that almost all admissible integers are represented by some such shifted form. To do this, we consider this problem applied to growing subsets of
![]() ${\mathcal{A}}$
, and the shifted binary forms corresponding to the elements in these subsets.
${\mathcal{A}}$
, and the shifted binary forms corresponding to the elements in these subsets.
We now define these growing subsets. We choose three growing parameters
![]() $N$
,
$N$
,
![]() $T$
, and
$T$
, and
![]() $X$
such that
$X$
such that
Since
![]() $T$
is a small power of
$T$
is a small power of
![]() $N$
, we have that
$N$
, we have that
![]() $X$
is almost of the scale of
$X$
is almost of the scale of
![]() $N^{1/2}$
. We further write
$N^{1/2}$
. We further write
where
![]() $\unicode[STIX]{x1D708}>0$
is a large number depending only on the spectral gap of
$\unicode[STIX]{x1D708}>0$
is a large number depending only on the spectral gap of
![]() ${\mathcal{A}}$
, and we define the following set (counting with multiplicity):
${\mathcal{A}}$
, and we define the following set (counting with multiplicity):
 $$\begin{eqnarray}\mathfrak{F}=\mathfrak{F}_{T}=\left\{\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FE}_{2}:\begin{array}{@{}c@{}}\unicode[STIX]{x1D6FE}_{1},\unicode[STIX]{x1D6FE}_{2}\in {\mathcal{A}}\\ T_{1}/2\leqslant \Vert M\unicode[STIX]{x1D6FE}_{1}\Vert \leqslant T_{1}\\ T_{2}/2\leqslant \Vert \unicode[STIX]{x1D6FE}_{2}\Vert \leqslant T_{2}\\ \Im (\overline{C_{M\unicode[STIX]{x1D6FE}}}D_{M\unicode[STIX]{x1D6FE}})\geqslant T/100\end{array}\right\}.\end{eqnarray}$$
$$\begin{eqnarray}\mathfrak{F}=\mathfrak{F}_{T}=\left\{\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FE}_{2}:\begin{array}{@{}c@{}}\unicode[STIX]{x1D6FE}_{1},\unicode[STIX]{x1D6FE}_{2}\in {\mathcal{A}}\\ T_{1}/2\leqslant \Vert M\unicode[STIX]{x1D6FE}_{1}\Vert \leqslant T_{1}\\ T_{2}/2\leqslant \Vert \unicode[STIX]{x1D6FE}_{2}\Vert \leqslant T_{2}\\ \Im (\overline{C_{M\unicode[STIX]{x1D6FE}}}D_{M\unicode[STIX]{x1D6FE}})\geqslant T/100\end{array}\right\}.\end{eqnarray}$$
Here
![]() $\Vert \cdot \Vert$
stands for the Frobenius norm.
$\Vert \cdot \Vert$
stands for the Frobenius norm.
The reason why we define
![]() $\mathfrak{F}_{T}$
using two parameters
$\mathfrak{F}_{T}$
using two parameters
![]() $T_{1}$
and
$T_{1}$
and
![]() $T_{2}$
is that this is the necessary setup for Lemma 5.1 which is a result from Bourgain and Kontorovich [Reference Bourgain and KontorovichBK14a], and one that we will be using in the minor arcs analysis in this paper. Lemmas 5.2 and 5.3 are also stated within this setup, although for these two results one can set up the problem with just a growing ball of radius
$T_{2}$
is that this is the necessary setup for Lemma 5.1 which is a result from Bourgain and Kontorovich [Reference Bourgain and KontorovichBK14a], and one that we will be using in the minor arcs analysis in this paper. Lemmas 5.2 and 5.3 are also stated within this setup, although for these two results one can set up the problem with just a growing ball of radius
![]() $T$
.
$T$
.
Finally, we wish to let two integers
![]() $x$
and
$x$
and
![]() $y$
range over two sets of integers that are
$y$
range over two sets of integers that are
![]() $\asymp X$
. For this reason we introduce a smooth function
$\asymp X$
. For this reason we introduce a smooth function
![]() $\unicode[STIX]{x1D713}$
supported on
$\unicode[STIX]{x1D713}$
supported on
![]() $[1,2]$
, such that
$[1,2]$
, such that
![]() $\unicode[STIX]{x1D713}\geqslant 0$
and
$\unicode[STIX]{x1D713}\geqslant 0$
and
![]() $\int _{\mathbb{R}}\unicode[STIX]{x1D713}(x)\,dx=1$
. If
$\int _{\mathbb{R}}\unicode[STIX]{x1D713}(x)\,dx=1$
. If
![]() $(Lx+1,Ly)=1$
, then
$(Lx+1,Ly)=1$
, then
![]() $\mathfrak{f}_{M\cdot \unicode[STIX]{x1D6FE}}(Lx+1,Ly)$
is a curvature.
$\mathfrak{f}_{M\cdot \unicode[STIX]{x1D6FE}}(Lx+1,Ly)$
is a curvature.
We then define
 $$\begin{eqnarray}{\mathcal{R}}_{N}(n)=\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ x,y\in \mathbb{Z} \\ (Lx+1,Ly)=1}}\unicode[STIX]{x1D713}\biggl(\frac{Lx+1}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Ly}{X}\biggr)\mathbf{1}\{\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)=n\}.\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{R}}_{N}(n)=\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ x,y\in \mathbb{Z} \\ (Lx+1,Ly)=1}}\unicode[STIX]{x1D713}\biggl(\frac{Lx+1}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Ly}{X}\biggr)\mathbf{1}\{\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)=n\}.\end{eqnarray}$$
If
![]() ${\mathcal{R}}_{N}(n)>0$
then
${\mathcal{R}}_{N}(n)>0$
then
![]() $n$
is a curvature. Our goal is to show that
$n$
is a curvature. Our goal is to show that
![]() ${\mathcal{R}}_{N}(n)>0$
for almost all
${\mathcal{R}}_{N}(n)>0$
for almost all
![]() $n$
, in the sense described in Theorem 1.6.
$n$
, in the sense described in Theorem 1.6.
From [Reference VinogradovVin14, Theorem 2.2], the size of
![]() $\mathfrak{F}_{T}$
is
$\mathfrak{F}_{T}$
is
![]() $\asymp T^{2\unicode[STIX]{x1D6FF}}$
(recall that
$\asymp T^{2\unicode[STIX]{x1D6FF}}$
(recall that
![]() $\unicode[STIX]{x1D6FF}$
is the critical exponent of
$\unicode[STIX]{x1D6FF}$
is the critical exponent of
![]() ${\mathcal{A}}$
). Given the definition of
${\mathcal{A}}$
). Given the definition of
![]() $\mathfrak{F}_{T}$
, the function
$\mathfrak{F}_{T}$
, the function
![]() ${\mathcal{R}}_{N}$
is supported on
${\mathcal{R}}_{N}$
is supported on
![]() $n\asymp N$
. We obtain
$n\asymp N$
. We obtain
It is expected that this is roughly equidistributed on the set of admissible integers, so that
for every admissible
![]() $n$
.
$n$
.
It would be ideal to show (4.5). However, current technology does not enable us to prove this. Instead, we will show that
![]() ${\mathcal{R}}_{N}(n)\gg T^{2\unicode[STIX]{x1D6FF}-2}$
for every admissible integer in
${\mathcal{R}}_{N}(n)\gg T^{2\unicode[STIX]{x1D6FF}-2}$
for every admissible integer in
![]() $[N/2,N]$
outside of an exceptional set of size
$[N/2,N]$
outside of an exceptional set of size
![]() $O(N^{1-\unicode[STIX]{x1D702}})$
for some
$O(N^{1-\unicode[STIX]{x1D702}})$
for some
![]() $\unicode[STIX]{x1D702}>0$
.
$\unicode[STIX]{x1D702}>0$
.
Notice that in the definition of
![]() ${\mathcal{R}}_{N}$
in (4.4), the second sum is over pairs of integers
${\mathcal{R}}_{N}$
in (4.4), the second sum is over pairs of integers
![]() $(Lx+1,Ly)$
which satisfy a coprimality condition that is hard to track directly in computations. We hence rewrite this sum as one over all pairs
$(Lx+1,Ly)$
which satisfy a coprimality condition that is hard to track directly in computations. We hence rewrite this sum as one over all pairs
![]() $(Lx+1,Ly)$
using Möbius orthogonality:
$(Lx+1,Ly)$
using Möbius orthogonality:
Then
Notice that if
![]() $u\mid (Lx+1,Ly)$
, then
$u\mid (Lx+1,Ly)$
, then
![]() $(u,L)=1$
, so
$(u,L)=1$
, so
![]() $y\equiv 0\;(\text{mod}\;u)$
and
$y\equiv 0\;(\text{mod}\;u)$
and
![]() $Lx\equiv -1\;(\text{mod}\;u)$
. Let
$Lx\equiv -1\;(\text{mod}\;u)$
. Let
![]() $u^{\ast }$
be the integer from
$u^{\ast }$
be the integer from
![]() $[1,L-1]$
such that
$[1,L-1]$
such that
![]() $uu^{\ast }\equiv 1\;(\text{mod}\;L)$
. Then we can write
$uu^{\ast }\equiv 1\;(\text{mod}\;L)$
. Then we can write
With this manipulation, the innermost sum becomes one over free variables
![]() $x,y$
, allowing us to use abelian harmonic analysis to analyze it.
$x,y$
, allowing us to use abelian harmonic analysis to analyze it.
To facilitate our analysis we will study a relative of
![]() ${\mathcal{R}}_{N}$
which we denote by
${\mathcal{R}}_{N}$
which we denote by
![]() ${\mathcal{R}}_{N}^{U}$
, where
${\mathcal{R}}_{N}^{U}$
, where
![]() $U$
is a small power of
$U$
is a small power of
![]() $N$
, and determined at (7.53). We restrict the
$N$
, and determined at (7.53). We restrict the
![]() $u$
-sum in (4.7) to
$u$
-sum in (4.7) to
![]() $u<U$
and define
$u<U$
and define
 $$\begin{eqnarray}{\mathcal{R}}_{N}^{U}(n)=\mathop{\sum }_{\substack{ u<U \\ (u,L)=1}}\unicode[STIX]{x1D707}(u)\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{x,y\in \mathbb{Z}}\unicode[STIX]{x1D713}\biggl(\frac{Lux+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Luy}{X}\biggr)\mathbf{1}\{\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },Luy)=n\}.\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{R}}_{N}^{U}(n)=\mathop{\sum }_{\substack{ u<U \\ (u,L)=1}}\unicode[STIX]{x1D707}(u)\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{x,y\in \mathbb{Z}}\unicode[STIX]{x1D713}\biggl(\frac{Lux+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Luy}{X}\biggr)\mathbf{1}\{\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },Luy)=n\}.\end{eqnarray}$$
The following lemma shows that the difference between
![]() ${\mathcal{R}}_{N}$
and
${\mathcal{R}}_{N}$
and
![]() ${\mathcal{R}}_{N}^{U}$
is small in
${\mathcal{R}}_{N}^{U}$
is small in
![]() $l_{1}$
.
$l_{1}$
.
Lemma 4.1.
 $$\begin{eqnarray}\displaystyle & & \displaystyle \mathop{\sum }_{n\in \mathbb{Z}}|{\mathcal{R}}_{N}(n)-{\mathcal{R}}_{N}^{U}(n)|\nonumber\\ \displaystyle & & \displaystyle \quad \leqslant \,\mathop{\sum }_{n\in \mathbb{Z}}\biggl|\mathop{\sum }_{u\geqslant U}\unicode[STIX]{x1D707}(u)\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{x,y\in \mathbb{Z}}\unicode[STIX]{x1D713}\biggl(\frac{Lux+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Luy}{X}\biggr)\mathbf{1}\{\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },Luy)=n\}\biggr|\nonumber\\ \displaystyle & & \displaystyle \quad \leqslant \,\mathop{\sum }_{n\in \mathbb{Z}}\mathop{\sum }_{u\geqslant U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{x,y\in \mathbb{Z}}\unicode[STIX]{x1D713}\biggl(\frac{Lux+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Luy}{X}\biggr)\mathbf{1}\{\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },Luy)=n\}\nonumber\\ \displaystyle & & \displaystyle \quad \leqslant \,\mathop{\sum }_{u\geqslant U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{x,y\in \mathbb{Z}}\unicode[STIX]{x1D713}\biggl(\frac{Lux+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Luy}{X}\biggr)\nonumber\\ \displaystyle & & \displaystyle \quad \ll \,\mathop{\sum }_{u\geqslant U}T^{2\unicode[STIX]{x1D6FF}}\frac{X^{2}}{u^{2}}\ll \frac{T^{2\unicode[STIX]{x1D6FF}}X^{2}}{U}.\Box \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \mathop{\sum }_{n\in \mathbb{Z}}|{\mathcal{R}}_{N}(n)-{\mathcal{R}}_{N}^{U}(n)|\nonumber\\ \displaystyle & & \displaystyle \quad \leqslant \,\mathop{\sum }_{n\in \mathbb{Z}}\biggl|\mathop{\sum }_{u\geqslant U}\unicode[STIX]{x1D707}(u)\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{x,y\in \mathbb{Z}}\unicode[STIX]{x1D713}\biggl(\frac{Lux+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Luy}{X}\biggr)\mathbf{1}\{\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },Luy)=n\}\biggr|\nonumber\\ \displaystyle & & \displaystyle \quad \leqslant \,\mathop{\sum }_{n\in \mathbb{Z}}\mathop{\sum }_{u\geqslant U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{x,y\in \mathbb{Z}}\unicode[STIX]{x1D713}\biggl(\frac{Lux+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Luy}{X}\biggr)\mathbf{1}\{\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },Luy)=n\}\nonumber\\ \displaystyle & & \displaystyle \quad \leqslant \,\mathop{\sum }_{u\geqslant U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{x,y\in \mathbb{Z}}\unicode[STIX]{x1D713}\biggl(\frac{Lux+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Luy}{X}\biggr)\nonumber\\ \displaystyle & & \displaystyle \quad \ll \,\mathop{\sum }_{u\geqslant U}T^{2\unicode[STIX]{x1D6FF}}\frac{X^{2}}{u^{2}}\ll \frac{T^{2\unicode[STIX]{x1D6FF}}X^{2}}{U}.\Box \nonumber\end{eqnarray}$$
We will study
![]() ${\mathcal{R}}_{N}$
(and
${\mathcal{R}}_{N}$
(and
![]() ${\mathcal{R}}_{N}^{U}$
) via its Fourier transform,
${\mathcal{R}}_{N}^{U}$
) via its Fourier transform,
 $$\begin{eqnarray}\widehat{{\mathcal{R}}}_{N}(\unicode[STIX]{x1D703})=\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ x,y\in \mathbb{Z} \\ (Lx+1,Ly)=1}}\unicode[STIX]{x1D713}\biggl(\frac{Lx+1}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Ly}{X}\biggr)e(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)\unicode[STIX]{x1D703}),\end{eqnarray}$$
$$\begin{eqnarray}\widehat{{\mathcal{R}}}_{N}(\unicode[STIX]{x1D703})=\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ x,y\in \mathbb{Z} \\ (Lx+1,Ly)=1}}\unicode[STIX]{x1D713}\biggl(\frac{Lx+1}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Ly}{X}\biggr)e(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)\unicode[STIX]{x1D703}),\end{eqnarray}$$
using the fact that we can recover
![]() ${\mathcal{R}}_{N}$
from
${\mathcal{R}}_{N}$
from
![]() $\widehat{{\mathcal{R}}}_{N}$
via the Fourier inversion formula,
$\widehat{{\mathcal{R}}}_{N}$
via the Fourier inversion formula,
It is in evaluating this integral in (4.10) that the circle method will be applied.
By Dirichlet’s approximation theorem, given any positive integer
![]() $J$
, for every real number
$J$
, for every real number
![]() $\unicode[STIX]{x1D703}\in [0,1)$
, there exist integers
$\unicode[STIX]{x1D703}\in [0,1)$
, there exist integers
![]() $r,q$
such that
$r,q$
such that
![]() $1\leqslant q\leqslant J$
and
$1\leqslant q\leqslant J$
and
![]() $|\unicode[STIX]{x1D703}-r/q|<1/(q\cdot J)$
. The integer
$|\unicode[STIX]{x1D703}-r/q|<1/(q\cdot J)$
. The integer
![]() $J$
is called the depth of approximation, and we will take
$J$
is called the depth of approximation, and we will take
The general philosophy of the circle method is that most of the contribution to the integral (4.10) should come from neighborhoods of rationals with small denominator. Such neighborhoods are called major arcs. One shows that (4.10) is bounded below, by bounding the major arcs below, and then bounding the minor arcs, considered an error term, above.
In our case the major arcs are comprised of
![]() $\unicode[STIX]{x1D703}\in [0,1]$
such that
$\unicode[STIX]{x1D703}\in [0,1]$
such that
![]() $|\unicode[STIX]{x1D703}-r/q|\leqslant K_{0}/N$
, where
$|\unicode[STIX]{x1D703}-r/q|\leqslant K_{0}/N$
, where
![]() $q\leqslant Q_{0}$
. Here
$q\leqslant Q_{0}$
. Here
![]() $Q_{0}$
and
$Q_{0}$
and
![]() $K_{0}$
are small powers of
$K_{0}$
are small powers of
![]() $N$
which depend on the spectral gap and are given in (5.1). Write
$N$
which depend on the spectral gap and are given in (5.1). Write
![]() $\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D703}-r/q$
.
$\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D703}-r/q$
.
To define what we call the major arc contribution, we first introduce the hat function
whose Fourier transform is
In particular,
![]() $\hat{\mathfrak{t}}$
is non-negative (we take
$\hat{\mathfrak{t}}$
is non-negative (we take
![]() $\hat{\mathfrak{t}}(0)=1$
).
$\hat{\mathfrak{t}}(0)=1$
).
From
![]() $\mathfrak{t}$
, we construct a spike function
$\mathfrak{t}$
, we construct a spike function
![]() $\mathfrak{T}$
, with period
$\mathfrak{T}$
, with period
![]() $1$
on
$1$
on
![]() $\mathbb{R}$
, to capture the major arcs:
$\mathbb{R}$
, to capture the major arcs:
Our main term is then
and the error term is
Similarly, we define
and
In Lemma 4.1 we have shown that
![]() $\Vert {\mathcal{R}}_{N}-{\mathcal{R}}_{N}^{U}\Vert _{l_{1}}$
is small. Running the same argument as in the proof of Lemma 4.1, one can bound the difference between
$\Vert {\mathcal{R}}_{N}-{\mathcal{R}}_{N}^{U}\Vert _{l_{1}}$
is small. Running the same argument as in the proof of Lemma 4.1, one can bound the difference between
![]() ${\mathcal{M}}_{N}$
and
${\mathcal{M}}_{N}$
and
![]() ${\mathcal{M}}_{N}^{U}$
in
${\mathcal{M}}_{N}^{U}$
in
![]() $l_{1}$
norm. Then, one obtains the following lemma.
$l_{1}$
norm. Then, one obtains the following lemma.
Lemma 4.2.
Together, Lemmas 4.1 and 4.2 then imply the following inequality.
Lemma 4.3.
Appropriate lower bounds on
![]() ${\mathcal{M}}_{N}(n)$
and average upper bounds on
${\mathcal{M}}_{N}(n)$
and average upper bounds on
![]() ${\mathcal{E}}_{N}^{U}$
are then combined to prove the main theorem. Specifically, in § 6 we show the following theorem.
${\mathcal{E}}_{N}^{U}$
are then combined to prove the main theorem. Specifically, in § 6 we show the following theorem.
Theorem 4.4. For any
![]() $n\in [N/2,N]\cap {\mathcal{K}}_{a}$
, we have
$n\in [N/2,N]\cap {\mathcal{K}}_{a}$
, we have
In §§ 7.2–7.4 we work towards giving an
![]() $l_{2}$
bound for
$l_{2}$
bound for
![]() ${\mathcal{E}}_{N}^{U}$
, as stated in the following theorem.
${\mathcal{E}}_{N}^{U}$
, as stated in the following theorem.
Theorem 4.5.
The value of
![]() $\unicode[STIX]{x1D702}$
will be described in the course of the proof.
$\unicode[STIX]{x1D702}$
will be described in the course of the proof.
Let
![]() $\mathbf{1}_{[N/2,N]}$
be the indicator function on
$\mathbf{1}_{[N/2,N]}$
be the indicator function on
![]() $\mathbb{Z}$
defined by
$\mathbb{Z}$
defined by
Then, using the Cauchy–Schwarz inequality, we have
replacing
![]() $\unicode[STIX]{x1D702}/2$
by
$\unicode[STIX]{x1D702}/2$
by
![]() $\unicode[STIX]{x1D702}$
.
$\unicode[STIX]{x1D702}$
.
Using (4.17) together with Lemma 4.3, we have
We are now able to prove Theorem 1.6, assuming Theorem 4.4 and (4.18).
Proof of Theorem 1.6.
Let
![]() $\mathfrak{E}(N)$
be the set of exceptional numbers
$\mathfrak{E}(N)$
be the set of exceptional numbers
![]() $[N/2,N]$
(those admissible but not occurring as curvatures). Then, by (4.18),
$[N/2,N]$
(those admissible but not occurring as curvatures). Then, by (4.18),
For
![]() $n\in \mathfrak{E}(N)$
,
$n\in \mathfrak{E}(N)$
,
![]() ${\mathcal{R}}_{N}(n)=0$
and, by Theorem 4.4,
${\mathcal{R}}_{N}(n)=0$
and, by Theorem 4.4,
![]() ${\mathcal{M}}_{N}(n)\gg T^{2\unicode[STIX]{x1D6FF}-2}$
. Therefore
${\mathcal{M}}_{N}(n)\gg T^{2\unicode[STIX]{x1D6FF}-2}$
. Therefore
Thus
so that
This is the desired result for the interval
![]() $[N/2,N]$
, and we extend it to the full interval
$[N/2,N]$
, and we extend it to the full interval
![]() $[0,N]$
as follows. Divide
$[0,N]$
as follows. Divide
![]() $[0,N]$
into a union of subintervals dyadically:
$[0,N]$
into a union of subintervals dyadically:
![]() $[0,N]=[N/2,N]\,\cup \,[N/4,N/2]\,\cup \,[N/8,N/4]\,\cup \,\cdots \,$
. Applying (4.19) to each subinterval (replacing
$[0,N]=[N/2,N]\,\cup \,[N/4,N/2]\,\cup \,[N/8,N/4]\,\cup \,\cdots \,$
. Applying (4.19) to each subinterval (replacing
![]() $N$
by
$N$
by
![]() $N/2^{m}$
for
$N/2^{m}$
for
![]() $0\leqslant m<\log _{2}(N)$
) and collecting the error terms, we obtain Theorem 1.6 as desired.◻
$0\leqslant m<\log _{2}(N)$
) and collecting the error terms, we obtain Theorem 1.6 as desired.◻
5 Preliminary lemmas
In this section we introduce several lemmas due to Bourgain and Kontorovich which will be used in later sections. Note that they are not stated exactly as the lemmas which we cite from [Reference Bourgain and KontorovichBK14a], which are stated in the framework of counting in orbits of the Apollonian group in
![]() $\text{O}_{\mathbb{R}}(3,1)$
acting on Descartes quadruples in
$\text{O}_{\mathbb{R}}(3,1)$
acting on Descartes quadruples in
![]() $\mathbb{Z}^{4}$
, while we use the lemmas in the context of subgroups of
$\mathbb{Z}^{4}$
, while we use the lemmas in the context of subgroups of
![]() $\text{PSL}_{2}({\mathcal{O}}_{K})$
acting on a circle. However, the proofs of these lemmas in [Reference Bourgain and KontorovichBK14a] are very general, and apply almost verbatim to the context in which we phrase them below, with their group
$\text{PSL}_{2}({\mathcal{O}}_{K})$
acting on a circle. However, the proofs of these lemmas in [Reference Bourgain and KontorovichBK14a] are very general, and apply almost verbatim to the context in which we phrase them below, with their group
![]() $\unicode[STIX]{x1D6E4}$
replaced by
$\unicode[STIX]{x1D6E4}$
replaced by
![]() ${\mathcal{A}}$
in our case, and the set of first coordinates of points in the orbit of
${\mathcal{A}}$
in our case, and the set of first coordinates of points in the orbit of
![]() $\unicode[STIX]{x1D6E4}$
acting on a vector replaced by the curvatures of the circles one gets as in the orbit of
$\unicode[STIX]{x1D6E4}$
acting on a vector replaced by the curvatures of the circles one gets as in the orbit of
![]() ${\mathcal{A}}$
that we consider. We note also that in Lemma 5.2 we sum over cosets of
${\mathcal{A}}$
that we consider. We note also that in Lemma 5.2 we sum over cosets of
![]() ${\mathcal{A}}(q)$
while Bourgain and Kontorovich sum over cosets of a larger subgroup. However, this is not necessary to execute the circle method as we do here. Finally, as stated below, Bourgain and Kontorovich’s bounds involving
${\mathcal{A}}(q)$
while Bourgain and Kontorovich sum over cosets of a larger subgroup. However, this is not necessary to execute the circle method as we do here. Finally, as stated below, Bourgain and Kontorovich’s bounds involving
![]() $T^{\unicode[STIX]{x1D6FF}}$
and
$T^{\unicode[STIX]{x1D6FF}}$
and
![]() $T^{\unicode[STIX]{x1D6E9}}$
are adjusted to involve
$T^{\unicode[STIX]{x1D6E9}}$
are adjusted to involve
![]() $T^{2\unicode[STIX]{x1D6FF}}$
and
$T^{2\unicode[STIX]{x1D6FF}}$
and
![]() $T^{2\unicode[STIX]{x1D6E9}}$
, respectively. This is because we work in
$T^{2\unicode[STIX]{x1D6E9}}$
, respectively. This is because we work in
![]() $\text{PSL}_{2}$
and not in
$\text{PSL}_{2}$
and not in
![]() $\text{SO}_{\mathbb{R}}(3,1)$
as is the case in [Reference Bourgain and KontorovichBK14a], and the spin homomorphism from
$\text{SO}_{\mathbb{R}}(3,1)$
as is the case in [Reference Bourgain and KontorovichBK14a], and the spin homomorphism from
![]() $\text{PSL}_{2}$
to
$\text{PSL}_{2}$
to
![]() $\text{SO}_{\mathbb{R}}(3,1)$
is quadratic in the entries of the matrices of
$\text{SO}_{\mathbb{R}}(3,1)$
is quadratic in the entries of the matrices of
![]() $\text{PSL}_{2}$
.
$\text{PSL}_{2}$
.
These results are the point at which the spectral gap for
![]() ${\mathcal{A}}$
feeds into our analysis. The first two of these are statements about equidistribution modulo
${\mathcal{A}}$
feeds into our analysis. The first two of these are statements about equidistribution modulo
![]() $q$
. The first says that the curvatures cannot have too strong a preference for a given congruence class modulo
$q$
. The first says that the curvatures cannot have too strong a preference for a given congruence class modulo
![]() $q$
, as
$q$
, as
![]() $\unicode[STIX]{x1D6FE}$
varies. It is used in the minor arc analysis.
$\unicode[STIX]{x1D6FE}$
varies. It is used in the minor arc analysis.
Lemma 5.1 (Bourgain and Kontorovich [Reference Bourgain and KontorovichBK14a, Lemma 5.2]).
There exist a positive constant
![]() $\unicode[STIX]{x1D708}$
and some
$\unicode[STIX]{x1D708}$
and some
![]() $\unicode[STIX]{x1D702}_{0}>0$
which only depend on the spectral gap of
$\unicode[STIX]{x1D702}_{0}>0$
which only depend on the spectral gap of
![]() ${\mathcal{A}}$
, such that for any
${\mathcal{A}}$
, such that for any
![]() $1\leqslant q<N$
and any
$1\leqslant q<N$
and any
![]() $r\;(\text{mod}\;q)$
,
$r\;(\text{mod}\;q)$
,
where
![]() $T=T_{1}T_{2}$
(for notation see the definition of
$T=T_{1}T_{2}$
(for notation see the definition of
![]() $\mathfrak{F}$
in (4.3)). The implied constant is independent of
$\mathfrak{F}$
in (4.3)). The implied constant is independent of
![]() $r$
.
$r$
.
The second lemma states that the behavior of the form
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
on
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
on
![]() $\unicode[STIX]{x1D6FE}$
from any given congruence class is independent of the congruence class, in the sense that each class contributes equally to an exponential sum. It is used for the setup of the major arc analysis, to separate the non-Archimedean and Archimedean contributions. Write
$\unicode[STIX]{x1D6FE}$
from any given congruence class is independent of the congruence class, in the sense that each class contributes equally to an exponential sum. It is used for the setup of the major arc analysis, to separate the non-Archimedean and Archimedean contributions. Write
![]() ${\mathcal{A}}(q)$
for the kernel of reduction modulo
${\mathcal{A}}(q)$
for the kernel of reduction modulo
![]() $q$
.
$q$
.
Lemma 5.2 (Bourgain and Kontorovich [Reference Bourgain and KontorovichBK14a], Lemma 5.3).
Let
![]() $1<K<T_{2}^{1/10}$
, fix
$1<K<T_{2}^{1/10}$
, fix
![]() $|\unicode[STIX]{x1D6FD}|<K/N$
, and fix
$|\unicode[STIX]{x1D6FD}|<K/N$
, and fix
![]() $x,y\asymp X$
. Then for any
$x,y\asymp X$
. Then for any
![]() $\unicode[STIX]{x1D6FE}_{0}\in {\mathcal{A}}$
, any
$\unicode[STIX]{x1D6FE}_{0}\in {\mathcal{A}}$
, any
![]() $q\geqslant 1$
, we have
$q\geqslant 1$
, we have
where
![]() $\unicode[STIX]{x1D6E9}_{1}<\unicode[STIX]{x1D6FF}$
depends only on the spectral gap for
$\unicode[STIX]{x1D6E9}_{1}<\unicode[STIX]{x1D6FF}$
depends only on the spectral gap for
![]() ${\mathcal{A}}$
, and the implied constant does not depend on
${\mathcal{A}}$
, and the implied constant does not depend on
![]() $q,\unicode[STIX]{x1D6FE}_{0},x$
or
$q,\unicode[STIX]{x1D6FE}_{0},x$
or
![]() $y$
.
$y$
.
The last lemma is used to bound the Archimedean piece of the major arc analysis. It uses the spectral gap to control the error in counting
![]() $\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}$
where
$\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}$
where
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
takes certain values.
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
takes certain values.
Lemma 5.3 (Bourgain and Kontorovich [Reference Bourgain and KontorovichBK14a, Lemma 5.4]).
Fix
![]() $N/2\leqslant n\leqslant N,1<K\leqslant T_{2}^{1/10}$
, and
$N/2\leqslant n\leqslant N,1<K\leqslant T_{2}^{1/10}$
, and
![]() $x,y\asymp X$
. Then
$x,y\asymp X$
. Then
where
![]() $\unicode[STIX]{x1D6E9}_{2}<\unicode[STIX]{x1D6FF}$
depends only on the spectral gap for
$\unicode[STIX]{x1D6E9}_{2}<\unicode[STIX]{x1D6FF}$
depends only on the spectral gap for
![]() ${\mathcal{A}}$
. The implied constant is independent of
${\mathcal{A}}$
. The implied constant is independent of
![]() $x,y$
and
$x,y$
and
![]() $n$
.
$n$
.
Let
![]() $\unicode[STIX]{x1D6E9}$
be the larger of the
$\unicode[STIX]{x1D6E9}$
be the larger of the
![]() $\unicode[STIX]{x1D6E9}$
s that satisfy Lemmas 5.2 and 5.3 respectively. Then we set the two parameters
$\unicode[STIX]{x1D6E9}$
s that satisfy Lemmas 5.2 and 5.3 respectively. Then we set the two parameters
![]() $Q_{0},K_{0}$
as
$Q_{0},K_{0}$
as
6 Major arc analysis
In this section we prove Theorem 4.4 bounding
![]() ${\mathcal{M}}_{N}(n)$
below. We give a brief overview of the argument, before treating all the details. First, we will write
${\mathcal{M}}_{N}(n)$
below. We give a brief overview of the argument, before treating all the details. First, we will write
where
![]() $\mathfrak{M}(n)$
is the Archimedean part and
$\mathfrak{M}(n)$
is the Archimedean part and
![]() $\mathfrak{S}_{Q_{0}}(n)$
is the non-Archimedean part (depending on a parameter
$\mathfrak{S}_{Q_{0}}(n)$
is the non-Archimedean part (depending on a parameter
![]() $Q_{0}$
controlling the size of the major arcs); both depend on
$Q_{0}$
controlling the size of the major arcs); both depend on
![]() $x,y$
. We need Lemma 5.2 (dependent on the spectral gap) in order to accomplish this separation of Archimedean from non-Archimedean.
$x,y$
. We need Lemma 5.2 (dependent on the spectral gap) in order to accomplish this separation of Archimedean from non-Archimedean.
We can use Lemma 5.3 to give a lower bound on the Archimedean part, and most of the attention of this section is given to bounding
![]() $\mathfrak{S}_{Q_{0}}(n)$
below. This requires a careful local analysis that is one of the novelties of our treatment as compared with previous works [Reference Bourgain and KontorovichBK14a, Reference ZhangZha18b].
$\mathfrak{S}_{Q_{0}}(n)$
below. This requires a careful local analysis that is one of the novelties of our treatment as compared with previous works [Reference Bourgain and KontorovichBK14a, Reference ZhangZha18b].
The limit
![]() $\mathfrak{S}(n)=\lim _{Q_{0}\rightarrow \infty }\mathfrak{S}_{Q_{0}}(n)$
is the singular series, whose purpose is to be supported only on the admissible values of
$\mathfrak{S}(n)=\lim _{Q_{0}\rightarrow \infty }\mathfrak{S}_{Q_{0}}(n)$
is the singular series, whose purpose is to be supported only on the admissible values of
![]() $n$
, and bounded below where it is supported. We break it down as
$n$
, and bounded below where it is supported. We break it down as
In turn,
where
![]() $\unicode[STIX]{x1D70F}_{q}(r)$
is the probability of the quadratic form
$\unicode[STIX]{x1D70F}_{q}(r)$
is the probability of the quadratic form
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
taking on the value
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
taking on the value
![]() $r$
modulo
$r$
modulo
![]() $q$
, as
$q$
, as
![]() $\unicode[STIX]{x1D6FE}$
ranges among cosets of
$\unicode[STIX]{x1D6FE}$
ranges among cosets of
![]() ${\mathcal{A}}$
modulo
${\mathcal{A}}$
modulo
![]() $q$
, and
$q$
, and
![]() $c_{q}$
is a Ramanujan sum, which is multiplicative with respect to
$c_{q}$
is a Ramanujan sum, which is multiplicative with respect to
![]() $q$
. For a prime
$q$
. For a prime
![]() $p$
, one should think of
$p$
, one should think of
![]() $B_{p}(n)$
as measuring some deviation from the equidistribution of the probabilities
$B_{p}(n)$
as measuring some deviation from the equidistribution of the probabilities
![]() $\unicode[STIX]{x1D70F}_{p}(n)$
modulo
$\unicode[STIX]{x1D70F}_{p}(n)$
modulo
![]() $p$
;
$p$
;
![]() $B_{p^{k}}(n)$
for larger
$B_{p^{k}}(n)$
for larger
![]() $k$
gives finer information about the behavior of these probabilities as we lift to powers of
$k$
gives finer information about the behavior of these probabilities as we lift to powers of
![]() $p$
. This is captured by the relationship
$p$
. This is captured by the relationship
This factor is non-zero if and only if
![]() $n$
is represented as a curvature modulo
$n$
is represented as a curvature modulo
![]() $p^{k}$
.
$p^{k}$
.
The goal, then, is to understand
![]() $B_{p^{k}}(n)$
. First, we use strong approximation for
$B_{p^{k}}(n)$
. First, we use strong approximation for
![]() ${\mathcal{A}}$
to show that the
${\mathcal{A}}$
to show that the
![]() $B_{p^{k}}(n)$
eventually vanish as
$B_{p^{k}}(n)$
eventually vanish as
![]() $k$
increases. In particular,
$k$
increases. In particular,
![]() $B_{p^{k}}(n)=0$
once we have uniform lifting in the sense of strong approximation (Lemmas 6.3 and 6.4). We find that for all but finitely many primes,
$B_{p^{k}}(n)=0$
once we have uniform lifting in the sense of strong approximation (Lemmas 6.3 and 6.4). We find that for all but finitely many primes,
![]() $B_{p^{k}}(n)=0$
for
$B_{p^{k}}(n)=0$
for
![]() $k\geqslant 2$
. Therefore
$k\geqslant 2$
. Therefore
![]() $\mathfrak{S}(n)$
is controlled by the product over good primes
$\mathfrak{S}(n)$
is controlled by the product over good primes
![]() $\prod _{p}^{\prime }(1+B_{p}(n))$
.
$\prod _{p}^{\prime }(1+B_{p}(n))$
.
The final step is to control
![]() $B_{p}(n)$
: we show that for
$B_{p}(n)$
: we show that for
![]() $p\nmid n$
,
$p\nmid n$
,
![]() $B_{p}(n)=O(1/p)$
, while for
$B_{p}(n)=O(1/p)$
, while for
![]() $p\mid n$
,
$p\mid n$
,
![]() $B_{p}(n)=O(1/p^{2})$
. This requires a direct counting argument, finding all solutions modulo
$B_{p}(n)=O(1/p^{2})$
. This requires a direct counting argument, finding all solutions modulo
![]() $p$
to the requirement that the curvature be equal to
$p$
to the requirement that the curvature be equal to
![]() $n$
; at its core is an argument using Gauss sums. In other words, we show that equidistribution of curvatures modulo
$n$
; at its core is an argument using Gauss sums. In other words, we show that equidistribution of curvatures modulo
![]() $p$
does not fail too badly.
$p$
does not fail too badly.
Now we begin. From (4.13), (4.12) and (4.9), we have
 $$\begin{eqnarray}\displaystyle {\mathcal{M}}_{N}(n) & = & \displaystyle \int _{0}^{1}\mathfrak{T}(\unicode[STIX]{x1D703})\widehat{{\mathcal{R}}}_{N}(\unicode[STIX]{x1D703})e(-n\unicode[STIX]{x1D703})\,d\unicode[STIX]{x1D703}\nonumber\\ \displaystyle & = & \displaystyle \int _{-\infty }^{\infty }\mathop{\sum }_{q<Q_{0}}\sum _{r(q)}\mathfrak{t}\biggl(\frac{N}{K_{0}}\unicode[STIX]{x1D6FD}\biggr)\widehat{{\mathcal{R}}}_{N}\biggl(\unicode[STIX]{x1D6FD}+\frac{r}{q}\biggr)e\biggl(-n\biggl(\unicode[STIX]{x1D6FD}+\frac{r}{q}\biggr)\biggr)\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & = & \displaystyle \mathop{\sum }_{\substack{ x,y\in \mathbb{Z} \\ (Lx+1,Ly)=1}}\unicode[STIX]{x1D713}\biggl(\frac{Lx+1}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Ly}{X}\biggr)\mathop{\sum }_{q<Q_{0}}\sum _{r(q)}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}e\biggl(\frac{r}{q}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n)\biggr)\nonumber\\ \displaystyle & & \displaystyle \cdot \,\int _{-\infty }^{\infty }\mathfrak{t}\biggl(\frac{N}{K_{0}}\unicode[STIX]{x1D6FD}\biggr)e(\unicode[STIX]{x1D6FD}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n))\,d\unicode[STIX]{x1D6FD}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{M}}_{N}(n) & = & \displaystyle \int _{0}^{1}\mathfrak{T}(\unicode[STIX]{x1D703})\widehat{{\mathcal{R}}}_{N}(\unicode[STIX]{x1D703})e(-n\unicode[STIX]{x1D703})\,d\unicode[STIX]{x1D703}\nonumber\\ \displaystyle & = & \displaystyle \int _{-\infty }^{\infty }\mathop{\sum }_{q<Q_{0}}\sum _{r(q)}\mathfrak{t}\biggl(\frac{N}{K_{0}}\unicode[STIX]{x1D6FD}\biggr)\widehat{{\mathcal{R}}}_{N}\biggl(\unicode[STIX]{x1D6FD}+\frac{r}{q}\biggr)e\biggl(-n\biggl(\unicode[STIX]{x1D6FD}+\frac{r}{q}\biggr)\biggr)\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & = & \displaystyle \mathop{\sum }_{\substack{ x,y\in \mathbb{Z} \\ (Lx+1,Ly)=1}}\unicode[STIX]{x1D713}\biggl(\frac{Lx+1}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Ly}{X}\biggr)\mathop{\sum }_{q<Q_{0}}\sum _{r(q)}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}e\biggl(\frac{r}{q}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n)\biggr)\nonumber\\ \displaystyle & & \displaystyle \cdot \,\int _{-\infty }^{\infty }\mathfrak{t}\biggl(\frac{N}{K_{0}}\unicode[STIX]{x1D6FD}\biggr)e(\unicode[STIX]{x1D6FD}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n))\,d\unicode[STIX]{x1D6FD}.\end{eqnarray}$$
Now we decompose the set
![]() $\mathfrak{F}$
as the intersection of
$\mathfrak{F}$
as the intersection of
![]() $\mathfrak{F}$
and the left cosets of
$\mathfrak{F}$
and the left cosets of
and apply Lemma 5.2 with
![]() $K=K_{0}$
to obtain
$K=K_{0}$
to obtain
 $$\begin{eqnarray}\displaystyle & & \displaystyle \mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}}e\biggl(\frac{r}{q}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n)\biggr)\cdot \int _{-\infty }^{\infty }\mathfrak{t}\biggl(\frac{N}{K_{0}}\unicode[STIX]{x1D6FD}\biggr)e(\unicode[STIX]{x1D6FD}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n))\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & & \displaystyle \quad =\mathop{\sum }_{\unicode[STIX]{x1D6FE}_{0}\in {\mathcal{A}}/{\mathcal{A}}(q)}e\biggl(\frac{r}{q}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}_{0}}(Lx+1,Ly)-n)\biggr)\int _{-\infty }^{\infty }\mathfrak{t}\biggl(\frac{N}{K_{0}}\unicode[STIX]{x1D6FD}\biggr)\mathop{\sum }_{\unicode[STIX]{x1D6FE}\equiv \unicode[STIX]{x1D6FE}_{0}(q)}e(\unicode[STIX]{x1D6FD}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n))\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & & \displaystyle \quad =i_{q}^{{\mathcal{A}}}\cdot \mathop{\sum }_{\unicode[STIX]{x1D6FE}_{0}\in {\mathcal{A}}/{\mathcal{A}}(q)}e\biggl(\frac{r}{q}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}_{0}}(Lx+1,Ly)-n)\biggr)\int _{-\infty }^{\infty }\mathfrak{t}\biggl(\frac{N}{K_{0}}\unicode[STIX]{x1D6FD}\biggr)\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}e(\unicode[STIX]{x1D6FD}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n))\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & & \displaystyle \qquad +\,O\biggl(\frac{T^{2\unicode[STIX]{x1D6E9}}K_{0}^{2}Q_{0}^{6}}{N}\biggr)\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{K_{0}}{N}\cdot \frac{1}{[{\mathcal{A}}:{\mathcal{A}}(q)]}\mathop{\sum }_{\unicode[STIX]{x1D6FE}_{0}\in {\mathcal{A}}/{\mathcal{A}}(q)}e\biggl(\frac{r}{q}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}_{0}}(Lx+1,Ly)-n)\biggr)\cdot \mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\hat{\mathfrak{t}}\biggl(\frac{K_{0}}{N}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n)\biggr)\nonumber\\ \displaystyle & & \displaystyle \qquad +\,O\biggl(\frac{T^{2\unicode[STIX]{x1D6E9}}K_{0}^{2}Q_{0}^{6}}{N}\biggr),\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}}e\biggl(\frac{r}{q}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n)\biggr)\cdot \int _{-\infty }^{\infty }\mathfrak{t}\biggl(\frac{N}{K_{0}}\unicode[STIX]{x1D6FD}\biggr)e(\unicode[STIX]{x1D6FD}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n))\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & & \displaystyle \quad =\mathop{\sum }_{\unicode[STIX]{x1D6FE}_{0}\in {\mathcal{A}}/{\mathcal{A}}(q)}e\biggl(\frac{r}{q}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}_{0}}(Lx+1,Ly)-n)\biggr)\int _{-\infty }^{\infty }\mathfrak{t}\biggl(\frac{N}{K_{0}}\unicode[STIX]{x1D6FD}\biggr)\mathop{\sum }_{\unicode[STIX]{x1D6FE}\equiv \unicode[STIX]{x1D6FE}_{0}(q)}e(\unicode[STIX]{x1D6FD}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n))\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & & \displaystyle \quad =i_{q}^{{\mathcal{A}}}\cdot \mathop{\sum }_{\unicode[STIX]{x1D6FE}_{0}\in {\mathcal{A}}/{\mathcal{A}}(q)}e\biggl(\frac{r}{q}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}_{0}}(Lx+1,Ly)-n)\biggr)\int _{-\infty }^{\infty }\mathfrak{t}\biggl(\frac{N}{K_{0}}\unicode[STIX]{x1D6FD}\biggr)\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}e(\unicode[STIX]{x1D6FD}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n))\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & & \displaystyle \qquad +\,O\biggl(\frac{T^{2\unicode[STIX]{x1D6E9}}K_{0}^{2}Q_{0}^{6}}{N}\biggr)\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{K_{0}}{N}\cdot \frac{1}{[{\mathcal{A}}:{\mathcal{A}}(q)]}\mathop{\sum }_{\unicode[STIX]{x1D6FE}_{0}\in {\mathcal{A}}/{\mathcal{A}}(q)}e\biggl(\frac{r}{q}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}_{0}}(Lx+1,Ly)-n)\biggr)\cdot \mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\hat{\mathfrak{t}}\biggl(\frac{K_{0}}{N}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n)\biggr)\nonumber\\ \displaystyle & & \displaystyle \qquad +\,O\biggl(\frac{T^{2\unicode[STIX]{x1D6E9}}K_{0}^{2}Q_{0}^{6}}{N}\biggr),\end{eqnarray}$$
where Lemma 5.2 is applied to obtain the third line above and
![]() $i_{q}^{{\mathcal{A}}}=1/[{\mathcal{A}}:{\mathcal{A}}(q)]$
. Inserting (6.2) into (6.1), we get
$i_{q}^{{\mathcal{A}}}=1/[{\mathcal{A}}:{\mathcal{A}}(q)]$
. Inserting (6.2) into (6.1), we get
 $$\begin{eqnarray}{\mathcal{M}}_{N}(n)=\mathop{\sum }_{\substack{ x,y\in \mathbb{Z} \\ (Lx+1,Ly)=1}}\unicode[STIX]{x1D713}\biggl(\frac{Lx+1}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Ly}{X}\biggr)\mathfrak{S}_{Q_{0}}(n)\mathfrak{M}(n)+O\biggl(\frac{T^{2\unicode[STIX]{x1D6E9}}X^{2}K_{0}^{2}Q_{0}^{8}}{N}\biggr),\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{M}}_{N}(n)=\mathop{\sum }_{\substack{ x,y\in \mathbb{Z} \\ (Lx+1,Ly)=1}}\unicode[STIX]{x1D713}\biggl(\frac{Lx+1}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Ly}{X}\biggr)\mathfrak{S}_{Q_{0}}(n)\mathfrak{M}(n)+O\biggl(\frac{T^{2\unicode[STIX]{x1D6E9}}X^{2}K_{0}^{2}Q_{0}^{8}}{N}\biggr),\end{eqnarray}$$
where
and
Here
![]() $c_{q}$
is the Ramanujan sum defined by
$c_{q}$
is the Ramanujan sum defined by
Fixing
![]() $n$
, we have that
$n$
, we have that
![]() $c_{q}(n)$
is multiplicative with respect to
$c_{q}(n)$
is multiplicative with respect to
![]() $q$
, and locally,
$q$
, and locally,
 $$\begin{eqnarray}c_{p^{k}}(n)=\left\{\begin{array}{@{}ll@{}}0\quad & \text{if }p^{m}\Vert n,m\leqslant k-2,\\ -p^{k-1}\quad & \text{if }p^{k-1}\Vert n,\\ p^{k-1}(p-1)\quad & \text{if }p^{k}|n.\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}c_{p^{k}}(n)=\left\{\begin{array}{@{}ll@{}}0\quad & \text{if }p^{m}\Vert n,m\leqslant k-2,\\ -p^{k-1}\quad & \text{if }p^{k-1}\Vert n,\\ p^{k-1}(p-1)\quad & \text{if }p^{k}|n.\end{array}\right.\end{eqnarray}$$
The error term in (6.3) is
![]() $O(T^{2\unicode[STIX]{x1D6FF}-2-\unicode[STIX]{x1D716}})$
by our choice of
$O(T^{2\unicode[STIX]{x1D6FF}-2-\unicode[STIX]{x1D716}})$
by our choice of
![]() $K_{0}$
(see (4.1) and (5.1)), where
$K_{0}$
(see (4.1) and (5.1)), where
![]() $\unicode[STIX]{x1D716}$
is any small positive number at most
$\unicode[STIX]{x1D716}$
is any small positive number at most
![]() $\frac{33}{20}(\unicode[STIX]{x1D6FF}-\unicode[STIX]{x1D6E9})>0$
. Applying Lemma 5.3 with
$\frac{33}{20}(\unicode[STIX]{x1D6FF}-\unicode[STIX]{x1D6E9})>0$
. Applying Lemma 5.3 with
![]() $K=K_{0}$
, we can give a lower bound for the Archimedean piece
$K=K_{0}$
, we can give a lower bound for the Archimedean piece
![]() $\mathfrak{M}(n)$
for any
$\mathfrak{M}(n)$
for any
![]() $N/2\leqslant n\leqslant N$
:
$N/2\leqslant n\leqslant N$
:
Therefore, Theorem 4.4 is proved once we show that
![]() $\mathfrak{S}_{Q_{0}}(n)\gg 1$
for every
$\mathfrak{S}_{Q_{0}}(n)\gg 1$
for every
![]() $n$
admissible (or, what actually suffices, once we have proven it up to log factors, since our aim is to get a power saving, which absorbs all log powers). The rest of this section is devoted to proving the following proposition.
$n$
admissible (or, what actually suffices, once we have proven it up to log factors, since our aim is to get a power saving, which absorbs all log powers). The rest of this section is devoted to proving the following proposition.
Proposition 6.1. We have
![]() $\mathfrak{S}_{Q_{0}}(n)\gg 1/(\text{log}\;n)$
if
$\mathfrak{S}_{Q_{0}}(n)\gg 1/(\text{log}\;n)$
if
![]() $n$
is admissible, and
$n$
is admissible, and
![]() $\mathfrak{S}_{Q_{0}}(n)\ll (\log n)/Q_{0}$
if
$\mathfrak{S}_{Q_{0}}(n)\ll (\log n)/Q_{0}$
if
![]() $n$
is not admissible.
$n$
is not admissible.
To understand
![]() $\mathfrak{S}_{Q_{0}}(n)$
, we first push
$\mathfrak{S}_{Q_{0}}(n)$
, we first push
![]() $Q_{0}$
to
$Q_{0}$
to
![]() $\infty$
. We define a formal singular series
$\infty$
. We define a formal singular series
where
So to understand
![]() $\mathfrak{S}_{Q_{0}}(n)$
or
$\mathfrak{S}_{Q_{0}}(n)$
or
![]() $\mathfrak{S}(n)$
one must understand
$\mathfrak{S}(n)$
one must understand
![]() $B_{q}(n)$
for each
$B_{q}(n)$
for each
![]() $q$
.
$q$
.
We rewrite
![]() $B_{q}(n)$
as
$B_{q}(n)$
as
where
The term
![]() $\unicode[STIX]{x1D70F}_{q}(r)$
can be viewed as the probability that a curvature is congruent to
$\unicode[STIX]{x1D70F}_{q}(r)$
can be viewed as the probability that a curvature is congruent to
![]() $r\;\text{mod}\;q$
, as
$r\;\text{mod}\;q$
, as
![]() $\unicode[STIX]{x1D6FE}$
ranges over
$\unicode[STIX]{x1D6FE}$
ranges over
![]() ${\mathcal{A}}$
. To get from (6.12) to (6.13) we used the fact that
${\mathcal{A}}$
. To get from (6.12) to (6.13) we used the fact that
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)=\unicode[STIX]{x1D705}(M\unicode[STIX]{x1D6FE}w_{x,y}(\mathbb{R}+\sqrt{\unicode[STIX]{x1D6E5}}/2))$
for some
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)=\unicode[STIX]{x1D705}(M\unicode[STIX]{x1D6FE}w_{x,y}(\mathbb{R}+\sqrt{\unicode[STIX]{x1D6E5}}/2))$
for some
![]() $w_{x,y}\in \unicode[STIX]{x1D6E4}(L)$
with left column
$w_{x,y}\in \unicode[STIX]{x1D6E4}(L)$
with left column
![]() $(Lx+1,Ly)^{T}$
.
$(Lx+1,Ly)^{T}$
.
First we need the multiplicativity of
![]() ${\mathcal{A}}$
which will lead to the multiplicativity of
${\mathcal{A}}$
which will lead to the multiplicativity of
![]() $B_{q}(n)$
.
$B_{q}(n)$
.
Lemma 6.2. Write
![]() $q=\prod _{i}p_{i}^{n_{i}}$
, then
$q=\prod _{i}p_{i}^{n_{i}}$
, then
Lemma 6.2 will lead immediately to the multiplicativity of
![]() $B_{q}(n)$
with respect to
$B_{q}(n)$
with respect to
![]() $q$
. A priori Lemma 6.2 is not true for a general group
$q$
. A priori Lemma 6.2 is not true for a general group
![]() ${\mathcal{A}}$
. If this is the case, we replace
${\mathcal{A}}$
. If this is the case, we replace
![]() ${\mathcal{A}}$
by some congruence subgroup of
${\mathcal{A}}$
by some congruence subgroup of
![]() ${\mathcal{A}}$
which satisfies the multiplicative property (such a subgroup exists by strong approximation in
${\mathcal{A}}$
which satisfies the multiplicative property (such a subgroup exists by strong approximation in
![]() $\text{SL}_{2}$
). As noted in § 2, we may move to a finite-index subgroup without loss of generality.
$\text{SL}_{2}$
). As noted in § 2, we may move to a finite-index subgroup without loss of generality.
Given this multiplicativity, we split (6.9) into an Euler product
The arithmetic meaning of each factor of the Euler product is illustrated by the following formula:
To see this, let
![]() $s_{\unicode[STIX]{x1D6FE}}$
be such that
$s_{\unicode[STIX]{x1D6FE}}$
be such that
![]() $p^{s_{\unicode[STIX]{x1D6FE}}}\Vert \mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n$
. Then,
$p^{s_{\unicode[STIX]{x1D6FE}}}\Vert \mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n$
. Then,
 $$\begin{eqnarray}\displaystyle & & \displaystyle 1+\mathop{\sum }_{m=1}^{k}B_{p^{m}}(n)\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{[{\mathcal{A}}:{\mathcal{A}}(p^{k})]}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in {\mathcal{A}}/{\mathcal{A}}(p^{k})}\biggl(1+\mathop{\sum }_{m=1}^{k}c_{p^{m}}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n)\biggr)\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{[{\mathcal{A}}:{\mathcal{A}}(p^{k})]}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in {\mathcal{A}}/{\mathcal{A}}(p^{k})}\left\{\begin{array}{@{}ll@{}}0 & s_{\unicode[STIX]{x1D6FE}}<k,\\ p^{k} & s_{\unicode[STIX]{x1D6FE}}\geqslant k.\end{array}\right.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle 1+\mathop{\sum }_{m=1}^{k}B_{p^{m}}(n)\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{[{\mathcal{A}}:{\mathcal{A}}(p^{k})]}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in {\mathcal{A}}/{\mathcal{A}}(p^{k})}\biggl(1+\mathop{\sum }_{m=1}^{k}c_{p^{m}}(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lx+1,Ly)-n)\biggr)\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{[{\mathcal{A}}:{\mathcal{A}}(p^{k})]}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in {\mathcal{A}}/{\mathcal{A}}(p^{k})}\left\{\begin{array}{@{}ll@{}}0 & s_{\unicode[STIX]{x1D6FE}}<k,\\ p^{k} & s_{\unicode[STIX]{x1D6FE}}\geqslant k.\end{array}\right.\nonumber\end{eqnarray}$$
Therefore,
![]() $1+B_{p}(n)+B_{p^{2}}(n)+\cdots +B_{p^{k}}(n)$
is non-zero if and only if
$1+B_{p}(n)+B_{p^{2}}(n)+\cdots +B_{p^{k}}(n)$
is non-zero if and only if
![]() $n$
is represented
$n$
is represented
![]() $(\text{mod}\;p^{k})$
.
$(\text{mod}\;p^{k})$
.
Our goal for the rest of the section is to access
![]() $\mathfrak{S}(n)$
(and prove Proposition 6.1) by analyzing the values of
$\mathfrak{S}(n)$
(and prove Proposition 6.1) by analyzing the values of
![]() $B_{p^{k}}(n)$
. First, we will show the following lemma.
$B_{p^{k}}(n)$
. First, we will show the following lemma.
Lemma 6.3. There is an integer
![]() $P_{\text{bad}}\geqslant 1$
such that:
$P_{\text{bad}}\geqslant 1$
such that:
(i) for any
 $p\nmid P_{\text{bad}}$
and
$p\nmid P_{\text{bad}}$
and
 $k\geqslant 2$
,
$k\geqslant 2$
,
 $B_{p^{k}}(n)=0$
;
$B_{p^{k}}(n)=0$
;(ii) for each of the finitely many primes
 $p\mid P_{\text{bad}}$
,
$p\mid P_{\text{bad}}$
,
 $\exists k_{p}^{\prime }$
such that
$\exists k_{p}^{\prime }$
such that
 $B_{p^{k}}(n)=0$
for any
$B_{p^{k}}(n)=0$
for any
 $k\geqslant k_{p}^{\prime }$
.
$k\geqslant k_{p}^{\prime }$
.
Indeed, Lemma 6.3 follows from the following fact for
![]() ${\mathcal{A}}(q)$
given by strong approximation in
${\mathcal{A}}(q)$
given by strong approximation in
![]() $\text{SL}_{2}$
.
$\text{SL}_{2}$
.
Lemma 6.4. There is an integer
![]() $L_{1}\geqslant 1$
such that:
$L_{1}\geqslant 1$
such that:
(i) for any
 $p\nmid L_{1}$
and
$p\nmid L_{1}$
and
 $k\geqslant 1$
, (6.16)
$k\geqslant 1$
, (6.16) $$\begin{eqnarray}{\mathcal{A}}(p^{k-1})/{\mathcal{A}}(p^{k})=\text{SL}_{2}({\mathcal{O}}_{K})(p^{k-1})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{k});\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{A}}(p^{k-1})/{\mathcal{A}}(p^{k})=\text{SL}_{2}({\mathcal{O}}_{K})(p^{k-1})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{k});\end{eqnarray}$$
(ii) for each of the finitely many primes
 $p\mid L_{1}$
,
$p\mid L_{1}$
,
 $\exists k_{p}$
such that (6.16) holds for any
$\exists k_{p}$
such that (6.16) holds for any
 $k\geqslant k_{p}$
.
$k\geqslant k_{p}$
.
We refer to primes that divide
![]() $P_{\text{bad}}$
as bad primes, and those that do not divide
$P_{\text{bad}}$
as bad primes, and those that do not divide
![]() $P_{\text{bad}}$
as good primes. We have given an explicit form of Lemma 6.4 in Theorem 8.1, which allows the computation of a valid
$P_{\text{bad}}$
as good primes. We have given an explicit form of Lemma 6.4 in Theorem 8.1, which allows the computation of a valid
![]() $L_{1}$
. We use a Hensel lifting argument to deduce Lemma 6.3 from Lemma 6.4.
$L_{1}$
. We use a Hensel lifting argument to deduce Lemma 6.3 from Lemma 6.4.
Proof of Lemma 6.3.
First we rewrite
![]() $B_{p^{k}}(n)$
:
$B_{p^{k}}(n)$
:
 $$\begin{eqnarray}\displaystyle B_{p^{k}}(n) & = & \displaystyle \frac{1}{[{\mathcal{A}}:{\mathcal{A}}(p^{k})]}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in {\mathcal{A}}/{\mathcal{A}}(p^{k})}c_{p^{k}}(F_{1}(\unicode[STIX]{x1D6FE})-n)\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{[{\mathcal{A}}:{\mathcal{A}}(p^{k})]}\mathop{\sum }_{\unicode[STIX]{x1D6FE}_{0}\in {\mathcal{A}}/{\mathcal{A}}(p^{k-1})}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}\in {\mathcal{A}}/{\mathcal{A}}(p^{k}) \\ \unicode[STIX]{x1D6FE}\equiv \unicode[STIX]{x1D6FE}_{0}(p^{k-1})}}c_{p^{k}}(F_{1}(\unicode[STIX]{x1D6FE})-n),\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle B_{p^{k}}(n) & = & \displaystyle \frac{1}{[{\mathcal{A}}:{\mathcal{A}}(p^{k})]}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in {\mathcal{A}}/{\mathcal{A}}(p^{k})}c_{p^{k}}(F_{1}(\unicode[STIX]{x1D6FE})-n)\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{[{\mathcal{A}}:{\mathcal{A}}(p^{k})]}\mathop{\sum }_{\unicode[STIX]{x1D6FE}_{0}\in {\mathcal{A}}/{\mathcal{A}}(p^{k-1})}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}\in {\mathcal{A}}/{\mathcal{A}}(p^{k}) \\ \unicode[STIX]{x1D6FE}\equiv \unicode[STIX]{x1D6FE}_{0}(p^{k-1})}}c_{p^{k}}(F_{1}(\unicode[STIX]{x1D6FE})-n),\end{eqnarray}$$
where
![]() $F_{1}(\unicode[STIX]{x1D6FE})=\unicode[STIX]{x1D705}(M\unicode[STIX]{x1D6FE}(\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2))$
and we view
$F_{1}(\unicode[STIX]{x1D6FE})=\unicode[STIX]{x1D705}(M\unicode[STIX]{x1D6FE}(\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2))$
and we view
![]() $F_{1}$
as an algebraic function over the real and imaginary parts of the entries of
$F_{1}$
as an algebraic function over the real and imaginary parts of the entries of
![]() $\unicode[STIX]{x1D6FE}$
. As we have assumed
$\unicode[STIX]{x1D6FE}$
. As we have assumed
![]() ${\mathcal{A}}\subset \operatorname{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
in § 2, we may write
${\mathcal{A}}\subset \operatorname{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
in § 2, we may write
We assume for the moment that
![]() $M$
is also in
$M$
is also in
![]() $\operatorname{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
, and write
$\operatorname{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
, and write
Then

As
![]() $\unicode[STIX]{x1D6FE}\in \text{PSL}_{2}({\mathcal{O}}_{K})$
, these variables are also subject to the following conditions:
$\unicode[STIX]{x1D6FE}\in \text{PSL}_{2}({\mathcal{O}}_{K})$
, these variables are also subject to the following conditions:
If
![]() $M$
is integral, one can check that the Jacobian matrix
$M$
is integral, one can check that the Jacobian matrix
maps
![]() $\mathbb{Z}_{p}^{8}$
onto
$\mathbb{Z}_{p}^{8}$
onto
![]() $\mathbb{Z}_{p}^{3}$
as a linear transformation, at each point of the affine variety
$\mathbb{Z}_{p}^{3}$
as a linear transformation, at each point of the affine variety
![]() $\mathbb{V}[\mathbb{Q}_{p}]$
defined by the following equations:
$\mathbb{V}[\mathbb{Q}_{p}]$
defined by the following equations:
 $$\begin{eqnarray}\left\{\begin{array}{@{}l@{}}F_{1}=n,\quad \\ F_{2}=0,\quad \\ F_{3}=0.\quad \end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\left\{\begin{array}{@{}l@{}}F_{1}=n,\quad \\ F_{2}=0,\quad \\ F_{3}=0.\quad \end{array}\right.\end{eqnarray}$$
For any
![]() $k\geqslant k_{p}$
(
$k\geqslant k_{p}$
(
![]() $k_{p}$
can be taken to be 2 if
$k_{p}$
can be taken to be 2 if
![]() $p$
is good), the lifting
$p$
is good), the lifting
![]() $\mathbb{V}[\mathbb{Z}/p^{k-1}\mathbb{Z}]\rightarrow \mathbb{V}[\mathbb{Z}/p^{k}\mathbb{Z}]$
becomes regular by Lemma 6.4, and for
$\mathbb{V}[\mathbb{Z}/p^{k-1}\mathbb{Z}]\rightarrow \mathbb{V}[\mathbb{Z}/p^{k}\mathbb{Z}]$
becomes regular by Lemma 6.4, and for
![]() $\unicode[STIX]{x1D6FE}_{0}$
such that
$\unicode[STIX]{x1D6FE}_{0}$
such that
![]() $F_{1}(\unicode[STIX]{x1D6FE}_{0})\equiv n\;(\text{mod}\;p^{k-1})$
, this gives
$F_{1}(\unicode[STIX]{x1D6FE}_{0})\equiv n\;(\text{mod}\;p^{k-1})$
, this gives
Returning to (6.17), from (6.7) the innermost sum of (6.17) is zero unless
![]() $F_{1}(\unicode[STIX]{x1D6FE}_{0})\equiv n\;(\text{mod}\;p^{k-1})$
. However, in this case, if
$F_{1}(\unicode[STIX]{x1D6FE}_{0})\equiv n\;(\text{mod}\;p^{k-1})$
. However, in this case, if
![]() $k\geqslant k_{p}$
, by (6.20) (at
$k\geqslant k_{p}$
, by (6.20) (at
![]() $k$
and
$k$
and
![]() $k+1$
) and (6.7), each innermost sum of (6.17) is still zero. Therefore
$k+1$
) and (6.7), each innermost sum of (6.17) is still zero. Therefore
![]() $B_{p^{k}}(n)=0$
, for
$B_{p^{k}}(n)=0$
, for
![]() $k\geqslant k_{p}$
.
$k\geqslant k_{p}$
.
Above, we assumed
![]() $M\in \operatorname{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
, and we found that the
$M\in \operatorname{PSL}_{2}(\mathbb{Z}[\sqrt{-d}])$
, and we found that the
![]() $k_{p}^{\prime }$
of Lemma 6.3 agree with the
$k_{p}^{\prime }$
of Lemma 6.3 agree with the
![]() $k_{p}$
of Lemma 6.4. If instead
$k_{p}$
of Lemma 6.4. If instead
![]() $M$
is fractional in the sense that the
$M$
is fractional in the sense that the
![]() $M_{ij}$
have denominator
$M_{ij}$
have denominator
![]() $q_{0}$
, then we need to multiply
$q_{0}$
, then we need to multiply
![]() $F_{1}$
by
$F_{1}$
by
![]() $q_{0}^{2}$
to make it integral. For any
$q_{0}^{2}$
to make it integral. For any
![]() $p^{n_{p}}||q_{0}$
, one can check that
$p^{n_{p}}||q_{0}$
, one can check that
![]() $\mathbb{Z}_{p}^{3}\subset (1/p^{2n_{p}})J(\mathbb{Z}_{p}^{8})$
. In this case,
$\mathbb{Z}_{p}^{3}\subset (1/p^{2n_{p}})J(\mathbb{Z}_{p}^{8})$
. In this case,
![]() $B_{p^{k}}(n)=0$
for
$B_{p^{k}}(n)=0$
for
![]() $k\geqslant k_{p}+2n_{p}$
.◻
$k\geqslant k_{p}+2n_{p}$
.◻
The obstruction number
![]() $L_{0}$
in the statement of Corollary 1.4 is thus given by
$L_{0}$
in the statement of Corollary 1.4 is thus given by
The computation of upper bounds on
![]() $k_{p}$
is given by Theorem 8.1, and some examples are given in § 9.
$k_{p}$
is given by Theorem 8.1, and some examples are given in § 9.
At this point, in order to prove Proposition 6.1, as there are only finitely many bad primes, we have shown that it suffices to analyze the contribution of
![]() $1+B_{p}(n)$
for good odd primes
$1+B_{p}(n)$
for good odd primes
![]() $p$
.
$p$
.
Lemma 6.5. Suppose
![]() $n$
is admissible. Let
$n$
is admissible. Let
![]() $p$
be an odd prime not dividing
$p$
be an odd prime not dividing
![]() $P_{\text{bad}}$
. Then
$P_{\text{bad}}$
. Then
![]() $B_{p}(n)=O(1/p^{2})$
if
$B_{p}(n)=O(1/p^{2})$
if
![]() $p\nmid n$
and
$p\nmid n$
and
![]() $B_{p}(n)=O(1/p)$
if
$B_{p}(n)=O(1/p)$
if
![]() $p\mid n$
, where the implied constants are independent of
$p\mid n$
, where the implied constants are independent of
![]() $n$
.
$n$
.
To prove this, we first prove the following result.
Lemma 6.6. Let
![]() $p$
be an odd prime not dividing
$p$
be an odd prime not dividing
![]() $P_{\text{bad}}$
. Then
$P_{\text{bad}}$
. Then
 $$\begin{eqnarray}\unicode[STIX]{x1D70F}_{p}(n)=\left\{\begin{array}{@{}ll@{}}\displaystyle \frac{1}{p}+O\biggl(\frac{1}{p^{3}}\biggr) & \text{if }n\not \equiv 0\;(\text{mod}\;p),\\ \displaystyle \frac{1}{p}+O\biggl(\frac{1}{p^{2}}\biggr) & \text{if }n\equiv 0\;(\text{mod}\;p),\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70F}_{p}(n)=\left\{\begin{array}{@{}ll@{}}\displaystyle \frac{1}{p}+O\biggl(\frac{1}{p^{3}}\biggr) & \text{if }n\not \equiv 0\;(\text{mod}\;p),\\ \displaystyle \frac{1}{p}+O\biggl(\frac{1}{p^{2}}\biggr) & \text{if }n\equiv 0\;(\text{mod}\;p),\end{array}\right.\end{eqnarray}$$
where the implied constants are independent of
![]() $n$
.
$n$
.
There are at least two proofs of this fact. One is the proof we give below, which works directly in the group
![]() $\text{SL}_{2}({\mathcal{O}}_{K})$
. Another approach is to consider the image under the spin homomorphism
$\text{SL}_{2}({\mathcal{O}}_{K})$
. Another approach is to consider the image under the spin homomorphism
![]() $\unicode[STIX]{x1D70C}$
of
$\unicode[STIX]{x1D70C}$
of
![]() ${\mathcal{A}}$
in
${\mathcal{A}}$
in
![]() $\text{O}_{Q}(\mathbb{Z})$
where
$\text{O}_{Q}(\mathbb{Z})$
where
![]() $Q(x_{1},x_{2},x_{3},x_{4})=x_{2}^{2}+dx_{3}^{2}+x_{1}x_{4}$
, and note that the set of curvatures we are interested in is, up to a factor of
$Q(x_{1},x_{2},x_{3},x_{4})=x_{2}^{2}+dx_{3}^{2}+x_{1}x_{4}$
, and note that the set of curvatures we are interested in is, up to a factor of
![]() $d$
, exactly the set of fourth coordinates of points in the orbit
$d$
, exactly the set of fourth coordinates of points in the orbit
![]() $\unicode[STIX]{x1D70C}({\mathcal{A}})v^{T}$
, where
$\unicode[STIX]{x1D70C}({\mathcal{A}})v^{T}$
, where
![]() $v=(-d,0,1,0)$
. By strong approximation, modulo
$v=(-d,0,1,0)$
. By strong approximation, modulo
![]() $p$
the orbit
$p$
the orbit
![]() $\unicode[STIX]{x1D70C}({\mathcal{A}})v^{T}$
is simply the set of all solutions to
$\unicode[STIX]{x1D70C}({\mathcal{A}})v^{T}$
is simply the set of all solutions to
![]() $Q(x_{1},x_{2},x_{3},x_{4})\equiv d\;(\text{mod}\;p)$
, and
$Q(x_{1},x_{2},x_{3},x_{4})\equiv d\;(\text{mod}\;p)$
, and
![]() $\unicode[STIX]{x1D70F}_{p}(n)$
is easily computed by counting representations modulo
$\unicode[STIX]{x1D70F}_{p}(n)$
is easily computed by counting representations modulo
![]() $p$
of
$p$
of
![]() $d$
by specific quadratic forms. This passing between
$d$
by specific quadratic forms. This passing between
![]() $\text{SL}_{2}(\mathbb{C})$
and
$\text{SL}_{2}(\mathbb{C})$
and
![]() $\text{O}_{\mathbb{R}}(3,1)$
is a nod to the description of Apollonian circle packings in [Reference Bourgain and KontorovichBK14a, Reference Graham, Lagarias, Mallows, Wilks and YanGLMWY03, Reference ZhangZha18b], where curvatures can be seen by looking at orbits of certain thin subgroups of
$\text{O}_{\mathbb{R}}(3,1)$
is a nod to the description of Apollonian circle packings in [Reference Bourgain and KontorovichBK14a, Reference Graham, Lagarias, Mallows, Wilks and YanGLMWY03, Reference ZhangZha18b], where curvatures can be seen by looking at orbits of certain thin subgroups of
![]() $\text{O}_{F}(\mathbb{Z})$
as described in the introduction. Since we describe Apollonian packings somewhat more geometrically, we present the proof from that point of view.
$\text{O}_{F}(\mathbb{Z})$
as described in the introduction. Since we describe Apollonian packings somewhat more geometrically, we present the proof from that point of view.
Proof. Let
![]() $p$
be an odd prime not dividing
$p$
be an odd prime not dividing
![]() $P_{\text{bad}}$
. Let
$P_{\text{bad}}$
. Let
![]() $\unicode[STIX]{x1D6FE}\in {\mathcal{A}}$
. Write
$\unicode[STIX]{x1D6FE}\in {\mathcal{A}}$
. Write
![]() $\unicode[STIX]{x1D6FE}_{p}$
for the reduction of
$\unicode[STIX]{x1D6FE}_{p}$
for the reduction of
![]() $\unicode[STIX]{x1D6FE}$
in
$\unicode[STIX]{x1D6FE}$
in
![]() ${\mathcal{A}}/{\mathcal{A}}(p)$
. By Lemma 6.4, we have that
${\mathcal{A}}/{\mathcal{A}}(p)$
. By Lemma 6.4, we have that
![]() $\unicode[STIX]{x1D6FE}_{p}$
ranges over all of
$\unicode[STIX]{x1D6FE}_{p}$
ranges over all of
Therefore, we have
For any commutative ring
![]() $R$
with identity, the allowable first columns of
$R$
with identity, the allowable first columns of
![]() $\text{SL}_{2}(R)$
form a set
$\text{SL}_{2}(R)$
form a set
We have that
![]() $\#U(R)=\#\mathbb{P}^{1}(R)\cdot \#R^{\ast }$
. Furthermore,
$\#U(R)=\#\mathbb{P}^{1}(R)\cdot \#R^{\ast }$
. Furthermore,
where we write
![]() $\operatorname{Stab}_{\ast }$
for the stabilizer of any one element of
$\operatorname{Stab}_{\ast }$
for the stabilizer of any one element of
![]() $U$
. In our case,
$U$
. In our case,
![]() $R=\mathbb{Z}[\sqrt{-d}]/(p)$
, this implies
$R=\mathbb{Z}[\sqrt{-d}]/(p)$
, this implies
We have
 $$\begin{eqnarray}\#\mathbb{P}^{1}(\mathbb{Z}[\sqrt{-d}]/(p))=\left\{\begin{array}{@{}ll@{}}\displaystyle p^{2}+1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1,\\ p^{2}+2p+1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=1,\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\#\mathbb{P}^{1}(\mathbb{Z}[\sqrt{-d}]/(p))=\left\{\begin{array}{@{}ll@{}}\displaystyle p^{2}+1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1,\\ p^{2}+2p+1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=1,\end{array}\right.\end{eqnarray}$$
and
 $$\begin{eqnarray}\#(\mathbb{Z}[\sqrt{-d}]/(p))^{\ast }=\left\{\begin{array}{@{}ll@{}}\displaystyle p^{2}-1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1,\\ \displaystyle p^{2}-2p+1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=1.\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\#(\mathbb{Z}[\sqrt{-d}]/(p))^{\ast }=\left\{\begin{array}{@{}ll@{}}\displaystyle p^{2}-1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1,\\ \displaystyle p^{2}-2p+1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=1.\end{array}\right.\end{eqnarray}$$
It remains to compute
![]() $\#\mathbb{V}[\mathbb{Z}/p\mathbb{Z}]$
. But we have
$\#\mathbb{V}[\mathbb{Z}/p\mathbb{Z}]$
. But we have
 $$\begin{eqnarray}\displaystyle \#\mathbb{V}[\mathbb{Z}/p\mathbb{Z}] & = & \displaystyle \#\{\unicode[STIX]{x1D706}\in \text{SL}_{2}(\mathbb{Z}[\sqrt{-d}]/(p)):F_{1}(\unicode[STIX]{x1D706})=n\}\nonumber\\ \displaystyle & = & \displaystyle \#\{v\in U(\mathbb{Z}[\sqrt{-d}]/(p)):F_{1}(v)=n\}\cdot \#\operatorname{Stab}_{\ast }(\text{SL}_{2}(\mathbb{Z}[\sqrt{-d}]/(p)))\nonumber\\ \displaystyle & = & \displaystyle p^{2}\#\{v\in U(\mathbb{Z}[\sqrt{-d}]/(p)):F_{1}(v)=n\}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \#\mathbb{V}[\mathbb{Z}/p\mathbb{Z}] & = & \displaystyle \#\{\unicode[STIX]{x1D706}\in \text{SL}_{2}(\mathbb{Z}[\sqrt{-d}]/(p)):F_{1}(\unicode[STIX]{x1D706})=n\}\nonumber\\ \displaystyle & = & \displaystyle \#\{v\in U(\mathbb{Z}[\sqrt{-d}]/(p)):F_{1}(v)=n\}\cdot \#\operatorname{Stab}_{\ast }(\text{SL}_{2}(\mathbb{Z}[\sqrt{-d}]/(p)))\nonumber\\ \displaystyle & = & \displaystyle p^{2}\#\{v\in U(\mathbb{Z}[\sqrt{-d}]/(p)):F_{1}(v)=n\}.\nonumber\end{eqnarray}$$
In the above, we use the notation
![]() $F_{1}(v)=F_{1}(\unicode[STIX]{x1D706})$
for any
$F_{1}(v)=F_{1}(\unicode[STIX]{x1D706})$
for any
![]() $\unicode[STIX]{x1D706}$
having bottom row
$\unicode[STIX]{x1D706}$
having bottom row
![]() $v$
(upon which
$v$
(upon which
![]() $F_{1}$
depends exclusively).
$F_{1}$
depends exclusively).
Therefore, it remains to compute
If we assume that
![]() $M=I$
, then we can write the equation
$M=I$
, then we can write the equation
![]() $F_{1}(\unicode[STIX]{x1D706})=F_{1}(v)=n$
explicitly in terms of
$F_{1}(\unicode[STIX]{x1D706})=F_{1}(v)=n$
explicitly in terms of
as
We count the number of solutions by evaluating the following exponential sum:
 $$\begin{eqnarray}\displaystyle & & \displaystyle \frac{1}{p}\mathop{\sum }_{s(p)}\mathop{\sum }_{c_{1},c_{2},d_{1},d_{2}(p)}e_{p}(s(c_{1}^{2}+c_{2}^{2}d+c_{1}d_{2}-c_{2}d_{1}-n))\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{p}\mathop{\sum }_{s(p)}\mathop{\sum }_{c_{1},c_{2},d_{1},d_{2}(p)}e_{p}(s(c_{1}+d_{2}/2)^{2}+sd(c_{2}-d_{1}/2d)^{2}-sd_{2}^{2}/4-sd_{1}^{2}/4d-sn)\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{p}\mathop{\sum }_{s\equiv 0(p)}\cdots +\frac{1}{p}\mathop{\sum }_{s\not \equiv 0(p)}\cdots \nonumber\\ \displaystyle & & \displaystyle \quad =p^{3}+\frac{1}{p}\cdot \mathop{\sum }_{s\neq 0(p)}p^{2}\biggl(\frac{s}{p}\biggr)\biggl(\frac{sd}{p}\biggr)\biggl(\frac{-s}{p}\biggr)\biggl(\frac{-s/d}{p}\biggr)\cdot e_{p}(-sn)\nonumber\\ \displaystyle & & \displaystyle \quad =\left\{\begin{array}{@{}ll@{}}p^{3}+p(p-1) & \text{if }n\equiv 0\;(\text{mod}\;p),\\ p^{3}-p & \text{if }n\not \equiv 0\;(\text{mod}\;p),\end{array}\right.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \frac{1}{p}\mathop{\sum }_{s(p)}\mathop{\sum }_{c_{1},c_{2},d_{1},d_{2}(p)}e_{p}(s(c_{1}^{2}+c_{2}^{2}d+c_{1}d_{2}-c_{2}d_{1}-n))\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{p}\mathop{\sum }_{s(p)}\mathop{\sum }_{c_{1},c_{2},d_{1},d_{2}(p)}e_{p}(s(c_{1}+d_{2}/2)^{2}+sd(c_{2}-d_{1}/2d)^{2}-sd_{2}^{2}/4-sd_{1}^{2}/4d-sn)\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{p}\mathop{\sum }_{s\equiv 0(p)}\cdots +\frac{1}{p}\mathop{\sum }_{s\not \equiv 0(p)}\cdots \nonumber\\ \displaystyle & & \displaystyle \quad =p^{3}+\frac{1}{p}\cdot \mathop{\sum }_{s\neq 0(p)}p^{2}\biggl(\frac{s}{p}\biggr)\biggl(\frac{sd}{p}\biggr)\biggl(\frac{-s}{p}\biggr)\biggl(\frac{-s/d}{p}\biggr)\cdot e_{p}(-sn)\nonumber\\ \displaystyle & & \displaystyle \quad =\left\{\begin{array}{@{}ll@{}}p^{3}+p(p-1) & \text{if }n\equiv 0\;(\text{mod}\;p),\\ p^{3}-p & \text{if }n\not \equiv 0\;(\text{mod}\;p),\end{array}\right.\nonumber\end{eqnarray}$$
where
![]() $(\frac{\cdot }{p})$
is the Legendre symbol and we obtain the second to last step by applying Gauss sums first to
$(\frac{\cdot }{p})$
is the Legendre symbol and we obtain the second to last step by applying Gauss sums first to
![]() $c_{1},c_{2}$
, then to
$c_{1},c_{2}$
, then to
![]() $d_{1},d_{2}$
.
$d_{1},d_{2}$
.
To get
![]() $\#\mathbb{V}[\mathbb{Z}/p\mathbb{Z}]$
we need to subtract the contribution from solutions not in
$\#\mathbb{V}[\mathbb{Z}/p\mathbb{Z}]$
we need to subtract the contribution from solutions not in
![]() $U(\mathbb{Z}[\sqrt{-d}]/(p))$
. It turns out that if
$U(\mathbb{Z}[\sqrt{-d}]/(p))$
. It turns out that if
![]() $n\equiv 0\;(\text{mod}\;p)$
then all such are solutions to (6.25); if
$n\equiv 0\;(\text{mod}\;p)$
then all such are solutions to (6.25); if
![]() $n\not \equiv 0\;(\text{mod}\;p)$
then none such are solutions. We thus arrive at the following result:
$n\not \equiv 0\;(\text{mod}\;p)$
then none such are solutions. We thus arrive at the following result:
 $$\begin{eqnarray}\displaystyle \#V[\mathbb{Z}/p\mathbb{Z}]=p^{2}\cdot \left\{\begin{array}{@{}ll@{}}\displaystyle p^{3}+p(p-1)-1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1\text{ and }n\equiv 0\;(\text{mod}\;p),\\ \displaystyle p^{3}-p & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1\text{ and }n\not \equiv 0\;(\text{mod}\;p),\\ \displaystyle p^{3}-p^{2}-p+1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=1\text{ and }n\equiv 0\;(\text{mod}\;p),\\ \displaystyle p^{3}-p & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=1\text{ and }n\not \equiv 0\;(\text{mod}\;p).\end{array}\right. & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \#V[\mathbb{Z}/p\mathbb{Z}]=p^{2}\cdot \left\{\begin{array}{@{}ll@{}}\displaystyle p^{3}+p(p-1)-1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1\text{ and }n\equiv 0\;(\text{mod}\;p),\\ \displaystyle p^{3}-p & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1\text{ and }n\not \equiv 0\;(\text{mod}\;p),\\ \displaystyle p^{3}-p^{2}-p+1 & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=1\text{ and }n\equiv 0\;(\text{mod}\;p),\\ \displaystyle p^{3}-p & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=1\text{ and }n\not \equiv 0\;(\text{mod}\;p).\end{array}\right. & & \displaystyle \nonumber\end{eqnarray}$$
Now, if
![]() $M\neq I$
, the effect of
$M\neq I$
, the effect of
![]() $M$
on equation (6.25) is to apply an invertible linear transformation to
$M$
on equation (6.25) is to apply an invertible linear transformation to
![]() $(\mathbb{Z}[\sqrt{-d}]/(p))^{2}$
(recall that we are dealing only with good primes
$(\mathbb{Z}[\sqrt{-d}]/(p))^{2}$
(recall that we are dealing only with good primes
![]() $p$
). This takes
$p$
). This takes
![]() $U(\mathbb{Z}[\sqrt{-d}]/(p))$
to
$U(\mathbb{Z}[\sqrt{-d}]/(p))$
to
![]() $U(\mathbb{Z}[\sqrt{-d}]/(p))$
. Therefore, the number of solutions
$U(\mathbb{Z}[\sqrt{-d}]/(p))$
. Therefore, the number of solutions
![]() $\#\mathbb{V}[\mathbb{Z}/p\mathbb{Z}]$
is unaffected.
$\#\mathbb{V}[\mathbb{Z}/p\mathbb{Z}]$
is unaffected.
Therefore, we obtain the formula for
![]() $\unicode[STIX]{x1D70F}_{p}(n)$
:
$\unicode[STIX]{x1D70F}_{p}(n)$
:
 $$\begin{eqnarray}\unicode[STIX]{x1D70F}_{p}(n)=\left\{\begin{array}{@{}ll@{}}\displaystyle \frac{p+1}{p^{2}+1} & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1\text{ and }n\equiv 0\;(\text{mod}\;p),\\ \displaystyle \frac{p}{p^{2}+1} & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1\text{ and }n\not \equiv 0\;(\text{mod}\;p),\\ \displaystyle \frac{1}{p+1} & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=1\text{ and }n\equiv 0\;(\text{mod}\;p),\\ \displaystyle \frac{p}{p^{2}-1} & \text{if }\biggl(\frac{-d}{p}\biggr)=1\text{ and }n\not \equiv 0\;(\text{mod}\;p),\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70F}_{p}(n)=\left\{\begin{array}{@{}ll@{}}\displaystyle \frac{p+1}{p^{2}+1} & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1\text{ and }n\equiv 0\;(\text{mod}\;p),\\ \displaystyle \frac{p}{p^{2}+1} & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=-1\text{ and }n\not \equiv 0\;(\text{mod}\;p),\\ \displaystyle \frac{1}{p+1} & \displaystyle \text{if }\biggl(\frac{-d}{p}\biggr)=1\text{ and }n\equiv 0\;(\text{mod}\;p),\\ \displaystyle \frac{p}{p^{2}-1} & \text{if }\biggl(\frac{-d}{p}\biggr)=1\text{ and }n\not \equiv 0\;(\text{mod}\;p),\end{array}\right.\end{eqnarray}$$
and indeed we have that
![]() $\unicode[STIX]{x1D70F}_{p}(n)=1/p+O(1/p^{3})$
if
$\unicode[STIX]{x1D70F}_{p}(n)=1/p+O(1/p^{3})$
if
![]() $n\not \equiv 0\;(\text{mod}\;p)$
and
$n\not \equiv 0\;(\text{mod}\;p)$
and
![]() $\unicode[STIX]{x1D70F}_{p}(n)=1/p+O(1/p^{2})$
if
$\unicode[STIX]{x1D70F}_{p}(n)=1/p+O(1/p^{2})$
if
![]() $n\equiv 0\;(\text{mod}\;p)$
as desired.◻
$n\equiv 0\;(\text{mod}\;p)$
as desired.◻
Proof of Lemma 6.5.
Recall from (6.11) that
 $$\begin{eqnarray}\displaystyle B_{p}(n) & = & \displaystyle \mathop{\sum }_{r(p)}\unicode[STIX]{x1D70F}_{p}(r)c_{p}(r-n)\nonumber\\ \displaystyle & = & \displaystyle \unicode[STIX]{x1D70F}_{p}(n)(p-1)+\mathop{\sum }_{\substack{ r(p) \\ r\not \equiv n(p)}}-\unicode[STIX]{x1D70F}_{p}(r)\nonumber\\ \displaystyle & = & \displaystyle \unicode[STIX]{x1D70F}_{p}(n)(p-1)-(1-\unicode[STIX]{x1D70F}_{p}(n))\nonumber\\ \displaystyle & = & \displaystyle p\unicode[STIX]{x1D70F}_{p}(n)-1.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle B_{p}(n) & = & \displaystyle \mathop{\sum }_{r(p)}\unicode[STIX]{x1D70F}_{p}(r)c_{p}(r-n)\nonumber\\ \displaystyle & = & \displaystyle \unicode[STIX]{x1D70F}_{p}(n)(p-1)+\mathop{\sum }_{\substack{ r(p) \\ r\not \equiv n(p)}}-\unicode[STIX]{x1D70F}_{p}(r)\nonumber\\ \displaystyle & = & \displaystyle \unicode[STIX]{x1D70F}_{p}(n)(p-1)-(1-\unicode[STIX]{x1D70F}_{p}(n))\nonumber\\ \displaystyle & = & \displaystyle p\unicode[STIX]{x1D70F}_{p}(n)-1.\nonumber\end{eqnarray}$$
Now apply Lemma 6.6. ◻
We now combine everything to obtain an estimate of
![]() $\mathfrak{S}(n)$
.
$\mathfrak{S}(n)$
.
Lemma 6.7. The term
![]() $\mathfrak{S}(n)\neq 0$
if and only if
$\mathfrak{S}(n)\neq 0$
if and only if
![]() $n$
is admissible, and when
$n$
is admissible, and when
![]() $n$
is admissible we have
$n$
is admissible we have
![]() $\mathfrak{S}(n)\gg 1/(\text{log}\;n)$
.
$\mathfrak{S}(n)\gg 1/(\text{log}\;n)$
.
Proof. We have already observed that
![]() $n$
is admissible if and only if it is represented modulo all integers, which occurs if and only if
$n$
is admissible if and only if it is represented modulo all integers, which occurs if and only if
![]() $\mathfrak{S}(n)\neq 0$
. If
$\mathfrak{S}(n)\neq 0$
. If
![]() $n$
is admissible, Lemma 6.3 demonstrates that
$n$
is admissible, Lemma 6.3 demonstrates that
![]() $\mathfrak{S}(n)\asymp \prod _{p\;\text{good}}(1+B_{p}(n))$
. Lemma 6.5 shows that
$\mathfrak{S}(n)\asymp \prod _{p\;\text{good}}(1+B_{p}(n))$
. Lemma 6.5 shows that
![]() $1+B_{p}(n)=1+O(1/p)$
or
$1+B_{p}(n)=1+O(1/p)$
or
![]() $1+O(1/p^{2})$
; the contribution from the latter converges, and the contribution from the former is bounded below by
$1+O(1/p^{2})$
; the contribution from the latter converges, and the contribution from the former is bounded below by
![]() $1/(\text{log}\,n)$
.◻
$1/(\text{log}\,n)$
.◻
Finally, we show that the difference between
![]() $\mathfrak{S}_{Q_{0}}(n)$
and
$\mathfrak{S}_{Q_{0}}(n)$
and
![]() $\mathfrak{S}(n)$
is indeed small.
$\mathfrak{S}(n)$
is indeed small.
Lemma 6.8. We have
Recall here that
![]() $Q_{0}$
is a small power of
$Q_{0}$
is a small power of
![]() $N$
.
$N$
.
Proof. Let
![]() $L_{1}$
be as in Lemma 6.4. Write
$L_{1}$
be as in Lemma 6.4. Write
![]() $q=q_{1}q_{2}q_{3}$
, where
$q=q_{1}q_{2}q_{3}$
, where
![]() $q_{1}=(q,L_{1}),q_{2}=(q/q_{1},n)$
, so that
$q_{1}=(q,L_{1}),q_{2}=(q/q_{1},n)$
, so that
![]() $(q_{3},L_{1}n)=1$
. Noting that
$(q_{3},L_{1}n)=1$
. Noting that
![]() $B_{q_{1}}(n)$
has a universal upper bound, and recalling that
$B_{q_{1}}(n)$
has a universal upper bound, and recalling that
![]() $B_{q}(n)$
is multiplicative with respect to
$B_{q}(n)$
is multiplicative with respect to
![]() $q$
, we have
$q$
, we have
 $$\begin{eqnarray}\displaystyle |\mathfrak{S}_{Q_{0}}(n)-\mathfrak{S}(n)| & {\leqslant} & \displaystyle \mathop{\sum }_{q>Q_{0}}|B_{q}(n)|\nonumber\\ \displaystyle & = & \displaystyle \mathop{\sum }_{q_{1}|L_{1}}|B_{q_{1}}(n)|\mathop{\sum }_{\substack{ (q_{2},L_{1})=1 \\ q_{2}|n}}|B_{q_{2}}(n)|\mathop{\sum }_{\substack{ (q_{3},L_{1}n)=1 \\ q_{1}q_{2}q_{3}\geqslant Q_{0}}}|B_{q_{3}}(n)|\nonumber\\ \displaystyle & \ll & \displaystyle \mathop{\sum }_{q_{1}|L_{1}}\mathop{\sum }_{\substack{ (q_{2},L_{1})=1 \\ q_{2}|n}}\frac{1}{q_{2}}\mathop{\sum }_{\substack{ (q_{3},L_{1}n)=1 \\ q_{3}\geqslant Q_{0}/q_{1}q_{2}}}\frac{1}{q_{3}^{2}}\ll \mathop{\sum }_{q_{2}|n}\frac{1}{q_{2}}\frac{q_{2}}{Q_{0}}\ll \frac{\log n}{Q_{0}}\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle |\mathfrak{S}_{Q_{0}}(n)-\mathfrak{S}(n)| & {\leqslant} & \displaystyle \mathop{\sum }_{q>Q_{0}}|B_{q}(n)|\nonumber\\ \displaystyle & = & \displaystyle \mathop{\sum }_{q_{1}|L_{1}}|B_{q_{1}}(n)|\mathop{\sum }_{\substack{ (q_{2},L_{1})=1 \\ q_{2}|n}}|B_{q_{2}}(n)|\mathop{\sum }_{\substack{ (q_{3},L_{1}n)=1 \\ q_{1}q_{2}q_{3}\geqslant Q_{0}}}|B_{q_{3}}(n)|\nonumber\\ \displaystyle & \ll & \displaystyle \mathop{\sum }_{q_{1}|L_{1}}\mathop{\sum }_{\substack{ (q_{2},L_{1})=1 \\ q_{2}|n}}\frac{1}{q_{2}}\mathop{\sum }_{\substack{ (q_{3},L_{1}n)=1 \\ q_{3}\geqslant Q_{0}/q_{1}q_{2}}}\frac{1}{q_{3}^{2}}\ll \mathop{\sum }_{q_{2}|n}\frac{1}{q_{2}}\frac{q_{2}}{Q_{0}}\ll \frac{\log n}{Q_{0}}\nonumber\end{eqnarray}$$
as desired. ◻
Lemmas 6.7 and 6.8 together imply Proposition 6.1. Therefore, by the discussion preceding Proposition 6.1, we have shown Theorem 4.4.
7 Minor arcs
The aim of this section is to prove
By Plancherel’s theorem, (7.1) leads to Theorem 4.5.
We bound the integral (7.1) above by
![]() ${\mathcal{I}}_{1}+{\mathcal{I}}_{2}+{\mathcal{I}}_{3}$
, where
${\mathcal{I}}_{1}+{\mathcal{I}}_{2}+{\mathcal{I}}_{3}$
, where
![]() $J$
is the depth of approximation (see (4.11)) and
$J$
is the depth of approximation (see (4.11)) and
The integrand is periodic on
![]() $\mathbb{R}$
modulo
$\mathbb{R}$
modulo
![]() $1$
, and by Dirichlet’s theorem on Diophantine approximation the domains of these integrals cover the circle
$1$
, and by Dirichlet’s theorem on Diophantine approximation the domains of these integrals cover the circle
![]() $\mathbb{R}$
modulo
$\mathbb{R}$
modulo
![]() $1$
. The first integral
$1$
. The first integral
![]() ${\mathcal{I}}_{1}$
concerns small
${\mathcal{I}}_{1}$
concerns small
![]() $q$
in the range of the major arc analysis, the second integral
$q$
in the range of the major arc analysis, the second integral
![]() ${\mathcal{I}}_{2}$
concerns
${\mathcal{I}}_{2}$
concerns
![]() $q$
in the intermediate range
$q$
in the intermediate range
![]() $Q_{0}\leqslant q<X$
, and the last integral
$Q_{0}\leqslant q<X$
, and the last integral
![]() ${\mathcal{I}}_{3}$
concerns large
${\mathcal{I}}_{3}$
concerns large
![]() $q$
.
$q$
.
In §7.2 we show
Then, in §§ 7.3 and 7.4 we divide
![]() $[Q_{0},X]$
dyadically and prove
$[Q_{0},X]$
dyadically and prove
where
![]() $Q_{0}\leqslant Q<X$
and
$Q_{0}\leqslant Q<X$
and
![]() $X\leqslant Q\leqslant J$
, respectively. In doing this, we deal with the ranges of
$X\leqslant Q\leqslant J$
, respectively. In doing this, we deal with the ranges of
![]() $Q$
corresponding to
$Q$
corresponding to
![]() ${\mathcal{I}}_{1}$
and
${\mathcal{I}}_{1}$
and
![]() ${\mathcal{I}}_{2}$
separately, and this will give the desired upper bounds on those sums.
${\mathcal{I}}_{2}$
separately, and this will give the desired upper bounds on those sums.
It is evident that whether or not
![]() $M$
is fractional has little effect in the minor arc analysis: the main player here is the congruence subgroup
$M$
is fractional has little effect in the minor arc analysis: the main player here is the congruence subgroup
![]() $\unicode[STIX]{x1D6E4}(L)$
which gives rise to shifted quadratic forms. We can simply replace the shifted quadratic form by a constant multiple of the form, and the analysis will run in exactly the same way.
$\unicode[STIX]{x1D6E4}(L)$
which gives rise to shifted quadratic forms. We can simply replace the shifted quadratic form by a constant multiple of the form, and the analysis will run in exactly the same way.
7.1 Lemmas for minor arcs
In this section, we include some lemmas which will be used in the minor arc analysis. The reader can choose to continue to the next section and refer back here for statements. These lemmas relate to the evaluation and bounds for exponential sums of the form
and certain of their averages. For simplicity we assume
![]() $q$
is odd. For
$q$
is odd. For
![]() $z\in \mathbb{Q}_{p}$
, we define
$z\in \mathbb{Q}_{p}$
, we define
We need the following lemma, which is a direct corollary of Gauss sums (see [Reference DavenportDav00, p. 13]).
Lemma 7.1. For
![]() $a,b\in \mathbb{Z}$
, we have
$a,b\in \mathbb{Z}$
, we have
 $$\begin{eqnarray}\displaystyle & & \displaystyle \mathop{\sum }_{x\in \mathbb{Z}/p^{m}\mathbb{Z}}e_{p^{m}}(ax^{2}+bx)\nonumber\\ \displaystyle & & \displaystyle \quad =\left\{\begin{array}{@{}ll@{}}p^{m}\cdot \mathbf{1}\{p^{m}|b\}\quad & \text{if }p^{m}|a,\\ \displaystyle p^{m/2}(p^{m},a)^{1/2}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},a))}\left(\begin{array}{@{}c@{}}\displaystyle \frac{\frac{a}{(p^{m-1},a)}}{p}\end{array}\right)e_{p}\biggl(-\frac{b^{2}}{4a}\biggr)\quad & \\ \quad \cdot \,\mathbf{1}\{\text{deg}_{p^{m}}(b)\geqslant \text{deg}_{p^{m}}(a)\}\quad & \text{if }p^{m}\nmid a,\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \mathop{\sum }_{x\in \mathbb{Z}/p^{m}\mathbb{Z}}e_{p^{m}}(ax^{2}+bx)\nonumber\\ \displaystyle & & \displaystyle \quad =\left\{\begin{array}{@{}ll@{}}p^{m}\cdot \mathbf{1}\{p^{m}|b\}\quad & \text{if }p^{m}|a,\\ \displaystyle p^{m/2}(p^{m},a)^{1/2}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},a))}\left(\begin{array}{@{}c@{}}\displaystyle \frac{\frac{a}{(p^{m-1},a)}}{p}\end{array}\right)e_{p}\biggl(-\frac{b^{2}}{4a}\biggr)\quad & \\ \quad \cdot \,\mathbf{1}\{\text{deg}_{p^{m}}(b)\geqslant \text{deg}_{p^{m}}(a)\}\quad & \text{if }p^{m}\nmid a,\end{array}\right.\end{eqnarray}$$
where
![]() $\unicode[STIX]{x1D716}(n)=0$
if
$\unicode[STIX]{x1D716}(n)=0$
if
![]() $n\equiv 1\;(\text{mod}\;4)$
and
$n\equiv 1\;(\text{mod}\;4)$
and
![]() $\unicode[STIX]{x1D716}(n)=1$
if
$\unicode[STIX]{x1D716}(n)=1$
if
![]() $n\equiv 3\;(\text{mod}\;4)$
, and
$n\equiv 3\;(\text{mod}\;4)$
, and
![]() $(\frac{\cdot }{p})$
is the Legendre symbol.
$(\frac{\cdot }{p})$
is the Legendre symbol.
The Legendre symbol
![]() $(\frac{a}{p})=1$
if
$(\frac{a}{p})=1$
if
![]() $a$
is a quadratic residue, and
$a$
is a quadratic residue, and
![]() $-1$
if it is a quadratic non-residue. By convention we also let
$-1$
if it is a quadratic non-residue. By convention we also let
![]() $(\frac{a}{p})=1$
if
$(\frac{a}{p})=1$
if
![]() $a\equiv 0\;(\text{mod}\;p)$
. The Legendre symbol is multiplicative only on the set of non-zero congruence classes
$a\equiv 0\;(\text{mod}\;p)$
. The Legendre symbol is multiplicative only on the set of non-zero congruence classes
![]() $\text{mod}\;p$
.
$\text{mod}\;p$
.
Write
![]() $g(x,y)=Ax^{2}+Bxy+Cy^{2}$
and
$g(x,y)=Ax^{2}+Bxy+Cy^{2}$
and
![]() $\unicode[STIX]{x1D6E5}_{g}=B^{2}-4AC$
. Let
$\unicode[STIX]{x1D6E5}_{g}=B^{2}-4AC$
. Let
![]() $k_{g}=\text{deg}_{p^{m}}(A,B,C)$
. If
$k_{g}=\text{deg}_{p^{m}}(A,B,C)$
. If
![]() $k_{g}<m$
and
$k_{g}<m$
and
![]() $p^{m}|\unicode[STIX]{x1D6E5}_{g}/p^{k_{g}}$
, we say that
$p^{m}|\unicode[STIX]{x1D6E5}_{g}/p^{k_{g}}$
, we say that
![]() $g$
is degenerate at
$g$
is degenerate at
![]() $p^{m}$
; in this case
$p^{m}$
; in this case
![]() $g$
is essentially a quadratic form of only one variable.
$g$
is essentially a quadratic form of only one variable.
From Lemma 7.1, we obtain the following result.
Lemma 7.2. Let
![]() $p$
be an odd prime. Let
$p$
be an odd prime. Let
![]() $k_{g}=\text{deg}_{p^{m}}(\text{gcd}(A,B,C))$
. If
$k_{g}=\text{deg}_{p^{m}}(\text{gcd}(A,B,C))$
. If
![]() $k_{g}=m$
, then
$k_{g}=m$
, then
If
![]() $k_{g}<m$
, then
$k_{g}<m$
, then
 $$\begin{eqnarray}\displaystyle S(p^{m},A,B,C,D,E) & = & \displaystyle p^{m}p^{k_{g}/2}\biggl(p^{m},\frac{\unicode[STIX]{x1D6E5}_{g}}{p^{k_{g}}}\biggr)^{1/2}i^{\unicode[STIX]{x1D716}(p^{m-k_{g}})}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},\unicode[STIX]{x1D6E5}_{g}/p^{k_{g}}))}e_{p^{m}}\biggl(\frac{g(E,-D)}{\unicode[STIX]{x1D6E5}_{g}}\biggr)\nonumber\\ \displaystyle & & \displaystyle \cdot \,(-1)^{\unicode[STIX]{x1D710}(g)}\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E),\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle S(p^{m},A,B,C,D,E) & = & \displaystyle p^{m}p^{k_{g}/2}\biggl(p^{m},\frac{\unicode[STIX]{x1D6E5}_{g}}{p^{k_{g}}}\biggr)^{1/2}i^{\unicode[STIX]{x1D716}(p^{m-k_{g}})}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},\unicode[STIX]{x1D6E5}_{g}/p^{k_{g}}))}e_{p^{m}}\biggl(\frac{g(E,-D)}{\unicode[STIX]{x1D6E5}_{g}}\biggr)\nonumber\\ \displaystyle & & \displaystyle \cdot \,(-1)^{\unicode[STIX]{x1D710}(g)}\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E),\end{eqnarray}$$
where
![]() $\unicode[STIX]{x1D710}(g)=0$
if
$\unicode[STIX]{x1D710}(g)=0$
if
![]() $g$
is non-degenerate at
$g$
is non-degenerate at
![]() $p^{m}$
and
$p^{m}$
and
![]() $(\frac{-\unicode[STIX]{x1D6E5}_{g}/(\unicode[STIX]{x1D6E5}_{g},p^{2m-2})}{p})=1$
, or
$(\frac{-\unicode[STIX]{x1D6E5}_{g}/(\unicode[STIX]{x1D6E5}_{g},p^{2m-2})}{p})=1$
, or
![]() $g$
is degenerate and the quadratic form
$g$
is degenerate and the quadratic form
![]() $g(x,y)/p^{k_{g}}$
can represent non-zero quadratic residue
$g(x,y)/p^{k_{g}}$
can represent non-zero quadratic residue
![]() $\text{mod}\;p$
;
$\text{mod}\;p$
;
![]() $\unicode[STIX]{x1D710}(g)=1$
otherwise. The function
$\unicode[STIX]{x1D710}(g)=1$
otherwise. The function
![]() $\unicode[STIX]{x1D712}(p^{m};A,B,C,D,E)=1$
if
$\unicode[STIX]{x1D712}(p^{m};A,B,C,D,E)=1$
if
![]() $S(p^{m},A,B,C,D,E)\neq 0$
, and
$S(p^{m},A,B,C,D,E)\neq 0$
, and
![]() $\unicode[STIX]{x1D712}(p^{m};A,B,C,D,E)=0$
if
$\unicode[STIX]{x1D712}(p^{m};A,B,C,D,E)=0$
if
![]() $S(p^{m},A,B,C,D,E)\neq 0$
.
$S(p^{m},A,B,C,D,E)\neq 0$
.
Proof. The proof proceeds case by case, and the statement of Lemma 7.2 is a synthesis of all cases.
If
![]() $k_{g}=m$
, the proof is trivial. We thus assume
$k_{g}=m$
, the proof is trivial. We thus assume
![]() $k_{g}<m$
. Then after a linear unimodular change of variables, we can rewrite
$k_{g}<m$
. Then after a linear unimodular change of variables, we can rewrite
where
![]() $\text{deg}_{p^{m}}(A^{\prime })=k_{g}$
and
$\text{deg}_{p^{m}}(A^{\prime })=k_{g}$
and
![]() $C^{\prime }=-\unicode[STIX]{x1D6E5}_{g}/4A^{\prime }$
. For instance, if
$C^{\prime }=-\unicode[STIX]{x1D6E5}_{g}/4A^{\prime }$
. For instance, if
![]() $\text{deg}_{p^{m}}(A)=\text{deg}_{p^{m}}(\text{gcd}(A,B,C))$
, then we can let
$\text{deg}_{p^{m}}(A)=\text{deg}_{p^{m}}(\text{gcd}(A,B,C))$
, then we can let
![]() $x^{\prime }=x+(B/2A)y$
,
$x^{\prime }=x+(B/2A)y$
,
![]() $y^{\prime }=y$
, in which case
$y^{\prime }=y$
, in which case
![]() $Ax^{2}+Bxy+Cy^{2}+Dx+Ey=A{x^{\prime }}^{2}+(C-B^{2}/4A){y^{\prime }}^{2}+Dx^{\prime }+(E-BD/2A)y^{\prime }$
. If, instead,
$Ax^{2}+Bxy+Cy^{2}+Dx+Ey=A{x^{\prime }}^{2}+(C-B^{2}/4A){y^{\prime }}^{2}+Dx^{\prime }+(E-BD/2A)y^{\prime }$
. If, instead,
![]() $\text{deg}_{p^{m}}(B)<\text{deg}_{p^{m}}(A),\text{deg}_{p^{m}}(C)$
, then we can apply the change
$\text{deg}_{p^{m}}(B)<\text{deg}_{p^{m}}(A),\text{deg}_{p^{m}}(C)$
, then we can apply the change
![]() $x^{\prime }=x+y$
,
$x^{\prime }=x+y$
,
![]() $y^{\prime }=x-y$
to reduce to the previous case.
$y^{\prime }=x-y$
to reduce to the previous case.
Now we can evaluate
![]() $S(p^{m},A,B,C,D,E)=S(p^{m},A^{\prime },0,C^{\prime },D^{\prime },E^{\prime })$
from Lemma 7.1. We have
$S(p^{m},A,B,C,D,E)=S(p^{m},A^{\prime },0,C^{\prime },D^{\prime },E^{\prime })$
from Lemma 7.1. We have
 $$\begin{eqnarray}\displaystyle & & \displaystyle S(p^{m},A,B,C,D,E)\nonumber\\ \displaystyle & & \displaystyle \quad =S(p^{m},A^{\prime },0,C^{\prime },D^{\prime },E^{\prime })\nonumber\\ \displaystyle & & \displaystyle \quad =p^{m}(p^{m},A^{\prime })^{1/2}(p^{m},C^{\prime })^{1/2}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},A^{\prime }))}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},C^{\prime }))}\nonumber\\ \displaystyle & & \displaystyle \qquad \cdot \,\left(\frac{\frac{A^{\prime }}{(A^{\prime },p^{m-1})}}{p}\right)\left(\frac{\frac{C^{\prime }}{(C^{\prime },p^{m-1})}}{p}\right)\mathbf{1}\left\{\begin{array}{@{}c@{}}\text{deg}_{p^{m}}(D^{\prime })\geqslant \text{deg}_{p^{m}}(A^{\prime })\\ \text{deg}_{p^{m}}(E^{\prime })\geqslant \text{deg}_{p^{m}}(C^{\prime })\end{array}\right\}e_{p^{m}}\biggl(\frac{g(E,-D)}{\unicode[STIX]{x1D6E5}_{g}}\biggr).\qquad\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle S(p^{m},A,B,C,D,E)\nonumber\\ \displaystyle & & \displaystyle \quad =S(p^{m},A^{\prime },0,C^{\prime },D^{\prime },E^{\prime })\nonumber\\ \displaystyle & & \displaystyle \quad =p^{m}(p^{m},A^{\prime })^{1/2}(p^{m},C^{\prime })^{1/2}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},A^{\prime }))}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},C^{\prime }))}\nonumber\\ \displaystyle & & \displaystyle \qquad \cdot \,\left(\frac{\frac{A^{\prime }}{(A^{\prime },p^{m-1})}}{p}\right)\left(\frac{\frac{C^{\prime }}{(C^{\prime },p^{m-1})}}{p}\right)\mathbf{1}\left\{\begin{array}{@{}c@{}}\text{deg}_{p^{m}}(D^{\prime })\geqslant \text{deg}_{p^{m}}(A^{\prime })\\ \text{deg}_{p^{m}}(E^{\prime })\geqslant \text{deg}_{p^{m}}(C^{\prime })\end{array}\right\}e_{p^{m}}\biggl(\frac{g(E,-D)}{\unicode[STIX]{x1D6E5}_{g}}\biggr).\qquad\end{eqnarray}$$
We interpret (7.11) in an intrinsic way. First, while all other factors are non-zero, the factor
is the same as the indicator function indicating whether
![]() $S$
is zero or not. So we have (7.12)
$S$
is zero or not. So we have (7.12)
![]() $=\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)$
.
$=\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)$
.
For the term
![]() $A^{\prime }$
, we know
$A^{\prime }$
, we know
![]() $\text{deg}_{p^{m}}(A^{\prime })=\deg _{p^{m}}(\text{gcd}(A,B,C))=k_{g}<m$
, and that
$\text{deg}_{p^{m}}(A^{\prime })=\deg _{p^{m}}(\text{gcd}(A,B,C))=k_{g}<m$
, and that
![]() $\text{deg}_{p^{m}}(C^{\prime })=\text{deg}_{p^{m}}(\unicode[STIX]{x1D6E5}_{g}/A^{\prime })$
.
$\text{deg}_{p^{m}}(C^{\prime })=\text{deg}_{p^{m}}(\unicode[STIX]{x1D6E5}_{g}/A^{\prime })$
.
If
![]() $p^{m}\nmid C^{\prime }$
, then
$p^{m}\nmid C^{\prime }$
, then
![]() $(\frac{A^{\prime }/(A^{\prime },p^{m-1})}{p})(\frac{C^{\prime }/(C^{\prime },p^{m-1})}{p})=(\frac{-\unicode[STIX]{x1D6E5}_{g}/(4(\unicode[STIX]{x1D6E5}_{g},p^{2m-2}))}{p})$
.
$(\frac{A^{\prime }/(A^{\prime },p^{m-1})}{p})(\frac{C^{\prime }/(C^{\prime },p^{m-1})}{p})=(\frac{-\unicode[STIX]{x1D6E5}_{g}/(4(\unicode[STIX]{x1D6E5}_{g},p^{2m-2}))}{p})$
.
If
![]() $p^{m}\mid C^{\prime }$
, then
$p^{m}\mid C^{\prime }$
, then
![]() $(\frac{C^{\prime }/(C^{\prime },p^{m-1})}{p})=1$
, and
$(\frac{C^{\prime }/(C^{\prime },p^{m-1})}{p})=1$
, and
 $$\begin{eqnarray}\biggl(\frac{\frac{A^{\prime }}{(A^{\prime },p^{m-1})}}{p}\biggr)=\left\{\begin{array}{@{}ll@{}}1\quad & \displaystyle \text{if non-zero quadratic residue }\frac{A^{\prime }}{(A^{\prime },p^{m-1})}\text{ is represented by }\\ \quad & g(x,y)/p^{k_{g}}\text{in }\mathbb{Z}/p\mathbb{Z},\\ -1\quad & \text{otherwise}.\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\biggl(\frac{\frac{A^{\prime }}{(A^{\prime },p^{m-1})}}{p}\biggr)=\left\{\begin{array}{@{}ll@{}}1\quad & \displaystyle \text{if non-zero quadratic residue }\frac{A^{\prime }}{(A^{\prime },p^{m-1})}\text{ is represented by }\\ \quad & g(x,y)/p^{k_{g}}\text{in }\mathbb{Z}/p\mathbb{Z},\\ -1\quad & \text{otherwise}.\end{array}\right.\end{eqnarray}$$
◻
We note here that the function
![]() $\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)$
concerns whether the
$\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)$
concerns whether the
![]() $p^{m}$
-degrees of the
$p^{m}$
-degrees of the
![]() $x,y$
coefficients are bigger than or equal to those of the
$x,y$
coefficients are bigger than or equal to those of the
![]() $x^{2},y^{2}$
coefficients after diagonalizing the quadratic part of
$x^{2},y^{2}$
coefficients after diagonalizing the quadratic part of
![]() $Ax^{2}+Bxy+Cy^{2}+Dx+Ey$
. We list the following two noteworthy properties of
$Ax^{2}+Bxy+Cy^{2}+Dx+Ey$
. We list the following two noteworthy properties of
![]() $\unicode[STIX]{x1D712}$
.
$\unicode[STIX]{x1D712}$
.
(i)
 $\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)$
is invariant under scaling of the quadratic part or the linear part, i.e. for any
$\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)$
is invariant under scaling of the quadratic part or the linear part, i.e. for any
 $(r,p)=1$
, (7.14)
$(r,p)=1$
, (7.14) $$\begin{eqnarray}\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)=\unicode[STIX]{x1D712}(p^{m},rA,rB,rC,D,E)=\unicode[STIX]{x1D712}(p^{m},A,B,C,rD,rE).\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)=\unicode[STIX]{x1D712}(p^{m},rA,rB,rC,D,E)=\unicode[STIX]{x1D712}(p^{m},A,B,C,rD,rE).\end{eqnarray}$$
(ii)
 $\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)$
is invariant under changing variables of
$\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)$
is invariant under changing variables of
 $x,y$
. If
$x,y$
. If
 $x=x_{1}+a,y=y_{1}+b$
, then (7.15)Comparing the coefficients of the quadratic parts and linear parts of (7.15), we have
$x=x_{1}+a,y=y_{1}+b$
, then (7.15)Comparing the coefficients of the quadratic parts and linear parts of (7.15), we have $$\begin{eqnarray}\displaystyle Ax^{2}+Bxy+Cy^{2}+Dx+Ey & = & \displaystyle Ax_{1}^{2}+Bx_{1}y_{1}+Cy_{1}^{2}+(2Aa+Bb+D)x_{1}\nonumber\\ \displaystyle & & \displaystyle +\,(2Cb+Ba+E)y_{1}+Aa^{2}+Bab+Cb^{2}+Da+Eb.\nonumber\\ \displaystyle & & \displaystyle\end{eqnarray}$$
(7.16)
$$\begin{eqnarray}\displaystyle Ax^{2}+Bxy+Cy^{2}+Dx+Ey & = & \displaystyle Ax_{1}^{2}+Bx_{1}y_{1}+Cy_{1}^{2}+(2Aa+Bb+D)x_{1}\nonumber\\ \displaystyle & & \displaystyle +\,(2Cb+Ba+E)y_{1}+Aa^{2}+Bab+Cb^{2}+Da+Eb.\nonumber\\ \displaystyle & & \displaystyle\end{eqnarray}$$
(7.16) $$\begin{eqnarray}\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)=\unicode[STIX]{x1D712}(p^{m},A,B,C,2Aa+Bb+D,2Cb+Ba+E).\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D712}(p^{m},A,B,C,D,E)=\unicode[STIX]{x1D712}(p^{m},A,B,C,2Aa+Bb+D,2Cb+Ba+E).\end{eqnarray}$$
In § 7.2 we will encounter the exponential sum
Write
The quadratic form has discriminant
![]() $\unicode[STIX]{x1D6E5}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}$
. We assume that
$\unicode[STIX]{x1D6E5}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}$
. We assume that
![]() $M$
is integral, so that
$M$
is integral, so that
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
is primitive and integral by Lemma 3.1. If
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
is primitive and integral by Lemma 3.1. If
![]() $M$
is not integral, then one needs to multiply the curvature formula by a universal constant, to obtain integrality. By Lemma 3.1, the gcd of the coefficients of
$M$
is not integral, then one needs to multiply the curvature formula by a universal constant, to obtain integrality. By Lemma 3.1, the gcd of the coefficients of
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
after this normalization is bounded for all
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
after this normalization is bounded for all
![]() $\unicode[STIX]{x1D6FE}$
, and consequently all the estimates from Lemmas 7.3–7.5 stand, up to a constant factor.
$\unicode[STIX]{x1D6FE}$
, and consequently all the estimates from Lemmas 7.3–7.5 stand, up to a constant factor.
We first give a bound for
![]() ${\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(q,u,r,\unicode[STIX]{x1D709},\unicode[STIX]{x1D701})$
.
${\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(q,u,r,\unicode[STIX]{x1D709},\unicode[STIX]{x1D701})$
.
Lemma 7.3. Assume that
![]() $(r,q)=1$
. Then
$(r,q)=1$
. Then
Proof of Lemma 7.3 for
 $q$
odd.
$q$
odd.
For the proof when
![]() $q$
is even, see the discussion at the end of this section.
$q$
is even, see the discussion at the end of this section.
First we consider the case
![]() $q=p^{m}$
. If
$q=p^{m}$
. If
![]() $p^{m}\mid u^{2}L^{2}$
, then we trivially bound
$p^{m}\mid u^{2}L^{2}$
, then we trivially bound
![]() $|{\mathcal{S}}_{\unicode[STIX]{x1D6FE}}|\leqslant 1$
and we automatically get the lemma. We thus assume
$|{\mathcal{S}}_{\unicode[STIX]{x1D6FE}}|\leqslant 1$
and we automatically get the lemma. We thus assume
![]() $p^{m}\nmid u^{2}L^{2}$
, then we apply the second case of Lemma 7.2 to analyze
$p^{m}\nmid u^{2}L^{2}$
, then we apply the second case of Lemma 7.2 to analyze
![]() ${\mathcal{S}}_{\unicode[STIX]{x1D6FE}}$
.
${\mathcal{S}}_{\unicode[STIX]{x1D6FE}}$
.
We write
 $$\begin{eqnarray}\displaystyle {\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(p^{m},u,r,\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}) & = & \displaystyle \frac{1}{p^{2m}}\mathop{\sum }_{x_{0},y_{0}(p^{m})}e_{p^{m}}(r\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux_{0}+uu^{\ast },Luy_{0})+x_{0}\unicode[STIX]{x1D709}+y_{0}\unicode[STIX]{x1D701})\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{p^{2m}}\mathop{\sum }_{x_{0},y_{0}(p^{m})}e_{p^{m}} (rL^{2}u^{2}(A^{\prime \prime }x_{0}^{2}+B^{\prime \prime }x_{0}y_{0}+C^{\prime \prime }y_{0}^{2})\nonumber\\ \displaystyle & & \displaystyle +\,(2rA^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D709})x_{0}+(rB^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D701})y_{0}+ru^{2}A^{\prime \prime }{u^{\ast }}^{2}+r\mathfrak{d}_{\unicode[STIX]{x1D6FE}}).\qquad\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(p^{m},u,r,\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}) & = & \displaystyle \frac{1}{p^{2m}}\mathop{\sum }_{x_{0},y_{0}(p^{m})}e_{p^{m}}(r\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux_{0}+uu^{\ast },Luy_{0})+x_{0}\unicode[STIX]{x1D709}+y_{0}\unicode[STIX]{x1D701})\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{p^{2m}}\mathop{\sum }_{x_{0},y_{0}(p^{m})}e_{p^{m}} (rL^{2}u^{2}(A^{\prime \prime }x_{0}^{2}+B^{\prime \prime }x_{0}y_{0}+C^{\prime \prime }y_{0}^{2})\nonumber\\ \displaystyle & & \displaystyle +\,(2rA^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D709})x_{0}+(rB^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D701})y_{0}+ru^{2}A^{\prime \prime }{u^{\ast }}^{2}+r\mathfrak{d}_{\unicode[STIX]{x1D6FE}}).\qquad\end{eqnarray}$$
Therefore, having the primitivity of
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
in mind and applying Lemma 7.2 to (7.18), we obtain (here
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
in mind and applying Lemma 7.2 to (7.18), we obtain (here
![]() $p^{k_{g}}=(p^{m},u^{2}L^{2})$
):
$p^{k_{g}}=(p^{m},u^{2}L^{2})$
):
 $$\begin{eqnarray}\displaystyle & & \displaystyle {\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(p^{m},u,r,\unicode[STIX]{x1D709},\unicode[STIX]{x1D701})\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{p^{m}}e_{p^{m}}(r\mathfrak{d}_{\unicode[STIX]{x1D6FE}}+ru^{2}A^{\prime \prime }{u^{\ast }}^{2})(p^{m},u^{2}L^{2})^{1/2}(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5})^{1/2}\nonumber\\ \displaystyle & & \displaystyle \qquad \times \,i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}))}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5}))}\cdot (-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}})}\nonumber\\ \displaystyle & & \displaystyle \qquad \cdot \,e_{p^{m}}\biggl(\frac{A^{\prime \prime }(rB^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D701})^{2}-B^{\prime \prime }(2rA^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D709})(rB^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D701})+C^{\prime \prime }(2rA^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D709})^{2}}{rL^{2}u^{2}({B^{\prime \prime }}^{2}-4A^{\prime \prime }C^{\prime \prime })}\biggr)\nonumber\\ \displaystyle & & \displaystyle \qquad \cdot \,\unicode[STIX]{x1D712}(p^{m},ru^{2}L^{2}A^{\prime \prime },ru^{2}L^{2}B^{\prime \prime },ru^{2}L^{2}C^{\prime \prime },2rA^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D709},rB^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D701})\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{p^{m}}e_{p^{m}}\biggl(r\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\frac{u^{\ast }\unicode[STIX]{x1D709}}{L}\biggr)(p^{m},u^{2}L^{2})^{1/2}(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5})^{1/2}\nonumber\\ \displaystyle & & \displaystyle \qquad \times \,i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}))}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5}))}\cdot (-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}})}\cdot e_{p^{m}}\biggl(\frac{\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D701},-\unicode[STIX]{x1D709})}{ru^{2}L^{2}\unicode[STIX]{x1D6E5}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}\biggr)\nonumber\\ \displaystyle & & \displaystyle \qquad \cdot \,\unicode[STIX]{x1D712}(p^{m},ru^{2}L^{2}A^{\prime \prime },ru^{2}L^{2}B^{\prime \prime },ru^{2}L^{2}C^{\prime \prime },2rA^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D709},rB^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D701}).\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle {\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(p^{m},u,r,\unicode[STIX]{x1D709},\unicode[STIX]{x1D701})\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{p^{m}}e_{p^{m}}(r\mathfrak{d}_{\unicode[STIX]{x1D6FE}}+ru^{2}A^{\prime \prime }{u^{\ast }}^{2})(p^{m},u^{2}L^{2})^{1/2}(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5})^{1/2}\nonumber\\ \displaystyle & & \displaystyle \qquad \times \,i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}))}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5}))}\cdot (-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}})}\nonumber\\ \displaystyle & & \displaystyle \qquad \cdot \,e_{p^{m}}\biggl(\frac{A^{\prime \prime }(rB^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D701})^{2}-B^{\prime \prime }(2rA^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D709})(rB^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D701})+C^{\prime \prime }(2rA^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D709})^{2}}{rL^{2}u^{2}({B^{\prime \prime }}^{2}-4A^{\prime \prime }C^{\prime \prime })}\biggr)\nonumber\\ \displaystyle & & \displaystyle \qquad \cdot \,\unicode[STIX]{x1D712}(p^{m},ru^{2}L^{2}A^{\prime \prime },ru^{2}L^{2}B^{\prime \prime },ru^{2}L^{2}C^{\prime \prime },2rA^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D709},rB^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D701})\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{p^{m}}e_{p^{m}}\biggl(r\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\frac{u^{\ast }\unicode[STIX]{x1D709}}{L}\biggr)(p^{m},u^{2}L^{2})^{1/2}(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5})^{1/2}\nonumber\\ \displaystyle & & \displaystyle \qquad \times \,i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}))}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5}))}\cdot (-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}})}\cdot e_{p^{m}}\biggl(\frac{\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D701},-\unicode[STIX]{x1D709})}{ru^{2}L^{2}\unicode[STIX]{x1D6E5}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}\biggr)\nonumber\\ \displaystyle & & \displaystyle \qquad \cdot \,\unicode[STIX]{x1D712}(p^{m},ru^{2}L^{2}A^{\prime \prime },ru^{2}L^{2}B^{\prime \prime },ru^{2}L^{2}C^{\prime \prime },2rA^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D709},rB^{\prime \prime }Lu^{2}u^{\ast }+\unicode[STIX]{x1D701}).\end{eqnarray}$$
From (7.19) we thus have
Using the multiplicativity of
![]() ${\mathcal{S}}_{\unicode[STIX]{x1D6FE}}$
, we obtain
${\mathcal{S}}_{\unicode[STIX]{x1D6FE}}$
, we obtain
 $$\begin{eqnarray}\displaystyle |{\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(q,u,r,\unicode[STIX]{x1D709},\unicode[STIX]{x1D701})| & {\leqslant} & \displaystyle \mathop{\prod }_{p_{i}^{n_{i}}\Vert q}\frac{(p^{n_{i}},u^{2}L^{2})(p^{n_{i}},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5})^{1/2}}{p^{n_{i}}}\nonumber\\ \displaystyle & {\leqslant} & \displaystyle \frac{(q,u^{2}L^{2})^{1/2}(q,u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5})^{1/2}}{q}\leqslant \frac{|\unicode[STIX]{x1D6E5}|^{1/2}u^{2}L^{2}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})}{q}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle |{\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(q,u,r,\unicode[STIX]{x1D709},\unicode[STIX]{x1D701})| & {\leqslant} & \displaystyle \mathop{\prod }_{p_{i}^{n_{i}}\Vert q}\frac{(p^{n_{i}},u^{2}L^{2})(p^{n_{i}},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5})^{1/2}}{p^{n_{i}}}\nonumber\\ \displaystyle & {\leqslant} & \displaystyle \frac{(q,u^{2}L^{2})^{1/2}(q,u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5})^{1/2}}{q}\leqslant \frac{|\unicode[STIX]{x1D6E5}|^{1/2}u^{2}L^{2}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})}{q}.\end{eqnarray}$$
◻
We will also encounter a certain average of such sums. Let
Set
![]() $q=p^{m}$
. From (7.19), we can write
$q=p^{m}$
. From (7.19), we can write
with
![]() $S_{1}$
and
$S_{1}$
and
![]() $S_{2}$
as follows. The factor
$S_{2}$
as follows. The factor
![]() $S_{1}$
consists of factors not involving
$S_{1}$
consists of factors not involving
![]() $r$
:
$r$
:
 $$\begin{eqnarray}\displaystyle S_{1} & = & \displaystyle \frac{1}{p^{2m}}(p^{m},u^{2}L^{2})(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5})^{1/2}(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2}\unicode[STIX]{x1D6E5})^{1/2}i^{2\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}))}\nonumber\\ \displaystyle & & \displaystyle \times \,i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5}))}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2}\unicode[STIX]{x1D6E5}))}e\biggl(\frac{u^{\ast }(\unicode[STIX]{x1D709}^{\prime }-\unicode[STIX]{x1D709})}{L}\biggr).\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle S_{1} & = & \displaystyle \frac{1}{p^{2m}}(p^{m},u^{2}L^{2})(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5})^{1/2}(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2}\unicode[STIX]{x1D6E5})^{1/2}i^{2\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}))}\nonumber\\ \displaystyle & & \displaystyle \times \,i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}\unicode[STIX]{x1D6E5}))}i^{\unicode[STIX]{x1D716}(p^{m}/(p^{m},u^{2}L^{2}\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2}\unicode[STIX]{x1D6E5}))}e\biggl(\frac{u^{\ast }(\unicode[STIX]{x1D709}^{\prime }-\unicode[STIX]{x1D709})}{L}\biggr).\end{eqnarray}$$
For
![]() $S_{2}$
, we have
$S_{2}$
, we have
 $$\begin{eqnarray}\displaystyle S_{2} & = & \displaystyle \sum _{r(p^{m})}e_{p^{m}}(r(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}))(-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}})}(-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }})}\cdot e_{p^{m}}\biggl(\frac{\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D701},-\unicode[STIX]{x1D709})}{ru^{2}L^{2}\unicode[STIX]{x1D6E5}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}\biggr)\nonumber\\ \displaystyle & & \displaystyle \times \,e_{p^{m}}\biggl(-\frac{\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}^{\prime }}(\unicode[STIX]{x1D701}^{\prime },-\unicode[STIX]{x1D709}^{\prime })}{ru^{2}L^{2}\unicode[STIX]{x1D6E5}\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2}}\biggr)\cdot \unicode[STIX]{x1D712}(\ast ).\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle S_{2} & = & \displaystyle \sum _{r(p^{m})}e_{p^{m}}(r(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}))(-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}})}(-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }})}\cdot e_{p^{m}}\biggl(\frac{\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D701},-\unicode[STIX]{x1D709})}{ru^{2}L^{2}\unicode[STIX]{x1D6E5}\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}\biggr)\nonumber\\ \displaystyle & & \displaystyle \times \,e_{p^{m}}\biggl(-\frac{\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}^{\prime }}(\unicode[STIX]{x1D701}^{\prime },-\unicode[STIX]{x1D709}^{\prime })}{ru^{2}L^{2}\unicode[STIX]{x1D6E5}\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2}}\biggr)\cdot \unicode[STIX]{x1D712}(\ast ).\end{eqnarray}$$
We can bound
![]() $S_{1}$
directly from (7.23):
$S_{1}$
directly from (7.23):
We note that
![]() $(-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}})},(-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }})}$
are multiplicative over
$(-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}})},(-1)^{\unicode[STIX]{x1D710}(ru^{2}L^{2}\mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }})}$
are multiplicative over
![]() $r$
. Moreover, from (7.14) and (7.16), we observe that with all other parameters fixed,
$r$
. Moreover, from (7.14) and (7.16), we observe that with all other parameters fixed,
![]() $\unicode[STIX]{x1D712}$
is a periodic function over
$\unicode[STIX]{x1D712}$
is a periodic function over
![]() $r$
with period dividing
$r$
with period dividing
![]() $(L,p^{m})$
. Therefore the function
$(L,p^{m})$
. Therefore the function
![]() $\unicode[STIX]{x1D712}(\ast )$
can be viewed as a function on
$\unicode[STIX]{x1D712}(\ast )$
can be viewed as a function on
![]() $(\mathbb{Z}/(p^{m},L)\mathbb{Z})^{\ast }$
bounded by
$(\mathbb{Z}/(p^{m},L)\mathbb{Z})^{\ast }$
bounded by
![]() $1$
, so can be written as at most
$1$
, so can be written as at most
![]() $(p^{m},L)$
linearly combined multiplicative characters on
$(p^{m},L)$
linearly combined multiplicative characters on
![]() $\mathbb{Z}/p^{m}\mathbb{Z}$
with coefficients bounded by 1. Therefore, the factor
$\mathbb{Z}/p^{m}\mathbb{Z}$
with coefficients bounded by 1. Therefore, the factor
![]() $S_{2}$
is a combination of at most
$S_{2}$
is a combination of at most
![]() $(p^{m},L)$
Kloosterman–Salié sums.
$(p^{m},L)$
Kloosterman–Salié sums.
If
![]() $\mathfrak{d}_{\unicode[STIX]{x1D6FE}}\neq \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
, applying Kloosterman’s elementary
$\mathfrak{d}_{\unicode[STIX]{x1D6FE}}\neq \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
, applying Kloosterman’s elementary
![]() $3/4$
bound for this type of sum [Reference ZhangZha14, Lemma 3.4.1], we obtain
$3/4$
bound for this type of sum [Reference ZhangZha14, Lemma 3.4.1], we obtain
If
![]() $\mathfrak{d}_{\unicode[STIX]{x1D6FE}}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
but
$\mathfrak{d}_{\unicode[STIX]{x1D6FE}}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
but
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D701},-\unicode[STIX]{x1D709})\neq \mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(\unicode[STIX]{x1D701}^{\prime },-\unicode[STIX]{x1D709}^{\prime })$
, then we can use the last two factors in the summand of (7.24) to obtain a bound for
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D701},-\unicode[STIX]{x1D709})\neq \mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(\unicode[STIX]{x1D701}^{\prime },-\unicode[STIX]{x1D709}^{\prime })$
, then we can use the last two factors in the summand of (7.24) to obtain a bound for
![]() $S_{2}$
. It can be checked that if
$S_{2}$
. It can be checked that if
![]() $S_{2}\neq 0$
, then the condition that
$S_{2}\neq 0$
, then the condition that
![]() $\unicode[STIX]{x1D712}(r;\ast )=1$
in (7.24) leads to
$\unicode[STIX]{x1D712}(r;\ast )=1$
in (7.24) leads to
Therefore, the elementary Kloosterman
![]() $3/4$
bound in this case gives
$3/4$
bound in this case gives
Collecting (7.25)–(7.27), using the multiplicativity of
![]() ${\mathcal{S}}(q,u,r,\unicode[STIX]{x1D6FE},\unicode[STIX]{x1D709},\unicode[STIX]{x1D701},\unicode[STIX]{x1D6FE}^{\prime },\unicode[STIX]{x1D709}^{\prime },\unicode[STIX]{x1D701}^{\prime })$
, and absorbing
${\mathcal{S}}(q,u,r,\unicode[STIX]{x1D6FE},\unicode[STIX]{x1D709},\unicode[STIX]{x1D701},\unicode[STIX]{x1D6FE}^{\prime },\unicode[STIX]{x1D709}^{\prime },\unicode[STIX]{x1D701}^{\prime })$
, and absorbing
![]() $\unicode[STIX]{x1D6E5},L$
in the
$\unicode[STIX]{x1D6E5},L$
in the
![]() $\ll$
relation, we obtain the following two lemmas in the case where
$\ll$
relation, we obtain the following two lemmas in the case where
![]() $q$
is odd.
$q$
is odd.
Lemma 7.4. If
![]() $\mathfrak{d}_{\unicode[STIX]{x1D6FE}}\neq \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
, then
$\mathfrak{d}_{\unicode[STIX]{x1D6FE}}\neq \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
, then
Lemma 7.5. If
![]() $\mathfrak{d}_{\unicode[STIX]{x1D6FE}}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
and
$\mathfrak{d}_{\unicode[STIX]{x1D6FE}}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
and
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D701},-\unicode[STIX]{x1D709})\neq \mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(\unicode[STIX]{x1D701}^{\prime },-\unicode[STIX]{x1D709}^{\prime })$
, then
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D701},-\unicode[STIX]{x1D709})\neq \mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(\unicode[STIX]{x1D701}^{\prime },-\unicode[STIX]{x1D709}^{\prime })$
, then
We briefly explain how to extend Lemmas 7.1–7.5 when
![]() $q$
is even. It is enough to consider
$q$
is even. It is enough to consider
![]() $q=2^{m}$
by multiplicativity. The extra complication arises in Lemma 7.1 when we complete squares for some exponential sums (e.g.
$q=2^{m}$
by multiplicativity. The extra complication arises in Lemma 7.1 when we complete squares for some exponential sums (e.g.
![]() $\sum _{x=0}^{7}e_{8}(x^{2}+x)$
): we encounter certain ‘restricted’ Gauss sums, meaning the sum index is restricted to certain congruence classes
$\sum _{x=0}^{7}e_{8}(x^{2}+x)$
): we encounter certain ‘restricted’ Gauss sums, meaning the sum index is restricted to certain congruence classes
![]() $\text{mod}\;2$
. This slightly alters the statement of Lemma 7.1 for
$\text{mod}\;2$
. This slightly alters the statement of Lemma 7.1 for
![]() $q=2^{m}$
. We can handle this by writing an indicator function of the allowed congruence classes. In Lemmas 7.3–7.5, we can handle the extra indicator function by writing it as a linear combination of two additive characters to the modulus 2. We obtain a linear combination of more Kloosterman–Salié sums in Lemmas 7.4 and 7.5, and this eventually gives an extra constant factor to the bound on
$q=2^{m}$
. We can handle this by writing an indicator function of the allowed congruence classes. In Lemmas 7.3–7.5, we can handle the extra indicator function by writing it as a linear combination of two additive characters to the modulus 2. We obtain a linear combination of more Kloosterman–Salié sums in Lemmas 7.4 and 7.5, and this eventually gives an extra constant factor to the bound on
![]() $|S_{2}|$
. The rest of the proof is the same.
$|S_{2}|$
. The rest of the proof is the same.
7.2 Minor arc analysis, part I
We begin by estimating
![]() ${\mathcal{I}}_{1}$
. First we take the Fourier transform of
${\mathcal{I}}_{1}$
. First we take the Fourier transform of
![]() ${\mathcal{R}}_{N}^{U}$
(defined at (4.8)):
${\mathcal{R}}_{N}^{U}$
(defined at (4.8)):
where
We will first give an
![]() $L^{\infty }$
bound for
$L^{\infty }$
bound for
![]() ${\mathcal{R}}_{u,\unicode[STIX]{x1D6FE}}$
(see (7.36)).
${\mathcal{R}}_{u,\unicode[STIX]{x1D6FE}}$
(see (7.36)).
Write
![]() $\unicode[STIX]{x1D703}=r/q+\unicode[STIX]{x1D6FD}$
and rearrange the order of
$\unicode[STIX]{x1D703}=r/q+\unicode[STIX]{x1D6FD}$
and rearrange the order of
![]() $x,y$
according to the congruence classes
$x,y$
according to the congruence classes
![]() $\text{mod}\;q$
:
$\text{mod}\;q$
:
 $$\begin{eqnarray}\displaystyle {\mathcal{R}}_{u,\unicode[STIX]{x1D6FE}}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr) & = & \displaystyle \mathop{\sum }_{x_{0},y_{0}(q)}e\biggl(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux_{0}+uu^{\ast },Luy_{0})\frac{r}{q}\biggr)\nonumber\\ \displaystyle & & \displaystyle \cdot \,\biggl[\mathop{\sum }_{\substack{ x\equiv x_{0}(q) \\ y\equiv y_{0}(q)}}\unicode[STIX]{x1D713}\biggl(\frac{Lux+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Luy}{X}\biggr)e(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },Luy)\unicode[STIX]{x1D6FD})\biggr].\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{R}}_{u,\unicode[STIX]{x1D6FE}}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr) & = & \displaystyle \mathop{\sum }_{x_{0},y_{0}(q)}e\biggl(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux_{0}+uu^{\ast },Luy_{0})\frac{r}{q}\biggr)\nonumber\\ \displaystyle & & \displaystyle \cdot \,\biggl[\mathop{\sum }_{\substack{ x\equiv x_{0}(q) \\ y\equiv y_{0}(q)}}\unicode[STIX]{x1D713}\biggl(\frac{Lux+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Luy}{X}\biggr)e(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },Luy)\unicode[STIX]{x1D6FD})\biggr].\end{eqnarray}$$
Applying Poisson summation to the
![]() $x,y$
sum in the bracket
$x,y$
sum in the bracket
![]() $[\cdot ]$
, we obtain
$[\cdot ]$
, we obtain
 $$\begin{eqnarray}\displaystyle [\cdot ] & = & \displaystyle \mathop{\sum }_{\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}\in \mathbb{Z}}\int _{-\infty }^{\infty }\int _{-\infty }^{\infty }\unicode[STIX]{x1D713}\biggl(\frac{Lu(x_{0}+qx)+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Lu(y_{0}+qy)}{X}\biggr)\nonumber\\ \displaystyle & & \displaystyle \cdot \,e(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lu(x_{0}+qx)+uu^{\ast },Lu(y_{0}+qy))\unicode[STIX]{x1D6FD})e(-x\unicode[STIX]{x1D709}-y\unicode[STIX]{x1D701})\,dx\,dy\nonumber\\ \displaystyle & = & \displaystyle \frac{X^{2}}{q^{2}L^{2}u^{2}}\mathop{\sum }_{\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}\in \mathbb{Z}}\int _{-\infty }^{\infty }\int _{-\infty }^{\infty }\unicode[STIX]{x1D713}(x)\unicode[STIX]{x1D713}(y)e\biggl(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Xx,Xy)\unicode[STIX]{x1D6FD}-\frac{X\unicode[STIX]{x1D709}}{quL}x-\frac{X\unicode[STIX]{x1D701}}{quL}y\biggr)e\biggl(\frac{u^{\ast }\unicode[STIX]{x1D709}}{Lq}\biggr)\,dx\,dy\nonumber\\ \displaystyle & & \displaystyle \cdot \,e_{q}(x_{0}\unicode[STIX]{x1D709}+y_{0}\unicode[STIX]{x1D701}).\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle [\cdot ] & = & \displaystyle \mathop{\sum }_{\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}\in \mathbb{Z}}\int _{-\infty }^{\infty }\int _{-\infty }^{\infty }\unicode[STIX]{x1D713}\biggl(\frac{Lu(x_{0}+qx)+uu^{\ast }}{X}\biggr)\unicode[STIX]{x1D713}\biggl(\frac{Lu(y_{0}+qy)}{X}\biggr)\nonumber\\ \displaystyle & & \displaystyle \cdot \,e(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lu(x_{0}+qx)+uu^{\ast },Lu(y_{0}+qy))\unicode[STIX]{x1D6FD})e(-x\unicode[STIX]{x1D709}-y\unicode[STIX]{x1D701})\,dx\,dy\nonumber\\ \displaystyle & = & \displaystyle \frac{X^{2}}{q^{2}L^{2}u^{2}}\mathop{\sum }_{\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}\in \mathbb{Z}}\int _{-\infty }^{\infty }\int _{-\infty }^{\infty }\unicode[STIX]{x1D713}(x)\unicode[STIX]{x1D713}(y)e\biggl(\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Xx,Xy)\unicode[STIX]{x1D6FD}-\frac{X\unicode[STIX]{x1D709}}{quL}x-\frac{X\unicode[STIX]{x1D701}}{quL}y\biggr)e\biggl(\frac{u^{\ast }\unicode[STIX]{x1D709}}{Lq}\biggr)\,dx\,dy\nonumber\\ \displaystyle & & \displaystyle \cdot \,e_{q}(x_{0}\unicode[STIX]{x1D709}+y_{0}\unicode[STIX]{x1D701}).\end{eqnarray}$$
Plugging (7.31) back into (7.30), we have
where
and
Note that the sum in (7.32) is principally supported on a few terms, since the
![]() ${\mathcal{J}}_{\unicode[STIX]{x1D6FE}}$
term decays quickly. We will use non-stationary and stationary phase methods to give bounds for the
${\mathcal{J}}_{\unicode[STIX]{x1D6FE}}$
term decays quickly. We will use non-stationary and stationary phase methods to give bounds for the
![]() ${\mathcal{J}}_{\unicode[STIX]{x1D6FE}}$
terms. We review the statements here, for reference.
${\mathcal{J}}_{\unicode[STIX]{x1D6FE}}$
terms. We review the statements here, for reference.
Proposition 7.6 [Reference ZhangZha18b, p. 94, Non-stationary phase].
Let
![]() $\unicode[STIX]{x1D719}$
be a smooth compactly supported function on
$\unicode[STIX]{x1D719}$
be a smooth compactly supported function on
![]() $(-\infty ,\infty )$
and
$(-\infty ,\infty )$
and
![]() $f$
be a function which, for
$f$
be a function which, for
![]() $x$
in the support of
$x$
in the support of
![]() $\unicode[STIX]{x1D719}$
, satisfies:
$\unicode[STIX]{x1D719}$
, satisfies:
(i)
 $|f^{\prime }(x)|>A>0$
;
$|f^{\prime }(x)|>A>0$
;(ii)
 $A\geqslant |f^{(2)}(x)|,\ldots ,|f^{(n)}(x)|$
.
$A\geqslant |f^{(2)}(x)|,\ldots ,|f^{(n)}(x)|$
.
Then
Proposition 7.7 [Reference ZhangZha18b, p. 95, Stationary phase].
Let
![]() $f$
be a quadratic polynomial of two variables
$f$
be a quadratic polynomial of two variables
![]() $x$
and
$x$
and
![]() $y$
whose homogeneous part has discriminant
$y$
whose homogeneous part has discriminant
![]() $-D$
with
$-D$
with
![]() $D>0$
. Let
$D>0$
. Let
![]() $\unicode[STIX]{x1D719}(x,y)$
be a smooth compactly supported function on
$\unicode[STIX]{x1D719}(x,y)$
be a smooth compactly supported function on
![]() $\mathbb{R}^{2}$
. Then
$\mathbb{R}^{2}$
. Then
We apply the non-stationary phase to
![]() ${\mathcal{J}}_{\unicode[STIX]{x1D6FE}}$
. We can obtain a bound
${\mathcal{J}}_{\unicode[STIX]{x1D6FE}}$
. We can obtain a bound
![]() $A$
as required in the statement by taking
$A$
as required in the statement by taking
(Note that the discriminant of
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
is bounded above by
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}$
is bounded above by
![]() $T^{4}$
.) Using the former, for example, the value of
$T^{4}$
.) Using the former, for example, the value of
![]() $A$
is then
$A$
is then
![]() $XU/quL$
, which is greater than
$XU/quL$
, which is greater than
![]() $1$
since
$1$
since
![]() $u<U$
,
$u<U$
,
![]() $q<Q_{0}$
and by (4.1) and (5.1).
$q<Q_{0}$
and by (4.1) and (5.1).
Therefore, the main contribution of the
![]() $\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}$
sum in (7.32) comes from the
$\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}$
sum in (7.32) comes from the
![]() $\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}$
terms such that
$\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}$
terms such that
![]() $X\unicode[STIX]{x1D709}/qu\ll T^{2}X^{2}|\unicode[STIX]{x1D6FD}|$
and
$X\unicode[STIX]{x1D709}/qu\ll T^{2}X^{2}|\unicode[STIX]{x1D6FD}|$
and
![]() $X\unicode[STIX]{x1D701}/qu\ll T^{2}X^{2}|\unicode[STIX]{x1D6FD}|$
, or in other words the terms
$X\unicode[STIX]{x1D701}/qu\ll T^{2}X^{2}|\unicode[STIX]{x1D6FD}|$
, or in other words the terms
![]() $\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}$
such that
$\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}$
such that
where we used
![]() $|\unicode[STIX]{x1D6FD}|\leqslant 1/qJ$
and
$|\unicode[STIX]{x1D6FD}|\leqslant 1/qJ$
and
![]() $J=T^{2}X$
(by (4.11)).
$J=T^{2}X$
(by (4.11)).
For the terms
![]() $\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}\ll u$
, we have an upper bound for
$\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}\ll u$
, we have an upper bound for
![]() ${\mathcal{J}}_{\unicode[STIX]{x1D6FE}}$
using the stationary phase:
${\mathcal{J}}_{\unicode[STIX]{x1D6FE}}$
using the stationary phase:
Lemma 7.3 and (7.35) together lead to a bound for
![]() ${\mathcal{R}}_{u,\unicode[STIX]{x1D6FE}}(r/q+\unicode[STIX]{x1D6FD})$
and hence for
${\mathcal{R}}_{u,\unicode[STIX]{x1D6FE}}(r/q+\unicode[STIX]{x1D6FD})$
and hence for
![]() $\widehat{{\mathcal{R}}}_{N}^{U}(r/q+\unicode[STIX]{x1D6FD})$
:
$\widehat{{\mathcal{R}}}_{N}^{U}(r/q+\unicode[STIX]{x1D6FD})$
:
Now we are ready to give an estimate for
![]() ${\mathcal{I}}_{1}$
. We rewrite
${\mathcal{I}}_{1}$
. We rewrite
![]() ${\mathcal{I}}_{1}$
as
${\mathcal{I}}_{1}$
as
We now split the integral
![]() $\int _{-1/qJ}^{1/qJ}$
above into three parts
$\int _{-1/qJ}^{1/qJ}$
above into three parts
![]() $\int _{-K_{0}/N}^{K_{0}/N}$
,
$\int _{-K_{0}/N}^{K_{0}/N}$
,
![]() $\int _{K_{0}/N}^{1/qJ}$
and
$\int _{K_{0}/N}^{1/qJ}$
and
![]() $\int _{-1/qJ}^{-K_{0}/N}$
. For each integral we use (7.36) to bound the
$\int _{-1/qJ}^{-K_{0}/N}$
. For each integral we use (7.36) to bound the
![]() $\widehat{{\mathcal{R}}}_{N}^{U}$
term. In the first integral, we use
$\widehat{{\mathcal{R}}}_{N}^{U}$
term. In the first integral, we use
and in the second and third integral, we trivially bound
![]() $|(1-\mathfrak{T}(r/q+\unicode[STIX]{x1D6FD}))|^{2}$
above by 1. Altogether, we have the following result.
$|(1-\mathfrak{T}(r/q+\unicode[STIX]{x1D6FD}))|^{2}$
above by 1. Altogether, we have the following result.
Lemma 7.8.
Since
![]() $K_{0}\gg Q_{0}^{3}\gg Q_{0}^{2}U^{2}N^{\unicode[STIX]{x1D716}}$
(see (7.53)), we have
$K_{0}\gg Q_{0}^{3}\gg Q_{0}^{2}U^{2}N^{\unicode[STIX]{x1D716}}$
(see (7.53)), we have
![]() ${\mathcal{I}}_{1}\ll T^{4\unicode[STIX]{x1D6FF}-4}N^{1-\unicode[STIX]{x1D716}}$
.
${\mathcal{I}}_{1}\ll T^{4\unicode[STIX]{x1D6FF}-4}N^{1-\unicode[STIX]{x1D716}}$
.
7.3 Minor arc analysis, part II
In this section we give an upper bound for
for
![]() $Q_{0}<Q<X$
and show the following lemma.
$Q_{0}<Q<X$
and show the following lemma.
Lemma 7.9.
Proof. Going back to (7.28), we apply the Cauchy–Schwarz inequality to the
![]() $u$
sum to get an upper bound for
$u$
sum to get an upper bound for
![]() $\widehat{{\mathcal{R}}}_{N}^{U}(r/q+\unicode[STIX]{x1D6FD})$
:
$\widehat{{\mathcal{R}}}_{N}^{U}(r/q+\unicode[STIX]{x1D6FD})$
:
Using (7.32), we obtain
 $$\begin{eqnarray}\displaystyle \sum _{r(q)}\biggl|\widehat{{\mathcal{R}}}_{N}^{U}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr)\biggr|^{2} & \ll & \displaystyle U\mathop{\sum }_{u<U}\frac{X^{4}}{u^{4}}\mathop{\sum }_{\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}\in \mathbb{Z}}\mathop{\sum }_{\unicode[STIX]{x1D709}^{\prime },\unicode[STIX]{x1D701}^{\prime }\in \mathbb{Z}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T}}{\mathcal{S}}(q,u,\unicode[STIX]{x1D6FE},\unicode[STIX]{x1D709},\unicode[STIX]{x1D701},\unicode[STIX]{x1D6FE}^{\prime },\unicode[STIX]{x1D709}^{\prime },\unicode[STIX]{x1D701}^{\prime })\nonumber\\ \displaystyle & & \displaystyle \cdot \,{\mathcal{J}}_{\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D6FD};q,u,\unicode[STIX]{x1D709},\unicode[STIX]{x1D701})\overline{{\mathcal{J}}_{\unicode[STIX]{x1D6FE}^{\prime }}(\unicode[STIX]{x1D6FD};q,u,\unicode[STIX]{x1D709}^{\prime },\unicode[STIX]{x1D701}^{\prime })}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \sum _{r(q)}\biggl|\widehat{{\mathcal{R}}}_{N}^{U}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr)\biggr|^{2} & \ll & \displaystyle U\mathop{\sum }_{u<U}\frac{X^{4}}{u^{4}}\mathop{\sum }_{\unicode[STIX]{x1D709},\unicode[STIX]{x1D701}\in \mathbb{Z}}\mathop{\sum }_{\unicode[STIX]{x1D709}^{\prime },\unicode[STIX]{x1D701}^{\prime }\in \mathbb{Z}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T}}{\mathcal{S}}(q,u,\unicode[STIX]{x1D6FE},\unicode[STIX]{x1D709},\unicode[STIX]{x1D701},\unicode[STIX]{x1D6FE}^{\prime },\unicode[STIX]{x1D709}^{\prime },\unicode[STIX]{x1D701}^{\prime })\nonumber\\ \displaystyle & & \displaystyle \cdot \,{\mathcal{J}}_{\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D6FD};q,u,\unicode[STIX]{x1D709},\unicode[STIX]{x1D701})\overline{{\mathcal{J}}_{\unicode[STIX]{x1D6FE}^{\prime }}(\unicode[STIX]{x1D6FD};q,u,\unicode[STIX]{x1D709}^{\prime },\unicode[STIX]{x1D701}^{\prime })}.\end{eqnarray}$$
By the non-stationary phase, the main contribution to (7.41) comes from the terms
![]() $\unicode[STIX]{x1D709},\unicode[STIX]{x1D701},\unicode[STIX]{x1D709}^{\prime },\unicode[STIX]{x1D701}^{\prime }\ll U$
, and for these terms, we have
$\unicode[STIX]{x1D709},\unicode[STIX]{x1D701},\unicode[STIX]{x1D709}^{\prime },\unicode[STIX]{x1D701}^{\prime }\ll U$
, and for these terms, we have
Using Lemma 7.4 together with (7.42), we obtain
 $$\begin{eqnarray}\displaystyle & & \displaystyle \sum _{r(q)}\biggl|\widehat{{\mathcal{R}}}_{N}^{U}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr)\biggr|^{2}\nonumber\\ \displaystyle & & \displaystyle \quad \ll \,\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T}}U^{6}X^{4}q^{-5/4+\unicode[STIX]{x1D716}}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})^{1/4}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})^{1/2}\min \bigg\{1,\frac{1}{T^{4}X^{4}\unicode[STIX]{x1D6FD}^{2}}\bigg\}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \sum _{r(q)}\biggl|\widehat{{\mathcal{R}}}_{N}^{U}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr)\biggr|^{2}\nonumber\\ \displaystyle & & \displaystyle \quad \ll \,\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T}}U^{6}X^{4}q^{-5/4+\unicode[STIX]{x1D716}}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})^{1/4}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})^{1/2}\min \bigg\{1,\frac{1}{T^{4}X^{4}\unicode[STIX]{x1D6FD}^{2}}\bigg\}.\end{eqnarray}$$
Observe that
![]() $qJ\leqslant T^{2}X^{2}$
, so that
$qJ\leqslant T^{2}X^{2}$
, so that
Plugging (7.43) and (7.44) into (7.39), we obtain
We split (7.45) into two parts
![]() ${\mathcal{I}}_{Q}^{(=)}$
and
${\mathcal{I}}_{Q}^{(=)}$
and
![]() ${\mathcal{I}}_{Q}^{(\neq )}$
according to whether
${\mathcal{I}}_{Q}^{(\neq )}$
according to whether
![]() $\mathfrak{d}_{\unicode[STIX]{x1D6FE}}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
or not. We first estimate
$\mathfrak{d}_{\unicode[STIX]{x1D6FE}}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
or not. We first estimate
![]() ${\mathcal{I}}_{Q}^{(=)}$
:
${\mathcal{I}}_{Q}^{(=)}$
:
 $$\begin{eqnarray}\displaystyle {\mathcal{I}}_{Q}^{(=)} & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{QT^{2}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{QT^{2}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}d\mathop{\sum }_{Q<q\leqslant 2Q}\mathbf{1}\{d|q\}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{QT^{2}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}Q\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}T^{2\unicode[STIX]{x1D6FF}}}{T^{2}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}1\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{1+\unicode[STIX]{x1D716}}U^{6}T^{4\unicode[STIX]{x1D6FF}-4}}{T^{\unicode[STIX]{x1D702}_{0}}},\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{I}}_{Q}^{(=)} & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{QT^{2}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{QT^{2}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}d\mathop{\sum }_{Q<q\leqslant 2Q}\mathbf{1}\{d|q\}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{QT^{2}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}Q\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}T^{2\unicode[STIX]{x1D6FF}}}{T^{2}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}1\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{1+\unicode[STIX]{x1D716}}U^{6}T^{4\unicode[STIX]{x1D6FF}-4}}{T^{\unicode[STIX]{x1D702}_{0}}},\end{eqnarray}$$
where we have bounded the number of divisors of
![]() $\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}$
by
$\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}$
by
![]() $N^{\unicode[STIX]{x1D716}}$
and in the last step we used Lemma 5.1 to estimate the sum (using modulus
$N^{\unicode[STIX]{x1D716}}$
and in the last step we used Lemma 5.1 to estimate the sum (using modulus
![]() $T$
in the statement of the lemma), with reference to (4.1).
$T$
in the statement of the lemma), with reference to (4.1).
Now we estimate
![]() ${\mathcal{I}}_{Q}^{(\neq )}$
. We introduce a new parameter
${\mathcal{I}}_{Q}^{(\neq )}$
. We introduce a new parameter
![]() $H$
and we further split
$H$
and we further split
![]() ${\mathcal{I}}_{Q}^{(\neq )}$
into
${\mathcal{I}}_{Q}^{(\neq )}$
into
![]() ${\mathcal{I}}_{Q}^{(\neq ,\geqslant )}$
and
${\mathcal{I}}_{Q}^{(\neq ,\geqslant )}$
and
![]() ${\mathcal{I}}_{Q}^{(\neq ,<)}$
according to
${\mathcal{I}}_{Q}^{(\neq ,<)}$
according to
![]() $(\mathfrak{d}_{\unicode[STIX]{x1D6FE}},\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})\geqslant H$
or not.
$(\mathfrak{d}_{\unicode[STIX]{x1D6FE}},\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})\geqslant H$
or not.
We first estimate
![]() ${\mathcal{I}}_{Q}^{(\neq ,\geqslant )}$
. Recall (7.43). Then we have
${\mathcal{I}}_{Q}^{(\neq ,\geqslant )}$
. Recall (7.43). Then we have
 $$\begin{eqnarray}\displaystyle {\mathcal{I}}_{Q}^{(\neq ,\geqslant )} & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{QT^{2}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ (\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }},\mathfrak{d}_{\unicode[STIX]{x1D6FE}})\geqslant H}}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})^{1/2}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{QT^{2}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ h|\mathfrak{d}_{\unicode[STIX]{x1D6FE}} \\ h\geqslant H}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}\equiv \mathfrak{d}_{\unicode[STIX]{x1D6FE}}(h)}}\mathop{\sum }_{q_{1}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}\mathop{\sum }_{q_{2}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2}}(q_{1}q_{2})^{1/2}\mathop{\sum }_{Q<q\leqslant 2Q}\mathbf{1}\{[q_{1},q_{2}]|q\}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{I}}_{Q}^{(\neq ,\geqslant )} & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{QT^{2}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ (\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }},\mathfrak{d}_{\unicode[STIX]{x1D6FE}})\geqslant H}}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})^{1/2}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{QT^{2}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ h|\mathfrak{d}_{\unicode[STIX]{x1D6FE}} \\ h\geqslant H}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}\equiv \mathfrak{d}_{\unicode[STIX]{x1D6FE}}(h)}}\mathop{\sum }_{q_{1}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}\mathop{\sum }_{q_{2}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2}}(q_{1}q_{2})^{1/2}\mathop{\sum }_{Q<q\leqslant 2Q}\mathbf{1}\{[q_{1},q_{2}]|q\}.\end{eqnarray}$$
Notice that
![]() $[q_{1},q_{2}]\geqslant (q_{1}q_{2})^{1/2}$
. Therefore,
$[q_{1},q_{2}]\geqslant (q_{1}q_{2})^{1/2}$
. Therefore,
 $$\begin{eqnarray}(7.47)\ll \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{T^{2}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ h|\mathfrak{d}_{\unicode[STIX]{x1D6FE}} \\ h\geqslant H}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}\equiv \mathfrak{d}_{\unicode[STIX]{x1D6FE}}(h)}}1.\end{eqnarray}$$
$$\begin{eqnarray}(7.47)\ll \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{T^{2}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ h|\mathfrak{d}_{\unicode[STIX]{x1D6FE}} \\ h\geqslant H}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}\equiv \mathfrak{d}_{\unicode[STIX]{x1D6FE}}(h)}}1.\end{eqnarray}$$
Again using Lemma 5.1, we have
 $$\begin{eqnarray}(7.48)\ll \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{T^{2}H^{\unicode[STIX]{x1D702}_{0}}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ h|\mathfrak{d}_{\unicode[STIX]{x1D6FE}} \\ h\geqslant H}}1\ll \frac{N^{1+\unicode[STIX]{x1D716}}U^{6}T^{4\unicode[STIX]{x1D6FF}-4}}{H^{\unicode[STIX]{x1D702}_{0}}}.\end{eqnarray}$$
$$\begin{eqnarray}(7.48)\ll \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{T^{2}H^{\unicode[STIX]{x1D702}_{0}}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ h|\mathfrak{d}_{\unicode[STIX]{x1D6FE}} \\ h\geqslant H}}1\ll \frac{N^{1+\unicode[STIX]{x1D716}}U^{6}T^{4\unicode[STIX]{x1D6FF}-4}}{H^{\unicode[STIX]{x1D702}_{0}}}.\end{eqnarray}$$
Now we estimate
![]() ${\mathcal{I}}_{Q}^{(\neq ,<)}$
. Using (7.43) and replacing
${\mathcal{I}}_{Q}^{(\neq ,<)}$
. Using (7.43) and replacing
![]() $(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2},(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})^{1/2}$
by
$(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2},(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})^{1/2}$
by
![]() $(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}),(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})$
, we have
$(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}),(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})$
, we have
 $$\begin{eqnarray}\displaystyle {\mathcal{I}}_{Q}^{(\neq ,<)} & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{T^{2}Q^{5/4}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ (\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }},\mathfrak{d}_{\unicode[STIX]{x1D6FE}})<H}}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})^{1/4}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}})(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{T^{2}Q^{5/4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{d_{1}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}\mathop{\sum }_{\substack{ d_{3}\leqslant 2Q}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{\prime }\equiv \mathfrak{d}_{\unicode[STIX]{x1D6FE}}(d_{3})}}\mathop{\sum }_{d_{2}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}}d_{1}d_{2}d_{3}^{1/4}\mathop{\sum }_{Q<q\leqslant 2Q}\mathbf{1}\{[d_{1},d_{2},d_{3}]|q\}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{I}}_{Q}^{(\neq ,<)} & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{T^{2}Q^{5/4}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ (\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }},\mathfrak{d}_{\unicode[STIX]{x1D6FE}})<H}}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})^{1/4}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}})(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}}{T^{2}Q^{5/4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{d_{1}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}\mathop{\sum }_{\substack{ d_{3}\leqslant 2Q}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{\prime }\equiv \mathfrak{d}_{\unicode[STIX]{x1D6FE}}(d_{3})}}\mathop{\sum }_{d_{2}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}}d_{1}d_{2}d_{3}^{1/4}\mathop{\sum }_{Q<q\leqslant 2Q}\mathbf{1}\{[d_{1},d_{2},d_{3}]|q\}.\end{eqnarray}$$
Writing
![]() $h=(\mathfrak{d}_{\unicode[STIX]{x1D6FE}},\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})$
, then
$h=(\mathfrak{d}_{\unicode[STIX]{x1D6FE}},\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})$
, then
![]() $d_{1}/h,d_{2}/h,d_{3}/h$
are mutually relatively prime. Since
$d_{1}/h,d_{2}/h,d_{3}/h$
are mutually relatively prime. Since
![]() $h<H$
, we have the estimate
$h<H$
, we have the estimate
Therefore,
 $$\begin{eqnarray}\displaystyle (7.50) & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}H^{2}}{T^{2}Q^{1/4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{d_{1}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}\mathop{\sum }_{\substack{ d_{3}\leqslant 2Q}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{\prime }\equiv \mathfrak{d}_{\unicode[STIX]{x1D6FE}}(d_{3})}}\mathop{\sum }_{d_{2}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}}d_{3}^{-3/4}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}H^{2}T^{4\unicode[STIX]{x1D6FF}}}{T^{2}Q^{1/4}}\mathop{\sum }_{d_{3}\leqslant 2Q}d_{3}^{-3/4-\unicode[STIX]{x1D702}_{0}}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{1+\unicode[STIX]{x1D716}}U^{6}H^{2}T^{4\unicode[STIX]{x1D6FF}-4}}{Q^{\unicode[STIX]{x1D702}_{0}}}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle (7.50) & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}H^{2}}{T^{2}Q^{1/4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{d_{1}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}\mathop{\sum }_{\substack{ d_{3}\leqslant 2Q}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{\prime }\equiv \mathfrak{d}_{\unicode[STIX]{x1D6FE}}(d_{3})}}\mathop{\sum }_{d_{2}|\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}}d_{3}^{-3/4}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{6}X^{2}H^{2}T^{4\unicode[STIX]{x1D6FF}}}{T^{2}Q^{1/4}}\mathop{\sum }_{d_{3}\leqslant 2Q}d_{3}^{-3/4-\unicode[STIX]{x1D702}_{0}}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{1+\unicode[STIX]{x1D716}}U^{6}H^{2}T^{4\unicode[STIX]{x1D6FF}-4}}{Q^{\unicode[STIX]{x1D702}_{0}}}.\end{eqnarray}$$
To make the terms at (7.46), (7.49), (7.52), and later on at (7.69)
![]() $\ll T^{4\unicode[STIX]{x1D6FF}-4}N^{1-\unicode[STIX]{x1D702}}$
for an appropriate positive
$\ll T^{4\unicode[STIX]{x1D6FF}-4}N^{1-\unicode[STIX]{x1D702}}$
for an appropriate positive
![]() $\unicode[STIX]{x1D702}$
, we can set
$\unicode[STIX]{x1D702}$
, we can set
Thus we have proven Lemma 7.9. ◻
7.4 Minor arc analysis, part III
In this section we give an upper bound for
![]() ${\mathcal{I}}_{Q}$
when
${\mathcal{I}}_{Q}$
when
![]() $Q$
is large, i.e.
$Q$
is large, i.e.
![]() $X<Q\leqslant J$
. We retain all notation from the previous sections. Namely, we show the following lemma.
$X<Q\leqslant J$
. We retain all notation from the previous sections. Namely, we show the following lemma.
Lemma 7.10.
Proof. Recall
 $$\begin{eqnarray}\widehat{{\mathcal{R}}}_{N}^{U}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr)=\mathop{\sum }_{\substack{ u<U \\ (u,L)=1}}\unicode[STIX]{x1D707}(u)\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}{\mathcal{R}}_{u,\unicode[STIX]{x1D6FE}}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr),\end{eqnarray}$$
$$\begin{eqnarray}\widehat{{\mathcal{R}}}_{N}^{U}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr)=\mathop{\sum }_{\substack{ u<U \\ (u,L)=1}}\unicode[STIX]{x1D707}(u)\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}{\mathcal{R}}_{u,\unicode[STIX]{x1D6FE}}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr),\end{eqnarray}$$
where
We insert extra harmonics by writing
![]() $e_{q}(r\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },uLy))$
into its Fourier expansion:
$e_{q}(r\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },uLy))$
into its Fourier expansion:
 $$\begin{eqnarray}\displaystyle & & \displaystyle e_{q}(r\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },uLy))\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{q^{2}}\mathop{\sum }_{m(q)}\mathop{\sum }_{n(q)}\mathop{\sum }_{s(q)}\mathop{\sum }_{t(q)}e_{q}(r\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lus+uu^{\ast },Lut)+sm+tn)e\biggl(-\frac{mx}{q}-\frac{ny}{q}\biggr)\nonumber\\ \displaystyle & & \displaystyle \quad =\mathop{\sum }_{m(q)}\mathop{\sum }_{n(q)}{\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(q,u,r,m,n)e\biggl(-\frac{mx}{q}-\frac{ny}{q}\biggr).\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle e_{q}(r\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lux+uu^{\ast },uLy))\nonumber\\ \displaystyle & & \displaystyle \quad =\frac{1}{q^{2}}\mathop{\sum }_{m(q)}\mathop{\sum }_{n(q)}\mathop{\sum }_{s(q)}\mathop{\sum }_{t(q)}e_{q}(r\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(Lus+uu^{\ast },Lut)+sm+tn)e\biggl(-\frac{mx}{q}-\frac{ny}{q}\biggr)\nonumber\\ \displaystyle & & \displaystyle \quad =\mathop{\sum }_{m(q)}\mathop{\sum }_{n(q)}{\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(q,u,r,m,n)e\biggl(-\frac{mx}{q}-\frac{ny}{q}\biggr).\end{eqnarray}$$
Inserting (7.56) into (7.55), we obtain
where
Now we apply the Cauchy–Schwarz inequality to the
![]() $u$
sum for
$u$
sum for
![]() $\widehat{{\mathcal{R}}}_{N}^{U}$
(see (7.40), (7.54)), and insert it back into
$\widehat{{\mathcal{R}}}_{N}^{U}$
(see (7.40), (7.54)), and insert it back into
![]() ${\mathcal{I}}_{Q}$
at (7.39). We have
${\mathcal{I}}_{Q}$
at (7.39). We have
 $$\begin{eqnarray}\displaystyle {\mathcal{I}}_{Q} & = & \displaystyle \mathop{\sum }_{Q<q\leqslant 2Q}\sum _{r(q)}\int _{-1/qJ}^{1/qJ}\biggl|\widehat{{\mathcal{R}}}_{N}^{U}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr)\biggr|^{2}\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & \ll & \displaystyle U\mathop{\sum }_{u<U}\mathop{\sum }_{Q<q\leqslant 2Q}\sum _{r(q)}\int _{-1/qJ}^{1/qJ}\biggl|\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}{\mathcal{R}}_{u,\unicode[STIX]{x1D6FE}}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr)\biggr|^{2}\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & \ll & \displaystyle U\mathop{\sum }_{u<U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }(q)}\biggl(\sum _{r(q)}{\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(q,u,r,m,n)\overline{{\mathcal{S}}_{\unicode[STIX]{x1D6FE}^{\prime }}(q,u,r,m^{\prime },n^{\prime })}\biggr)\nonumber\\ \displaystyle & & \displaystyle \quad \cdot \,\int _{-1/qJ}^{1/qJ}\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}}\biggl(\unicode[STIX]{x1D6FD},X,u,\frac{m}{q},\frac{n}{q}\biggr)\overline{\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}^{\prime }}\biggl(\unicode[STIX]{x1D6FD},X,u,\frac{m^{\prime }}{q},\frac{n^{\prime }}{q}\biggr)}\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & = & \displaystyle U\mathop{\sum }_{u<U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }(q)}{\mathcal{S}}(q,u,\unicode[STIX]{x1D6FE},m,n,\unicode[STIX]{x1D6FE}^{\prime },m^{\prime },n^{\prime })\nonumber\\ \displaystyle & & \displaystyle \quad \cdot \,\int _{-1/qJ}^{1/qJ}\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}}\biggl(\unicode[STIX]{x1D6FD},X,u,\frac{m}{q},\frac{n}{q}\biggr)\overline{\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}^{\prime }}\biggl(\unicode[STIX]{x1D6FD},X,u,\frac{m^{\prime }}{q},\frac{n^{\prime }}{q}\biggr)}\,d\unicode[STIX]{x1D6FD}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{I}}_{Q} & = & \displaystyle \mathop{\sum }_{Q<q\leqslant 2Q}\sum _{r(q)}\int _{-1/qJ}^{1/qJ}\biggl|\widehat{{\mathcal{R}}}_{N}^{U}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr)\biggr|^{2}\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & \ll & \displaystyle U\mathop{\sum }_{u<U}\mathop{\sum }_{Q<q\leqslant 2Q}\sum _{r(q)}\int _{-1/qJ}^{1/qJ}\biggl|\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}{\mathcal{R}}_{u,\unicode[STIX]{x1D6FE}}\biggl(\frac{r}{q}+\unicode[STIX]{x1D6FD}\biggr)\biggr|^{2}\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & \ll & \displaystyle U\mathop{\sum }_{u<U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }(q)}\biggl(\sum _{r(q)}{\mathcal{S}}_{\unicode[STIX]{x1D6FE}}(q,u,r,m,n)\overline{{\mathcal{S}}_{\unicode[STIX]{x1D6FE}^{\prime }}(q,u,r,m^{\prime },n^{\prime })}\biggr)\nonumber\\ \displaystyle & & \displaystyle \quad \cdot \,\int _{-1/qJ}^{1/qJ}\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}}\biggl(\unicode[STIX]{x1D6FD},X,u,\frac{m}{q},\frac{n}{q}\biggr)\overline{\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}^{\prime }}\biggl(\unicode[STIX]{x1D6FD},X,u,\frac{m^{\prime }}{q},\frac{n^{\prime }}{q}\biggr)}\,d\unicode[STIX]{x1D6FD}\nonumber\\ \displaystyle & = & \displaystyle U\mathop{\sum }_{u<U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }(q)}{\mathcal{S}}(q,u,\unicode[STIX]{x1D6FE},m,n,\unicode[STIX]{x1D6FE}^{\prime },m^{\prime },n^{\prime })\nonumber\\ \displaystyle & & \displaystyle \quad \cdot \,\int _{-1/qJ}^{1/qJ}\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}}\biggl(\unicode[STIX]{x1D6FD},X,u,\frac{m}{q},\frac{n}{q}\biggr)\overline{\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}^{\prime }}\biggl(\unicode[STIX]{x1D6FD},X,u,\frac{m^{\prime }}{q},\frac{n^{\prime }}{q}\biggr)}\,d\unicode[STIX]{x1D6FD}.\end{eqnarray}$$
Applying Poisson summation and non-stationary phase to
![]() $\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}}$
and
$\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}}$
and
![]() $\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}^{\prime }}$
, we see that the main contribution to (7.59) comes from the terms
$\unicode[STIX]{x1D706}_{\unicode[STIX]{x1D6FE}^{\prime }}$
, we see that the main contribution to (7.59) comes from the terms
![]() $m$
,
$m$
,
![]() $n$
,
$n$
,
![]() $m^{\prime }$
,
$m^{\prime }$
,
![]() $n^{\prime }$
$n^{\prime }$
![]() $\ll qu/X$
. For these terms, we use the trivial bound:
$\ll qu/X$
. For these terms, we use the trivial bound:
From (7.59) and (7.60) we have
We split (7.61) into
![]() ${\mathcal{I}}_{Q}^{(=)}$
and
${\mathcal{I}}_{Q}^{(=)}$
and
![]() ${\mathcal{I}}_{Q}^{(\neq )}$
according to whether
${\mathcal{I}}_{Q}^{(\neq )}$
according to whether
![]() $\mathfrak{d}_{\unicode[STIX]{x1D6FE}}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
or not:
$\mathfrak{d}_{\unicode[STIX]{x1D6FE}}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}$
or not:
 $$\begin{eqnarray}{\mathcal{I}}_{Q}^{(=)}=\frac{UX^{4}}{QJ}\mathop{\sum }_{u<U}\frac{1}{u^{4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }\ll uq/X}|{\mathcal{S}}(q,u,\unicode[STIX]{x1D6FE},m,n,\unicode[STIX]{x1D6FE}^{\prime },m^{\prime },n^{\prime })|\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{I}}_{Q}^{(=)}=\frac{UX^{4}}{QJ}\mathop{\sum }_{u<U}\frac{1}{u^{4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }\ll uq/X}|{\mathcal{S}}(q,u,\unicode[STIX]{x1D6FE},m,n,\unicode[STIX]{x1D6FE}^{\prime },m^{\prime },n^{\prime })|\end{eqnarray}$$
and
 $$\begin{eqnarray}{\mathcal{I}}_{Q}^{(\neq )}=\frac{UX^{4}}{QJ}\mathop{\sum }_{u<U}\frac{1}{u^{4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}\neq \mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }\ll uq/X}|{\mathcal{S}}(q,u,\unicode[STIX]{x1D6FE},m,n,\unicode[STIX]{x1D6FE}^{\prime },m^{\prime },n^{\prime })|.\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{I}}_{Q}^{(\neq )}=\frac{UX^{4}}{QJ}\mathop{\sum }_{u<U}\frac{1}{u^{4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}\neq \mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }\ll uq/X}|{\mathcal{S}}(q,u,\unicode[STIX]{x1D6FE},m,n,\unicode[STIX]{x1D6FE}^{\prime },m^{\prime },n^{\prime })|.\end{eqnarray}$$
We first deal with
![]() ${\mathcal{I}}_{Q}^{(\neq )}$
. Using Lemma 7.4 to bound
${\mathcal{I}}_{Q}^{(\neq )}$
. Using Lemma 7.4 to bound
![]() $|{\mathcal{S}}|$
, we obtain:
$|{\mathcal{S}}|$
, we obtain:
 $$\begin{eqnarray}\displaystyle & & \displaystyle {\mathcal{I}}_{Q}^{(\neq )}\ll \nonumber\\ \displaystyle & & \displaystyle \quad \,\frac{UX^{4}}{QJ}\mathop{\sum }_{u<U}\frac{1}{u^{4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}\neq \mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }\ll uq/X}u^{4}q^{-5/4+\unicode[STIX]{x1D716}}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})^{1/4}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})^{1/2}.\nonumber\\ \displaystyle & & \displaystyle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle {\mathcal{I}}_{Q}^{(\neq )}\ll \nonumber\\ \displaystyle & & \displaystyle \quad \,\frac{UX^{4}}{QJ}\mathop{\sum }_{u<U}\frac{1}{u^{4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}\neq \mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }\ll uq/X}u^{4}q^{-5/4+\unicode[STIX]{x1D716}}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})^{1/4}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})^{1/2}.\nonumber\\ \displaystyle & & \displaystyle\end{eqnarray}$$
Bounding
![]() $(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})$
,
$(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}-\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }})$
,
![]() $(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})$
,
$(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})$
,
![]() $(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})$
by
$(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})$
by
![]() $T,T^{2},T^{2}$
respectively, we obtain
$T,T^{2},T^{2}$
respectively, we obtain
Since
![]() $Q\leqslant J=T^{2}X$
, the term in the parentheses above is
$Q\leqslant J=T^{2}X$
, the term in the parentheses above is
![]() $\ll U^{2}T^{17/4}X^{-1/4}$
, and thus we have obtained a significant power saving for
$\ll U^{2}T^{17/4}X^{-1/4}$
, and thus we have obtained a significant power saving for
![]() ${\mathcal{I}}_{Q}^{(\neq )}$
.
${\mathcal{I}}_{Q}^{(\neq )}$
.
Now we deal with
![]() ${\mathcal{I}}_{Q}^{(=)}$
. We split
${\mathcal{I}}_{Q}^{(=)}$
. We split
![]() ${\mathcal{I}}_{Q}^{(=)}$
into
${\mathcal{I}}_{Q}^{(=)}$
into
![]() ${\mathcal{I}}_{Q}^{(=,=)}$
and
${\mathcal{I}}_{Q}^{(=,=)}$
and
![]() ${\mathcal{I}}_{Q}^{(=,\neq )}$
according to whether
${\mathcal{I}}_{Q}^{(=,\neq )}$
according to whether
![]() $\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(n,-m)=\mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(n^{\prime },-m^{\prime })$
or not. We first give an upper bound for
$\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(n,-m)=\mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(n^{\prime },-m^{\prime })$
or not. We first give an upper bound for
![]() ${\mathcal{I}}_{Q}^{(=,\neq )}$
. From Lemma 7.5,
${\mathcal{I}}_{Q}^{(=,\neq )}$
. From Lemma 7.5,
 $$\begin{eqnarray}\displaystyle & & \displaystyle {\mathcal{I}}_{Q}^{(=,\neq )}\nonumber\\ \displaystyle & & \displaystyle \quad \ll \,\frac{UX^{4}}{QJ}\mathop{\sum }_{u<U}\frac{1}{u^{4}}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }\ll uq/X}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}} \\ \mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D709},-\unicode[STIX]{x1D701})\neq \mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(\unicode[STIX]{x1D709}^{\prime },-\unicode[STIX]{x1D701}^{\prime })}}\mathop{\sum }_{Q<q\leqslant 2Q}u^{4}q^{-5/4}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})^{1/2}\nonumber\\ \displaystyle & & \displaystyle \qquad \cdot \,|\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(n,-m)-\mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(n^{\prime },-m^{\prime })|^{1/4}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle {\mathcal{I}}_{Q}^{(=,\neq )}\nonumber\\ \displaystyle & & \displaystyle \quad \ll \,\frac{UX^{4}}{QJ}\mathop{\sum }_{u<U}\frac{1}{u^{4}}\mathop{\sum }_{m,n,m^{\prime },n^{\prime }\ll uq/X}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}} \\ \mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(\unicode[STIX]{x1D709},-\unicode[STIX]{x1D701})\neq \mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(\unicode[STIX]{x1D709}^{\prime },-\unicode[STIX]{x1D701}^{\prime })}}\mathop{\sum }_{Q<q\leqslant 2Q}u^{4}q^{-5/4}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})^{1/2}\nonumber\\ \displaystyle & & \displaystyle \qquad \cdot \,|\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(n,-m)-\mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(n^{\prime },-m^{\prime })|^{1/4}.\end{eqnarray}$$
We bound
![]() $(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}),(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})$
by
$(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}),(q,\mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}^{2})$
by
![]() $T^{2}$
and
$T^{2}$
and
![]() $|\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(n,-m)-\mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(n^{\prime },-m^{\prime })|$
by
$|\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(n,-m)-\mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(n^{\prime },-m^{\prime })|$
by
![]() $T^{2}u^{2}q^{2}/X^{2}$
, so that we have
$T^{2}u^{2}q^{2}/X^{2}$
, so that we have
where we have used
![]() $Q\leqslant T^{2}X$
. Again we obtain a significant power saving from (7.67).
$Q\leqslant T^{2}X$
. Again we obtain a significant power saving from (7.67).
Finally, we estimate
![]() ${\mathcal{I}}_{Q}^{(=,=)}$
. From Lemma 7.4 and (7.62), we have
${\mathcal{I}}_{Q}^{(=,=)}$
. From Lemma 7.4 and (7.62), we have
 $$\begin{eqnarray}\displaystyle & & \displaystyle {\mathcal{I}}_{Q}^{(=,=)}\nonumber\\ \displaystyle & & \displaystyle \quad \ll \,\frac{N^{\unicode[STIX]{x1D716}}UX^{4}}{QJ}\mathop{\sum }_{u<U}\frac{1}{u^{4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n\ll uq/X}\frac{(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2},q)}{q}u^{4}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{\substack{ m^{\prime },n^{\prime }\ll uq/X \\ \mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(n^{\prime },-m^{\prime })=\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(n,-m)}}1.\qquad\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle {\mathcal{I}}_{Q}^{(=,=)}\nonumber\\ \displaystyle & & \displaystyle \quad \ll \,\frac{N^{\unicode[STIX]{x1D716}}UX^{4}}{QJ}\mathop{\sum }_{u<U}\frac{1}{u^{4}}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n\ll uq/X}\frac{(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2},q)}{q}u^{4}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{\substack{ m^{\prime },n^{\prime }\ll uq/X \\ \mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(n^{\prime },-m^{\prime })=\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(n,-m)}}1.\qquad\end{eqnarray}$$
To analyze (7.68), we introduce the following two lemmas, the proofs of which are minor adaptations of the proofs of Lemmas 3.15 and 3.16 from [Reference ZhangZha18b].
Lemma 7.11. Fix
![]() $\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}$
. Then we have
$\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}$
. Then we have
 $$\begin{eqnarray}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{\substack{ m^{\prime },n^{\prime }\ll uq/X \\ \mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(n^{\prime },-m^{\prime })=\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(n,-m)}}1\ll N^{\unicode[STIX]{x1D716}}(\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(n,-m),\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}.\end{eqnarray}$$
$$\begin{eqnarray}\mathop{\sum }_{\substack{ \unicode[STIX]{x1D6FE}^{\prime }\in \mathfrak{F}_{T} \\ \mathfrak{d}_{\unicode[STIX]{x1D6FE}^{\prime }}=\mathfrak{d}_{\unicode[STIX]{x1D6FE}}}}\mathop{\sum }_{\substack{ m^{\prime },n^{\prime }\ll uq/X \\ \mathfrak{f}_{M\unicode[STIX]{x1D6FE}^{\prime }}(n^{\prime },-m^{\prime })=\mathfrak{f}_{M\unicode[STIX]{x1D6FE}}(n,-m)}}1\ll N^{\unicode[STIX]{x1D716}}(\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(n,-m),\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}.\end{eqnarray}$$
Lemma 7.12. For any
![]() $\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}$
,
$\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}$
,
![]() $d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}$
and any integer
$d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}$
and any integer
![]() $W>0$
, we have
$W>0$
, we have
 $$\begin{eqnarray}\mathop{\sum }_{\substack{ m,n\leqslant W \\ \tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(n,-m)\equiv 0(d)}}1\ll W^{2}d^{-1/2}+W.\end{eqnarray}$$
$$\begin{eqnarray}\mathop{\sum }_{\substack{ m,n\leqslant W \\ \tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(n,-m)\equiv 0(d)}}1\ll W^{2}d^{-1/2}+W.\end{eqnarray}$$
We now return to (7.68). From Lemmas 7.11 and 7.12, we have
 $$\begin{eqnarray}\displaystyle {\mathcal{I}}_{Q}^{(=,=)} & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}UX^{4}}{QJ}\mathop{\sum }_{u<U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n\ll uq/X}\frac{(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2},q)}{q}(\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(m,-n),\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}UX^{4}}{QJ}\mathop{\sum }_{u<U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\frac{(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2},q)}{q}\mathop{\sum }_{d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}d^{1/2}\mathop{\sum }_{m,n\ll uq/X}\mathbf{1}\{\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(m,-n)\equiv 0(d)\}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}UX^{4}}{QJ}\mathop{\sum }_{u<U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\frac{(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2},q)}{q}\mathop{\sum }_{d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}d^{1/2}\biggl(\frac{u^{2}q^{2}}{X^{2}d^{1/2}}+\frac{uq}{X}\biggr)\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{4}X^{4}}{QJ}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\frac{(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2},q)}{q}\cdot \frac{T^{2}q}{X}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{4}X^{3}T^{2}}{QJ}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}d\mathop{\sum }_{\substack{ Q<q\leqslant 2Q \\ q\equiv 0(d)}}1\nonumber\\ \displaystyle & \ll & \displaystyle N^{\unicode[STIX]{x1D716}}U^{4}X^{2}T^{2\unicode[STIX]{x1D6FF}}\ll N^{1+\unicode[STIX]{x1D716}}T^{4\unicode[STIX]{x1D6FF}-4}(U^{4}T^{2-2\unicode[STIX]{x1D6FF}})\nonumber\\ \displaystyle & \ll & \displaystyle N^{1-\unicode[STIX]{x1D702}}T^{4\unicode[STIX]{x1D6FF}-4}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{I}}_{Q}^{(=,=)} & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}UX^{4}}{QJ}\mathop{\sum }_{u<U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\mathop{\sum }_{m,n\ll uq/X}\frac{(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2},q)}{q}(\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(m,-n),\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2})^{1/2}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}UX^{4}}{QJ}\mathop{\sum }_{u<U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\frac{(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2},q)}{q}\mathop{\sum }_{d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}d^{1/2}\mathop{\sum }_{m,n\ll uq/X}\mathbf{1}\{\tilde{\mathfrak{f}}_{M\unicode[STIX]{x1D6FE}}(m,-n)\equiv 0(d)\}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}UX^{4}}{QJ}\mathop{\sum }_{u<U}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\frac{(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2},q)}{q}\mathop{\sum }_{d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}d^{1/2}\biggl(\frac{u^{2}q^{2}}{X^{2}d^{1/2}}+\frac{uq}{X}\biggr)\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{4}X^{4}}{QJ}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{Q<q\leqslant 2Q}\frac{(\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2},q)}{q}\cdot \frac{T^{2}q}{X}\nonumber\\ \displaystyle & \ll & \displaystyle \frac{N^{\unicode[STIX]{x1D716}}U^{4}X^{3}T^{2}}{QJ}\mathop{\sum }_{\unicode[STIX]{x1D6FE}\in \mathfrak{F}_{T}}\mathop{\sum }_{d|\mathfrak{d}_{\unicode[STIX]{x1D6FE}}^{2}}d\mathop{\sum }_{\substack{ Q<q\leqslant 2Q \\ q\equiv 0(d)}}1\nonumber\\ \displaystyle & \ll & \displaystyle N^{\unicode[STIX]{x1D716}}U^{4}X^{2}T^{2\unicode[STIX]{x1D6FF}}\ll N^{1+\unicode[STIX]{x1D716}}T^{4\unicode[STIX]{x1D6FF}-4}(U^{4}T^{2-2\unicode[STIX]{x1D6FF}})\nonumber\\ \displaystyle & \ll & \displaystyle N^{1-\unicode[STIX]{x1D702}}T^{4\unicode[STIX]{x1D6FF}-4}.\end{eqnarray}$$
Thus we have a power saving here, too.
Putting together, (7.64), (7.66) and (7.69) leads to the desired bound in Lemma 7.10. ◻
With the bounds on
![]() ${\mathcal{I}}_{1}$
,
${\mathcal{I}}_{1}$
,
![]() ${\mathcal{I}}_{2}$
, and
${\mathcal{I}}_{2}$
, and
![]() ${\mathcal{I}}_{3}$
that we have obtained here, Theorem 4.5 and the main Theorem 1.6 follow.
${\mathcal{I}}_{3}$
that we have obtained here, Theorem 4.5 and the main Theorem 1.6 follow.
8 Spectral gap for a class of Kleinian groups
In this section, we prove Theorem 1.3, which will in particular imply that a familial group
![]() ${\mathcal{A}}$
has a geometric spectral gap. Theorem 1.3 concerns more generally an infinite-covolume, geometrically finite, Zariski dense Kleinian group
${\mathcal{A}}$
has a geometric spectral gap. Theorem 1.3 concerns more generally an infinite-covolume, geometrically finite, Zariski dense Kleinian group
![]() ${\mathcal{A}}<\text{PSL}_{2}({\mathcal{O}}_{K})$
containing a Zariski dense subgroup
${\mathcal{A}}<\text{PSL}_{2}({\mathcal{O}}_{K})$
containing a Zariski dense subgroup
![]() $\unicode[STIX]{x1D6E4}<\text{PSL}_{2}(\mathbb{Z})$
.
$\unicode[STIX]{x1D6E4}<\text{PSL}_{2}(\mathbb{Z})$
.
We first simplify the situation by moving to
![]() $\text{SL}_{2}$
instead of
$\text{SL}_{2}$
instead of
![]() $\text{PSL}_{2}$
. In particular, letting
$\text{PSL}_{2}$
. In particular, letting
![]() ${\mathcal{A}}^{\prime }$
be the preimage of
${\mathcal{A}}^{\prime }$
be the preimage of
![]() ${\mathcal{A}}$
in
${\mathcal{A}}$
in
![]() $\text{SL}_{2}$
, then the quotients
$\text{SL}_{2}$
, then the quotients
![]() ${\mathcal{A}}^{\prime }(q)\backslash \mathbb{H}$
and
${\mathcal{A}}^{\prime }(q)\backslash \mathbb{H}$
and
![]() ${\mathcal{A}}(q)\backslash \mathbb{H}$
are the same for all
${\mathcal{A}}(q)\backslash \mathbb{H}$
are the same for all
![]() $q\in \mathbb{Z}^{+}$
. Therefore, their geometric spectral theories agree. The properties of being geometrically finite, infinite-covolume, Zariski dense and having a Zariski dense surface subgroup are preserved.
$q\in \mathbb{Z}^{+}$
. Therefore, their geometric spectral theories agree. The properties of being geometrically finite, infinite-covolume, Zariski dense and having a Zariski dense surface subgroup are preserved.
Assume that
![]() ${\mathcal{A}}$
is not itself contained in
${\mathcal{A}}$
is not itself contained in
![]() $\text{SL}_{2}(\mathbb{Z})$
(in which case it has a spectral gap in the senses described below by [Reference Bourgain and VarjúBV12]).
$\text{SL}_{2}(\mathbb{Z})$
(in which case it has a spectral gap in the senses described below by [Reference Bourgain and VarjúBV12]).
Assume also that
![]() $\unicode[STIX]{x1D6E4}$
has a multiplicative structure, in the sense that for any
$\unicode[STIX]{x1D6E4}$
has a multiplicative structure, in the sense that for any
![]() $q=\prod _{i}p_{i}^{n_{i}}$
,
$q=\prod _{i}p_{i}^{n_{i}}$
,
For if
![]() $\unicode[STIX]{x1D6E4}$
does not have this multiplicative structure, we replace
$\unicode[STIX]{x1D6E4}$
does not have this multiplicative structure, we replace
![]() $\unicode[STIX]{x1D6E4}$
by
$\unicode[STIX]{x1D6E4}$
by
![]() $\widehat{\unicode[STIX]{x1D6E4}}:=\unicode[STIX]{x1D6E4}\cap \unicode[STIX]{x1D6EC}$
, where
$\widehat{\unicode[STIX]{x1D6E4}}:=\unicode[STIX]{x1D6E4}\cap \unicode[STIX]{x1D6EC}$
, where
![]() $\unicode[STIX]{x1D6EC}$
is a principal congruence subgroup of
$\unicode[STIX]{x1D6EC}$
is a principal congruence subgroup of
![]() $\text{SL}_{2}(\mathbb{Z})$
, so that
$\text{SL}_{2}(\mathbb{Z})$
, so that
![]() $\widehat{\unicode[STIX]{x1D6E4}}$
has a multiplicative structure. The existence of this subgroup is guaranteed by the strong approximation property. Then
$\widehat{\unicode[STIX]{x1D6E4}}$
has a multiplicative structure. The existence of this subgroup is guaranteed by the strong approximation property. Then
![]() $\widehat{\unicode[STIX]{x1D6E4}}$
still has Zariski closure
$\widehat{\unicode[STIX]{x1D6E4}}$
still has Zariski closure
![]() $\text{SL}_{2}(\mathbb{R})$
as it is finite index in
$\text{SL}_{2}(\mathbb{R})$
as it is finite index in
![]() $\unicode[STIX]{x1D6E4}$
(see (0.13) in [Reference MargulisMar91, ch. I]).
$\unicode[STIX]{x1D6E4}$
(see (0.13) in [Reference MargulisMar91, ch. I]).
As a byproduct, we prove a version of strong approximation for
![]() ${\mathcal{A}}$
, as follows.
${\mathcal{A}}$
, as follows.
Theorem 8.1. Let
![]() ${\mathcal{A}}$
and
${\mathcal{A}}$
and
![]() $\unicode[STIX]{x1D6E4}$
be as above. There exists an integer
$\unicode[STIX]{x1D6E4}$
be as above. There exists an integer
![]() $P_{\text{bad}}$
depending on
$P_{\text{bad}}$
depending on
![]() ${\mathcal{A}}$
such that if
${\mathcal{A}}$
such that if
![]() $q\in \mathbb{Z}$
, with
$q\in \mathbb{Z}$
, with
![]() $q=q_{\text{bad}}\cdot q_{1}$
where
$q=q_{\text{bad}}\cdot q_{1}$
where
![]() $(q_{1},P_{\text{bad}})=1$
, we have the following.
$(q_{1},P_{\text{bad}})=1$
, we have the following.
(i)
 $$\begin{eqnarray}{\mathcal{A}}/{\mathcal{A}}(q)\cong {\mathcal{A}}/{\mathcal{A}}(q_{\text{bad}})\times {\mathcal{A}}/{\mathcal{A}}(q_{1}).\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{A}}/{\mathcal{A}}(q)\cong {\mathcal{A}}/{\mathcal{A}}(q_{\text{bad}})\times {\mathcal{A}}/{\mathcal{A}}(q_{1}).\end{eqnarray}$$
(ii)
 $$\begin{eqnarray}\displaystyle {\mathcal{A}}/{\mathcal{A}}(q_{1}) & = & \displaystyle \text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(q_{1})\nonumber\\ \displaystyle & \cong & \displaystyle \mathop{\prod }_{p_{i}^{n_{i}}||q_{1}}\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p_{i}^{n_{i}}).\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{A}}/{\mathcal{A}}(q_{1}) & = & \displaystyle \text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(q_{1})\nonumber\\ \displaystyle & \cong & \displaystyle \mathop{\prod }_{p_{i}^{n_{i}}||q_{1}}\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p_{i}^{n_{i}}).\nonumber\end{eqnarray}$$
(iii) For each
 $p|P_{\text{bad}}$
, there exists
$p|P_{\text{bad}}$
, there exists
 $m_{p}\geqslant 1$
such that for all
$m_{p}\geqslant 1$
such that for all
 $k_{p}\geqslant m_{p}$
, Moreover, we can choose
$k_{p}\geqslant m_{p}$
, Moreover, we can choose $$\begin{eqnarray}{\mathcal{A}}(p^{m_{p}})/{\mathcal{A}}(p^{k_{p}})=\text{SL}(2,{\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}(2,{\mathcal{O}}_{K})(p^{k_{p}}).\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{A}}(p^{m_{p}})/{\mathcal{A}}(p^{k_{p}})=\text{SL}(2,{\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}(2,{\mathcal{O}}_{K})(p^{k_{p}}).\end{eqnarray}$$
 $m_{p}$
so that
$m_{p}$
so that
 $m_{p}\leqslant m_{p}^{\prime }+\unicode[STIX]{x1D704}_{p}$
, where
$m_{p}\leqslant m_{p}^{\prime }+\unicode[STIX]{x1D704}_{p}$
, where
 $m_{p}^{\prime }$
is the smallest positive power
$m_{p}^{\prime }$
is the smallest positive power
 $m$
of
$m$
of
 $p$
such that for all
$p$
such that for all
 $k_{p}^{\prime }\geqslant m_{p}^{\prime }$
, and
$k_{p}^{\prime }\geqslant m_{p}^{\prime }$
, and $$\begin{eqnarray}\unicode[STIX]{x1D6E4}(p^{m_{p}^{\prime }})/\unicode[STIX]{x1D6E4}(p^{k_{p}^{\prime }})=\text{SL}_{2}(\mathbb{Z})(p^{m_{p}^{\prime }})/\text{SL}_{2}(\mathbb{Z})(p^{k_{p}^{\prime }})\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E4}(p^{m_{p}^{\prime }})/\unicode[STIX]{x1D6E4}(p^{k_{p}^{\prime }})=\text{SL}_{2}(\mathbb{Z})(p^{m_{p}^{\prime }})/\text{SL}_{2}(\mathbb{Z})(p^{k_{p}^{\prime }})\end{eqnarray}$$
 $\unicode[STIX]{x1D704}_{p}$
is the smallest non-negative integer such that In this notation, the action of
$\unicode[STIX]{x1D704}_{p}$
is the smallest non-negative integer such that In this notation, the action of $$\begin{eqnarray}p^{\unicode[STIX]{x1D704}_{p}}\mathfrak{sl}(2,\mathbb{Z}_{p}\otimes _{\mathbb{Z}}{\mathcal{O}}_{K})\subset \operatorname{Span}_{\mathbb{Z}_{p}}({\mathcal{A}}\cdot \mathfrak{sl}(2,\mathbb{Z}_{p})).\end{eqnarray}$$
$$\begin{eqnarray}p^{\unicode[STIX]{x1D704}_{p}}\mathfrak{sl}(2,\mathbb{Z}_{p}\otimes _{\mathbb{Z}}{\mathcal{O}}_{K})\subset \operatorname{Span}_{\mathbb{Z}_{p}}({\mathcal{A}}\cdot \mathfrak{sl}(2,\mathbb{Z}_{p})).\end{eqnarray}$$
 ${\mathcal{A}}$
is the restriction of the adjoint action of the Lie group
${\mathcal{A}}$
is the restriction of the adjoint action of the Lie group
 $\text{SL}$
on its Lie algebra
$\text{SL}$
on its Lie algebra
 $\mathfrak{sl}$
, i.e. conjugation.
$\mathfrak{sl}$
, i.e. conjugation.(iv) If
 $p|P_{\text{bad}}$
, then
$p|P_{\text{bad}}$
, then
 $p$
can only possibly be one of the following:
$p$
can only possibly be one of the following: (a)
 $p=2,3$
; or
$p=2,3$
; or(b)
 $p$
is such that
$p$
is such that
 $\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p)\neq \text{SL}_{2}(\mathbb{Z}/p\mathbb{Z})$
; or
$\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p)\neq \text{SL}_{2}(\mathbb{Z}/p\mathbb{Z})$
; or(c)
 $p$
is a common factor of all curvatures in the associated orbit
$p$
is a common factor of all curvatures in the associated orbit
 ${\mathcal{A}}\cdot \mathbb{P}^{1}(\mathbb{R})$
(after scaling all raw curvatures by
${\mathcal{A}}\cdot \mathbb{P}^{1}(\mathbb{R})$
(after scaling all raw curvatures by
 $1/\sqrt{-\unicode[STIX]{x1D6E5}}$
).
$1/\sqrt{-\unicode[STIX]{x1D6E5}}$
).
Note that in the case of a familial group
![]() ${\mathcal{A}}$
(which is the object of this paper),
${\mathcal{A}}$
(which is the object of this paper),
![]() $\unicode[STIX]{x1D6E4}$
can be taken to be the principal congruence subgroup of
$\unicode[STIX]{x1D6E4}$
can be taken to be the principal congruence subgroup of
![]() $\text{SL}_{2}(\mathbb{Z})$
contained in
$\text{SL}_{2}(\mathbb{Z})$
contained in
![]() ${\mathcal{A}}$
, in which case the bad primes of the second kind in the theorem above are simply those dividing the level of this congruence subgroup.
${\mathcal{A}}$
, in which case the bad primes of the second kind in the theorem above are simply those dividing the level of this congruence subgroup.
We begin with the definitions of geometric and combinatorial spectral gaps for
![]() ${\mathcal{A}}$
. Let
${\mathcal{A}}$
. Let
![]() $\unicode[STIX]{x1D6E5}$
be the hyperbolic Laplacian operator associated to the metric
$\unicode[STIX]{x1D6E5}$
be the hyperbolic Laplacian operator associated to the metric
![]() $ds^{2}=(dx^{2}+dy^{2}+dz^{2})/z^{2}$
on
$ds^{2}=(dx^{2}+dy^{2}+dz^{2})/z^{2}$
on
![]() $\mathbb{H}^{3}$
:
$\mathbb{H}^{3}$
:
For any integer
![]() $q>1$
, let
$q>1$
, let
![]() ${\mathcal{A}}(q)$
denote the kernel of reduction modulo
${\mathcal{A}}(q)$
denote the kernel of reduction modulo
![]() $q$
. The operator
$q$
. The operator
![]() $\unicode[STIX]{x1D6E5}$
is symmetric and positive definite on
$\unicode[STIX]{x1D6E5}$
is symmetric and positive definite on
![]() $L^{2}({\mathcal{A}}(q)\backslash \mathbb{H}^{3})$
with the standard inner product. From Lax and Phillips [Reference Lax and PhillipsLP82], the discrete spectrum consists of finitely many eigenvalues
$L^{2}({\mathcal{A}}(q)\backslash \mathbb{H}^{3})$
with the standard inner product. From Lax and Phillips [Reference Lax and PhillipsLP82], the discrete spectrum consists of finitely many eigenvalues
From [Reference SullivanSul84], we have
![]() $\unicode[STIX]{x1D706}_{0}(q)\not =\unicode[STIX]{x1D706}_{1}(q)$
. If there exists
$\unicode[STIX]{x1D706}_{0}(q)\not =\unicode[STIX]{x1D706}_{1}(q)$
. If there exists
![]() $\unicode[STIX]{x1D716}>0$
independent of
$\unicode[STIX]{x1D716}>0$
independent of
![]() $q$
such that
$q$
such that
![]() $\unicode[STIX]{x1D706}_{1}(q)-\unicode[STIX]{x1D706}_{0}(q)\geqslant \unicode[STIX]{x1D716}$
for all
$\unicode[STIX]{x1D706}_{1}(q)-\unicode[STIX]{x1D706}_{0}(q)\geqslant \unicode[STIX]{x1D716}$
for all
![]() $q$
then
$q$
then
![]() $\unicode[STIX]{x1D716}$
is called a geometric spectral gap and
$\unicode[STIX]{x1D716}$
is called a geometric spectral gap and
![]() ${\mathcal{A}}$
is said to have a geometric spectral gap.
${\mathcal{A}}$
is said to have a geometric spectral gap.
We now recall the definition of a combinatorial spectral gap for
![]() ${\mathcal{A}}$
. Suppose
${\mathcal{A}}$
. Suppose
![]() ${\mathcal{A}}$
has a finite symmetric generating set
${\mathcal{A}}$
has a finite symmetric generating set
![]() $S$
. Let
$S$
. Let
denote the eigenvalues of the averaging operator
![]() $T_{{\mathcal{A}},S}:=1-\unicode[STIX]{x1D6E5}_{{\mathcal{A}},S}/|S|$
where
$T_{{\mathcal{A}},S}:=1-\unicode[STIX]{x1D6E5}_{{\mathcal{A}},S}/|S|$
where
![]() $\unicode[STIX]{x1D6E5}_{{\mathcal{A}},S}$
is the discrete Laplacian operator
$\unicode[STIX]{x1D6E5}_{{\mathcal{A}},S}$
is the discrete Laplacian operator
We say that
![]() ${\mathcal{A}}$
has a combinatorial spectral gap if there are a finite symmetric collection of generators
${\mathcal{A}}$
has a combinatorial spectral gap if there are a finite symmetric collection of generators
![]() $S$
and a positive
$S$
and a positive
![]() $\unicode[STIX]{x1D716}$
such that
$\unicode[STIX]{x1D716}$
such that
for all positive integers
![]() $q$
, where
$q$
, where
![]() $\unicode[STIX]{x1D716}$
is independent of
$\unicode[STIX]{x1D716}$
is independent of
![]() $q$
(here
$q$
(here
![]() $\overline{S}$
denotes the image of
$\overline{S}$
denotes the image of
![]() $S$
modulo
$S$
modulo
![]() $q$
). Informally, a spectral gap for
$q$
). Informally, a spectral gap for
![]() ${\mathcal{A}}/{\mathcal{A}}(q)$
gives a measure of how quickly a random walk on the Cayley graph of
${\mathcal{A}}/{\mathcal{A}}(q)$
gives a measure of how quickly a random walk on the Cayley graph of
![]() ${\mathcal{A}}/{\mathcal{A}}(q)$
reaches the whole graph. A spectral gap for
${\mathcal{A}}/{\mathcal{A}}(q)$
reaches the whole graph. A spectral gap for
![]() ${\mathcal{A}}$
indicates a uniform rate for all
${\mathcal{A}}$
indicates a uniform rate for all
![]() $q$
.
$q$
.
We now give an overview of the proof of Theorem 1.3. Let
![]() $T$
be an element of
$T$
be an element of
![]() ${\mathcal{A}}$
which does not normalize
${\mathcal{A}}$
which does not normalize
![]() $\text{SL}_{2}(\mathbb{R})$
, i.e.
$\text{SL}_{2}(\mathbb{R})$
, i.e.
![]() $T\not \in \text{SL}_{2}(\mathbb{R})\,\cup \,i\text{SL}_{2}(\mathbb{R})\big(\!\begin{smallmatrix}1 & 0\\ 0 & -1\end{smallmatrix}\!\big)$
. Write
$T\not \in \text{SL}_{2}(\mathbb{R})\,\cup \,i\text{SL}_{2}(\mathbb{R})\big(\!\begin{smallmatrix}1 & 0\\ 0 & -1\end{smallmatrix}\!\big)$
. Write
![]() $\unicode[STIX]{x1D6E4}^{\prime }=T\unicode[STIX]{x1D6E4}T^{-1}$
, and let
$\unicode[STIX]{x1D6E4}^{\prime }=T\unicode[STIX]{x1D6E4}T^{-1}$
, and let
![]() ${\mathcal{A}}^{\prime }=\langle \unicode[STIX]{x1D6E4},\unicode[STIX]{x1D6E4}^{\prime }\rangle <{\mathcal{A}}$
. We first prove a combinatorial spectral gap for
${\mathcal{A}}^{\prime }=\langle \unicode[STIX]{x1D6E4},\unicode[STIX]{x1D6E4}^{\prime }\rangle <{\mathcal{A}}$
. We first prove a combinatorial spectral gap for
![]() ${\mathcal{A}}^{\prime }$
, using ideas similar to those of Varjú in the appendix of [Reference Bourgain and KontorovichBK14a], some of which have also been used by Sarnak in [Reference SarnakSar90], Shalom in [Reference ShalomSha99], and Kassabov, Lubotzky and Nikolov in [Reference Kassabov, Lubotzky and NikolovKLN06]. We then convert this to a combinatorial spectral gap for
${\mathcal{A}}^{\prime }$
, using ideas similar to those of Varjú in the appendix of [Reference Bourgain and KontorovichBK14a], some of which have also been used by Sarnak in [Reference SarnakSar90], Shalom in [Reference ShalomSha99], and Kassabov, Lubotzky and Nikolov in [Reference Kassabov, Lubotzky and NikolovKLN06]. We then convert this to a combinatorial spectral gap for
![]() ${\mathcal{A}}$
. Finally, we use the fact that a combinatorial spectral gap for
${\mathcal{A}}$
. Finally, we use the fact that a combinatorial spectral gap for
![]() ${\mathcal{A}}$
implies a geometric spectral gap if the Hausdorff dimension of the limit set of
${\mathcal{A}}$
implies a geometric spectral gap if the Hausdorff dimension of the limit set of
![]() ${\mathcal{A}}$
is greater than
${\mathcal{A}}$
is greater than
![]() $1$
via a variant of [Reference Bourgain, Gamburd and SarnakBGS11, Theorem 1.2], which states that geometric and combinatorial spectral gaps co-occur as long as the Hausdorff dimension of the limit set of the group is greater than
$1$
via a variant of [Reference Bourgain, Gamburd and SarnakBGS11, Theorem 1.2], which states that geometric and combinatorial spectral gaps co-occur as long as the Hausdorff dimension of the limit set of the group is greater than
![]() $1$
.
$1$
.
Proposition 8.2.
![]() ${\mathcal{A}}^{\prime }$
has a combinatorial spectral gap.
${\mathcal{A}}^{\prime }$
has a combinatorial spectral gap.
As a Zariski dense subgroup of
![]() $\text{SL}_{2}(\mathbb{Z})$
,
$\text{SL}_{2}(\mathbb{Z})$
,
![]() $\unicode[STIX]{x1D6E4}$
is known to have a spectral gap (see [Reference Bourgain and VarjúBV12]), and therefore so does
$\unicode[STIX]{x1D6E4}$
is known to have a spectral gap (see [Reference Bourgain and VarjúBV12]), and therefore so does
![]() $\unicode[STIX]{x1D6E4}^{\prime }$
. We will show that
$\unicode[STIX]{x1D6E4}^{\prime }$
. We will show that
![]() ${\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
is made up of a bounded number of copies of
${\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
is made up of a bounded number of copies of
![]() $\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(q)$
and
$\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(q)$
and
![]() $\unicode[STIX]{x1D6E4}^{\prime }/\unicode[STIX]{x1D6E4}^{\prime }(q)$
, which will imply a spectral gap for
$\unicode[STIX]{x1D6E4}^{\prime }/\unicode[STIX]{x1D6E4}^{\prime }(q)$
, which will imply a spectral gap for
![]() ${\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
. To be precise, we quote a lemma due to Varjú.
${\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
. To be precise, we quote a lemma due to Varjú.
Lemma 8.3 [Reference Bourgain and KontorovichBK14a, Lemma A.4].
Let
![]() $G$
be a finite group with a finite symmetric generating set
$G$
be a finite group with a finite symmetric generating set
![]() $S$
. Suppose that
$S$
. Suppose that
![]() $G_{1},\ldots ,G_{k}<G$
, and that for each
$G_{1},\ldots ,G_{k}<G$
, and that for each
![]() $g\in G$
, there exist
$g\in G$
, there exist
![]() $g_{i}\in G_{i}$
such that
$g_{i}\in G_{i}$
such that
![]() $g=g_{1}g_{2}\ldots g_{k}$
. Then
$g=g_{1}g_{2}\ldots g_{k}$
. Then
As a consequence, we immediately have the following result.
Lemma 8.4. Let
![]() $G$
be a group with a finite symmetric generating set
$G$
be a group with a finite symmetric generating set
![]() $S$
and a tuple
$S$
and a tuple
![]() $(G_{1},G_{2},\ldots ,G_{k})$
of subgroups of
$(G_{1},G_{2},\ldots ,G_{k})$
of subgroups of
![]() $G$
such that:
$G$
such that:
(i) each
 $G_{i}$
has a spectral gap;
$G_{i}$
has a spectral gap;(ii)
 $S\cap G_{i}\neq \emptyset$
for each
$S\cap G_{i}\neq \emptyset$
for each
 $G_{i}$
;
$G_{i}$
;(iii) for each integer
 $q$
, for each
$q$
, for each
 $g\in \unicode[STIX]{x1D70C}_{q}(G)$
, it is possible to write
$g\in \unicode[STIX]{x1D70C}_{q}(G)$
, it is possible to write
 $g=g_{1}g_{2}\cdots g_{k}$
where
$g=g_{1}g_{2}\cdots g_{k}$
where
 $g_{i}\in \unicode[STIX]{x1D70C}_{q}(G_{i})$
, i.e.
$g_{i}\in \unicode[STIX]{x1D70C}_{q}(G_{i})$
, i.e.  $$\begin{eqnarray}\unicode[STIX]{x1D70C}_{q}(G)=\mathop{\prod }_{i=1}^{k}\unicode[STIX]{x1D70C}_{q}(G_{i}).\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70C}_{q}(G)=\mathop{\prod }_{i=1}^{k}\unicode[STIX]{x1D70C}_{q}(G_{i}).\end{eqnarray}$$
Then
![]() $G$
has a combinatorial spectral gap.
$G$
has a combinatorial spectral gap.
To verify the hypotheses of Lemma 8.4 for
![]() $G={\mathcal{A}}^{\prime }$
, we will use
$G={\mathcal{A}}^{\prime }$
, we will use
![]() $k=2k_{0}$
and the tuple
$k=2k_{0}$
and the tuple
![]() $(G_{1},G_{2},\ldots ,G_{2k_{0}})=(\unicode[STIX]{x1D6E4},\unicode[STIX]{x1D6E4}^{\prime },\unicode[STIX]{x1D6E4},\unicode[STIX]{x1D6E4}^{\prime },\ldots ,\unicode[STIX]{x1D6E4}^{\prime })$
. Write
$(G_{1},G_{2},\ldots ,G_{2k_{0}})=(\unicode[STIX]{x1D6E4},\unicode[STIX]{x1D6E4}^{\prime },\unicode[STIX]{x1D6E4},\unicode[STIX]{x1D6E4}^{\prime },\ldots ,\unicode[STIX]{x1D6E4}^{\prime })$
. Write
Then, for the third hypothesis of Lemma 8.4, we need to show the following lemma.
Lemma 8.5. There exists some
![]() $k_{0}$
such that
$k_{0}$
such that
![]() $A_{k_{0}}(q)={\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
for every
$A_{k_{0}}(q)={\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
for every
![]() $q$
.
$q$
.
Our approach is to break
![]() $q$
into prime powers, and prove a universal bound for prime powers for all but finitely many ‘bad primes’. We therefore break the proof into two lemmas dealing with the good primes and bad primes, respectively. The first lemma uses some geometric arguments to construct elements of
$q$
into prime powers, and prove a universal bound for prime powers for all but finitely many ‘bad primes’. We therefore break the proof into two lemmas dealing with the good primes and bad primes, respectively. The first lemma uses some geometric arguments to construct elements of
![]() ${\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(p^{m})$
in terms of
${\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(p^{m})$
in terms of
![]() $\unicode[STIX]{x1D6E4}$
and
$\unicode[STIX]{x1D6E4}$
and
![]() $\unicode[STIX]{x1D6E4}^{\prime }$
. The second lemma works prime by prime, and uses the Lie algebra
$\unicode[STIX]{x1D6E4}^{\prime }$
. The second lemma works prime by prime, and uses the Lie algebra
![]() $\mathfrak{sl}_{2}$
to lift to higher powers of
$\mathfrak{sl}_{2}$
to lift to higher powers of
![]() $p$
uniformly.
$p$
uniformly.
Lemma 8.6. There exists a finite set of primes
![]() ${\mathcal{S}}$
such that, for
${\mathcal{S}}$
such that, for
![]() $p\notin {\mathcal{S}}$
, and for all
$p\notin {\mathcal{S}}$
, and for all
![]() $m\geqslant 1$
, we have
$m\geqslant 1$
, we have
Proof. Throughout the proof we assume
![]() $p\not \in {\mathcal{S}}$
, and we augment
$p\not \in {\mathcal{S}}$
, and we augment
![]() ${\mathcal{S}}$
as necessary while preserving its finiteness.
${\mathcal{S}}$
as necessary while preserving its finiteness.
Consider
![]() $C_{T}=T^{-1}\cdot \mathbb{P}^{1}(\mathbb{R})$
. If
$C_{T}=T^{-1}\cdot \mathbb{P}^{1}(\mathbb{R})$
. If
![]() $C_{T}$
is a line, let
$C_{T}$
is a line, let
![]() $\unicode[STIX]{x1D6FE}$
be the identity matrix. Otherwise it is a circle, and we write
$\unicode[STIX]{x1D6FE}$
be the identity matrix. Otherwise it is a circle, and we write
![]() $r\sqrt{d}$
for its radius,
$r\sqrt{d}$
for its radius,
![]() $x_{0}+\sqrt{-d}y_{0}$
for its center, and let
$x_{0}+\sqrt{-d}y_{0}$
for its center, and let
Note that
![]() $x_{0},y_{0},r$
are rational numbers which may be written with denominator
$x_{0},y_{0},r$
are rational numbers which may be written with denominator
![]() $b$
, the curvature of
$b$
, the curvature of
![]() $C_{T}$
(formulas for these integers in terms of the entries of
$C_{T}$
(formulas for these integers in terms of the entries of
![]() $T$
are given in [Reference StangeSta18b, Proposition 3.7]). Then the intersection points of
$T$
are given in [Reference StangeSta18b, Proposition 3.7]). Then the intersection points of
![]() $\unicode[STIX]{x1D6FE}T^{-1}\cdot \mathbb{P}^{1}(\mathbb{R})$
with the imaginary axis are of the form
$\unicode[STIX]{x1D6FE}T^{-1}\cdot \mathbb{P}^{1}(\mathbb{R})$
with the imaginary axis are of the form
![]() $\sqrt{-d}s$
where, in the case where
$\sqrt{-d}s$
where, in the case where
![]() $C_{T}$
is a line,
$C_{T}$
is a line,
![]() $\sqrt{-d}s$
is the height of the line, and if
$\sqrt{-d}s$
is the height of the line, and if
![]() $C_{T}$
is a circle,
$C_{T}$
is a circle,
![]() $s=y_{0}\pm r$
, and
$s=y_{0}\pm r$
, and
![]() $r\sqrt{d}$
is the radius of
$r\sqrt{d}$
is the radius of
![]() $T^{-1}\cdot \mathbb{P}^{1}(\mathbb{R})$
. In any case, choose such an
$T^{-1}\cdot \mathbb{P}^{1}(\mathbb{R})$
. In any case, choose such an
![]() $s$
, and remark that
$s$
, and remark that
![]() $\unicode[STIX]{x1D6FE}$
and
$\unicode[STIX]{x1D6FE}$
and
![]() $s$
are defined over
$s$
are defined over
![]() $\mathbb{Q}$
.
$\mathbb{Q}$
.
Consider reduction modulo
![]() $p^{m}{\mathcal{O}}_{K}$
on the projective line:
$p^{m}{\mathcal{O}}_{K}$
on the projective line:
Then the reduction map
is equivariant with respect to reduction on the projective line. Let
![]() ${\mathcal{S}}$
contain any primes where
${\mathcal{S}}$
contain any primes where
is not surjective for some
![]() $m\geqslant 1$
(there are finitely many such, by strong approximation for
$m\geqslant 1$
(there are finitely many such, by strong approximation for
![]() $\unicode[STIX]{x1D6E4}$
). We allow for
$\unicode[STIX]{x1D6E4}$
). We allow for
![]() $p$
to be inert, split, or ramified.
$p$
to be inert, split, or ramified.
Let
![]() ${\mathcal{S}}$
also contain any primes appearing in denominators of
${\mathcal{S}}$
also contain any primes appearing in denominators of
![]() $\unicode[STIX]{x1D6FE}$
, so that
$\unicode[STIX]{x1D6FE}$
, so that
![]() $\unicode[STIX]{x1D70C}_{p^{m}}(\unicode[STIX]{x1D6FE})$
is defined and has a lift in
$\unicode[STIX]{x1D70C}_{p^{m}}(\unicode[STIX]{x1D6FE})$
is defined and has a lift in
![]() $\unicode[STIX]{x1D6E4}$
. By expanding
$\unicode[STIX]{x1D6E4}$
. By expanding
![]() ${\mathcal{S}}$
, we may assume
${\mathcal{S}}$
, we may assume
![]() $p$
and
$p$
and
![]() $s$
are coprime.
$s$
are coprime.
Therefore
![]() $s$
is invertible modulo
$s$
is invertible modulo
![]() $p^{m}$
and there is a representation
$p^{m}$
and there is a representation
![]() $\unicode[STIX]{x1D719}_{s}:{\mathcal{O}}_{K}/(p^{m})\rightarrow M(2,\mathbb{Z}/(p^{m}))$
given by
$\unicode[STIX]{x1D719}_{s}:{\mathcal{O}}_{K}/(p^{m})\rightarrow M(2,\mathbb{Z}/(p^{m}))$
given by
In particular, the eigenvalues of the matrix are
![]() $x\pm y\sqrt{-d}$
, and the determinant is the norm
$x\pm y\sqrt{-d}$
, and the determinant is the norm
![]() $N(x+y\sqrt{-d})$
. It has exactly two fixed points modulo
$N(x+y\sqrt{-d})$
. It has exactly two fixed points modulo
![]() $p^{m}$
, namely
$p^{m}$
, namely
![]() $\pm s\sqrt{-d}$
.
$\pm s\sqrt{-d}$
.
Let
![]() $x$
and
$x$
and
![]() $y$
be a solution to
$y$
be a solution to
![]() $x^{2}+dy^{2}\equiv 1\;(\text{mod}\;p^{m})$
having
$x^{2}+dy^{2}\equiv 1\;(\text{mod}\;p^{m})$
having
![]() $\gcd (xy,p)=1$
. The existence of such is a consequence of an argument with Gauss sums [Reference CasselsCas78, Exercise 13(v), p. 32], if
$\gcd (xy,p)=1$
. The existence of such is a consequence of an argument with Gauss sums [Reference CasselsCas78, Exercise 13(v), p. 32], if
![]() $p\geqslant 5$
. Therefore let
$p\geqslant 5$
. Therefore let
![]() $2,3\in {\mathcal{S}}$
. Then
$2,3\in {\mathcal{S}}$
. Then
![]() $x+y\sqrt{-d}$
is of norm
$x+y\sqrt{-d}$
is of norm
![]() $1$
modulo
$1$
modulo
![]() $p^{m}$
, so that
$p^{m}$
, so that
![]() $\unicode[STIX]{x1D719}_{s}(x+y\sqrt{-d})$
is in
$\unicode[STIX]{x1D719}_{s}(x+y\sqrt{-d})$
is in
![]() $\text{SL}_{2}(\mathbb{Z}/(p^{m}))$
, and therefore has a lift, call it
$\text{SL}_{2}(\mathbb{Z}/(p^{m}))$
, and therefore has a lift, call it
![]() $T_{0}$
, in
$T_{0}$
, in
![]() $\unicode[STIX]{x1D6E4}$
. We guarantee that neither of
$\unicode[STIX]{x1D6E4}$
. We guarantee that neither of
![]() $(x\pm y\sqrt{-d})^{2}$
are equivalent to integers modulo
$(x\pm y\sqrt{-d})^{2}$
are equivalent to integers modulo
![]() $p^{m}$
(i.e. in the subring
$p^{m}$
(i.e. in the subring
![]() $\mathbb{Z}/(p^{m})\subset {\mathcal{O}}_{K}/(p^{m})$
), since
$\mathbb{Z}/(p^{m})\subset {\mathcal{O}}_{K}/(p^{m})$
), since
![]() $p\nmid 2xy$
by construction.
$p\nmid 2xy$
by construction.
Therefore
![]() $T\unicode[STIX]{x1D6FE}^{-1}T_{0}\unicode[STIX]{x1D6FE}T^{-1}$
, considered modulo
$T\unicode[STIX]{x1D6FE}^{-1}T_{0}\unicode[STIX]{x1D6FE}T^{-1}$
, considered modulo
![]() $p^{m}$
, has a fixed point in
$p^{m}$
, has a fixed point in
![]() $\mathbb{P}^{1}(\mathbb{Z}/(p^{m}))$
. Since
$\mathbb{P}^{1}(\mathbb{Z}/(p^{m}))$
. Since
![]() $\text{SL}_{2}(\mathbb{Z}/(p^{m}))$
is transitive on
$\text{SL}_{2}(\mathbb{Z}/(p^{m}))$
is transitive on
![]() $\mathbb{P}^{1}(\mathbb{Z}/(p^{m}))$
, we can conjugate this fixed point to
$\mathbb{P}^{1}(\mathbb{Z}/(p^{m}))$
, we can conjugate this fixed point to
![]() $\infty$
modulo
$\infty$
modulo
![]() $p^{m}$
. Therefore, we find an element
$p^{m}$
. Therefore, we find an element
![]() $T_{1}$
in
$T_{1}$
in
![]() $\unicode[STIX]{x1D6E4}T\unicode[STIX]{x1D6E4}T^{-1}\unicode[STIX]{x1D6E4}$
which fixes
$\unicode[STIX]{x1D6E4}T\unicode[STIX]{x1D6E4}T^{-1}\unicode[STIX]{x1D6E4}$
which fixes
![]() $\infty$
modulo
$\infty$
modulo
![]() $p^{m}$
.
$p^{m}$
.
So
![]() $T_{1}$
has the form
$T_{1}$
has the form
where
![]() $a_{0},a_{1}\in {\mathcal{O}}_{K}$
,
$a_{0},a_{1}\in {\mathcal{O}}_{K}$
,
![]() $a_{0}a_{1}\equiv 1\;(\text{mod}\;p^{m})$
. As
$a_{0}a_{1}\equiv 1\;(\text{mod}\;p^{m})$
. As
![]() $a_{0}$
and
$a_{0}$
and
![]() $a_{1}$
are the eigenvalues of
$a_{1}$
are the eigenvalues of
![]() $T_{1}$
and hence
$T_{1}$
and hence
![]() $T_{0}$
, they are
$T_{0}$
, they are
![]() $x\pm y\sqrt{-d}$
. In particular, we have arranged that
$x\pm y\sqrt{-d}$
. In particular, we have arranged that
![]() $a_{0}^{2}\not \in \mathbb{Z}/(p^{m})$
.
$a_{0}^{2}\not \in \mathbb{Z}/(p^{m})$
.
Now take
We know
![]() $a_{0}^{2}\notin \mathbb{Z}/(p^{m})$
and
$a_{0}^{2}\notin \mathbb{Z}/(p^{m})$
and
![]() $a_{0}^{2}$
is invertible. Now, this implies that
$a_{0}^{2}$
is invertible. Now, this implies that
![]() $a_{0}^{2}\mathbb{Z}/(p^{m})+\mathbb{Z}/(p^{m})={\mathcal{O}}_{K}/(p^{m})$
. This implies that all upper triangular matrices are in
$a_{0}^{2}\mathbb{Z}/(p^{m})+\mathbb{Z}/(p^{m})={\mathcal{O}}_{K}/(p^{m})$
. This implies that all upper triangular matrices are in
![]() $\unicode[STIX]{x1D6E4}T\unicode[STIX]{x1D6E4}T^{-1}\unicode[STIX]{x1D6E4}T\unicode[STIX]{x1D6E4}T^{-1}\unicode[STIX]{x1D6E4}$
modulo
$\unicode[STIX]{x1D6E4}T\unicode[STIX]{x1D6E4}T^{-1}\unicode[STIX]{x1D6E4}T\unicode[STIX]{x1D6E4}T^{-1}\unicode[STIX]{x1D6E4}$
modulo
![]() $p^{m}$
.
$p^{m}$
.
The rest of the proof follows Varjú. Specifically, an exactly analogous argument shows that the lower triangular matrices with
![]() $1$
s on the diagonal are also in
$1$
s on the diagonal are also in
![]() $\unicode[STIX]{x1D6E4}T\unicode[STIX]{x1D6E4}T^{-1}\unicode[STIX]{x1D6E4}T\unicode[STIX]{x1D6E4}T^{-1}\unicode[STIX]{x1D6E4}$
modulo
$\unicode[STIX]{x1D6E4}T\unicode[STIX]{x1D6E4}T^{-1}\unicode[STIX]{x1D6E4}T\unicode[STIX]{x1D6E4}T^{-1}\unicode[STIX]{x1D6E4}$
modulo
![]() $p^{m}$
. Therefore, in
$p^{m}$
. Therefore, in
![]() $A_{7}(p^{m})$
we obtain all elements of the form
$A_{7}(p^{m})$
we obtain all elements of the form
This includes any matrix
![]() $\unicode[STIX]{x1D6FE}$
with lower-left entry not congruent to
$\unicode[STIX]{x1D6FE}$
with lower-left entry not congruent to
![]() $0$
modulo
$0$
modulo
![]() $p$
, since it is possible to solve for
$p$
, since it is possible to solve for
![]() $a,b,c$
modulo
$a,b,c$
modulo
![]() $p^{m}$
in that circumstance. As this is more than half of the group
$p^{m}$
in that circumstance. As this is more than half of the group
![]() $\unicode[STIX]{x1D70C}_{p^{m}}({\mathcal{A}}^{\prime })$
, the lemma is proved.◻
$\unicode[STIX]{x1D70C}_{p^{m}}({\mathcal{A}}^{\prime })$
, the lemma is proved.◻
Lemma 8.7. Let
![]() $p$
be any prime. Then there exist some positive integers
$p$
be any prime. Then there exist some positive integers
![]() $k_{p}$
and
$k_{p}$
and
![]() $m_{p}$
such that
$m_{p}$
such that
for all
![]() $m\geqslant m_{p}$
.
$m\geqslant m_{p}$
.
Proof. Let
![]() $\text{SL}_{2}$
act on
$\text{SL}_{2}$
act on
![]() $\mathfrak{sl}_{2}$
via the standard adjoint action of a Lie group on its Lie algebra by conjugation, i.e.
$\mathfrak{sl}_{2}$
via the standard adjoint action of a Lie group on its Lie algebra by conjugation, i.e.
We will first find a
![]() $\mathbb{Q}_{p}$
-basis of
$\mathbb{Q}_{p}$
-basis of
![]() $\mathfrak{sl}(2,\mathbb{Q}_{p}\otimes _{\mathbb{Q}}K_{d})$
formed of elements from
$\mathfrak{sl}(2,\mathbb{Q}_{p}\otimes _{\mathbb{Q}}K_{d})$
formed of elements from
![]() $\mathfrak{sl}(2,\mathbb{Q})$
and
$\mathfrak{sl}(2,\mathbb{Q})$
and
![]() $\unicode[STIX]{x1D6E4}T~\cdot ~\mathfrak{sl}(2,\mathbb{Q})$
. Using this basis, we will apply an inductive argument to show that, for all
$\unicode[STIX]{x1D6E4}T~\cdot ~\mathfrak{sl}(2,\mathbb{Q})$
. Using this basis, we will apply an inductive argument to show that, for all
![]() $m\geqslant m_{p}$
(where
$m\geqslant m_{p}$
(where
![]() $m_{p}$
will be defined below), a finite-index subgroup of
$m_{p}$
will be defined below), a finite-index subgroup of
![]() $\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{m})$
, whose index is independent of
$\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{m})$
, whose index is independent of
![]() $m$
, is contained in
$m$
, is contained in
![]() $A_{4}(p^{m})$
.
$A_{4}(p^{m})$
.
To find the aforementioned basis, we begin with the standard basis for the real Lie algebra
![]() $\mathfrak{sl}(2,\mathbb{R})$
:
$\mathfrak{sl}(2,\mathbb{R})$
:
The above is also a
![]() $\mathbb{Q}_{p}$
-basis for
$\mathbb{Q}_{p}$
-basis for
![]() $\mathfrak{sl}(2,\mathbb{Q}_{p})$
for any
$\mathfrak{sl}(2,\mathbb{Q}_{p})$
for any
![]() $p$
, and a
$p$
, and a
![]() $\mathbb{Q}$
-basis for
$\mathbb{Q}$
-basis for
![]() $\mathfrak{sl}(2,\mathbb{Q})$
.
$\mathfrak{sl}(2,\mathbb{Q})$
.
First, we remark that
![]() $\unicode[STIX]{x1D6E4}(v)$
spans
$\unicode[STIX]{x1D6E4}(v)$
spans
![]() $\text{SL}_{2}(\mathbb{R})(v)$
over
$\text{SL}_{2}(\mathbb{R})(v)$
over
![]() $\mathbb{R}$
for any non-zero
$\mathbb{R}$
for any non-zero
![]() $v\in \mathfrak{sl}(2,\mathbb{C})$
. For since
$v\in \mathfrak{sl}(2,\mathbb{C})$
. For since
![]() $\unicode[STIX]{x1D6E4}$
is Zariski dense in
$\unicode[STIX]{x1D6E4}$
is Zariski dense in
![]() $\text{SL}_{2}$
, and the adjoint action is Zariski continuous, the Zariski closure of the orbit
$\text{SL}_{2}$
, and the adjoint action is Zariski continuous, the Zariski closure of the orbit
![]() $\unicode[STIX]{x1D6E4}(v)$
in
$\unicode[STIX]{x1D6E4}(v)$
in
![]() $\mathfrak{sl}(2,\mathbb{R})$
is
$\mathfrak{sl}(2,\mathbb{R})$
is
![]() $\text{SL}_{2}(\mathbb{R})(v)$
.
$\text{SL}_{2}(\mathbb{R})(v)$
.
Next, we claim that the orbit
![]() $\text{SL}_{2}(\mathbb{R})(v)$
must be of real dimension
$\text{SL}_{2}(\mathbb{R})(v)$
must be of real dimension
![]() $3$
. This follows from irreducibility of the adjoint action of
$3$
. This follows from irreducibility of the adjoint action of
![]() $\text{SL}_{2}(\mathbb{R})$
on
$\text{SL}_{2}(\mathbb{R})$
on
![]() $\mathfrak{sl}(2,\mathbb{R})$
in the case where
$\mathfrak{sl}(2,\mathbb{R})$
in the case where
![]() $v\in \mathfrak{sl}(2,\mathbb{R})$
. In fact, the same elementary irreducibility argument shows that the orbit
$v\in \mathfrak{sl}(2,\mathbb{R})$
. In fact, the same elementary irreducibility argument shows that the orbit
![]() $\text{SL}_{2}(\mathbb{R})(v)$
for any
$\text{SL}_{2}(\mathbb{R})(v)$
for any
![]() $v\in \mathfrak{sl}(2,\mathbb{C})$
is at least three-dimensional (any
$v\in \mathfrak{sl}(2,\mathbb{C})$
is at least three-dimensional (any
![]() $v$
can be conjugated to be diagonal, hence
$v$
can be conjugated to be diagonal, hence
![]() $\unicode[STIX]{x1D706}H$
with
$\unicode[STIX]{x1D706}H$
with
![]() $\unicode[STIX]{x1D706}\in \mathbb{C}$
; then conjugations and linear combinations yield
$\unicode[STIX]{x1D706}\in \mathbb{C}$
; then conjugations and linear combinations yield
![]() $\unicode[STIX]{x1D706}R$
and
$\unicode[STIX]{x1D706}R$
and
![]() $\unicode[STIX]{x1D706}L$
).
$\unicode[STIX]{x1D706}L$
).
Furthermore, for
![]() $v\notin \mathfrak{sl}(2,\mathbb{R})$
, we have
$v\notin \mathfrak{sl}(2,\mathbb{R})$
, we have
![]() $\text{SL}_{2}(\mathbb{R})(v)\cap \mathfrak{sl}(2,\mathbb{R})=\{0\}$
. By dimensional considerations, then, in this case
$\text{SL}_{2}(\mathbb{R})(v)\cap \mathfrak{sl}(2,\mathbb{R})=\{0\}$
. By dimensional considerations, then, in this case
Next, we show that the stabilizer of
![]() $\mathfrak{sl}(2,\mathbb{R})$
under the adjoint action of
$\mathfrak{sl}(2,\mathbb{R})$
under the adjoint action of
![]() $\text{SL}_{2}(\mathbb{C})$
is exactly
$\text{SL}_{2}(\mathbb{C})$
is exactly
![]() $\text{SL}_{2}(\mathbb{R})\,\cup \,i\text{SL}_{2}(\mathbb{R})$
. For, suppose
$\text{SL}_{2}(\mathbb{R})\,\cup \,i\text{SL}_{2}(\mathbb{R})$
. For, suppose
![]() $M$
is in the stabilizer. Then, taking
$M$
is in the stabilizer. Then, taking
![]() $\mathfrak{m}\in \mathfrak{sl}(2,\mathbb{R})\cap \text{SL}_{2}(\mathbb{R})$
(e.g. an elliptic element of order
$\mathfrak{m}\in \mathfrak{sl}(2,\mathbb{R})\cap \text{SL}_{2}(\mathbb{R})$
(e.g. an elliptic element of order
![]() $2$
with fixed points on
$2$
with fixed points on
![]() $\mathbb{P}^{1}(\mathbb{R})$
), we find that it must stabilize the circle
$\mathbb{P}^{1}(\mathbb{R})$
), we find that it must stabilize the circle
![]() $M(\mathbb{P}^{1}(\mathbb{R}))$
, which is only possible if
$M(\mathbb{P}^{1}(\mathbb{R}))$
, which is only possible if
![]() $M(\mathbb{P}^{1}(\mathbb{R}))=\mathbb{P}^{1}(\mathbb{R})$
. Hence the stabilizer of
$M(\mathbb{P}^{1}(\mathbb{R}))=\mathbb{P}^{1}(\mathbb{R})$
. Hence the stabilizer of
![]() $\mathfrak{sl}(2,\mathbb{R})$
is contained in the stabilizer of
$\mathfrak{sl}(2,\mathbb{R})$
is contained in the stabilizer of
![]() $\mathbb{P}^{1}(\mathbb{R})$
under the
$\mathbb{P}^{1}(\mathbb{R})$
under the
![]() $\text{SL}_{2}(\mathbb{C})$
action on
$\text{SL}_{2}(\mathbb{C})$
action on
![]() $\mathbb{P}^{1}(\mathbb{C})$
.
$\mathbb{P}^{1}(\mathbb{C})$
.
We have assumed
![]() $T\not \in \text{SL}_{2}(\mathbb{R})\,\cup \,i\text{SL}_{2}(\mathbb{R})\big(\!\begin{smallmatrix}1 & 0\\ 0 & -1\end{smallmatrix}\!\big)$
. Hence, by simplicity,
$T\not \in \text{SL}_{2}(\mathbb{R})\,\cup \,i\text{SL}_{2}(\mathbb{R})\big(\!\begin{smallmatrix}1 & 0\\ 0 & -1\end{smallmatrix}\!\big)$
. Hence, by simplicity,
![]() $T(\mathfrak{sl}(2,\mathbb{R}))\cap \mathfrak{sl}(2,\mathbb{R})=\{0\}$
. In particular, we may take any
$T(\mathfrak{sl}(2,\mathbb{R}))\cap \mathfrak{sl}(2,\mathbb{R})=\{0\}$
. In particular, we may take any
![]() $w\in \mathfrak{sl}(2,\mathbb{Q})$
, and obtain
$w\in \mathfrak{sl}(2,\mathbb{Q})$
, and obtain
![]() $T(w)\not \in \mathfrak{sl}(2,\mathbb{R})$
. We may now conclude that for some appropriate choice of
$T(w)\not \in \mathfrak{sl}(2,\mathbb{R})$
. We may now conclude that for some appropriate choice of
![]() $\unicode[STIX]{x1D6FE}_{2},\unicode[STIX]{x1D6FE}_{3},\unicode[STIX]{x1D6FE}_{4}\in \unicode[STIX]{x1D6E4}$
, we have
$\unicode[STIX]{x1D6FE}_{2},\unicode[STIX]{x1D6FE}_{3},\unicode[STIX]{x1D6FE}_{4}\in \unicode[STIX]{x1D6E4}$
, we have
Let
![]() $W$
denote this basis, where we have chosen
$W$
denote this basis, where we have chosen
![]() $w\in \mathfrak{sl}(2,\mathbb{Z})$
.
$w\in \mathfrak{sl}(2,\mathbb{Z})$
.
We may actually conclude that
![]() $W$
is a
$W$
is a
![]() $\mathbb{Q}$
-basis of
$\mathbb{Q}$
-basis of
![]() $\mathfrak{sl}(2,K_{d})$
, which is 3-dimensional over
$\mathfrak{sl}(2,K_{d})$
, which is 3-dimensional over
![]() $K_{d}$
, hence 6-dimensional over
$K_{d}$
, hence 6-dimensional over
![]() $\mathbb{Q}$
. We may extend scalars and find that
$\mathbb{Q}$
. We may extend scalars and find that
![]() $W$
is also a
$W$
is also a
![]() $\mathbb{Q}_{p}$
-basis of
$\mathbb{Q}_{p}$
-basis of
![]() $\mathfrak{sl}(2,\mathbb{Q}_{p}\otimes _{\mathbb{Q}}K_{d})$
.
$\mathfrak{sl}(2,\mathbb{Q}_{p}\otimes _{\mathbb{Q}}K_{d})$
.
We have therefore found the desired
![]() $\mathbb{Q}_{p}$
-basis of
$\mathbb{Q}_{p}$
-basis of
![]() $\text{SL}_{2}(\mathbb{Q}_{p}\otimes _{\mathbb{Q}}K_{d})$
, namely
$\text{SL}_{2}(\mathbb{Q}_{p}\otimes _{\mathbb{Q}}K_{d})$
, namely
![]() $W$
.
$W$
.
Next we define
![]() $m_{p}$
. Since
$m_{p}$
. Since
![]() $\unicode[STIX]{x1D6E4}$
is Zariski dense, for each
$\unicode[STIX]{x1D6E4}$
is Zariski dense, for each
![]() $p$
we can find a positive
$p$
we can find a positive
![]() $m_{p}^{\prime }$
such that for all
$m_{p}^{\prime }$
such that for all
![]() $m\geqslant m_{p}^{\prime }$
,
$m\geqslant m_{p}^{\prime }$
,
![]() $\unicode[STIX]{x1D6E4}(p^{m})$
is dense in
$\unicode[STIX]{x1D6E4}(p^{m})$
is dense in
![]() $\text{SL}_{2}(\mathbb{Z}_{p})(p^{m})$
. For technical reasons, we take
$\text{SL}_{2}(\mathbb{Z}_{p})(p^{m})$
. For technical reasons, we take
![]() $m_{p}=m_{p}^{\prime }+\unicode[STIX]{x1D704}_{p}$
where
$m_{p}=m_{p}^{\prime }+\unicode[STIX]{x1D704}_{p}$
where
![]() $\unicode[STIX]{x1D704}_{p}$
is the smallest non-negative integer so that
$\unicode[STIX]{x1D704}_{p}$
is the smallest non-negative integer so that
This
![]() $\unicode[STIX]{x1D704}_{p}$
is necessarily finite. In the case where
$\unicode[STIX]{x1D704}_{p}$
is necessarily finite. In the case where
![]() $W$
is a
$W$
is a
![]() $\mathbb{Z}_{p}$
-integral basis of
$\mathbb{Z}_{p}$
-integral basis of
![]() $\mathfrak{sl}(2,\mathbb{Z}_{p}\otimes _{\mathbb{Z}}{\mathcal{O}}_{K})$
, then
$\mathfrak{sl}(2,\mathbb{Z}_{p}\otimes _{\mathbb{Z}}{\mathcal{O}}_{K})$
, then
![]() $\unicode[STIX]{x1D704}_{p}=0$
, and the technical condition may be dropped in the sense that
$\unicode[STIX]{x1D704}_{p}=0$
, and the technical condition may be dropped in the sense that
![]() $m_{p}=m_{p}^{\prime }$
.
$m_{p}=m_{p}^{\prime }$
.
Next, we prove the following claim: for any
![]() $g\in \text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{m})$
and any
$g\in \text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{m})$
and any
![]() $m\geqslant m_{p}$
, we may express
$m\geqslant m_{p}$
, we may express
![]() $g$
as
$g$
as
for some
![]() $L_{1},L_{2},L_{3},L_{4}\in \unicode[STIX]{x1D6E4}$
. This would imply
$L_{1},L_{2},L_{3},L_{4}\in \unicode[STIX]{x1D6E4}$
. This would imply
![]() $g\in A_{4}(p^{m})$
.
$g\in A_{4}(p^{m})$
.
We prove this by induction. The base case
![]() $m=m_{p}$
is trivial. Suppose for
$m=m_{p}$
is trivial. Suppose for
![]() $m=k$
we can find
$m=k$
we can find
![]() $L_{1,k},L_{2,k},L_{3,k},L_{4,k}\in \unicode[STIX]{x1D6E4}$
such that
$L_{1,k},L_{2,k},L_{3,k},L_{4,k}\in \unicode[STIX]{x1D6E4}$
such that
Then
for some
![]() $u\in \mathfrak{sl}(2,\mathbb{Z}_{p}\otimes _{\mathbb{Z}}{\mathcal{O}}_{K})$
. Therefore, using the basis
$u\in \mathfrak{sl}(2,\mathbb{Z}_{p}\otimes _{\mathbb{Z}}{\mathcal{O}}_{K})$
. Therefore, using the basis
![]() $W$
, and the fact that
$W$
, and the fact that
![]() ${\mathcal{A}}$
,
${\mathcal{A}}$
,
![]() $R$
,
$R$
,
![]() $L$
give a
$L$
give a
![]() $\mathbb{Z}_{p}$
-integral basis for
$\mathbb{Z}_{p}$
-integral basis for
![]() $\mathfrak{sl}(2,\mathbb{Z}_{p})$
, we can find
$\mathfrak{sl}(2,\mathbb{Z}_{p})$
, we can find
![]() $u_{1}\in \mathfrak{sl}(2,\mathbb{Z}_{p})$
, and
$u_{1}\in \mathfrak{sl}(2,\mathbb{Z}_{p})$
, and
![]() $t_{2},t_{3},t_{4}\in \mathbb{Q}_{p}$
so that
$t_{2},t_{3},t_{4}\in \mathbb{Q}_{p}$
so that
If
![]() $W$
forms a
$W$
forms a
![]() $\mathbb{Z}_{p}$
-integral basis for
$\mathbb{Z}_{p}$
-integral basis for
![]() $\mathfrak{sl}(2,\mathbb{Z}_{p}\otimes _{\mathbb{Z}}{\mathcal{O}}_{K})$
, then
$\mathfrak{sl}(2,\mathbb{Z}_{p}\otimes _{\mathbb{Z}}{\mathcal{O}}_{K})$
, then
![]() $t_{i}\in \mathbb{Z}_{p}$
for
$t_{i}\in \mathbb{Z}_{p}$
for
![]() $i=2,3,4$
. Otherwise,
$i=2,3,4$
. Otherwise,
(by (8.1)). This implies
Since
![]() $\unicode[STIX]{x1D6E4}(p^{k-\unicode[STIX]{x1D704}_{p}})$
is dense in
$\unicode[STIX]{x1D6E4}(p^{k-\unicode[STIX]{x1D704}_{p}})$
is dense in
![]() $\text{SL}_{2}(\mathbb{Z}_{p})(p^{k-\unicode[STIX]{x1D704}_{p}})$
, we can find
$\text{SL}_{2}(\mathbb{Z}_{p})(p^{k-\unicode[STIX]{x1D704}_{p}})$
, we can find
![]() $\unicode[STIX]{x1D6FD}_{1},\unicode[STIX]{x1D6FD}_{2},\unicode[STIX]{x1D6FD}_{3},\unicode[STIX]{x1D6FD}_{4}\in \unicode[STIX]{x1D6E4}$
such that
$\unicode[STIX]{x1D6FD}_{1},\unicode[STIX]{x1D6FD}_{2},\unicode[STIX]{x1D6FD}_{3},\unicode[STIX]{x1D6FD}_{4}\in \unicode[STIX]{x1D6E4}$
such that
![]() $\unicode[STIX]{x1D6FD}_{i}\equiv I\;(\text{mod}\;p^{k-\unicode[STIX]{x1D704}_{p}})$
and
$\unicode[STIX]{x1D6FD}_{i}\equiv I\;(\text{mod}\;p^{k-\unicode[STIX]{x1D704}_{p}})$
and
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FD}_{1} & \equiv & \displaystyle I+p^{k}u_{1}\quad (\text{mod}\;p^{k+1}),\nonumber\\ \displaystyle \unicode[STIX]{x1D6FD}_{2} & \equiv & \displaystyle I+t_{2}p^{k}w\quad (\text{mod}\;p^{k+1}),\nonumber\\ \displaystyle \unicode[STIX]{x1D6FD}_{3} & \equiv & \displaystyle I+t_{3}p^{k}w\quad (\text{mod}\;p^{k+1}),\nonumber\\ \displaystyle \unicode[STIX]{x1D6FD}_{4} & \equiv & \displaystyle I+t_{4}p^{k}w\quad (\text{mod}\;p^{k+1}).\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FD}_{1} & \equiv & \displaystyle I+p^{k}u_{1}\quad (\text{mod}\;p^{k+1}),\nonumber\\ \displaystyle \unicode[STIX]{x1D6FD}_{2} & \equiv & \displaystyle I+t_{2}p^{k}w\quad (\text{mod}\;p^{k+1}),\nonumber\\ \displaystyle \unicode[STIX]{x1D6FD}_{3} & \equiv & \displaystyle I+t_{3}p^{k}w\quad (\text{mod}\;p^{k+1}),\nonumber\\ \displaystyle \unicode[STIX]{x1D6FD}_{4} & \equiv & \displaystyle I+t_{4}p^{k}w\quad (\text{mod}\;p^{k+1}).\nonumber\end{eqnarray}$$
Then we set
![]() $L_{i,k+1}=L_{i,k}\unicode[STIX]{x1D6FD}_{i}$
for
$L_{i,k+1}=L_{i,k}\unicode[STIX]{x1D6FD}_{i}$
for
![]() $i=1,2,3,4$
. This is enough to prove the statement for
$i=1,2,3,4$
. This is enough to prove the statement for
![]() $m=k+1$
(here, we rely on the fact that
$m=k+1$
(here, we rely on the fact that
![]() $k,k-\unicode[STIX]{x1D704}_{p}\geqslant 1$
):
$k,k-\unicode[STIX]{x1D704}_{p}\geqslant 1$
):
This completes the induction. Thus, we have
![]() $g\in A_{4}(p^{m})$
for any
$g\in A_{4}(p^{m})$
for any
![]() $g\in \text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{m})$
and any
$g\in \text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{m})$
and any
![]() $m\geqslant m_{p}$
.
$m\geqslant m_{p}$
.
Now,
![]() $[\text{SL}_{2}({\mathcal{O}}_{K}):\text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})]\leqslant p^{6m_{p}}$
. It must be that
$[\text{SL}_{2}({\mathcal{O}}_{K}):\text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})]\leqslant p^{6m_{p}}$
. It must be that
![]() $A_{1}(p^{m})$
contains something outside
$A_{1}(p^{m})$
contains something outside
![]() $\text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{m})$
. Therefore,
$\text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{m})$
. Therefore,
![]() $A_{4+1}(p^{m})$
contains at least two cosets,
$A_{4+1}(p^{m})$
contains at least two cosets,
![]() $A_{4+2}(p^{m})$
contains at least three cosets, and so forth. So if we set
$A_{4+2}(p^{m})$
contains at least three cosets, and so forth. So if we set
![]() $k_{p}=4+p^{6m_{p}}$
, we have
$k_{p}=4+p^{6m_{p}}$
, we have
![]() $A_{k_{p}}(p^{m})={\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(p^{m})$
for all
$A_{k_{p}}(p^{m})={\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(p^{m})$
for all
![]() $m\geqslant m_{p}$
.◻
$m\geqslant m_{p}$
.◻
Proof of Lemma 8.5.
For each
![]() $p$
and
$p$
and
![]() $m$
, there is a
$m$
, there is a
![]() $k_{p}$
such that
$k_{p}$
such that
For
![]() $p\in {\mathcal{S}}$
, this
$p\in {\mathcal{S}}$
, this
![]() $k_{p,m}$
is uniform with respect to
$k_{p,m}$
is uniform with respect to
![]() $p$
(Lemma 8.6), while for any fixed
$p$
(Lemma 8.6), while for any fixed
![]() $p\notin {\mathcal{S}}$
, this
$p\notin {\mathcal{S}}$
, this
![]() $k_{p,m}$
is uniform for
$k_{p,m}$
is uniform for
![]() $m\geqslant m_{p}$
(Lemma 8.7). As
$m\geqslant m_{p}$
(Lemma 8.7). As
![]() ${\mathcal{S}}$
is finite, the supremum of the
${\mathcal{S}}$
is finite, the supremum of the
![]() $k_{p,m}$
is finite, say
$k_{p,m}$
is finite, say
![]() $k_{0}$
. Therefore,
$k_{0}$
. Therefore,
for any
![]() $p$
,
$p$
,
![]() $m$
.
$m$
.
We have assumed
![]() $\unicode[STIX]{x1D6E4}$
and therefore
$\unicode[STIX]{x1D6E4}$
and therefore
![]() $\unicode[STIX]{x1D6E4}^{\prime }$
have a multiplicative structure. In other words, for any
$\unicode[STIX]{x1D6E4}^{\prime }$
have a multiplicative structure. In other words, for any
![]() $q=\prod _{i}p_{i}^{n_{i}}$
, we have
$q=\prod _{i}p_{i}^{n_{i}}$
, we have
 $$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(q)\cong \mathop{\prod }_{i}\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p_{i}^{n_{i}}), & \displaystyle \nonumber\\ \displaystyle & \displaystyle \unicode[STIX]{x1D6E4}^{\prime }/\unicode[STIX]{x1D6E4}^{\prime }(q)\cong \mathop{\prod }_{i}\unicode[STIX]{x1D6E4}^{\prime }/\unicode[STIX]{x1D6E4}^{\prime }(p_{i}^{n_{i}}). & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(q)\cong \mathop{\prod }_{i}\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p_{i}^{n_{i}}), & \displaystyle \nonumber\\ \displaystyle & \displaystyle \unicode[STIX]{x1D6E4}^{\prime }/\unicode[STIX]{x1D6E4}^{\prime }(q)\cong \mathop{\prod }_{i}\unicode[STIX]{x1D6E4}^{\prime }/\unicode[STIX]{x1D6E4}^{\prime }(p_{i}^{n_{i}}). & \displaystyle \nonumber\end{eqnarray}$$
A direct corollary is that
![]() ${\mathcal{A}}^{\prime }$
also has a multiplicative structure,
${\mathcal{A}}^{\prime }$
also has a multiplicative structure,
since
![]() ${\mathcal{A}}^{\prime }$
is generated by
${\mathcal{A}}^{\prime }$
is generated by
![]() $\unicode[STIX]{x1D6E4}$
and
$\unicode[STIX]{x1D6E4}$
and
![]() $\unicode[STIX]{x1D6E4}^{\prime }$
, and that
$\unicode[STIX]{x1D6E4}^{\prime }$
, and that
![]() $A_{k_{0}}$
has a multiplicative structure,
$A_{k_{0}}$
has a multiplicative structure,
These isomorphisms are compatible so that the composition of isomorphisms
is the identity map. Therefore,
as desired. ◻
Proof of Proposition 8.2.
We verify the hypotheses of Lemma 8.4 for
![]() $G={\mathcal{A}}^{\prime }$
,
$G={\mathcal{A}}^{\prime }$
,
![]() $S=S^{\prime }\,\cup \,TS^{\prime }T^{-1}$
, where
$S=S^{\prime }\,\cup \,TS^{\prime }T^{-1}$
, where
![]() $S$
is a finite set of generators for
$S$
is a finite set of generators for
![]() $\unicode[STIX]{x1D6E4}$
,
$\unicode[STIX]{x1D6E4}$
,
![]() $k=2k_{0}$
, and
$k=2k_{0}$
, and
![]() $(G_{1},G_{2},\ldots ,G_{2k_{0}})=(\unicode[STIX]{x1D6E4},\unicode[STIX]{x1D6E4}^{\prime },\unicode[STIX]{x1D6E4},\unicode[STIX]{x1D6E4}^{\prime },\ldots ,\unicode[STIX]{x1D6E4}^{\prime })$
. The group
$(G_{1},G_{2},\ldots ,G_{2k_{0}})=(\unicode[STIX]{x1D6E4},\unicode[STIX]{x1D6E4}^{\prime },\unicode[STIX]{x1D6E4},\unicode[STIX]{x1D6E4}^{\prime },\ldots ,\unicode[STIX]{x1D6E4}^{\prime })$
. The group
![]() $\unicode[STIX]{x1D6E4}$
has a spectral gap as a Zariski dense subgroup of
$\unicode[STIX]{x1D6E4}$
has a spectral gap as a Zariski dense subgroup of
![]() $\text{SL}_{2}(\mathbb{Z})$
, by [Reference Bourgain and VarjúBV12]; hence
$\text{SL}_{2}(\mathbb{Z})$
, by [Reference Bourgain and VarjúBV12]; hence
![]() $\unicode[STIX]{x1D6E4}^{\prime }$
does also. The second hypothesis is immediate, and the third is verified by Lemma 8.5. Therefore
$\unicode[STIX]{x1D6E4}^{\prime }$
does also. The second hypothesis is immediate, and the third is verified by Lemma 8.5. Therefore
![]() ${\mathcal{A}}^{\prime }$
has a combinatorial spectral gap.◻
${\mathcal{A}}^{\prime }$
has a combinatorial spectral gap.◻
Next, we wish to pass from
![]() ${\mathcal{A}}^{\prime }$
to
${\mathcal{A}}^{\prime }$
to
![]() ${\mathcal{A}}$
.
${\mathcal{A}}$
.
Proposition 8.8.
![]() ${\mathcal{A}}$
has a combinatorial spectral gap.
${\mathcal{A}}$
has a combinatorial spectral gap.
Before proving this, we note that our main spectral theorem follows immediately.
Proof of Theorem 1.3.
The theorem follows from the fact that
![]() ${\mathcal{A}}$
has a combinatorial spectral gap (Proposition 8.8) and from a version of [Reference Bourgain, Gamburd and SarnakBGS11, Theorem 1.2] for
${\mathcal{A}}$
has a combinatorial spectral gap (Proposition 8.8) and from a version of [Reference Bourgain, Gamburd and SarnakBGS11, Theorem 1.2] for
![]() $\text{SL}_{2}({\mathcal{O}}_{K})$
giving equivalence of geometric and spectral gaps when the Hausdorff dimension of the limit set of the group is greater than
$\text{SL}_{2}({\mathcal{O}}_{K})$
giving equivalence of geometric and spectral gaps when the Hausdorff dimension of the limit set of the group is greater than
![]() $1$
, which would follow from the arguments in [Reference Bourgain, Gamburd and SarnakBGS11] modified as described in the paragraph preceding [Reference Bourgain, Gamburd and SarnakBGS11, Theorem 2.1].◻
$1$
, which would follow from the arguments in [Reference Bourgain, Gamburd and SarnakBGS11] modified as described in the paragraph preceding [Reference Bourgain, Gamburd and SarnakBGS11, Theorem 2.1].◻
To prove Proposition 8.8, we recall an equivalent condition for a combinatorial spectral gap to the one given at the beginning of this section. Given a graph
![]() $G$
and subset
$G$
and subset
![]() $V$
, write
$V$
, write
![]() $\unicode[STIX]{x2202}V$
for the set of edges joining
$\unicode[STIX]{x2202}V$
for the set of edges joining
![]() $V$
to its complement in
$V$
to its complement in
![]() $G$
. Then define the expansion ratio of
$G$
. Then define the expansion ratio of
![]() $G$
to be
$G$
to be
Let
![]() $\unicode[STIX]{x1D716}_{G}$
be the gap between the two biggest eigenvalues of the discrete Laplacian operator on
$\unicode[STIX]{x1D716}_{G}$
be the gap between the two biggest eigenvalues of the discrete Laplacian operator on
![]() $G$
. It is known the expansion ratio of
$G$
. It is known the expansion ratio of
![]() $G$
is related to
$G$
is related to
![]() $\unicode[STIX]{x1D716}_{G}$
by the inequalities [Reference KowalskiKow, Propositions 3.2.31, 3.2.33]:
$\unicode[STIX]{x1D716}_{G}$
by the inequalities [Reference KowalskiKow, Propositions 3.2.31, 3.2.33]:
where
![]() $M_{G}$
is the maximum valence of vertices in
$M_{G}$
is the maximum valence of vertices in
![]() $G$
. In particular,
$G$
. In particular,
![]() $h_{\unicode[STIX]{x1D70C}_{q}G}$
is bounded away from
$h_{\unicode[STIX]{x1D70C}_{q}G}$
is bounded away from
![]() $0$
uniformly with respect to
$0$
uniformly with respect to
![]() $q$
if and only if
$q$
if and only if
![]() $G$
satisfies a combinatorial spectral gap.
$G$
satisfies a combinatorial spectral gap.
Proof of Proposition 8.8.
We will demonstrate the existence of a positive constant
![]() $h$
such that for any positive integer
$h$
such that for any positive integer
![]() $q$
, and any
$q$
, and any
![]() $V\subset {\mathcal{A}}/{\mathcal{A}}(q)$
with
$V\subset {\mathcal{A}}/{\mathcal{A}}(q)$
with
![]() $|V|\leqslant \frac{1}{2}|{\mathcal{A}}/{\mathcal{A}}(q)|$
, we have
$|V|\leqslant \frac{1}{2}|{\mathcal{A}}/{\mathcal{A}}(q)|$
, we have
We use the corresponding property for
![]() ${\mathcal{A}}^{\prime }$
(which has a combinatorial spectral gap by Proposition 8.2). Let
${\mathcal{A}}^{\prime }$
(which has a combinatorial spectral gap by Proposition 8.2). Let
![]() $h_{0}$
be such that for any positive integer
$h_{0}$
be such that for any positive integer
![]() $q$
and any
$q$
and any
![]() $V\subset {\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
with
$V\subset {\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
with
![]() $|V|\leqslant \frac{1}{2}|{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)|$
, we have
$|V|\leqslant \frac{1}{2}|{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)|$
, we have
By the strong approximation property for
![]() ${\mathcal{A}}$
and
${\mathcal{A}}$
and
![]() ${\mathcal{A}}^{\prime }$
, there is a universal
${\mathcal{A}}^{\prime }$
, there is a universal
![]() $M$
such that the index
$M$
such that the index
![]() $[{\mathcal{A}}/{\mathcal{A}}(q):{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)]\leqslant M$
. Let
$[{\mathcal{A}}/{\mathcal{A}}(q):{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)]\leqslant M$
. Let
![]() $S$
be a finite generating set for
$S$
be a finite generating set for
![]() ${\mathcal{A}}$
, which is symmetric under inverses (this exists since we assume
${\mathcal{A}}$
, which is symmetric under inverses (this exists since we assume
![]() ${\mathcal{A}}$
is geometrically finite, hence finitely generated). We say that two cosets
${\mathcal{A}}$
is geometrically finite, hence finitely generated). We say that two cosets
![]() $a{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
and
$a{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
and
![]() $a^{\prime }{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
are connected if there exists some
$a^{\prime }{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
are connected if there exists some
![]() $s\in S$
such that
$s\in S$
such that
![]() $sa{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)=a^{\prime }{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
. By the symmetry of
$sa{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)=a^{\prime }{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
. By the symmetry of
![]() $S$
, this connectedness is an equivalence relation.
$S$
, this connectedness is an equivalence relation.
Fix
![]() $q$
. Let
$q$
. Let
![]() $a_{1}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q),\ldots ,a_{l}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
be the cosets of
$a_{1}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q),\ldots ,a_{l}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
be the cosets of
![]() ${\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
in
${\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
in
![]() ${\mathcal{A}}/{\mathcal{A}}(q)$
, with
${\mathcal{A}}/{\mathcal{A}}(q)$
, with
![]() $l\leqslant M$
. If
$l\leqslant M$
. If
![]() $l=1$
, then (8.3) follows trivially from (8.4), with
$l=1$
, then (8.3) follows trivially from (8.4), with
![]() $h=h_{0}$
, for this value of
$h=h_{0}$
, for this value of
![]() $q$
. Therefore, assume
$q$
. Therefore, assume
![]() $l\geqslant 2$
.
$l\geqslant 2$
.
Let
![]() $V_{i}=V\cap a_{i}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
and define
$V_{i}=V\cap a_{i}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
and define
Case 1:
![]() $\unicode[STIX]{x1D705}\leqslant |V|/10l^{2}$
. Then we have
$\unicode[STIX]{x1D705}\leqslant |V|/10l^{2}$
. Then we have
From this, one finds that for each
![]() $i$
,
$i$
,
Case 1a: If
![]() $|V|\leqslant \frac{10}{22}|{\mathcal{A}}/{\mathcal{A}}(q)|$
, then each
$|V|\leqslant \frac{10}{22}|{\mathcal{A}}/{\mathcal{A}}(q)|$
, then each
![]() $|V_{i}|\leqslant \frac{1}{2}|{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)|$
. Applying (8.4), we have
$|V_{i}|\leqslant \frac{1}{2}|{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)|$
. Applying (8.4), we have
Therefore,
Case 1b: If
![]() $|V|\geqslant \frac{10}{22}|{\mathcal{A}}/{\mathcal{A}}(q)|$
, then from (8.5) we have
$|V|\geqslant \frac{10}{22}|{\mathcal{A}}/{\mathcal{A}}(q)|$
, then from (8.5) we have
And then it can be found that
Case 2:
![]() $\unicode[STIX]{x1D705}\geqslant |V|/10l^{2}$
. There exists
$\unicode[STIX]{x1D705}\geqslant |V|/10l^{2}$
. There exists
![]() $s\in S$
such that
$s\in S$
such that
![]() $sa_{i}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)=a_{j}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
and
$sa_{i}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)=a_{j}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
and
![]() $\big||V_{i}|-|V_{j}|\big|=\unicode[STIX]{x1D705}$
. Since multiplication by
$\big||V_{i}|-|V_{j}|\big|=\unicode[STIX]{x1D705}$
. Since multiplication by
![]() $s$
is a bijection between
$s$
is a bijection between
![]() $a_{i}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
and
$a_{i}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
and
![]() $a_{j}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
, by the pigeonhole principle, multiplication by
$a_{j}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)$
, by the pigeonhole principle, multiplication by
![]() $s$
must map at least
$s$
must map at least
![]() $\unicode[STIX]{x1D705}$
elements from the bigger set, say
$\unicode[STIX]{x1D705}$
elements from the bigger set, say
![]() $V_{i}$
, to
$V_{i}$
, to
![]() $a_{j}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)-V_{j}$
, so we have at least
$a_{j}{\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(q)-V_{j}$
, so we have at least
Combining (8.6)–(8.8), we find we can set
![]() $h=\min \{9h_{0}/11,1/10M^{2}\}$
.◻
$h=\min \{9h_{0}/11,1/10M^{2}\}$
.◻
Lastly, we prove the statement of explicit strong approximation, with reference to the proof of the spectral gap just completed.
Proof of Theorem 8.1.
First, we isolate the primes
![]() $p$
for which
$p$
for which
![]() ${\mathcal{A}}/{\mathcal{A}}(p)\neq \text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p)$
. Lemma 8.6 shows that
${\mathcal{A}}/{\mathcal{A}}(p)\neq \text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p)$
. Lemma 8.6 shows that
![]() ${\mathcal{A}}/{\mathcal{A}}(p)=\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p)$
for ‘good’ primes, but in the course of the proof we throw a variety of primes into
${\mathcal{A}}/{\mathcal{A}}(p)=\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p)$
for ‘good’ primes, but in the course of the proof we throw a variety of primes into
![]() ${\mathcal{S}}$
, for which we do not prove this; they are to be dealt with as bad primes. The first class of primes placed in
${\mathcal{S}}$
, for which we do not prove this; they are to be dealt with as bad primes. The first class of primes placed in
![]() ${\mathcal{S}}$
are those arising from the denominator of
${\mathcal{S}}$
are those arising from the denominator of
![]() $\unicode[STIX]{x1D6FE}$
. The denominator of
$\unicode[STIX]{x1D6FE}$
. The denominator of
![]() $\unicode[STIX]{x1D6FE}$
is always a divisor of the curvature of
$\unicode[STIX]{x1D6FE}$
is always a divisor of the curvature of
![]() $T^{-1}\cdot \mathbb{P}^{1}(\mathbb{R})$
[Reference StangeSta18b, Proposition 3.7]. Therefore, by choice of
$T^{-1}\cdot \mathbb{P}^{1}(\mathbb{R})$
[Reference StangeSta18b, Proposition 3.7]. Therefore, by choice of
![]() $T$
(applying an element of
$T$
(applying an element of
![]() $\unicode[STIX]{x1D6E4}$
to
$\unicode[STIX]{x1D6E4}$
to
![]() $T^{-1}$
), we can avoid any prime not dividing all curvatures in
$T^{-1}$
), we can avoid any prime not dividing all curvatures in
![]() ${\mathcal{A}}\cdot \mathbb{P}^{1}(\mathbb{R})$
. The second class of primes removed are those not coprime to
${\mathcal{A}}\cdot \mathbb{P}^{1}(\mathbb{R})$
. The second class of primes removed are those not coprime to
![]() $s$
. However, by choice of
$s$
. However, by choice of
![]() $s$
, we can again avoid any odd prime not dividing the curvature
$s$
, we can again avoid any odd prime not dividing the curvature
![]() $b$
(since
$b$
(since
![]() $r=1/b$
, so that
$r=1/b$
, so that
![]() $s=(y\pm 1)/b$
for some integer
$s=(y\pm 1)/b$
for some integer
![]() $y$
[Reference StangeSta18b, Proposition 3.7]). Other primes moved to
$y$
[Reference StangeSta18b, Proposition 3.7]). Other primes moved to
![]() ${\mathcal{S}}$
during the proof are those
${\mathcal{S}}$
during the proof are those
![]() $p$
for which
$p$
for which
![]() $\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p^{m})\neq \text{SL}_{2}(\mathbb{Z}/p^{m}\mathbb{Z})$
for some
$\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p^{m})\neq \text{SL}_{2}(\mathbb{Z}/p^{m}\mathbb{Z})$
for some
![]() $m\geqslant 1$
, and the special primes
$m\geqslant 1$
, and the special primes
![]() $p=2,3$
. Note that if
$p=2,3$
. Note that if
![]() $\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p)=\text{SL}_{2}(\mathbb{Z}/p\mathbb{Z})$
, then by a lemma due to Serre [Reference SerreSer89, Lemma 3, p. IV-23] one automatically has that
$\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p)=\text{SL}_{2}(\mathbb{Z}/p\mathbb{Z})$
, then by a lemma due to Serre [Reference SerreSer89, Lemma 3, p. IV-23] one automatically has that
![]() $\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p^{m})=\text{SL}_{2}(\mathbb{Z}/p^{m}\mathbb{Z})$
. Hence the statement in part 3(b) of Theorem 8.1 is equivalent to
$\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p^{m})=\text{SL}_{2}(\mathbb{Z}/p^{m}\mathbb{Z})$
. Hence the statement in part 3(b) of Theorem 8.1 is equivalent to
![]() $\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p^{m})\neq \text{SL}_{2}(\mathbb{Z}/p^{m}\mathbb{Z})$
for some
$\unicode[STIX]{x1D6E4}/\unicode[STIX]{x1D6E4}(p^{m})\neq \text{SL}_{2}(\mathbb{Z}/p^{m}\mathbb{Z})$
for some
![]() $m\geqslant 1$
. For all primes not contained in
$m\geqslant 1$
. For all primes not contained in
![]() ${\mathcal{S}}$
, the proof demonstrates that
${\mathcal{S}}$
, the proof demonstrates that
![]() ${\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(p)=\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p)$
, which implies
${\mathcal{A}}^{\prime }/{\mathcal{A}}^{\prime }(p)=\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p)$
, which implies
![]() ${\mathcal{A}}/{\mathcal{A}}(p)=\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p)$
.
${\mathcal{A}}/{\mathcal{A}}(p)=\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p)$
.
Now let
![]() $P_{\text{bad}}$
be the product of the primes of
$P_{\text{bad}}$
be the product of the primes of
![]() ${\mathcal{S}}$
as above. We obtain parts (1) and (2) immediately from the fact that
${\mathcal{S}}$
as above. We obtain parts (1) and (2) immediately from the fact that
![]() ${\mathcal{A}}/{\mathcal{A}}(p)=\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p)$
for all other primes. Part (4) is by definition.
${\mathcal{A}}/{\mathcal{A}}(p)=\text{SL}_{2}({\mathcal{O}}_{K})/\text{SL}_{2}({\mathcal{O}}_{K})(p)$
for all other primes. Part (4) is by definition.
It remains to prove part (3). Let
![]() $p|P_{\text{bad}}$
. In the course of the proof of Lemma 8.7, we find that
$p|P_{\text{bad}}$
. In the course of the proof of Lemma 8.7, we find that
![]() ${\mathcal{A}}^{\prime }(p^{m_{p}})/{\mathcal{A}}^{\prime }(p^{k})=\text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{k})$
, where by judicious choice of the basis
${\mathcal{A}}^{\prime }(p^{m_{p}})/{\mathcal{A}}^{\prime }(p^{k})=\text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{k})$
, where by judicious choice of the basis
![]() $W$
in the proof,
$W$
in the proof,
![]() $m_{p}=m_{p}^{\prime }+\unicode[STIX]{x1D704}_{p}$
where
$m_{p}=m_{p}^{\prime }+\unicode[STIX]{x1D704}_{p}$
where
![]() $\unicode[STIX]{x1D704}_{p}$
is as defined as the smallest non-negative integer so that
$\unicode[STIX]{x1D704}_{p}$
is as defined as the smallest non-negative integer so that
However, if the goal is only that
![]() ${\mathcal{A}}(p^{m_{p}})/{\mathcal{A}}(p^{k})=\text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{k})$
, and not a spectral gap for
${\mathcal{A}}(p^{m_{p}})/{\mathcal{A}}(p^{k})=\text{SL}_{2}({\mathcal{O}}_{K})(p^{m_{p}})/\text{SL}_{2}({\mathcal{O}}_{K})(p^{k})$
, and not a spectral gap for
![]() ${\mathcal{A}}$
, the proof of Lemma 8.7 can be modified for
${\mathcal{A}}$
, the proof of Lemma 8.7 can be modified for
![]() ${\mathcal{A}}$
instead of
${\mathcal{A}}$
instead of
![]() ${\mathcal{A}}^{\prime }$
, as follows. Using the same justification, we find that
${\mathcal{A}}^{\prime }$
, as follows. Using the same justification, we find that
![]() $\operatorname{Span}_{\mathbb{Q}_{p}}({\mathcal{A}}(\mathfrak{sl}(2,\mathbb{Q}_{p})))$
is of dimension
$\operatorname{Span}_{\mathbb{Q}_{p}}({\mathcal{A}}(\mathfrak{sl}(2,\mathbb{Q}_{p})))$
is of dimension
![]() $6$
, hence we can find a
$6$
, hence we can find a
![]() $\mathbb{Q}_{p}$
-basis of
$\mathbb{Q}_{p}$
-basis of
![]() $\mathfrak{sl}(2,\mathbb{Q}_{p}\otimes _{\mathbb{Q}}K_{d})$
of the form
$\mathfrak{sl}(2,\mathbb{Q}_{p}\otimes _{\mathbb{Q}}K_{d})$
of the form
where
![]() $w\in \mathfrak{sl}(2,\mathbb{Z}_{p})$
. We may choose
$w\in \mathfrak{sl}(2,\mathbb{Z}_{p})$
. We may choose
![]() $w$
and
$w$
and
![]() $a_{i}$
such that we have a
$a_{i}$
such that we have a
![]() $\mathbb{Z}_{p}$
-basis for
$\mathbb{Z}_{p}$
-basis for
![]() $\operatorname{Span}_{\mathbb{Z}_{p}}({\mathcal{A}}(\mathfrak{sl}(2,\mathbb{Z}_{p})))$
. Running the rest of the proof with
$\operatorname{Span}_{\mathbb{Z}_{p}}({\mathcal{A}}(\mathfrak{sl}(2,\mathbb{Z}_{p})))$
. Running the rest of the proof with
![]() $a_{i}$
in place of
$a_{i}$
in place of
![]() $\unicode[STIX]{x1D6FE}_{i}T$
, we no longer obtain a spectral gap but we obtain surjectivity with the stated
$\unicode[STIX]{x1D6FE}_{i}T$
, we no longer obtain a spectral gap but we obtain surjectivity with the stated
![]() $\unicode[STIX]{x1D704}_{p}$
.◻
$\unicode[STIX]{x1D704}_{p}$
.◻
9 Example packings
As discussed in the introduction, Kontorovich and Nakamura present a collection of examples which satisfy the hypotheses of Theorem 1.6. Here we first present one explicit example appearing in Kontorovich and Nakamura satisfying the hypotheses of Theorem 1.6. Second, we verify that the hypotheses hold for the entire family of
![]() $K$
-Apollonian packings.
$K$
-Apollonian packings.
9.1 A cuboctohedral packing
The packing presented here is neither the Apollonian packing, nor any
![]() $K$
-Apollonian packing, but it appears as an example of a super-integral polyhedral packing of Kontorovich and Nakamura [Reference Kontorovich and NakamuraKN17]. The packing is shown in Figure 1, where cuboctahedral symmetry is evident.
$K$
-Apollonian packing, but it appears as an example of a super-integral polyhedral packing of Kontorovich and Nakamura [Reference Kontorovich and NakamuraKN17]. The packing is shown in Figure 1, where cuboctahedral symmetry is evident.
Define
 $$\begin{eqnarray}\displaystyle & \displaystyle G_{1}=\langle c_{1}(z)=\overline{z}+\sqrt{-6},~c_{2}(z)=\frac{\overline{z}}{(-\sqrt{-6}/6)\overline{z}+1},~c_{3}(z)=\frac{(1+\sqrt{-6})\overline{z}-3\sqrt{-6}}{(\sqrt{-6}/3)\overline{z}+1-\sqrt{-6}}\rangle , & \displaystyle \nonumber\\ \displaystyle & \displaystyle G_{2}=\langle a_{1}(z)=-\overline{z},~a_{2}(z)=-\overline{z}+6,~a_{3}(z)=\frac{\overline{z}}{\overline{z}-1},~a_{4}(z)=\frac{5\overline{z}-12}{2\overline{z}-5}\rangle . & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle G_{1}=\langle c_{1}(z)=\overline{z}+\sqrt{-6},~c_{2}(z)=\frac{\overline{z}}{(-\sqrt{-6}/6)\overline{z}+1},~c_{3}(z)=\frac{(1+\sqrt{-6})\overline{z}-3\sqrt{-6}}{(\sqrt{-6}/3)\overline{z}+1-\sqrt{-6}}\rangle , & \displaystyle \nonumber\\ \displaystyle & \displaystyle G_{2}=\langle a_{1}(z)=-\overline{z},~a_{2}(z)=-\overline{z}+6,~a_{3}(z)=\frac{\overline{z}}{\overline{z}-1},~a_{4}(z)=\frac{5\overline{z}-12}{2\overline{z}-5}\rangle . & \displaystyle \nonumber\end{eqnarray}$$
Define
![]() ${\mathcal{A}}^{\prime \prime }$
as a group generated by the 14 reflections
${\mathcal{A}}^{\prime \prime }$
as a group generated by the 14 reflections
Note that
These 14 reflections correspond to the 14 faces of a cuboctahedron. The fundamental domain therefore consists of hyperbolic upper half 3-space minus
![]() $14$
tangent geodesic hemispheres. This shows that
$14$
tangent geodesic hemispheres. This shows that
![]() ${\mathcal{A}}^{\prime \prime }$
is of infinite covolume but geometrically finite.
${\mathcal{A}}^{\prime \prime }$
is of infinite covolume but geometrically finite.
Let
![]() ${\mathcal{A}}={\mathcal{A}}^{\prime \prime }\cap \operatorname{PSL}_{2}({\mathcal{O}}_{K})$
. The limit set of
${\mathcal{A}}={\mathcal{A}}^{\prime \prime }\cap \operatorname{PSL}_{2}({\mathcal{O}}_{K})$
. The limit set of
![]() ${\mathcal{A}}^{\prime \prime }$
is shown in Figure 1. Since
${\mathcal{A}}^{\prime \prime }$
is shown in Figure 1. Since
![]() $[{\mathcal{A}}^{\prime \prime }:{\mathcal{A}}]$
is finite, this limit set is the closure of a union of finitely many
$[{\mathcal{A}}^{\prime \prime }:{\mathcal{A}}]$
is finite, this limit set is the closure of a union of finitely many
![]() $K$
-rational Möbius images of a single circle orbit; in this case, of
$K$
-rational Möbius images of a single circle orbit; in this case, of
![]() ${\mathcal{A}}C$
where
${\mathcal{A}}C$
where
![]() $C=\widehat{\mathbb{R}}+\sqrt{-6}$
. Therefore we aim to demonstrate that
$C=\widehat{\mathbb{R}}+\sqrt{-6}$
. Therefore we aim to demonstrate that
![]() ${\mathcal{A}}$
is an infinite-covolume, geometrically finite, Zariski dense, familial Kleinian group.
${\mathcal{A}}$
is an infinite-covolume, geometrically finite, Zariski dense, familial Kleinian group.
The geometric finiteness and infinite covolume are inherited by
![]() ${\mathcal{A}}$
from
${\mathcal{A}}$
from
![]() ${\mathcal{A}}^{\prime \prime }$
, as it is finite index. By arguments exactly analogous to those in [Reference StangeSta18a, Theorems 9.3–9.4], the limit sets of
${\mathcal{A}}^{\prime \prime }$
, as it is finite index. By arguments exactly analogous to those in [Reference StangeSta18a, Theorems 9.3–9.4], the limit sets of
![]() ${\mathcal{A}}^{\prime \prime }$
and
${\mathcal{A}}^{\prime \prime }$
and
![]() ${\mathcal{A}}$
have Hausdorff dimension greater than
${\mathcal{A}}$
have Hausdorff dimension greater than
![]() $1$
and are Zariski dense.
$1$
and are Zariski dense.
It simply remains to prove the following lemma.
Lemma 9.1. The group
![]() $G_{2}$
is a congruence subgroup of
$G_{2}$
is a congruence subgroup of
![]() $\operatorname{PGL}_{2}(\mathbb{Z})$
.
$\operatorname{PGL}_{2}(\mathbb{Z})$
.
This implies
![]() $G_{2}\cap {\mathcal{A}}$
is a congruence subgroup of
$G_{2}\cap {\mathcal{A}}$
is a congruence subgroup of
![]() $\operatorname{PSL}_{2}(\mathbb{Z})$
.
$\operatorname{PSL}_{2}(\mathbb{Z})$
.
Proof. We will show that
![]() $G_{2}$
contains the principal congruence subgroup
$G_{2}$
contains the principal congruence subgroup
![]() $\unicode[STIX]{x1D6E4}(6)$
. Let
$\unicode[STIX]{x1D6E4}(6)$
. Let
These matrices generate
![]() $\operatorname{PSL}_{2}(\mathbb{Z})$
. We will use the fact that
$\operatorname{PSL}_{2}(\mathbb{Z})$
. We will use the fact that
![]() $\unicode[STIX]{x1D6E4}(6)$
is the subgroup generated by the following elements [Reference HsuHsu96, p. 1357]:
$\unicode[STIX]{x1D6E4}(6)$
is the subgroup generated by the following elements [Reference HsuHsu96, p. 1357]:
![]() $L^{6},R^{6},L^{2}R^{3}L^{-2}R^{-3},L^{3}R^{2}L^{-3}R^{-2}$
. It suffices now to verify that
$L^{6},R^{6},L^{2}R^{3}L^{-2}R^{-3},L^{3}R^{2}L^{-3}R^{-2}$
. It suffices now to verify that
 $$\begin{eqnarray}\displaystyle & \displaystyle (a_{1}a_{2})^{-1}=L^{6}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle (a_{1}a_{3})^{-6}=R^{6}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle (a_{1}a_{4})^{-1}(a_{1}a_{3})^{4}=L^{2}R^{3}L^{-2}R^{-3}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle (a_{1}a_{2})^{-1}a_{1}a_{4}(a_{1}a_{3})^{2}=L^{3}R^{2}L^{-3}R^{-2}.\Box & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle (a_{1}a_{2})^{-1}=L^{6}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle (a_{1}a_{3})^{-6}=R^{6}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle (a_{1}a_{4})^{-1}(a_{1}a_{3})^{4}=L^{2}R^{3}L^{-2}R^{-3}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle (a_{1}a_{2})^{-1}a_{1}a_{4}(a_{1}a_{3})^{2}=L^{3}R^{2}L^{-3}R^{-2}.\Box & \displaystyle \nonumber\end{eqnarray}$$
Finally, we apply Theorem 8.1. The potential bad primes are exactly
![]() $p=2,3$
, since the curvatures of the packing are coprime and the congruence subgroup is of level
$p=2,3$
, since the curvatures of the packing are coprime and the congruence subgroup is of level
![]() $6$
. Letting
$6$
. Letting
![]() $T_{0}=a_{3}a_{1}\in {\mathcal{A}}$
and
$T_{0}=a_{3}a_{1}\in {\mathcal{A}}$
and
![]() $V=c_{1}a_{3}c_{1}a_{1}\in {\mathcal{A}}$
, and using the notation
$V=c_{1}a_{3}c_{1}a_{1}\in {\mathcal{A}}$
, and using the notation
![]() $H,L,R$
for the basis of
$H,L,R$
for the basis of
![]() $\mathfrak{sl}(2,\mathbb{Z})$
as in the proof of Lemma 8.7, one can compute the following elements of
$\mathfrak{sl}(2,\mathbb{Z})$
as in the proof of Lemma 8.7, one can compute the following elements of
![]() ${\mathcal{A}}\cdot \mathfrak{sl}(2,\mathbb{Z})$
:
${\mathcal{A}}\cdot \mathfrak{sl}(2,\mathbb{Z})$
:
These are enough to verify that
![]() $\unicode[STIX]{x1D704}_{2}\leqslant 1$
and
$\unicode[STIX]{x1D704}_{2}\leqslant 1$
and
![]() $\unicode[STIX]{x1D704}_{3}=0$
. Therefore the modulus of the congruence obstruction divides
$\unicode[STIX]{x1D704}_{3}=0$
. Therefore the modulus of the congruence obstruction divides
![]() $12$
. By way of experimental confirmation, computing curvatures up to and including
$12$
. By way of experimental confirmation, computing curvatures up to and including
![]() $159$
appearing in the limit set packing (Figure 1), we find that the curvatures missing in this range are exactly those
$159$
appearing in the limit set packing (Figure 1), we find that the curvatures missing in this range are exactly those
![]() $\equiv 7,9,11\;(\text{mod}\;12)$
as well as
$\equiv 7,9,11\;(\text{mod}\;12)$
as well as
![]() $13$
and
$13$
and
![]() $16$
.
$16$
.
9.2
 $K$
-Apollonian packings
$K$
-Apollonian packings
In this subsection we show that all
![]() $K$
-Apollonian circle packings satisfy the hypotheses of Theorem 1.6. For an example of a
$K$
-Apollonian circle packings satisfy the hypotheses of Theorem 1.6. For an example of a
![]() $K$
-Apollonian packing, see Figure 2.
$K$
-Apollonian packing, see Figure 2.
The (strong)
![]() $K$
-Apollonian groups defined in [Reference StangeSta18a] are shown there to be finitely generated Zariski dense subgroups of
$K$
-Apollonian groups defined in [Reference StangeSta18a] are shown there to be finitely generated Zariski dense subgroups of
![]() $\operatorname{PSL}_{2}({\mathcal{O}}_{K})$
containing congruence subgroups (either
$\operatorname{PSL}_{2}({\mathcal{O}}_{K})$
containing congruence subgroups (either
![]() $\unicode[STIX]{x1D6F1}(2)$
or
$\unicode[STIX]{x1D6F1}(2)$
or
![]() $\unicode[STIX]{x1D6E4}^{3}$
in the notation of [Reference StangeSta18a, § 10]). They are of infinite covolume since they are of infinite index, and each packing contains the horizontal line
$\unicode[STIX]{x1D6E4}^{3}$
in the notation of [Reference StangeSta18a, § 10]). They are of infinite covolume since they are of infinite index, and each packing contains the horizontal line
![]() $\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2$
. Therefore all the hypotheses of Theorem 1.6 are satisfied save geometric finiteness. For that, it suffices to consider the remark following Theorem 1.6.
$\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2$
. Therefore all the hypotheses of Theorem 1.6 are satisfied save geometric finiteness. For that, it suffices to consider the remark following Theorem 1.6.
However, it may be useful to give an explicit description of a group associated to the packing. For each imaginary quadratic field
![]() $K$
, we may use an adaptation of the weak
$K$
, we may use an adaptation of the weak
![]() $K$
-Apollonian group given in [Reference StangeSta18a, Theorem 9.2]:
$K$
-Apollonian group given in [Reference StangeSta18a, Theorem 9.2]:
This group has the
![]() $K$
-Apollonian packing as a limit set, and this limit set is of the form
$K$
-Apollonian packing as a limit set, and this limit set is of the form
![]() ${\mathcal{A}}^{\prime }\widehat{\mathbb{R}}={\mathcal{A}}^{\prime }(\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2)$
. It has the following fundamental domain, given here as a list of the boundaries in
${\mathcal{A}}^{\prime }\widehat{\mathbb{R}}={\mathcal{A}}^{\prime }(\widehat{\mathbb{R}}+\sqrt{\unicode[STIX]{x1D6E5}}/2)$
. It has the following fundamental domain, given here as a list of the boundaries in
![]() $\widehat{\mathbb{C}}$
of its geodesic walls:
$\widehat{\mathbb{C}}$
of its geodesic walls:
 $$\begin{eqnarray}\displaystyle & \displaystyle A:\Re (z)=0,\quad \Im (z)\leqslant \Im (\unicode[STIX]{x1D70F})/2, & \displaystyle \nonumber\\ \displaystyle & \displaystyle B:\Im (z)=\Im (\unicode[STIX]{x1D70F})/2,\quad 0\leqslant \Re (z)\leqslant 1, & \displaystyle \nonumber\\ \displaystyle & \displaystyle C:\Re (z)=1,\quad \Im (z)\leqslant \Im (\unicode[STIX]{x1D70F})/2, & \displaystyle \nonumber\\ \displaystyle & \displaystyle D:|z-1/2|=1/2. & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle A:\Re (z)=0,\quad \Im (z)\leqslant \Im (\unicode[STIX]{x1D70F})/2, & \displaystyle \nonumber\\ \displaystyle & \displaystyle B:\Im (z)=\Im (\unicode[STIX]{x1D70F})/2,\quad 0\leqslant \Re (z)\leqslant 1, & \displaystyle \nonumber\\ \displaystyle & \displaystyle C:\Re (z)=1,\quad \Im (z)\leqslant \Im (\unicode[STIX]{x1D70F})/2, & \displaystyle \nonumber\\ \displaystyle & \displaystyle D:|z-1/2|=1/2. & \displaystyle \nonumber\end{eqnarray}$$
It is straightforward to verify that this region satisfies the Poincaré polyhedron theorem and is therefore a fundamental domain for
![]() ${\mathcal{A}}$
; it is therefore geometrically finite and of infinite covolume. It has
${\mathcal{A}}$
; it is therefore geometrically finite and of infinite covolume. It has
![]() $\operatorname{PSL}_{2}(\mathbb{Z})<{\mathcal{A}}$
(in the form of the first two generators above). It is Zariski dense by the same arguments as in [Reference StangeSta18a, § 10].
$\operatorname{PSL}_{2}(\mathbb{Z})<{\mathcal{A}}$
(in the form of the first two generators above). It is Zariski dense by the same arguments as in [Reference StangeSta18a, § 10].
In order to apply Theorem 1.6, we need only pass to the finite-index subgroup
![]() ${\mathcal{A}}={\mathcal{A}}^{\prime }\cap \operatorname{PSL}_{2}({\mathcal{O}}_{K})$
, by replacing
${\mathcal{A}}={\mathcal{A}}^{\prime }\cap \operatorname{PSL}_{2}({\mathcal{O}}_{K})$
, by replacing
![]() $V$
with
$V$
with
The curvatures of the
![]() $K$
-Apollonian circle packings are primitive integral (after scaling by
$K$
-Apollonian circle packings are primitive integral (after scaling by
![]() $\sqrt{-\unicode[STIX]{x1D6E5}}$
). Therefore, with this choice of group, Theorem 8.1 tells us immediately that the only primes of bad reduction for strong approximation are
$\sqrt{-\unicode[STIX]{x1D6E5}}$
). Therefore, with this choice of group, Theorem 8.1 tells us immediately that the only primes of bad reduction for strong approximation are
![]() $2$
and
$2$
and
![]() $3$
. In fact, it tells us more. Write
$3$
. In fact, it tells us more. Write
![]() $L,R,H$
for the usual generators of
$L,R,H$
for the usual generators of
![]() $\mathfrak{sl}(2,\mathbb{Z})$
as in the proof of Lemma 8.7. Then following matrices are among
$\mathfrak{sl}(2,\mathbb{Z})$
as in the proof of Lemma 8.7. Then following matrices are among
![]() ${\mathcal{A}}\cdot \mathfrak{sl}(2,\mathbb{Z})$
:
${\mathcal{A}}\cdot \mathfrak{sl}(2,\mathbb{Z})$
:
Using these suffices to verify that for
![]() $\unicode[STIX]{x1D6E5}\equiv 0\;(\text{mod}\;4)$
,
$\unicode[STIX]{x1D6E5}\equiv 0\;(\text{mod}\;4)$
,
![]() $\unicode[STIX]{x1D704}_{2}\leqslant 2$
and
$\unicode[STIX]{x1D704}_{2}\leqslant 2$
and
![]() $\unicode[STIX]{x1D704}_{3}=0$
; while for
$\unicode[STIX]{x1D704}_{3}=0$
; while for
![]() $\unicode[STIX]{x1D6E5}\not \equiv 0\;(\text{mod}\;4)$
,
$\unicode[STIX]{x1D6E5}\not \equiv 0\;(\text{mod}\;4)$
,
![]() $\unicode[STIX]{x1D704}_{2}\leqslant 1$
and
$\unicode[STIX]{x1D704}_{2}\leqslant 1$
and
![]() $\unicode[STIX]{x1D704}_{3}=0$
. Then Theorem 8.1 tells us that the modulus of the congruence obstruction for
$\unicode[STIX]{x1D704}_{3}=0$
. Then Theorem 8.1 tells us that the modulus of the congruence obstruction for
![]() $K$
-Apollonian packings is a divisor of
$K$
-Apollonian packings is a divisor of
![]() $24$
in all cases, and in fact a divisor of
$24$
in all cases, and in fact a divisor of
![]() $12$
if
$12$
if
![]() $\unicode[STIX]{x1D6E5}\not \equiv 0\;(\text{mod}\;4)$
. This is in accordance with [Reference StangeSta18a, Conjecture 1.4], which gives an explicit prediction for the modulus for the congruence obstruction.
$\unicode[STIX]{x1D6E5}\not \equiv 0\;(\text{mod}\;4)$
. This is in accordance with [Reference StangeSta18a, Conjecture 1.4], which gives an explicit prediction for the modulus for the congruence obstruction.
Acknowledgements
We would like to thank Hee Oh for raising the question of how general the methods in [Reference Bourgain and FuchsBF11, Reference Bourgain and KontorovichBK14a] are, which is what motivated this paper. We also thank Nathan Dunfield, Alireza Salehi-Golsefidy, Alex Kontorovich, Chris Leininger, Kei Nakamura and Hee Oh for helpful conversations. We would also like to thank the referee for many very helpful and thoughtful comments.