Article contents
A Note on Fine Graphs and Homological Isoperimetric Inequalities
Published online by Cambridge University Press: 20 November 2018
Abstract
In the framework of homological characterizations of relative hyperbolicity, Groves and Manning posed the question of whether a simply connected 2-complex
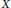 $X$
with a linear homological isoperimetric inequality, a bound on the length of attachingmaps of 2-cells, and finitely many 2-cells adjacent to any edge must have a fine 1-skeleton. We provide a positive answer to this question. We revisit a homological characterization of relative hyperbolicity and show that a group
$X$
with a linear homological isoperimetric inequality, a bound on the length of attachingmaps of 2-cells, and finitely many 2-cells adjacent to any edge must have a fine 1-skeleton. We provide a positive answer to this question. We revisit a homological characterization of relative hyperbolicity and show that a group
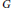 $G$
is hyperbolic relative to a collection of subgroups
$G$
is hyperbolic relative to a collection of subgroups
 $P$
if and only if
$P$
if and only if
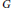 $G$
acts cocompactly with finite edge stabilizers on a connected 2-dimensional cell complex with a linear homological isoperimetric inequality and
$G$
acts cocompactly with finite edge stabilizers on a connected 2-dimensional cell complex with a linear homological isoperimetric inequality and
 $P$
is a collection of representatives of conjugacy classes of vertex stabilizers.
$P$
is a collection of representatives of conjugacy classes of vertex stabilizers.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2016
References
- 5
- Cited by


