Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Clark, Pete L.
2009.
On the Hasse principle for Shimura curves.
Israel Journal of Mathematics,
Vol. 171,
Issue. 1,
p.
349.
Cadoret, Anna
and
Dèbes, Pierre
2009.
Abelian constraints in inverse Galois theory.
manuscripta mathematica,
Vol. 128,
Issue. 3,
p.
329.
Breuer, Florian
2010.
Torsion bounds for elliptic curves and Drinfeld modules.
Journal of Number Theory,
Vol. 130,
Issue. 5,
p.
1241.
THORNE, JACK A.
2015.
AND THE ARITHMETIC OF A FAMILY OF NON-HYPERELLIPTIC CURVES OF GENUS 3.
Forum of Mathematics, Pi,
Vol. 3,
Issue. ,
Clark, Pete L.
Milosevic, Marko
and
Pollack, Paul
2018.
Typically bounding torsion.
Journal of Number Theory,
Vol. 192,
Issue. ,
p.
150.
Gaudron, Éric
and
Rémond, Gaël
2018.
Torsion des variétés abéliennes CM.
Proceedings of the American Mathematical Society,
Vol. 146,
Issue. 7,
p.
2741.
Romano, Beth
and
Thorne, Jack A.
2018.
On the arithmetic of simple singularities of type E.
Research in Number Theory,
Vol. 4,
Issue. 2,
Melistas, Mentzelos
2022.
Purely additive reduction of abelian varieties with torsion.
Journal of Number Theory,
Vol. 239,
Issue. ,
p.
21.
Ishii, Shun
2022.
On isogeny characters of Drinfeld modules of rank two.
Mathematische Zeitschrift,
Vol. 301,
Issue. 1,
p.
455.
Smith, Hanson
2022.
Frobenius finds non-monogenic division fields of abelian varieties.
International Journal of Number Theory,
Vol. 18,
Issue. 10,
p.
2299.
OZEKI, Yoshiyasu
2023.
Torsion of algebraic groups and iterate extensions associated with Lubin–Tate formal groups.
Journal of the Mathematical Society of Japan,
Vol. 75,
Issue. 2,
Melistas, Mentzelos
2024.
Reduction types of CM curves.
Pacific Journal of Mathematics,
Vol. 329,
Issue. 2,
p.
233.
Melistas, Mentzelos
2024.
Torsion and twists of abelian varieties.
Bulletin of the London Mathematical Society,
Vol. 56,
Issue. 2,
p.
589.
Zhang, Robin
2024.
The abcd conjecture, uniform boundedness, and dynamical systems.
Publications mathématiques de Besançon. Algèbre et théorie des nombres,
p.
119.
Ozeki, Yoshiyasu
2024.
Explicit bounds on torsion of CM abelian
varieties over p-adic fields with values in Lubin–Tate extensions.
Pacific Journal of Mathematics,
Vol. 330,
Issue. 1,
p.
171.
Laga, Jef
Schembri, Ciaran
Shnidman, Ari
and
Voight, John
2024.
Rational torsion points on abelian surfaces with quaternionic multiplication.
Forum of Mathematics, Sigma,
Vol. 12,
Issue. ,
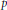 $p$-adic field
$p$-adic field 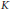 $K$ has anisotropic reduction
$K$ has anisotropic reduction 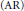 $(\text{AR})$ if the special fiber of its Néron minimal model does not contain a nontrivial split torus. This includes all abelian varieties with potentially good reduction and, in particular, those with complex or quaternionic multiplication. We give a bound for the size of the
$(\text{AR})$ if the special fiber of its Néron minimal model does not contain a nontrivial split torus. This includes all abelian varieties with potentially good reduction and, in particular, those with complex or quaternionic multiplication. We give a bound for the size of the 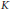 $K$-rational torsion subgroup of a
$K$-rational torsion subgroup of a 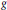 $g$-dimensional
$g$-dimensional  $\text{AR}$ variety depending only on
$\text{AR}$ variety depending only on 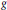 $g$ and the numerical invariants of
$g$ and the numerical invariants of 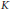 $K$ (the absolute ramification index and the cardinality of the residue field). Applying these bounds to abelian varieties over a number field with everywhere locally anisotropic reduction, we get bounds which, as a function of
$K$ (the absolute ramification index and the cardinality of the residue field). Applying these bounds to abelian varieties over a number field with everywhere locally anisotropic reduction, we get bounds which, as a function of 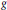 $g$, are close to optimal. In particular, we determine the possible cardinalities of the torsion subgroup of an
$g$, are close to optimal. In particular, we determine the possible cardinalities of the torsion subgroup of an  $\text{AR}$ abelian surface over the rational numbers, up to a set of 11 values which are not known to occur. The largest such value is 72.
$\text{AR}$ abelian surface over the rational numbers, up to a set of 11 values which are not known to occur. The largest such value is 72.