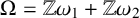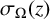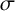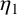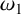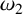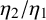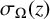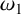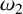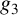1 Introduction
In 2011, Masser [Reference Masser6] proved that for any positive integer
![]() $d,$
there exists an effective constant
$d,$
there exists an effective constant
![]() $C>0$
such that for all
$C>0$
such that for all
![]() $H>e^e,$
there are at most
$H>e^e,$
there are at most
![]() $C({\log H}/{\log \log H})^2$
algebraic numbers
$C({\log H}/{\log \log H})^2$
algebraic numbers
![]() $\alpha \in (2,3)$
, with
$\alpha \in (2,3)$
, with
![]() $\zeta (\alpha )$
also algebraic, such that both
$\zeta (\alpha )$
also algebraic, such that both
![]() $\alpha ,\zeta (\alpha )$
have degrees at most d and multiplicative heights at most
$\alpha ,\zeta (\alpha )$
have degrees at most d and multiplicative heights at most
![]() $H,$
where
$H,$
where
![]() $\zeta (z)$
is the Riemann zeta function. Recall that for an algebraic number
$\zeta (z)$
is the Riemann zeta function. Recall that for an algebraic number
![]() $\alpha $
of degree
$\alpha $
of degree
![]() $d,$
its multiplicative height is defined by
$d,$
its multiplicative height is defined by
![]() $H(\alpha )=(M(\alpha ))^{1/d},$
where
$H(\alpha )=(M(\alpha ))^{1/d},$
where
![]() $M(\alpha )$
is its Mahler measure. In the same paper, Masser suggested some possible extensions of his method to other classes of functions. There have been several results already published based on his suggestions of which a recent result by Boxall et al. [Reference Boxall, Chalebgwa and Jones2] is closely related to our work. To state the main results of [Reference Boxall, Chalebgwa and Jones2], we need to introduce some notation.
$M(\alpha )$
is its Mahler measure. In the same paper, Masser suggested some possible extensions of his method to other classes of functions. There have been several results already published based on his suggestions of which a recent result by Boxall et al. [Reference Boxall, Chalebgwa and Jones2] is closely related to our work. To state the main results of [Reference Boxall, Chalebgwa and Jones2], we need to introduce some notation.
Let
![]() $\Omega $
be a lattice in
$\Omega $
be a lattice in
![]() $\mathbb {C}.$
Throughout our discussion, we fix a
$\mathbb {C}.$
Throughout our discussion, we fix a
![]() $\mathbb {Z}$
-basis
$\mathbb {Z}$
-basis
![]() $\{\omega _1,\omega _2\}$
of
$\{\omega _1,\omega _2\}$
of
![]() $\Omega $
such that
$\Omega $
such that
![]() $\tau =\omega _2/\omega _1$
lies in the upper half plane
$\tau =\omega _2/\omega _1$
lies in the upper half plane
![]() $\mathbb {H}$
of
$\mathbb {H}$
of
![]() $\mathbb {C}$
with
$\mathbb {C}$
with
![]() $|\tau |\geq 1$
and the real part of
$|\tau |\geq 1$
and the real part of
![]() $\tau $
lies in the interval
$\tau $
lies in the interval
![]() $\big[{-}\tfrac 12,\tfrac 12\big].$
Such a basis always exists. Let
$\big[{-}\tfrac 12,\tfrac 12\big].$
Such a basis always exists. Let
![]() $\eta _1$
and
$\eta _1$
and
![]() $\eta _2$
be the quasi-periods associated to
$\eta _2$
be the quasi-periods associated to
![]() $\omega _1$
and
$\omega _1$
and
![]() $\omega _2$
, respectively. For a pair
$\omega _2$
, respectively. For a pair
![]() $\alpha ,\beta $
of algebraic numbers, we put
$\alpha ,\beta $
of algebraic numbers, we put
![]() $H(\alpha ,\beta )=\max \{H(\alpha ),H(\,\beta )\}.$
In [Reference Boxall, Chalebgwa and Jones2], the authors proved two results. The first [Reference Boxall, Chalebgwa and Jones2, Theorem 1.1] is for lattices
$H(\alpha ,\beta )=\max \{H(\alpha ),H(\,\beta )\}.$
In [Reference Boxall, Chalebgwa and Jones2], the authors proved two results. The first [Reference Boxall, Chalebgwa and Jones2, Theorem 1.1] is for lattices
![]() $\Omega $
for which
$\Omega $
for which
![]() $\omega _1,\omega _2$
are algebraic: if
$\omega _1,\omega _2$
are algebraic: if
![]() $\mbox {Im}(\tau )\leq 1.9$
and
$\mbox {Im}(\tau )\leq 1.9$
and
![]() $\omega _1$
and
$\omega _1$
and
![]() $\omega _2$
are both algebraic, where
$\omega _2$
are both algebraic, where
![]() $\mbox {Im}(z)$
is the imaginary part of
$\mbox {Im}(z)$
is the imaginary part of
![]() $z,$
then there exists a constant
$z,$
then there exists a constant
![]() $C_1=C_1(\Omega )>0$
such that for all
$C_1=C_1(\Omega )>0$
such that for all
![]() $d\geq e$
and
$d\geq e$
and
![]() $H\geq e^e,$
there are at most
$H\geq e^e,$
there are at most
algebraic numbers z such that
![]() $[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d,H(z,\sigma _{\Omega }(z))\leq H$
and
$[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d,H(z,\sigma _{\Omega }(z))\leq H$
and
![]() $z\notin \Omega .$
Their second result [Reference Boxall, Chalebgwa and Jones2, Theorem 1.2] deals with the case in which the invariants
$z\notin \Omega .$
Their second result [Reference Boxall, Chalebgwa and Jones2, Theorem 1.2] deals with the case in which the invariants
are both algebraic (
![]() $\omega _1,\omega _2$
need not be algebraic) and here also
$\omega _1,\omega _2$
need not be algebraic) and here also
![]() $\mbox {Im}(\tau )\leq 1.9$
, where
$\mbox {Im}(\tau )\leq 1.9$
, where
![]() $\Omega '=\Omega \setminus \{0\}$
. In this case, instead of the bound (1.1), they give the bound
$\Omega '=\Omega \setminus \{0\}$
. In this case, instead of the bound (1.1), they give the bound
for the number of algebraic numbers z such that
![]() $[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d,H(z,\sigma _{\Omega }(z))\,{\leq}\, H.$
Here,
$[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d,H(z,\sigma _{\Omega }(z))\,{\leq}\, H.$
Here,
![]() $C_2$
is a constant depending only on
$C_2$
is a constant depending only on
![]() $\Omega .$
The importance of the results in [Reference Boxall, Chalebgwa and Jones2] is that they count algebraic points of bounded degrees and heights on the entire graph of
$\Omega .$
The importance of the results in [Reference Boxall, Chalebgwa and Jones2] is that they count algebraic points of bounded degrees and heights on the entire graph of
![]() $\sigma _{\Omega }(z)$
. Earlier, Besson [Reference Besson1] also proved similar results for the number of algebraic points on the graph of the Weierstrass
$\sigma _{\Omega }(z)$
. Earlier, Besson [Reference Besson1] also proved similar results for the number of algebraic points on the graph of the Weierstrass
![]() $\sigma $
-function, but his results are restricted to bounded domains. One of the main ingredients in [Reference Boxall, Chalebgwa and Jones2] is the lower bound of
$\sigma $
-function, but his results are restricted to bounded domains. One of the main ingredients in [Reference Boxall, Chalebgwa and Jones2] is the lower bound of
![]() $\sigma _{\Omega }(z)$
in terms of the exponential function
$\sigma _{\Omega }(z)$
in terms of the exponential function
![]() $e^z$
and the values of
$e^z$
and the values of
![]() $\sigma _{\Omega }(z)$
on the fundamental domain P enclosed by the parallelogram with vertices
$\sigma _{\Omega }(z)$
on the fundamental domain P enclosed by the parallelogram with vertices
![]() $\tfrac 12(\pm \omega _1\pm \omega _2).$
In this paper, we extend the main results of [Reference Boxall, Chalebgwa and Jones2] to a general
$\tfrac 12(\pm \omega _1\pm \omega _2).$
In this paper, we extend the main results of [Reference Boxall, Chalebgwa and Jones2] to a general
![]() $\tau \in \mathbb {H}$
under the assumption that
$\tau \in \mathbb {H}$
under the assumption that
![]() $\rho =\eta _2/\eta _1$
is a nonzero real number. With this assumption, we are only able to count the algebraic points of
$\rho =\eta _2/\eta _1$
is a nonzero real number. With this assumption, we are only able to count the algebraic points of
![]() $\sigma _{\Omega }(z)$
in an unbounded subset
$\sigma _{\Omega }(z)$
in an unbounded subset
![]() $\mathcal {A}_{\rho }$
of
$\mathcal {A}_{\rho }$
of
![]() $\mathbb {C}$
defined as follows. First, put
$\mathbb {C}$
defined as follows. First, put
and
Finally, define
 $$ \begin{align*} \mathcal{A}_{\rho}= \begin{cases} \mathcal{A}^+ & \text{if } \rho>0,\\ \mathcal{A}^- & \text{if } \rho<0. \end{cases} \end{align*} $$
$$ \begin{align*} \mathcal{A}_{\rho}= \begin{cases} \mathcal{A}^+ & \text{if } \rho>0,\\ \mathcal{A}^- & \text{if } \rho<0. \end{cases} \end{align*} $$
Our first result is an analogue of [Reference Boxall, Chalebgwa and Jones2, Theorem 1.1].
Theorem 1.1. Let
![]() $\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
be a lattice in
$\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
be a lattice in
![]() $\mathbb {C}$
such that
$\mathbb {C}$
such that
![]() $\omega _1$
and
$\omega _1$
and
![]() $\omega _2$
are both algebraic. Assume that
$\omega _2$
are both algebraic. Assume that
![]() $\rho = \eta _2/\eta _1$
is a nonzero real number. Then there exists a constant
$\rho = \eta _2/\eta _1$
is a nonzero real number. Then there exists a constant
![]() $C_3=C_3(\Omega )>0$
such that for all
$C_3=C_3(\Omega )>0$
such that for all
![]() $d\geq e$
and
$d\geq e$
and
![]() $H\geq e^e,$
there are at most
$H\geq e^e,$
there are at most
algebraic numbers z such that
![]() $z\in \mathcal {A}_{\rho }$
,
$z\in \mathcal {A}_{\rho }$
,
![]() $[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d~\mbox {and}~H(z,\sigma _{\Omega }(z))\leq H.$
$[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d~\mbox {and}~H(z,\sigma _{\Omega }(z))\leq H.$
Our second result is for
![]() $g_2,g_3$
algebraic, analogous to [Reference Boxall, Chalebgwa and Jones2, Theorem 1.2].
$g_2,g_3$
algebraic, analogous to [Reference Boxall, Chalebgwa and Jones2, Theorem 1.2].
Theorem 1.2. Let
![]() $\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
be a lattice in
$\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
be a lattice in
![]() $\mathbb {C}$
such that
$\mathbb {C}$
such that
![]() $g_2$
and
$g_2$
and
![]() $g_3$
are both algebraic. Assume that
$g_3$
are both algebraic. Assume that
![]() $\rho = \eta _2/\eta _1$
is a nonzero real number. Then there exists a constant
$\rho = \eta _2/\eta _1$
is a nonzero real number. Then there exists a constant
![]() $C_4=C_4(\Omega )>0$
such that for all
$C_4=C_4(\Omega )>0$
such that for all
![]() $d\geq e$
and
$d\geq e$
and
![]() $H\geq e^e,$
there are at most
$H\geq e^e,$
there are at most
algebraic numbers z such that
![]() $z\in \mathcal {A}_{\rho }$
,
$z\in \mathcal {A}_{\rho }$
,
![]() $[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d~\mbox {and}~H(z,\sigma _{\Omega }(z))\leq H.$
$[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d~\mbox {and}~H(z,\sigma _{\Omega }(z))\leq H.$
We also prove the following more general result with no assumptions on the quantities
![]() $\omega _1,\omega _2,g_2$
and
$\omega _1,\omega _2,g_2$
and
![]() $g_3.$
In this case, we are only able to count the algebraic points of
$g_3.$
In this case, we are only able to count the algebraic points of
![]() $\sigma _{\Omega }(z)$
which are not close to the lattice points.
$\sigma _{\Omega }(z)$
which are not close to the lattice points.
Theorem 1.3. Let
![]() $\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
be a lattice in
$\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
be a lattice in
![]() $\mathbb {C}.$
Assume that
$\mathbb {C}.$
Assume that
![]() $\rho = \eta _2/\eta _1$
is a nonzero real number. Let
$\rho = \eta _2/\eta _1$
is a nonzero real number. Let
![]() $0<\delta <\min \{1,|\omega _1+\omega _2|/2,|\omega _1-\omega _2|/2\}.$
Then there exists a constant
$0<\delta <\min \{1,|\omega _1+\omega _2|/2,|\omega _1-\omega _2|/2\}.$
Then there exists a constant
![]() $C_5=C_5(\delta ,\Omega )>0$
such that for all
$C_5=C_5(\delta ,\Omega )>0$
such that for all
![]() $d\geq e$
and
$d\geq e$
and
![]() $H\geq e^e,$
there are at most
$H\geq e^e,$
there are at most
algebraic numbers z such that
![]() $z\in \mathcal {A}_{\rho },[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d,~H(z,\sigma _{\Omega }(z))\leq H$
,
$z\in \mathcal {A}_{\rho },[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d,~H(z,\sigma _{\Omega }(z))\leq H$
,
![]() $\mbox {dist}(z,\Omega )\geq \delta ,$
where
$\mbox {dist}(z,\Omega )\geq \delta ,$
where
Like [Reference Boxall, Chalebgwa and Jones2], in all our results, we count algebraic points of
![]() $\sigma _{\Omega }(z)$
on some unbounded regions of
$\sigma _{\Omega }(z)$
on some unbounded regions of
![]() $\mathbb {C}.$
Since
$\mathbb {C}.$
Since
![]() $\mathbb {R}$
is unbounded, by a result of Heins [Reference Heins4, page 114], we deduce that there are uncountably many lattices
$\mathbb {R}$
is unbounded, by a result of Heins [Reference Heins4, page 114], we deduce that there are uncountably many lattices
![]() $\Omega $
with
$\Omega $
with
![]() $|\tau |>1.9$
and
$|\tau |>1.9$
and
![]() $\eta _2/\eta _1\in \mathbb {R}\setminus \{0\}.$
However, despite Theorem 1.2, we do not know a single example of a lattice
$\eta _2/\eta _1\in \mathbb {R}\setminus \{0\}.$
However, despite Theorem 1.2, we do not know a single example of a lattice
![]() $\Omega $
with algebraic invariants
$\Omega $
with algebraic invariants
![]() $g_2,g_3$
for which there exists a nonzero algebraic number
$g_2,g_3$
for which there exists a nonzero algebraic number
![]() $\alpha $
such that
$\alpha $
such that
![]() $\sigma _{\Omega }(\alpha )$
is algebraic. It is, in fact, expected that there is no such
$\sigma _{\Omega }(\alpha )$
is algebraic. It is, in fact, expected that there is no such
![]() $\alpha .$
However, the number of algebraic numbers
$\alpha .$
However, the number of algebraic numbers
![]() $\alpha $
with degrees at most d and multiplicative heights at most H is at most
$\alpha $
with degrees at most d and multiplicative heights at most H is at most
![]() $4^d H^d,$
which means that such
$4^d H^d,$
which means that such
![]() $\alpha $
are very rare.
$\alpha $
are very rare.
We end this section with three results which are needed for the proof of our theorems.
Proposition 1.4 [Reference Besson1, Théorème 1.2]
Let
![]() $T\geq 1$
be an integer and
$T\geq 1$
be an integer and
![]() $R\geq 2$
be a real number. Consider any nonzero polynomial
$R\geq 2$
be a real number. Consider any nonzero polynomial
![]() $P(X,Y)\in \mathbb {C}[X,Y]$
of degree at most T in each variable. Then there exists an effective constant
$P(X,Y)\in \mathbb {C}[X,Y]$
of degree at most T in each variable. Then there exists an effective constant
![]() $C_6>0$
such that the function
$C_6>0$
such that the function
![]() $P(z,\sigma _{\Omega }(z))$
has at most
$P(z,\sigma _{\Omega }(z))$
has at most
zeros in the disk
![]() $|z|\leq R.$
$|z|\leq R.$
Proposition 1.5 [Reference Masser6, Proposition 2]
Fix integers
![]() $d\geq 1,T\geq \sqrt {8d}$
and positive real numbers
$d\geq 1,T\geq \sqrt {8d}$
and positive real numbers
![]() $A,Z,M$
and H with
$A,Z,M$
and H with
![]() $H\geq 1.$
Let
$H\geq 1.$
Let
![]() $f_1,f_2$
be two analytic functions on a neighbourhood of the disk
$f_1,f_2$
be two analytic functions on a neighbourhood of the disk
![]() $|z|\leq 2Z.$
Suppose that
$|z|\leq 2Z.$
Suppose that
![]() $|f_1(z)|\leq M,|f_2(z)|\leq M$
for all
$|f_1(z)|\leq M,|f_2(z)|\leq M$
for all
![]() $|z|\leq 2Z.$
Let
$|z|\leq 2Z.$
Let
![]() $\mathcal {Z}\subseteq \mathbb {C}$
be finite and such that for all
$\mathcal {Z}\subseteq \mathbb {C}$
be finite and such that for all
![]() $z,z'\in \mathcal{Z}{:}$
$z,z'\in \mathcal{Z}{:}$
-
(1)
 $|z|\leq Z;$
$|z|\leq Z;$
-
(2)
 $|z-z'|\leq 1/A;$
$|z-z'|\leq 1/A;$
-
(3)
 $[\mathbb {Q}(f_1(z),f_2(z)):\mathbb {Q}]\leq d;$
$[\mathbb {Q}(f_1(z),f_2(z)):\mathbb {Q}]\leq d;$
-
(4)
 $H(f_1(z),f_2(z))\leq H.$
$H(f_1(z),f_2(z))\leq H.$
If
then there exists a nonzero polynomial
![]() $P\in \mathbb {Z}[X,Y]$
of total degree at most T such that
$P\in \mathbb {Z}[X,Y]$
of total degree at most T such that
![]() $P(f_1(z),f_2(z))=0 \ \mbox {for all}~z\in \mathcal {Z}.$
$P(f_1(z),f_2(z))=0 \ \mbox {for all}~z\in \mathcal {Z}.$
Proposition 1.6 [Reference Masser5, Lemma 7.1]
For any Weierstrass
![]() $\sigma $
-function
$\sigma $
-function
![]() $\sigma _{\Omega }(z),$
there exists a constant
$\sigma _{\Omega }(z),$
there exists a constant
![]() $C_7=C_7(\Omega )$
such that for any
$C_7=C_7(\Omega )$
such that for any
![]() $R\geq 1,$
$R\geq 1,$
Our paper is organised as follows. In Section 2, we prove an analogue of [Reference Boxall, Chalebgwa and Jones2, Proposition 2.1] for
![]() $z\in \mathcal {A}_{\rho }.$
Then we prove Theorem 1.1 in Section 3, Theorem 1.2 in Section 4 and Theorem 1.3 in Section 5.
$z\in \mathcal {A}_{\rho }.$
Then we prove Theorem 1.1 in Section 3, Theorem 1.2 in Section 4 and Theorem 1.3 in Section 5.
2 Lower bound
Recall that P is the fundamental domain of the lattice
![]() $\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
enclosed by the parallelogram with vertices
$\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
enclosed by the parallelogram with vertices
![]() $\tfrac 12(\pm \omega _1\pm \omega _2).$
$\tfrac 12(\pm \omega _1\pm \omega _2).$
Proposition 2.1. Let
![]() $\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
be a lattice in
$\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
be a lattice in
![]() $\mathbb {C}$
with
$\mathbb {C}$
with
![]() $\rho = \eta _2/\eta _1$
a nonzero real number. Then there exist positive constants r and C depending only on
$\rho = \eta _2/\eta _1$
a nonzero real number. Then there exist positive constants r and C depending only on
![]() $\Omega $
such that for all
$\Omega $
such that for all
![]() $z\in \mathcal {A}_{\rho }$
with
$z\in \mathcal {A}_{\rho }$
with
![]() $|z|\geq r$
, there exists
$|z|\geq r$
, there exists
![]() $z_0\in P$
with
$z_0\in P$
with
Proof. As in the proof of [Reference Boxall, Chalebgwa and Jones2, Proposition 2.1], we may assume that
![]() $\Omega =\mathbb {Z}+\mathbb {Z}\tau .$
Let
$\Omega =\mathbb {Z}+\mathbb {Z}\tau .$
Let
![]() $z\in \mathcal {A}_{\rho }$
and
$z\in \mathcal {A}_{\rho }$
and
![]() $z_0\in P$
be such that
$z_0\in P$
be such that
![]() $z=z_0+m+n\tau $
for some integers m and
$z=z_0+m+n\tau $
for some integers m and
![]() $n.$
Then
$n.$
Then
(see [Reference Masser7, page 255]). Hence,
where
![]() $R(m,n,z_0)=\operatorname {Re}[(m\eta _1+n\eta _2)(z_0+m/2+(n/2)\tau )].$
Note that
$R(m,n,z_0)=\operatorname {Re}[(m\eta _1+n\eta _2)(z_0+m/2+(n/2)\tau )].$
Note that
Further, from Legendre’s relation
![]() $\eta _1\tau -\eta _2=2\pi i,$
we obtain
$\eta _1\tau -\eta _2=2\pi i,$
we obtain
Moreover,
Therefore,
 $$ \begin{align*} \operatorname{Re}\bigg(\frac{\eta_1}2\bigg)m^2+\operatorname{Re}\bigg(\frac{\eta_1\tau+\eta_2}{2}\bigg)mn+\operatorname{Re}\bigg(\frac{\eta_2\tau}{2}\bigg)n^2&=\operatorname{Re}(\eta_1)\bigg(\frac{m^2} 2+\rho mn+\frac{\rho^2}2 n^2\bigg)\\&=\frac {1} 2\operatorname{Re}(\eta_1)( n\rho + m )^2. \end{align*} $$
$$ \begin{align*} \operatorname{Re}\bigg(\frac{\eta_1}2\bigg)m^2+\operatorname{Re}\bigg(\frac{\eta_1\tau+\eta_2}{2}\bigg)mn+\operatorname{Re}\bigg(\frac{\eta_2\tau}{2}\bigg)n^2&=\operatorname{Re}(\eta_1)\bigg(\frac{m^2} 2+\rho mn+\frac{\rho^2}2 n^2\bigg)\\&=\frac {1} 2\operatorname{Re}(\eta_1)( n\rho + m )^2. \end{align*} $$
Hence,
 $$ \begin{align*} R(m,n,z_0)&=\frac {1} 2\operatorname{Re}(\eta_1)( n\rho + m )^2+\operatorname{Re}(\eta_1z_0)(n\rho+m)\\&=(n\rho+ m )\bigg[\frac{\operatorname{Re}(\eta_1)}{2} (n\rho+ m )+\operatorname{Re}(\eta_1z_0)\bigg]. \end{align*} $$
$$ \begin{align*} R(m,n,z_0)&=\frac {1} 2\operatorname{Re}(\eta_1)( n\rho + m )^2+\operatorname{Re}(\eta_1z_0)(n\rho+m)\\&=(n\rho+ m )\bigg[\frac{\operatorname{Re}(\eta_1)}{2} (n\rho+ m )+\operatorname{Re}(\eta_1z_0)\bigg]. \end{align*} $$
(Recall that by Dirichlet’s theorem, there are infinitely many pairs of integers
![]() $(m,n)$
such that either
$(m,n)$
such that either
![]() $ \rho + m/n=0$
or
$ \rho + m/n=0$
or
![]() $| \rho + m/n|< 1 /{n^2}.$
For this reason, we need to restrict the values of
$| \rho + m/n|< 1 /{n^2}.$
For this reason, we need to restrict the values of
![]() $m,n.$
) Also, since
$m,n.$
) Also, since
![]() $\eta _2/\eta _1=\tau -2\pi i/\eta _1$
and
$\eta _2/\eta _1=\tau -2\pi i/\eta _1$
and
![]() $\eta _2/\eta _1$
is real, we have
$\eta _2/\eta _1$
is real, we have
![]() $\mbox {Im}(\tau -2\pi i/\eta _1)=0.$
So,
$\mbox {Im}(\tau -2\pi i/\eta _1)=0.$
So,
 $$ \begin{align*}\mbox{Im}(\tau)=2\pi\operatorname{Re}\bigg(\frac{1}{\eta_1}\bigg)=2\pi \frac{\operatorname{Re}(\eta_1)}{\operatorname{Re}(\eta_1)^2+\mbox{Im}(\eta_1)^2}.\end{align*} $$
$$ \begin{align*}\mbox{Im}(\tau)=2\pi\operatorname{Re}\bigg(\frac{1}{\eta_1}\bigg)=2\pi \frac{\operatorname{Re}(\eta_1)}{\operatorname{Re}(\eta_1)^2+\mbox{Im}(\eta_1)^2}.\end{align*} $$
Since
![]() $\mbox {Im}(\tau )>0,$
we have
$\mbox {Im}(\tau )>0,$
we have
![]() $\operatorname {Re}(\eta _1)>0.$
$\operatorname {Re}(\eta _1)>0.$
Case 1:
![]() $\rho>0.$
Suppose
$\rho>0.$
Suppose
![]() $ m>0,n>0$
. Then there exists a positive constant
$ m>0,n>0$
. Then there exists a positive constant
![]() $r=r(\Omega )$
such that whenever
$r=r(\Omega )$
such that whenever
![]() $|z|>r,$
we have
$|z|>r,$
we have
for some positive constants
![]() $c_1,c_2$
depending only on
$c_1,c_2$
depending only on
![]() $\Omega .$
However,
$\Omega .$
However,
for some constant
![]() $c_3=c_3(\Omega )>0.$
Hence,
$c_3=c_3(\Omega )>0.$
Hence,
for some constant
![]() $c_4=c_4(\Omega )>0.$
If
$c_4=c_4(\Omega )>0.$
If
![]() $m<0, n<0$
, then consider the point
$m<0, n<0$
, then consider the point
![]() $-z_0-m-n\tau .$
Clearly,
$-z_0-m-n\tau .$
Clearly,
![]() $-z_0\in P.$
Therefore, from (2.1), we obtain
$-z_0\in P.$
Therefore, from (2.1), we obtain
However, since
![]() $\sigma _{\Omega }(z)$
is an odd function,
$\sigma _{\Omega }(z)$
is an odd function,
and the required result follows.
Case 2:
![]() $\rho <0.$
The proof of this case is similar to Case 1 and therefore we omit it.
$\rho <0.$
The proof of this case is similar to Case 1 and therefore we omit it.
3 Proof of Theorem 1.1
Recall that
![]() $\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
is a lattice in
$\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
is a lattice in
![]() $\mathbb {C}$
and
$\mathbb {C}$
and
![]() $\rho = \eta _2/\eta _1$
is a nonzero real number. Throughout this section, let r and C denote the constants from Proposition 2.1. In the following,
$\rho = \eta _2/\eta _1$
is a nonzero real number. Throughout this section, let r and C denote the constants from Proposition 2.1. In the following,
![]() $c_5,\ldots ,c_{17}$
denote positive constants depending only on
$c_5,\ldots ,c_{17}$
denote positive constants depending only on
![]() $\Omega $
(and independent of d and H). Since
$\Omega $
(and independent of d and H). Since
there exists an
![]() $\varepsilon $
with
$\varepsilon $
with
![]() $0<\varepsilon <1/2$
such that
$0<\varepsilon <1/2$
such that
whenever
![]() $|z|<\varepsilon .$
We fix such an
$|z|<\varepsilon .$
We fix such an
![]() $\varepsilon .$
$\varepsilon .$
Lemma 3.1. Let
![]() $z\in \mathcal {A}_{\rho } $
and
$z\in \mathcal {A}_{\rho } $
and
![]() $z_0\in P$
be such that
$z_0\in P$
be such that
![]() $z-z_0\in \Omega $
with
$z-z_0\in \Omega $
with
![]() $|z_0|\geq \varepsilon .$
Assume that
$|z_0|\geq \varepsilon .$
Assume that
![]() $|z|\geq r$
and both z,
$|z|\geq r$
and both z,
![]() $\sigma _{\Omega }(z)$
are algebraic with
$\sigma _{\Omega }(z)$
are algebraic with
![]() $[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
$[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
![]() $H(z,\sigma _{\Omega }(z))\leq H$
for some
$H(z,\sigma _{\Omega }(z))\leq H$
for some
![]() $d\geq e$
and
$d\geq e$
and
![]() $H\geq e.$
Then
$H\geq e.$
Then
![]() $|z|\leq C_8\sqrt {d\log H}$
for some constant
$|z|\leq C_8\sqrt {d\log H}$
for some constant
![]() $C_8=C_8(\Omega )>0.$
$C_8=C_8(\Omega )>0.$
Proof. Let
![]() $S=\{z\in P:|z|<\varepsilon \}.$
Note that
$S=\{z\in P:|z|<\varepsilon \}.$
Note that
![]() $P\setminus S$
is compact. Since
$P\setminus S$
is compact. Since
![]() $\sigma _{\Omega }(z)$
is continuous and nonzero in
$\sigma _{\Omega }(z)$
is continuous and nonzero in
![]() $P\setminus S,$
for all
$P\setminus S,$
for all
![]() $z\in P\setminus S,$
we have
$z\in P\setminus S,$
we have
![]() $|\sigma _{\Omega }(z)|\geq c_5.$
Since
$|\sigma _{\Omega }(z)|\geq c_5.$
Since
![]() $|z_0|\geq \varepsilon ,$
we have
$|z_0|\geq \varepsilon ,$
we have
![]() $|\sigma _{\Omega }(z_0)|\geq c_5.$
Now from Proposition 2.1,
$|\sigma _{\Omega }(z_0)|\geq c_5.$
Now from Proposition 2.1,
However, since
![]() $[\mathbb {Q}(\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
$[\mathbb {Q}(\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
![]() $H(\sigma _{\Omega }(z))\leq H,$
we have
$H(\sigma _{\Omega }(z))\leq H,$
we have
![]() $|\sigma _{\Omega }(z)|\leq H^d.$
So
$|\sigma _{\Omega }(z)|\leq H^d.$
So
and therefore,
This completes the proof of the lemma.
Lemma 3.2. Let
![]() $z\in \mathcal {A}_{\rho } $
and
$z\in \mathcal {A}_{\rho } $
and
![]() $z_0\in P$
be such that
$z_0\in P$
be such that
![]() $z-z_0\in \Omega $
with
$z-z_0\in \Omega $
with
![]() $|z_0|< \varepsilon .$
Assume that
$|z_0|< \varepsilon .$
Assume that
![]() $|z|\geq r$
and both z,
$|z|\geq r$
and both z,
![]() $\sigma _{\Omega }(z)$
are algebraic with
$\sigma _{\Omega }(z)$
are algebraic with
![]() $[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
$[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
![]() $H(z,\sigma _{\Omega }(z))\leq H$
for some
$H(z,\sigma _{\Omega }(z))\leq H$
for some
![]() $d\geq e$
and
$d\geq e$
and
![]() $H\geq e.$
For all
$H\geq e.$
For all
![]() $B>0$
and for all
$B>0$
and for all
![]() $N\geq \sqrt {d\log H},$
if
$N\geq \sqrt {d\log H},$
if
![]() $|z|\geq \sqrt {{(2+B)}/{C}}N,$
then
$|z|\geq \sqrt {{(2+B)}/{C}}N,$
then
![]() $\log |z_0|\leq -BN^2.$
$\log |z_0|\leq -BN^2.$
Proof. Let
![]() $z\in \mathcal {A}_{\rho }$
with
$z\in \mathcal {A}_{\rho }$
with
![]() $|z|\geq r.$
Let
$|z|\geq r.$
Let
![]() $z_0\in P$
be such that
$z_0\in P$
be such that
![]() $z-z_0\in \Omega .$
From Proposition 2.1,
$z-z_0\in \Omega .$
From Proposition 2.1,
Using
![]() $|\sigma _{\Omega }(z)|\leq H^d$
and
$|\sigma _{\Omega }(z)|\leq H^d$
and
![]() $N\geq \sqrt {d\log H},$
we obtain
$N\geq \sqrt {d\log H},$
we obtain
For any
![]() $B>0,$
put
$B>0,$
put
 $$ \begin{align*}A=\sqrt{\frac{2+B}{C}}.\end{align*} $$
$$ \begin{align*}A=\sqrt{\frac{2+B}{C}}.\end{align*} $$
If
![]() $|z|\geq AN,$
then from (3.2), we deduce that
$|z|\geq AN,$
then from (3.2), we deduce that
![]() $CA^2N^2+\log |\sigma _{\Omega }(z_0)|\leq N^2.$
So,
$CA^2N^2+\log |\sigma _{\Omega }(z_0)|\leq N^2.$
So,
![]() $\log |\sigma _{\Omega }(z_0)|\leq (1-CA^2)N^2.$
Since
$\log |\sigma _{\Omega }(z_0)|\leq (1-CA^2)N^2.$
Since
![]() $|z_0|\leq \varepsilon ,$
applying (3.1), we obtain
$|z_0|\leq \varepsilon ,$
applying (3.1), we obtain
Thus, the result follows.
Lemma 3.3. Assume that
![]() $\omega _1$
and
$\omega _1$
and
![]() $\omega _2$
are both algebraic. Let
$\omega _2$
are both algebraic. Let
![]() $z\in \mathcal {A}_{\rho }$
be such that
$z\in \mathcal {A}_{\rho }$
be such that
![]() $|z|\geq r$
and both
$|z|\geq r$
and both
![]() $z,\sigma _{\Omega }(z)$
are algebraic with
$z,\sigma _{\Omega }(z)$
are algebraic with
![]() $[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
$[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
![]() ${H(z,\sigma _{\Omega }(z))\leq H}$
, where
${H(z,\sigma _{\Omega }(z))\leq H}$
, where
![]() $d\geq e$
and
$d\geq e$
and
![]() $H\geq e.$
Then there exists a constant
$H\geq e.$
Then there exists a constant
![]() $C_9=C_9(\Omega )>0$
such that
$C_9=C_9(\Omega )>0$
such that
![]() $|z|\leq C_9d\sqrt {\log H}.$
$|z|\leq C_9d\sqrt {\log H}.$
Proof. Suppose
![]() $z\in \mathcal {A}_{\rho }.$
Choose
$z\in \mathcal {A}_{\rho }.$
Choose
![]() $z_0\in P$
such that
$z_0\in P$
such that
![]() $z-z_0\in \Omega .$
If
$z-z_0\in \Omega .$
If
![]() $|z_0|\geq \varepsilon ,$
then by Lemma 3.1,
$|z_0|\geq \varepsilon ,$
then by Lemma 3.1,
![]() $|z|\leq c_8\sqrt {d\log H}.$
So we assume that
$|z|\leq c_8\sqrt {d\log H}.$
So we assume that
![]() $|z_0|<\varepsilon .$
Let
$|z_0|<\varepsilon .$
Let
![]() $\omega =z-z_0.$
By [Reference Boxall, Chalebgwa and Jones2, Lemma 3.5], if
$\omega =z-z_0.$
By [Reference Boxall, Chalebgwa and Jones2, Lemma 3.5], if
![]() $\omega =k\omega _1+l\omega _2$
, then
$\omega =k\omega _1+l\omega _2$
, then
However, since
![]() $H(z)\leq H$
and
$H(z)\leq H$
and
![]() $[\mathbb {Q}(z):\mathbb {Q}]\leq d,$
we deduce that
$[\mathbb {Q}(z):\mathbb {Q}]\leq d,$
we deduce that
![]() $|z|\leq H^d.$
So
$|z|\leq H^d.$
So
![]() $H(k)=|k|\leq c_{10}|z|\leq c_{10}H^d$
and similarly
$H(k)=|k|\leq c_{10}|z|\leq c_{10}H^d$
and similarly
![]() $H(l)\leq c_{10}H^d.$
Now, using the inequality
$H(l)\leq c_{10}H^d.$
Now, using the inequality
together with the bounds
and
we deduce that
 $$ \begin{align*} \log |z_0|\geq \log(1/M(z_0))&= -[\mathbb{Q}(z_0):\mathbb{Q}]\log (H(z_0))\\&\geq -c_{12}d((2d+1)\log H+\log c_{11})\geq -c_{13}d^2\log H, \end{align*} $$
$$ \begin{align*} \log |z_0|\geq \log(1/M(z_0))&= -[\mathbb{Q}(z_0):\mathbb{Q}]\log (H(z_0))\\&\geq -c_{12}d((2d+1)\log H+\log c_{11})\geq -c_{13}d^2\log H, \end{align*} $$
where
![]() $M(\alpha )$
is the Mahler measure of
$M(\alpha )$
is the Mahler measure of
![]() $\alpha .$
Applying Lemma 3.2 with
$\alpha .$
Applying Lemma 3.2 with
![]() $B=c_{13}$
and
$B=c_{13}$
and
![]() $N=d\sqrt {\log H},$
we deduce that
$N=d\sqrt {\log H},$
we deduce that
![]() $|z|\leq c_{14}d\sqrt {\log H},$
where
$|z|\leq c_{14}d\sqrt {\log H},$
where
![]() $c_{14}=\sqrt {{(2+c_{13})}/{C}}.$
Taking
$c_{14}=\sqrt {{(2+c_{13})}/{C}}.$
Taking
![]() $C_9=\max (c_8, c_{14}),$
we obtain the required result.
$C_9=\max (c_8, c_{14}),$
we obtain the required result.
Proof of Theorem 1.1
Define
Put
From Lemma 3.3,
![]() $|z|\leq C_9d\sqrt {\log H}\leq Z$
and
$|z|\leq C_9d\sqrt {\log H}\leq Z$
and
![]() $|z-z'|\leq 1/A$
for all
$|z-z'|\leq 1/A$
for all
![]() $z,z'\in \mathcal {Z}_1.$
However, from Proposition 1.6, there exists a constant
$z,z'\in \mathcal {Z}_1.$
However, from Proposition 1.6, there exists a constant
![]() $c_{15}\geq 1$
such that for all
$c_{15}\geq 1$
such that for all
![]() $z\in \mathcal {A}_{\rho },$
$z\in \mathcal {A}_{\rho },$
Put
![]() $M=c_{15}^{Z^2}.$
Then,
$M=c_{15}^{Z^2}.$
Then,
![]() $|z|\leq M$
and
$|z|\leq M$
and
![]() $|\sigma _{\Omega }(z)|\leq M$
for all
$|\sigma _{\Omega }(z)|\leq M$
for all
![]() $|z|\leq 2Z.$
With these choices of
$|z|\leq 2Z.$
With these choices of
![]() $A,Z$
and
$A,Z$
and
![]() $M,$
the conditions of Proposition 1.5 are satisfied. If we take
$M,$
the conditions of Proposition 1.5 are satisfied. If we take
![]() $T=c_{16}d^3\log H$
for a sufficiently large
$T=c_{16}d^3\log H$
for a sufficiently large
![]() $c_{16}>0,$
then (1.2) is satisfied. Hence by Proposition 1.5, there exists a nonzero polynomial
$c_{16}>0,$
then (1.2) is satisfied. Hence by Proposition 1.5, there exists a nonzero polynomial
![]() $P\in \mathbb {Z}[X,Y]$
of total degree at most T such that
$P\in \mathbb {Z}[X,Y]$
of total degree at most T such that
![]() $P(z,\sigma _{\Omega }(z))=0$
for all
$P(z,\sigma _{\Omega }(z))=0$
for all
![]() $z\in \mathcal {Z}_1.$
Finally taking
$z\in \mathcal {Z}_1.$
Finally taking
![]() $R=C_9d\sqrt {\log H}$
and
$R=C_9d\sqrt {\log H}$
and
![]() $T=c_{16}d^3\log H$
in Proposition 1.4, we deduce that there are at most
$T=c_{16}d^3\log H$
in Proposition 1.4, we deduce that there are at most
zeros of
![]() $P(z,\sigma _{\Omega }(z))$
in the region
$P(z,\sigma _{\Omega }(z))$
in the region
![]() $|z|\leq R.$
Hence, the number of elements in the set
$|z|\leq R.$
Hence, the number of elements in the set
![]() $\mathcal {Z}_1$
is at most
$\mathcal {Z}_1$
is at most
![]() $c_{17}d^6(\log d)(\log H)^2\log \log H.$
This completes the proof.
$c_{17}d^6(\log d)(\log H)^2\log \log H.$
This completes the proof.
4 Proof of Theorem 1.2
Throughout this section, let
![]() $\Omega $
denote a lattice in
$\Omega $
denote a lattice in
![]() $\mathbb {C}$
with algebraic invariants
$\mathbb {C}$
with algebraic invariants
![]() $g_2,g_3.$
In this section,
$g_2,g_3.$
In this section,
![]() $c_{18},\ldots ,c_{27}$
denote various constants which depend only on
$c_{18},\ldots ,c_{27}$
denote various constants which depend only on
![]() $\Omega .$
We first state the following transcendence measure for the nonzero elements of
$\Omega .$
We first state the following transcendence measure for the nonzero elements of
![]() $\Omega $
, due to David and Hirata-Kohno.
$\Omega $
, due to David and Hirata-Kohno.
Lemma 4.1 [Reference David and Hirata-Kohno3, Theorem 1.6]
Let
![]() $\Omega $
be a lattice in
$\Omega $
be a lattice in
![]() $\mathbb {C}.$
Let
$\mathbb {C}.$
Let
![]() $d\geq 1$
and
$d\geq 1$
and
![]() $H\geq 3$
be real numbers. Let
$H\geq 3$
be real numbers. Let
![]() $\alpha $
be an algebraic number with
$\alpha $
be an algebraic number with
![]() $[\mathbb {Q}(\alpha ):\mathbb {Q}]\leq d$
and
$[\mathbb {Q}(\alpha ):\mathbb {Q}]\leq d$
and
![]() $H(\alpha )\leq H.$
Then there exists a constant
$H(\alpha )\leq H.$
Then there exists a constant
![]() $C_{10}=C_{10}(\Omega )>0$
such that
$C_{10}=C_{10}(\Omega )>0$
such that
for all
![]() $\omega \in \Omega \setminus \{0\}.$
$\omega \in \Omega \setminus \{0\}.$
The following is an analogue of [Reference Boxall, Chalebgwa and Jones2, Proposition 4.2].
Lemma 4.2. Assume that
![]() $\rho =\eta _2/\eta _1$
is a nonzero real number. Let
$\rho =\eta _2/\eta _1$
is a nonzero real number. Let
![]() $d\geq 1, H\geq 3$
be real numbers. There exist positive constants
$d\geq 1, H\geq 3$
be real numbers. There exist positive constants
![]() $C_{11},C_{12}$
depending only on
$C_{11},C_{12}$
depending only on
![]() $\Omega $
such that the following holds. If
$\Omega $
such that the following holds. If
![]() $z,z'\in \mathcal {A}_{\rho }$
with
$z,z'\in \mathcal {A}_{\rho }$
with
![]() $[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d, [\mathbb {Q}(z',\sigma _{\Omega }(z')):\mathbb {Q}]\leq d$
,
$[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d, [\mathbb {Q}(z',\sigma _{\Omega }(z')):\mathbb {Q}]\leq d$
,
![]() $H(z,\sigma _{\Omega }(z))\leq H$
and
$H(z,\sigma _{\Omega }(z))\leq H$
and
![]() $H(z',\sigma _{\Omega }(z'))\leq H,$
then
$H(z',\sigma _{\Omega }(z'))\leq H,$
then
or there exists
![]() $\omega ,\omega '\in \Omega $
such that
$\omega ,\omega '\in \Omega $
such that
with
![]() $z'/z=\omega '/\omega \in \mathbb {Q}.$
$z'/z=\omega '/\omega \in \mathbb {Q}.$
Proof. The proof follows the same line of argument as in [Reference Boxall, Chalebgwa and Jones2, Proposition 4.2], so we omit it here.
Lemma 4.3. Assume that
![]() $\rho =\eta _2/\eta _1$
is a nonzero real number and
$\rho =\eta _2/\eta _1$
is a nonzero real number and
![]() $g_2,g_3$
are both algebraic. Let
$g_2,g_3$
are both algebraic. Let
![]() $d\geq 1, H\geq 3$
be real numbers. Let r be from Proposition 2.1 and
$d\geq 1, H\geq 3$
be real numbers. Let r be from Proposition 2.1 and
![]() $C_{11}$
be from Lemma 4.2. Consider the set
$C_{11}$
be from Lemma 4.2. Consider the set
 $$ \begin{align*} S=\bigg\{z\in \mathcal{A}_{\rho}: &\, [\mathbb{Q}(z,\sigma_{\Omega}(z)): \mathbb{Q}]\leq d, H(z,\sigma_{\Omega}(z))\leq H,\\ &\text{and}\ |z|>\max\bigg\{r,C_{11}\sqrt{d^9(\log d)^2\log H}\bigg\}\bigg\}. \end{align*} $$
$$ \begin{align*} S=\bigg\{z\in \mathcal{A}_{\rho}: &\, [\mathbb{Q}(z,\sigma_{\Omega}(z)): \mathbb{Q}]\leq d, H(z,\sigma_{\Omega}(z))\leq H,\\ &\text{and}\ |z|>\max\bigg\{r,C_{11}\sqrt{d^9(\log d)^2\log H}\bigg\}\bigg\}. \end{align*} $$
Then there exists a positive constant
![]() $C_{13}=C_{13}(\Omega )$
such that the number of elements of S is at most
$C_{13}=C_{13}(\Omega )$
such that the number of elements of S is at most
Proof. We follow the strategy given in [Reference Boxall, Chalebgwa and Jones2]. Suppose
![]() $z,z'\in S.$
Then,
$z,z'\in S.$
Then,
So by Lemma 4.2, there exist
![]() $\omega ,\omega '\in \Omega $
such that
$\omega ,\omega '\in \Omega $
such that
with
![]() $z'/z=\omega '/\omega \in \mathbb {Q}.$
This implies
$z'/z=\omega '/\omega \in \mathbb {Q}.$
This implies
![]() $z, z'$
are not periods of
$z, z'$
are not periods of
![]() $\Omega .$
$\Omega .$
Put
![]() $\omega '/\omega =q.$
Let
$\omega '/\omega =q.$
Let
![]() $\omega ^*\in \Omega \setminus \{0\}$
be of minimum modulus on the line joining
$\omega ^*\in \Omega \setminus \{0\}$
be of minimum modulus on the line joining
![]() $0$
and
$0$
and
![]() $\omega .$
Then
$\omega .$
Then
![]() $\omega =m\omega ^*$
for some nonzero integer
$\omega =m\omega ^*$
for some nonzero integer
![]() $m.$
Let
$m.$
Let
![]() $z^*=z/m.$
Note that
$z^*=z/m.$
Note that
![]() $z^*\in \mathcal {A}_{\rho }.$
Also, since
$z^*\in \mathcal {A}_{\rho }.$
Also, since
![]() $\omega '$
and
$\omega '$
and
![]() $\omega $
lie on the same line,
$\omega $
lie on the same line,
![]() $\omega '=m_1\omega ^*$
for some
$\omega '=m_1\omega ^*$
for some
![]() $m_1\in \mathbb {Z}.$
So
$m_1\in \mathbb {Z}.$
So
![]() $qm\omega ^*=m_1\omega ^*.$
Hence,
$qm\omega ^*=m_1\omega ^*.$
Hence,
![]() $qm=m_1.$
Now,
$qm=m_1.$
Now,
![]() $z'=qz=qmz^*=m_1z^*.$
So
$z'=qz=qmz^*=m_1z^*.$
So
![]() $z'$
is an integer multiple of
$z'$
is an integer multiple of
![]() $z^*.$
Thus, if we show that
$z^*.$
Thus, if we show that
whenever
![]() $nz^*\in S$
for some
$nz^*\in S$
for some
![]() $n\in \mathbb {Z}$
, we are done. Indeed, just now we have seen that if
$n\in \mathbb {Z}$
, we are done. Indeed, just now we have seen that if
![]() $z'\in S$
, then
$z'\in S$
, then
![]() $z'=nz^*$
for some
$z'=nz^*$
for some
![]() $n\in \mathbb {N}.$
Accordingly, we assume
$n\in \mathbb {N}.$
Accordingly, we assume
![]() $nz^*\in S$
for some
$nz^*\in S$
for some
![]() $n\in \mathbb {Z}.$
Put
$n\in \mathbb {Z}.$
Put
![]() $nz^*=z".$
Thus,
$nz^*=z".$
Thus,
![]() $z"\in \mathcal {A}_{\rho }.$
Let
$z"\in \mathcal {A}_{\rho }.$
Let
![]() $z_0\in P$
be such that
$z_0\in P$
be such that
![]() $z"-z_0=\omega"\in \Omega .$
Since both
$z"-z_0=\omega"\in \Omega .$
Since both
![]() $z"$
and z belong to
$z"$
and z belong to
![]() $S,$
we have
$S,$
we have
![]() $z"/z=\omega "/\omega .$
Hence,
$z"/z=\omega "/\omega .$
Hence,
![]() $nz^*/mz^*=\omega "/m\omega ^*,$
or equivalently
$nz^*/mz^*=\omega "/m\omega ^*,$
or equivalently
![]() $\omega "=n\omega ^*.$
Since
$\omega "=n\omega ^*.$
Since
![]() $z"\in \mathcal {A}_{\rho },$
from Proposition 2.1, we obtain
$z"\in \mathcal {A}_{\rho },$
from Proposition 2.1, we obtain
Note that
![]() $z_0\neq 0.$
Therefore,
$z_0\neq 0.$
Therefore,
![]() $|\sigma _{\Omega }(z_0)/z_0|>e^{c_{18}}.$
Hence,
$|\sigma _{\Omega }(z_0)/z_0|>e^{c_{18}}.$
Hence,
 $$ \begin{align*} \log |\sigma_{\Omega}(z")| & \geq \log |z_0|+c_{19}+C|z"|^2\\ & = \log |z"-\omega"|+c_{19}+C|z"|^2\\ & \geq \log |nz^*-n\omega^*|+c_{19}+Cn^2|z^*|^2\\ & \geq \log |z^*-\omega^*|+c_{19}+Cn^2|z^*|^2. \end{align*} $$
$$ \begin{align*} \log |\sigma_{\Omega}(z")| & \geq \log |z_0|+c_{19}+C|z"|^2\\ & = \log |z"-\omega"|+c_{19}+C|z"|^2\\ & \geq \log |nz^*-n\omega^*|+c_{19}+Cn^2|z^*|^2\\ & \geq \log |z^*-\omega^*|+c_{19}+Cn^2|z^*|^2. \end{align*} $$
Write
![]() $\omega =k\omega _1+l\omega _2$
with integers
$\omega =k\omega _1+l\omega _2$
with integers
![]() $k,l.$
As we have seen earlier in the proof of Lemma 3.3,
$k,l.$
As we have seen earlier in the proof of Lemma 3.3,
![]() $\max (|k|,|l|)\leq c_{20}|z|\leq c_{20}H^d.$
Further, since
$\max (|k|,|l|)\leq c_{20}|z|\leq c_{20}H^d.$
Further, since
![]() $\omega =m\omega ^*,$
we see that m divides both
$\omega =m\omega ^*,$
we see that m divides both
![]() $k,l.$
We deduce that
$k,l.$
We deduce that
![]() $|m|\leq c_{20}H^d.$
So,
$|m|\leq c_{20}H^d.$
So,
Since
![]() $z^*=z/m$
is algebraic, by Lemma 4.1, we deduce that
$z^*=z/m$
is algebraic, by Lemma 4.1, we deduce that
 $$ \begin{align*} \log |z^*-\omega^*|\geq &-c_{22}d^4(\log d)^2d(\log H)|\omega^*|^2(1+\max\{0,\log |\omega^*|\})^3\\ \geq &-c_{23}d^5(\log d)^2(\log H)|z^*|^2(1+\max\{0,\log |z^*|\})^3. \end{align*} $$
$$ \begin{align*} \log |z^*-\omega^*|\geq &-c_{22}d^4(\log d)^2d(\log H)|\omega^*|^2(1+\max\{0,\log |\omega^*|\})^3\\ \geq &-c_{23}d^5(\log d)^2(\log H)|z^*|^2(1+\max\{0,\log |z^*|\})^3. \end{align*} $$
So,
 $$ \begin{align*} -c_{23}d^5(\log d)^2(\log H)|z^*|^2(1+\max\{0,\log |z^*|\})^3+c_{19}+Cn^2|z^*|^2 & \leq \log |\sigma_{\Omega}(z")| \\ & \leq d\log H. \end{align*} $$
$$ \begin{align*} -c_{23}d^5(\log d)^2(\log H)|z^*|^2(1+\max\{0,\log |z^*|\})^3+c_{19}+Cn^2|z^*|^2 & \leq \log |\sigma_{\Omega}(z")| \\ & \leq d\log H. \end{align*} $$
In other words,
This completes the proof of the lemma.
Proof of Theorem 1.2
To prove Theorem 1.2, by Lemma 4.3, we only need to count the number of elements in the set
 $$ \begin{align*} \mathcal{Z}_2= \bigg\{z\in \mathcal{A}_{\rho}: &\, [\mathbb{Q}(z,\sigma_{\Omega}(z)):\mathbb{Q}]\leq d,~H(z,\sigma_{\Omega}(z))\leq H, \\ & \text{and}~ |z|\leq C_{11}\sqrt{d^9(\log d)^2\log H}\bigg\}. \end{align*} $$
$$ \begin{align*} \mathcal{Z}_2= \bigg\{z\in \mathcal{A}_{\rho}: &\, [\mathbb{Q}(z,\sigma_{\Omega}(z)):\mathbb{Q}]\leq d,~H(z,\sigma_{\Omega}(z))\leq H, \\ & \text{and}~ |z|\leq C_{11}\sqrt{d^9(\log d)^2\log H}\bigg\}. \end{align*} $$
Put
Then
![]() $|z|\leq Z$
and
$|z|\leq Z$
and
![]() $|z-z'|\leq 1/A$
for all
$|z-z'|\leq 1/A$
for all
![]() $z,z'\in \mathcal {Z}_2.$
By Proposition 1.6, for all
$z,z'\in \mathcal {Z}_2.$
By Proposition 1.6, for all
![]() $z\in \mathcal {A}_{\rho },$
$z\in \mathcal {A}_{\rho },$
Put
![]() $M=c_{25}^{Z^2}.$
Then,
$M=c_{25}^{Z^2}.$
Then,
![]() $|z|\leq M$
and
$|z|\leq M$
and
![]() $|\sigma _{\Omega }(z)|\leq M$
for all
$|\sigma _{\Omega }(z)|\leq M$
for all
![]() $|z|\leq 2Z.$
With these choices of
$|z|\leq 2Z.$
With these choices of
![]() $A,Z$
and
$A,Z$
and
![]() $M,$
the conditions of Proposition 1.5 are satisfied. If we take
$M,$
the conditions of Proposition 1.5 are satisfied. If we take
![]() $T=c_{26}d^{10}(\log d)^2\log H$
for a sufficiently large
$T=c_{26}d^{10}(\log d)^2\log H$
for a sufficiently large
![]() $c_{26}>0,$
then (1.2) is satisfied. Thus, by Proposition 1.5, we deduce that there exists a nonzero polynomial
$c_{26}>0,$
then (1.2) is satisfied. Thus, by Proposition 1.5, we deduce that there exists a nonzero polynomial
![]() $P\in \mathbb {Z}[X,Y]$
of total degree at most T such that
$P\in \mathbb {Z}[X,Y]$
of total degree at most T such that
![]() $P(z,\sigma _{\Omega }(z))=0~\mbox {for all}~z\in \mathcal {Z}_2.$
$P(z,\sigma _{\Omega }(z))=0~\mbox {for all}~z\in \mathcal {Z}_2.$
Finally taking
![]() $R\ {=}\ C_{11}\sqrt {d^9(\log d)^2\log H}$
and
$R\ {=}\ C_{11}\sqrt {d^9(\log d)^2\log H}$
and
![]() $T\ {=}\ c_{26}d^{10}(\log d)^2\log H$
in Proposition 1.4, we deduce that there are at most
$T\ {=}\ c_{26}d^{10}(\log d)^2\log H$
in Proposition 1.4, we deduce that there are at most
zeros of
![]() $P(z,\sigma _{\Omega }(z))$
in the region
$P(z,\sigma _{\Omega }(z))$
in the region
![]() $|z|\leq R.$
Hence, the number of elements in the set
$|z|\leq R.$
Hence, the number of elements in the set
![]() $\mathcal {Z}_2$
is at most
$\mathcal {Z}_2$
is at most
![]() $c_{27}d^{20}(\log d)^5(\log H)^2\log \log H.$
Since
$c_{27}d^{20}(\log d)^5(\log H)^2\log \log H.$
Since
from Lemma 4.3, there are at most
algebraic numbers z such that
![]() $z\in \mathcal {A}_{\rho }$
,
$z\in \mathcal {A}_{\rho }$
,
![]() $[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
$[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
![]() $H(z,\sigma _{\Omega }(z))\leq H.$
This completes the proof of the theorem.
$H(z,\sigma _{\Omega }(z))\leq H.$
This completes the proof of the theorem.
5 Proof of Theorem 1.3
Throughout this section, let
![]() $\delta ,r$
denote the constants from the statements of Theorem 1.3 and Proposition 2.1.
$\delta ,r$
denote the constants from the statements of Theorem 1.3 and Proposition 2.1.
Lemma 5.1. Let
![]() $\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
be a lattice in
$\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$
be a lattice in
![]() $\mathbb {C}$
with
$\mathbb {C}$
with
![]() $\rho = \eta _2/\eta _1$
a nonzero real number. Let
$\rho = \eta _2/\eta _1$
a nonzero real number. Let
![]() $z\in \mathcal {A}_{\rho } $
be such that
$z\in \mathcal {A}_{\rho } $
be such that
![]() $|z|\geq r$
and
$|z|\geq r$
and
![]() $\mbox {dist}(z,\Omega )\geq \delta .$
For any
$\mbox {dist}(z,\Omega )\geq \delta .$
For any
![]() $d\geq e$
and
$d\geq e$
and
![]() $H\geq e,$
if both z and
$H\geq e,$
if both z and
![]() $\sigma _{\Omega }(z)$
are algebraic with
$\sigma _{\Omega }(z)$
are algebraic with
![]() $[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
$[\mathbb {Q}(z,\sigma _{\Omega }(z)):\mathbb {Q}]\leq d$
and
![]() $H(z,\sigma _{\Omega }(z))\leq H,$
then there exists a constant
$H(z,\sigma _{\Omega }(z))\leq H,$
then there exists a constant
![]() $C_{14}=C_{14}(\delta ,\Omega )$
such that
$C_{14}=C_{14}(\delta ,\Omega )$
such that
![]() $|z|\leq C_{14}\sqrt {d\log H}.$
$|z|\leq C_{14}\sqrt {d\log H}.$
Proof. Let
![]() $z\in \mathcal {A}_{\rho }$
and
$z\in \mathcal {A}_{\rho }$
and
![]() $z_0\in P$
be such that
$z_0\in P$
be such that
![]() $z-z_0=m_1\omega _1+n_1\omega _2\in \Omega .$
Since
$z-z_0=m_1\omega _1+n_1\omega _2\in \Omega .$
Since
![]() $\mbox {dist}(z,\Omega )\geq \delta ,$
we have
$\mbox {dist}(z,\Omega )\geq \delta ,$
we have
![]() $|z_0|=|z-m_1\omega _1-n_1\omega _2|\geq \delta .$
Let
$|z_0|=|z-m_1\omega _1-n_1\omega _2|\geq \delta .$
Let
![]() $\varepsilon $
denote the constant from Lemma 3.1.
$\varepsilon $
denote the constant from Lemma 3.1.
Case 1:
![]() $\delta \geq \varepsilon .$
Since
$\delta \geq \varepsilon .$
Since
![]() $|z_0|\geq \delta ,$
we have
$|z_0|\geq \delta ,$
we have
![]() $|z_0|\geq \varepsilon .$
So by Lemma 3.1, there exists a constant
$|z_0|\geq \varepsilon .$
So by Lemma 3.1, there exists a constant
![]() $c_{28}=c_{28}(\Omega )$
such that
$c_{28}=c_{28}(\Omega )$
such that
![]() $|z|\leq c_{28}\sqrt {d\log H}.$
Hence, the lemma is proved.
$|z|\leq c_{28}\sqrt {d\log H}.$
Hence, the lemma is proved.
Case 2:
![]() $\delta < \varepsilon .$
Suppose
$\delta < \varepsilon .$
Suppose
![]() $\delta \leq \mbox {dist}(z,\Omega )<\varepsilon $
, so
$\delta \leq \mbox {dist}(z,\Omega )<\varepsilon $
, so
![]() $\delta \leq |z_0|<\varepsilon .$
Hence,
$\delta \leq |z_0|<\varepsilon .$
Hence,
where
![]() $B=\log 1/\delta $
and
$B=\log 1/\delta $
and
![]() $N=\sqrt {d\log H}.$
Since
$N=\sqrt {d\log H}.$
Since
![]() $|z_0|<\varepsilon ,$
applying Lemma 3.2, we obtain
$|z_0|<\varepsilon ,$
applying Lemma 3.2, we obtain
 $$ \begin{align*}|z|\leq\sqrt{\frac{2+\log 1/\delta}{C}}\sqrt{d\log H},\end{align*} $$
$$ \begin{align*}|z|\leq\sqrt{\frac{2+\log 1/\delta}{C}}\sqrt{d\log H},\end{align*} $$
where C is as in Lemma 3.2.
Now suppose
![]() $\mbox {dist}(z,\Omega )\geq \varepsilon $
, so
$\mbox {dist}(z,\Omega )\geq \varepsilon $
, so
![]() $|z_0|\geq \varepsilon .$
As in Case 1,
$|z_0|\geq \varepsilon .$
As in Case 1,
![]() $|z|\leq c_{28}\sqrt {d\log H}.$
Taking
$|z|\leq c_{28}\sqrt {d\log H}.$
Taking
![]() $C_{14}=\max\kern-1.5pt\Big(c_{28}, \sqrt {{(2+\log 1/\delta )}/{C}}\Big),$
we get the required result.
$C_{14}=\max\kern-1.5pt\Big(c_{28}, \sqrt {{(2+\log 1/\delta )}/{C}}\Big),$
we get the required result.
Proof of Theorem 1.3
Define
Put
From Lemma 5.1,
![]() $|z|\leq C_{14}\sqrt {d\log H}\leq Z$
and
$|z|\leq C_{14}\sqrt {d\log H}\leq Z$
and
![]() $|z-z'|\leq 1/A$
for all
$|z-z'|\leq 1/A$
for all
![]() $z,z'\in \mathcal {Z}_3.$
By Proposition 1.6,
$z,z'\in \mathcal {Z}_3.$
By Proposition 1.6,
Put
![]() $M=c_{29}^{Z^2}.$
Then,
$M=c_{29}^{Z^2}.$
Then,
![]() $|z|\leq M$
and
$|z|\leq M$
and
![]() $|\sigma _{\Omega }(z)|\leq M$
for all
$|\sigma _{\Omega }(z)|\leq M$
for all
![]() $|z|\leq 2Z.$
With these choices of
$|z|\leq 2Z.$
With these choices of
![]() $A,Z$
and
$A,Z$
and
![]() $M,$
the conditions of Proposition 1.5 are satisfied. If we take
$M,$
the conditions of Proposition 1.5 are satisfied. If we take
![]() $T=c_{30}d^2\log H$
for some sufficiently large constant
$T=c_{30}d^2\log H$
for some sufficiently large constant
![]() $c_{30}=c_{30}(\delta ,\Omega ),$
then (1.2) is satisfied. Thus, by applying Proposition 1.5, there exists a nonzero polynomial
$c_{30}=c_{30}(\delta ,\Omega ),$
then (1.2) is satisfied. Thus, by applying Proposition 1.5, there exists a nonzero polynomial
![]() $P\in \mathbb {Z}[X,Y]$
of total degree at most T such that
$P\in \mathbb {Z}[X,Y]$
of total degree at most T such that
![]() $P(z,\sigma _{\Omega }(z))=0~\mbox {for all}~z\in \mathcal {Z}_3.$
$P(z,\sigma _{\Omega }(z))=0~\mbox {for all}~z\in \mathcal {Z}_3.$
Finally taking
![]() $R=C_{14}\sqrt {d\log H}$
and
$R=C_{14}\sqrt {d\log H}$
and
![]() $T=c_{30}d^2\log H$
in Proposition 1.4, we deduce that there are at most
$T=c_{30}d^2\log H$
in Proposition 1.4, we deduce that there are at most
zeros of
![]() $P(z,\sigma _{\Omega }(z))$
in the region
$P(z,\sigma _{\Omega }(z))$
in the region
![]() $|z|\leq R$
for some constant
$|z|\leq R$
for some constant
![]() $c_{31}=c_{31}(\delta ,\Omega )>0.$
Hence, the number of elements in the set
$c_{31}=c_{31}(\delta ,\Omega )>0.$
Hence, the number of elements in the set
![]() $\mathcal {Z}_3$
is at most
$\mathcal {Z}_3$
is at most
![]() $c_{31}d^4(\log d)(\log H)^2\log \log H.$
This completes the proof of the theorem.
$c_{31}d^4(\log d)(\log H)^2\log \log H.$
This completes the proof of the theorem.