Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-27T15:18:47.058Z Has data issue: false hasContentIssue false
  • English
  • Français

A deformation of the Alexander polynomials of knots yielding lens spaces

Published online by Cambridge University Press:  17 April 2009

Teruhisa Kadokami  and
Yuichi Yamada
Teruhisa Kadokami
Affiliation:
Department of Mathematics, Osaka City University, Sugimoto3–3–138, Sumiyoshi-ku, Osaka 558–8585, Japan, e-mail: kadokami@sci.osaka-cu.ac.jp
Yuichi Yamada
Affiliation:
Department of Systems Engineering, The University of Electro-Communications, 1–5–1 Chofu-gaoka, Chofu, tokyo 182–8585, Japan, e-mail: yyyamada@sugaku.e-one.uec.ac.jp
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

For a knot K in a homology 3-sphere Σ, by Σ(K;p/q), we denote the resulting 3-manifold of p/q-surgery along K. We say that the manifold or the surgery is of lens type if Σ(K;p/q) has the same Reidemeister torsion as a lens space.

We prove that, for Σ(K;p/q) to be of lens type, it is a necessary and sufficient condition that the Alexander polynomial ΔK(t) of K is equal to that of an (i, j)-torus knot T(i, j) modulo (tp – 1).

We also deduce two results: If Σ(K;p/q) has the same Reidemeister torsion as L(p, q') then (1) 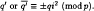(2) The multiple of ΣK(tk) over k ∈ (i) is ±tm modulo (tp – 1), where (i) is the subgroup in (Z/pZ)×/{±1} generated by i. Conversely, if a subgroup H of (Z/pZ)×/{±l} satisfying that the product of ΣK(tk) (k ∈ H) is ±tm modulo (tp – 1), then H includes i or j.

Here, i, j are the parameters of the torus knot above.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 75 , Issue 1 , February 2007 , pp. 75 - 89
Copyright
Copyright © Australian Mathematical Society 2007

References

[1]Baker, K., Knots on once-punctured torus fibers, Dissertation (The university of Texas Austin, 2004).Google Scholar
[2]Baker, K., ‘Surgery descriptions and volumes of Berge knots I: Large volume Berge knots’, arXiv:math.GT/0509054.Google Scholar
[3]Berge, J., ‘Some knots with surgeries yielding lens spaces’, (Unpublished manuscript, 1990).Google Scholar
[4]Berge, J., ‘The knots in D2 × S1 which have nontrivial Dehn surgeries that yield D2 × Sl’, Topology Appl. 38 (1991), 1–19.CrossRefGoogle Scholar
[5]Fintushel, R. and Stern, R.J., ‘Constructing lens spaces by surgery on knots’, Math. Z. 175 (1980), 33–51.CrossRefGoogle Scholar
[6]Franz, W., ‘Über die Torsion einer Überdeckung’, J. Reine Angew. Math. 173 (1935), 245–254.CrossRefGoogle Scholar
[7]Acuña, F. González and Short, H., ‘Cyclic branched coverings of knots and homology spheres’, Rev. Mat. Univ. Complut. Madrid 4 (1991), 97–120.Google Scholar
[8]Hironaka, E., ‘The Lehmer polynomial and pretzel links’, Canad. Math. Bull. 44 (2001), 440–451.CrossRefGoogle Scholar
[9]Ichihara, K., Saito, T. and Teragaito, M., ‘Alexander polynomials of doubly primitive knots’, Proc. of Amer. Math. Soc. 135 (2007), 605–615.CrossRefGoogle Scholar
[10]Kadokami, T., Seifert complex for links and 2-variable Alexander matrices, (Suzuki, Shin'ichi, Editor), Proceedings of Knots'96 (World Sci. Publising, River Edge, 1997), pp. 395–409.Google Scholar
[11]Kadokami, T., On 2-component links with trivial components, Proceedings of Applied Mathematics Workshop 8, The 5th Korea-Japan School of Knots and Links (Center for Applied Mathematics, KAIS, Taejon, Korea, 1997), pp. 71–93.Google Scholar
[12]Kadokami, T., ‘Reidemeister torsion and lens surgeries on knots in homology 3-spheres I’, Osaka J. Math 43 (2006), 1–15.Google Scholar
[13]Kadokami, T., ‘Reidemeister torsion and lens surgeries on knots in homology 3-spheres II', (preprint (2005)).Google Scholar
[14]Kadokami, T. and Yamada, Y., ‘Reidemeister torsion and lens surgeries on (-2,m, n)-pretzel knots’, Kobe J. Math. 23 (2006), 65–78.Google Scholar
[15]Kronheimer, P.B., Mrowka, T.S.Ozsváth, P. and Szabó, Z., ‘Monopoles and lens space surgeries’, arXiv:math.GT/0310164.Google Scholar
[16]Lehmer, D.H., ‘Factorization of certain cyclotomic functions’, Ann. of Math. (2) 34 (1933), 461–479.CrossRefGoogle Scholar
[17]Maruyama, N., ‘On Dehn surgery along a certain family of knots’, J. Tsuda College 19 (1987), 261–280.Google Scholar
[18]Milnor, J., ‘A duality theorem for Reidemeister torsion’, Ann. of Math. (2) 76 (1962), 137–147.CrossRefGoogle Scholar
[19]Moser, L., ‘Elementary surgery along a torus knot’, Pacific J. Math. 38 (1971), 737–745.CrossRefGoogle Scholar
[20]Noguchi, A., ‘Zeros of the Alexander polynomial of knot’, (preprint (2003)).Google Scholar
[21]Ozsváth, P. and Szabó, Z., ‘Absolutely graded Floer homologies and intersection forms for four-manifolds with boundary’, Adv. Math. 173 (2003), 179–261.CrossRefGoogle Scholar
[22]Ozsváth, P. and Szabó, Z., ‘On knot Floer homology and lens space surgeries’, Topology 44 (2005), 1281–1300.CrossRefGoogle Scholar
[23]Riley, R., ‘Growth of order of homology of cyclic branched covers of knots’, Bull. London Math. Soc. 22 (1990), 287–297.CrossRefGoogle Scholar
[24]Saito, T., ‘Dehn surgery and (l, l)-knots in lens space’, Topology Appl. (to appear).Google Scholar
[25]Saito, T., ‘The dual knots of doubly primitive knots’, (preprint (2004)).Google Scholar
[26]Sakai, T., ‘Reidemeister torsion of a homology lens space’, Kobe J. Math. 1 (1984), 47–50.Google Scholar
[27]Silver, D. and Williams, S., ‘Mahler measure, links and and homology growth’, Topology 41 (2002), 979–991.CrossRefGoogle Scholar
[28]Tange, M., ‘Ozsváth Szabó's correction term and lens surgery’, (preprint (2005)).Google Scholar
[29]Turaev, V.G., ‘Reidemeister torsion in knot theory’, Russian Math. Surveys 41 (1986), 119–182.CrossRefGoogle Scholar
[30]Turaev, V.G., Introduction to combinatorial torsions Notes taken by Felix Schlenk, Lectures in Mathematics ETH Zürich (Birkhäuser Verlag, Basel, 2001).CrossRefGoogle Scholar
[31]Turaev, V.G., Torsions of 3-dimensional Manifolds, Progress in Mathematics 208 (Birkhäuser Verlag, Basel, 2002).CrossRefGoogle Scholar
[32]Washington, L.C., Introduction to cyclotomic fields, Graduate Texts in Mathematics 83 (Springer-Verlag, New York, 1982).CrossRefGoogle Scholar
[33]Yamada, Y., ‘Berge's knots in the fiber surfaces of genus one, lens spaces and framed links’, J. Knot Theory Ramifications 14 (2005), 177–188.CrossRefGoogle Scholar
[34]Yamada, Y., ‘Two equalities on the Alexander polynomial of the pretzel knot of type (-2, 3, 7)’, Bull. Univ. Electro-Comm. 18–1.2 (2006), 47–52.Google Scholar