No CrossRef data available.
Article contents
AN OPTIMAL LINEAR FILTER FOR ESTIMATION OF RANDOM FUNCTIONS IN HILBERT SPACE
Published online by Cambridge University Press: 07 January 2021
Abstract
Let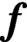 
$\boldsymbol{f}$
be a square-integrable, zero-mean, random vector with observable realizations in a Hilbert space H, and let 
$\boldsymbol{g}$
be an associated square-integrable, zero-mean, random vector with realizations which are not observable in a Hilbert space K. We seek an optimal filter in the form of a closed linear operator X acting on the observable realizations of a proximate vector 
$\boldsymbol{f}_{\epsilon } \approx \boldsymbol{f}$
that provides the best estimate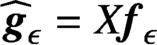 
$\widehat{\boldsymbol{g}}_{\epsilon} = X \boldsymbol{f}_{\epsilon}$
of the vector 
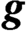$\boldsymbol{g}$
. We assume the required covariance operators are known. The results are illustrated with a typical example.
MSC classification
Primary:
49J55: Problems involving randomness
- Type
- Research Article
- Information
- Copyright
- © Australian Mathematical Society 2021
References
Albrecht, A., Howlett, P. and Verma, G., “The fundamental equations for the generalized resolvent of an elementary pencil in a unital Banach algebra”, Linear Algebra Appl. 574 (2019) 216–251; doi:10.1016/j.laa.2019.03.032.CrossRefGoogle Scholar
Albrecht, A., Howlett, P. and Verma, G., “Inversion of operator pencils on Banach space using Jordan chains when the generalized resolvent has an isolated essential singularity”, Linear Algebra Appl. 595 (2020) 33–62; doi:10 1016/j.laa.2020.02.030.CrossRefGoogle Scholar
Ash, M., “Neither a worst convergent series nor a best divergent series exists”, Coll. Math. J. 28 (1997) 296–297; doi:10.1080/07468342.1997.11973879.CrossRefGoogle Scholar
Avrachenkov, K. E., Filar, J. A. and Howlett, P. G., Analytic perturbation theory and its applications (SIAM, Philadelphia, PA, 2013).CrossRefGoogle Scholar
Balakrishnan, A. V., Applied functional analysis, Volume 3 of Appl. Math. (Springer, New York, 1976).Google Scholar
Cotter, S. L., Dashti, M., Robinson, J. C. and Stuart, A. M., “Bayesian inverse problems for functions and applications to fluid mechanics”, Inverse Probl. 25 (2009) 115008; doi:10.1088/0266-5611/25/11/115008.CrossRefGoogle Scholar
Dunford, N. and Schwartz, J. T., Linear operators, Part 1: General theory (Wiley, New York, 1988).Google Scholar
Engl, H. W. and Nashed, M. Z., “New extremal characterizations of generalized inverses of linear operators”, J. Math. Anal. Appl. 82 (1981) 566–586; doi:10.1016/0022-247X(81)90217-1.CrossRefGoogle Scholar
Fomin, V. N. and Ruzhansky, M. V., “Abstract optimal linear filtering”, SIAM J. Control Optim. 38 (2000) 1334–1352; doi:10.1137/S036301299834778X.CrossRefGoogle Scholar
Howlett, P. G., “Input retrieval in finite dimensional linear systems”, ANZIAM J. (formerly J. Austral. Math. Soc. Ser. B) 23 (1982) 357–382; doi:10.1017/S033427000000031X.Google Scholar
Howlett, P. G., Pearce, C. E. M. and Torokhti, A. P., “An optimal linear filter for random signals with realisations in a separable Hilbert space”, ANZIAM J. 44 (2003) 485–500; doi:10.1017/S1446181100012888.CrossRefGoogle Scholar
Hua, Y. and Liu, W. Q., “Generalized Karhunen–Loeve transform”, IEEE Signal Process. Lett. 5 (1998) 141–142; doi:10.1109/97.681430.Google Scholar
Nashed, M. Z., “Inner, outer and generalized inverses in Banach and Hilbert spaces”, Numer. Func. Anal. Opt. 9 (1987) 261–325; doi:10.1080/01630568708816235.CrossRefGoogle Scholar
Naylor, A. W. and Sell, G. R., Linear operator theory in engineering and science, Volume 40 of Appl. Math. Sci. (Springer-Verlag, New York, 1982).CrossRefGoogle Scholar
Sorenson, H. W., Parameter estimation, principles and problems (Marcel Dekker, New York, 1980).Google Scholar
Yamashita, Y. and Ogawa, H., “Relative Karhunen–Loeve transform”, IEEE Trans. Signal Process. 44 (1996) 371–378; doi:10.1109/78.485932.CrossRefGoogle Scholar
Yosida, K., Functional analysis, 5th edn, Classics Math. (Springer-Verlag, New York, 1978).CrossRefGoogle Scholar