Hostname: page-component-8448b6f56d-gtxcr Total loading time: 0 Render date: 2024-04-24T13:24:34.597Z Has data issue: false hasContentIssue false
  • English
  • Français

Parking functions: interdisciplinary connections

Part of: Combinatorics Combinatorial probability

Published online by Cambridge University Press:  17 January 2023

Mei Yin
Mei Yin*
Affiliation:
University of Denver
*
*Postal address: Department of Mathematics, University of Denver, Denver, CO 80208, USA. Email address: mei.yin@du.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

Suppose that m drivers each choose a preferred parking space in a linear car park with n spots. In order, each driver goes to their chosen spot and parks there if possible, and otherwise takes the next available spot if it exists. If all drivers park successfully, the sequence of choices is called a parking function. Classical parking functions correspond to the case $m=n$.

We investigate various probabilistic properties of a uniform parking function. Through a combinatorial construction termed a parking function multi-shuffle, we give a formula for the law of multiple coordinates in the generic situation 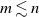$m \lesssim n$. We further deduce all possible covariances: between two coordinates, between a coordinate and an unattempted spot, and between two unattempted spots. This asymptotic scenario in the generic situation 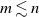$m \lesssim n$ is in sharp contrast with that of the special situation $m=n$.

A generalization of parking functions called interval parking functions is also studied, in which each driver is willing to park only in a fixed interval of spots. We construct a family of bijections between interval parking functions with n cars and n spots and edge-labeled spanning trees with 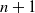$n+1$ vertices and a specified root.

Keywords

Parking functionmulti-shuffleasymptotic expansionAbel’s multinomial theoremedge-labeled spanning tree

MSC classification

Primary: 60C05: Combinatorial probability
Secondary: 05A16: Asymptotic enumeration 05A19: Combinatorial identities, bijective combinatorics
Type
Original Article
Information
Advances in Applied Probability , Volume 55 , Issue 3 , September 2023 , pp. 768 - 792
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Applied Probability Trust

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adeniran, A. et al. (2020). Enumerating parking completions using Join and Split. Electron. J. Combinatorics 27, paper no. 44, 19 pp.Google Scholar
Björner, A. and Brenti, F. (2005). Combinatorics of Coxeter Groups. Springer, New York.Google Scholar
Cameron, P. J., Johannsen, D., Prellberg, T. and Schweitzer, P. (2008). Counting defective parking functions. Electron. J. Combinatorics 15, paper no. 92, 15 pp.Google Scholar
Chassaing, P. and Marckert, J.-F. (2001). Parking functions, empirical processes, and the width of rooted labeled trees. Electron. J. Combinatorics 8, paper no. 14, 19 pp.Google Scholar
Colaric, E., DeMuse, R., Martin, J. L. and Yin, M. (2021). Interval parking functions. Adv. Appl. Math. 123, paper no. 102129.10.1016/j.aam.2020.102129CrossRefGoogle Scholar
Cori, R. and Rossin, D. (2000). On the sandpile group of dual graphs. Europ. J. Combinatorics 21, 447–459.10.1006/eujc.1999.0366CrossRefGoogle Scholar
Diaconis, P. and Hicks, A. (2017). Probabilizing parking functions. Adv. Appl. Math. 89, 125–155.10.1016/j.aam.2017.05.004CrossRefGoogle Scholar
Du Cloux, F. (1999). A transducer approach to Coxeter groups. J. Symbolic Comput. 27, 311–324.10.1006/jsco.1998.0254CrossRefGoogle Scholar
Ehrenborg, R. and Happ, A. (2018). Parking cars after a trailer. Australas. J. Combinatorics 70, 402–406.Google Scholar
Foata, D. and Riordan, J. (1974). Mappings of acyclic and parking functions. Aequat. Math. 10, 10–22.10.1007/BF01834776CrossRefGoogle Scholar
Gessel, I. M. and Seo, S. (2006). A refinement of Cayley’s formula for trees. Electron. J. Combinatorics 11, paper no. 27, 23 pp.Google Scholar
Haiman, M. D. (1994). Conjectures on the quotient ring by diagonal invariants. J. Algebraic Combin. 3, 17–76.10.1023/A:1022450120589CrossRefGoogle Scholar
Kenyon, R. and Yin, M. (2021). Parking functions: from combinatorics to probability. Preprint. Available at https://arxiv.org/abs/2103.17180.Google Scholar
Kung, J. P. S. and Yan, C. H. (2003). Expected sums of general parking functions. Ann. Combinatorics 7, 481–493.10.1007/s00026-003-0198-7CrossRefGoogle Scholar
Pitman, J. (2002). Forest volume decompositions and Abel–Cayley–Hurwitz multinomial expansions. J. Combinatorial Theory A 98, 175–191.10.1006/jcta.2001.3238CrossRefGoogle Scholar
Pitman, J. and Stanley, R. P. (2002). A polytope related to empirical distributions, plane trees, parking functions, and the associahedron. Discrete Comput. Geom. 27, 603–634.Google Scholar
Riordan, J. (1968). Combinatorial Identities. John Wiley, New York.Google Scholar
Stanley, R. P. (1997). Parking functions and noncrossing partitions. Electron. J. Combinatorics 4, paper no. 20, 14 pp.Google Scholar
Stanley, R. P. (1998). Hyperplane arrangements, parking functions and tree inversions. In Mathematical Essays in Honor of Gian-Carlo Rota, eds B. E. Sagan and R. P. Stanley, Birkhäuser, Boston, pp. 359–375.10.1007/978-1-4612-4108-9_19CrossRefGoogle Scholar
Yan, C. H. (2001). Generalized parking functions, tree inversions, and multicolored graphs. Adv. Appl. Math. 27, 641–670.10.1006/aama.2001.0754CrossRefGoogle Scholar
Yan, C. H. (2015). Parking functions. In Handbook of Enumerative Combinatorics, ed. M. Bóna, CRC Press, Boca Raton, pp. 835–893.Google Scholar